Hostname: page-component-848d4c4894-pftt2 Total loading time: 0 Render date: 2024-05-30T06:33:45.726Z Has data issue: false hasContentIssue false
  • English
  • Français

DIVISIBILITY OF SUMS OF PARTITION NUMBERS BY MULTIPLES OF 2 AND 3

Part of: Combinatorics Additive number theory; partitions

Published online by Cambridge University Press:  22 December 2023

NAYANDEEP DEKA BARUAH Open the ORCID record for NAYANDEEP DEKA BARUAH [Opens in a new window]
NAYANDEEP DEKA BARUAH*
Affiliation:
Department of Mathematical Sciences, Tezpur University, Napaam 784028, Assam, India
*
e-mail: nayan@tezu.ernet.in
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that certain sums of partition numbers are divisible by multiples of 2 and 3. For example, if 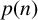$p(n)$ denotes the number of unrestricted partitions of a positive integer n (and 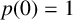$p(0)=1$, $p(n)=0$ for $n<0$), then for all nonnegative integers m,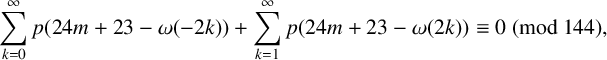

$$ \begin{align*}\sum_{k=0}^\infty p(24m+23-\omega(-2k))+\sum_{k=1}^\infty p(24m+23-\omega(2k))\equiv 0~ (\text{mod}~144),\end{align*} $$

where 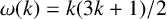$\omega (k)=k(3k+1)/2$.

Keywords

partition functionsum of partition numbersℓ-regular overpartitionssingular overpartitions

MSC classification

Primary: 11P81: Elementary theory of partitions
Secondary: 05A17: Partitions of integers 11P83: Partitions; congruences and congruential restrictions 05A19: Combinatorial identities, bijective combinatorics
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , First View , pp. 1 - 9
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlgren, S., ‘Distribution of the partition function modulo composite integers $M$ ’, Math. Ann. 318 (2000), 795–803.Google Scholar
Ahmed, Z. and Baruah, N. D., ‘New congruences for Andrews’ singular overpartitions’, Int. J. Number Theory 11 (2015), 2247–2264.Google Scholar
Andrews, G. E., The Theory of Partitions (Addison-Wesley, Reading, MA, 1976).Google Scholar
Andrews, G. E., ‘Singular overpartitions’, Int. J. Number Theory 11 (2015), 1523–1533.Google Scholar
Ballantine, C. and Merca, M., ‘Parity of sums of partition numbers and squares in arithmetic progressions’, Ramanujan J. 44 (2017), 617–630.Google Scholar
Barman, R. and Ray, C., ‘Congruences for $\ell$ -regular overpartitions and Andrews’ singular overpartitions’, Ramanujan J. 45 (2018), 497–515.Google Scholar
Barman, R. and Ray, C., ‘Divisibility of Andrews’ singular overpartitions by powers of 2 and 3’, Res. Number Theory 5 (2019), Article no. 22.Google Scholar
Chen, S.-C., Hirschhorn, M. D. and Sellers, J. A., ‘Arithmetic properties of Andrews’ singular overpartitions’, Int. J. Number Theory 11 (2015), 1463–1476.Google Scholar
Chern, S., ‘New congruences for $\ell$ -regular overpartitions’, Integers 17 (2017), Article no. A22.Google Scholar
Corteel, S. and Lovejoy, J., ‘Overpartitions’, Trans. Amer. Math. Soc. 356 (2004), 1623–1635.Google Scholar
Hao, R. X. J. and Shen, E. Y. Y., ‘On the number of $l$ -regular overpartitions’, Int. J. Number Theory 17 (2021), 2153–2173.Google Scholar
Hong, L. and Zhang, S., ‘Proof of the Ballantine–Merca conjecture and theta function identities modulo 2’, Proc. Amer. Math. Soc., to appear.Google Scholar
Hu, W., Yao, O. X. M. and Zhao, T., ‘New parity results of sums of partitions and squares in arithmetic progressions’, Contrib. Discrete Math. 14 (2019), 117–129.Google Scholar
Li, X. and Yao, O. X. M., ‘New infinite families of congruences for Andrews’ $\left(k,i\right)$ -singular overpartitions’, Quaest. Math. 41 (2018), 1005–1019.Google Scholar
Lovejoy, J., ‘Gordon’s theorem for overpartitions’, J. Combin. Theory Ser. A 103 (2003), 393–401.Google Scholar
Mahadeva Naika, M. S. and Gireesh, D. S., ‘Congruences for Andrews’ singular overpartitions’, J. Number Theory 165 (2016), 109–130.Google Scholar
Ono, K., ‘On the parity of the partition function in arithmetic progressions’, J. reine angew. Math. 472 (1996), 1–15.Google Scholar
Ono, K., ‘Distribution of the partition function modulo $m$ ’, Ann. Math. 151 (2000), 293–307.Google Scholar
Radu, C.-S., ‘A proof of Subbarao’s conjecture’, J. reine angew. Math. 672 (2012), 161–175.Google Scholar
Ramanujan, S., ‘Some properties of $p(n)$ , the number of partitions of $n$ ’, Proc. Cambridge Philos. Soc. 19 (1919), 207–210.Google Scholar
Ramanujan, S., Collected Papers of Srinivasa Ramanujan (Cambridge University Press, Cambridge, 1927); reprinted by Chelsea, New York, 1962, and by the American Mathematical Society, Providence, RI, 2000.Google Scholar
Shen, E. Y. Y., ‘Arithmetic properties of $l$ -regular overpartitions’, Int. J. Number Theory 12 (2016), 841–852.Google Scholar
Shen, E. Y. Y., ‘Congruences modulo 9 for singular overpartitions’, Int. J. Number Theory 13 (2017), 717–724.Google Scholar
Subbarao, M. V., ‘Remarks on the partition function’, Amer. Math. Monthly 73 (1966), 851–854.Google Scholar
Sumanth Bharadwaj, H. S., Hemanthkumar, B. and Mahadeva Naika, M. S., ‘On 3- and 9-regular overpartitions modulo powers of 3’, Colloq. Math. 154 (2018), 121–130.Google Scholar
Yao, O. X. M., ‘Congruences modulo 16, 32, and 64 for Andrews’s singular overpartitions’, Ramanujan J. 43 (2017), 215–228.Google Scholar