Hostname: page-component-848d4c4894-pjpqr Total loading time: 0 Render date: 2024-06-15T17:30:51.942Z Has data issue: false hasContentIssue false
  • English
  • Français

The structure of sidewall boundary layers in confined rotating Rayleigh–Bénard convection

Published online by Cambridge University Press:  27 June 2013

R. P. J. Kunnen ,
H. J. H. Clercx  and
G. J. F. van Heijst
R. P. J. Kunnen*
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Centre for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands
H. J. H. Clercx
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Centre for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands Department of Applied Mathematics, University of Twente, P.O. Box 217, 7500 AE Enschede, The Netherlands
G. J. F. van Heijst
Affiliation:
Fluid Dynamics Laboratory, Department of Applied Physics and J. M. Burgers Centre for Fluid Dynamics, Eindhoven University of Technology, P.O. Box 513, 5600 MB Eindhoven, The Netherlands
*
†Email address for correspondence: r.p.j.kunnen@tue.nl
Get access Rights & Permissions [Opens in a new window]

Abstract

Turbulent rotating convection is usually studied in a cylindrical geometry, as this is its most convenient experimental realization. In our previous work (Kunnen et al., J. Fluid Mech., vol. 688, 2011, pp. 422–442) we studied turbulent rotating convection in a cylinder with the emphasis on the boundary layers. A secondary circulation with a convoluted spatial structure has been observed in mean velocity plots. Here we present a linear boundary-layer analysis of this flow, which leads to a model of the circulation. The model consists of two independent parts: an internal recirculation within the sidewall boundary layer, and a bulk-driven domain-filling circulation. 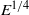Both contributions exhibit the typical structure of the Stewartson boundary layer near the sidewall: a sandwich structure of two boundary layers of typical thicknesses ${E}^{1/ 4} $ and 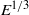${E}^{1/ 3} $, where 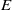$E$ is the Ekman number. Although the structure of the bulk-driven circulation may change considerably depending on the Ekman number, the boundary-layer recirculation is present at all Ekman numbers in the range 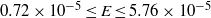$0. 72\times 1{0}^{- 5} \leq E\leq 5. 76\times 1{0}^{- 5} $ considered here.

JFM classification

Boundary Layers: Boundary layer structure Geophysical and Geological Flows: Rotating flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 727 , 25 July 2013 , pp. 509 - 532
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.Google Scholar
Barcilon, V. & Pedlosky, J. 1967a Linear theory of rotating stratified fluid flows. J. Fluid Mech. 29, 1–16.Google Scholar
Barcilon, V. & Pedlosky, J. 1967b A unified linear theory of homogeneous and stratified rotating fluids. J. Fluid Mech. 29, 609–621.Google Scholar
Benton, E. R. & Clark, A. 1974 Spin-up. Annu. Rev. Fluid Mech. 6, 257–280.Google Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Oxford University Press.Google Scholar
Duck, P. W. & Foster, M. R. 2001 Spin-up of homogeneous and stratified fluids. Annu. Rev. Fluid Mech. 33, 231–263.Google Scholar
Ecke, R. E. & Liu, Y. 1998 Traveling-wave and vortex states in rotating Rayleigh–Bénard convection. Intl J. Engng Sci. 36, 1471–1480.CrossRefGoogle Scholar
Greenspan, H. P. 1968 The Theory of Rotating Fluids. Cambridge University Press.Google Scholar
Greenspan, H. P. & Howard, L. N. 1963 On a time-dependent motion of a rotating fluid. J. Fluid Mech. 17, 385–404.Google Scholar
Hart, J. E., Kittelman, S. & Ohlsen, D. R. 2002 Mean flow precession and temperature probability density functions in turbulent rotating convection. Phys. Fluids 14, 955–962.Google Scholar
Hart, J. E. & Ohlsen, D. R. 1999 On the thermal offset in turbulent rotating convection. Phys. Fluids 11, 2101–2107.CrossRefGoogle Scholar
van Heijst, G. J. F. 1983 The shear-layer structure in a rotating fluid near a differentially rotating sidewall. J. Fluid Mech. 130, 1–12.CrossRefGoogle Scholar
van Heijst, G. J. F. 1986 Fluid flow in a partially-filled rotating cylinder. J. Engng Maths 20, 233–250.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2008a Breakdown of large-scale circulation in turbulent rotating convection. Europhys. Lett. 84, 24001.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2008b Enhanced vertical inhomogeneity in turbulent rotating convection. Phys. Rev. Lett. 101, 174501.Google Scholar
Kunnen, R. P. J., Geurts, B. J. & Clercx, H. J. H. 2010a Experimental and numerical investigation of turbulent rotating convection. J. Fluid Mech. 642, 445–476.CrossRefGoogle Scholar
Kunnen, R. P. J., Geurts, B. J. & Clercx, H. J. H. 2010b Vortex statistics in turbulent rotating convection. Phys. Rev. E 80, 036314.Google Scholar
Kunnen, R. P. J., Stevens, R. J. A. M., Overkamp, J., Sun, C., van Heijst, G. J. F. & Clercx, H. J. H. 2011 The role of Stewartson and Ekman layers in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 422–442.CrossRefGoogle Scholar
Lighthill, M. J. 1968 Introduction to Fourier Analysis and Generalized Functions. Cambridge University Press.Google Scholar
Marshall, J. & Schott, F. 1999 Open-ocean convection: observations, theory, and models. Rev. Geophys. 37, 1–64.Google Scholar
Miesch, M. S. 2000 The coupling of solar convection and rotation. Solar Phys. 192, 59–89.Google Scholar
Moore, D. W. & Saffman, P. G. 1969 The structure of free vertical shear layers in a rotating fluid and the motion produced by a slowly rising body. Phil. Trans. R. Soc. A 264, 597–634.Google Scholar
Niemela, J. J., Babuin, S. & Sreenivasan, K. R. 2010 Turbulent rotating convection at high Rayleigh and Taylor numbers. J. Fluid Mech. 649, 509–522.Google Scholar
Proudman, I. 1956 The almost-rigid rotation of viscous flow between concentric spheres. J. Fluid Mech. 1, 505–516.Google Scholar
Rossby, H. T. 1969 A study of Bénard convection with and without rotation. J. Fluid Mech. 36, 309–335.Google Scholar
van Santen, H., Kleijn, C. R. & van den Akker, H. E. A. 2000 On turbulent flows in cold-wall CVD reactors. J. Cryst. Growth 212, 299–310.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2010 Optimal Prandtl number for heat transfer in rotating Rayleigh–Bénard convection. New J. Phys. 12, 075005.CrossRefGoogle Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2012 Breakdown of the large-scale circulation in $\gamma = 1/ 2$ rotating Rayleigh–Bénard flow. Phys. Rev. E 86, 056311.Google Scholar
Stevens, R. J. A. M., Overkamp, J., Lohse, D. & Clercx, H. J. H. 2011 Effect of aspect ratio on vortex distribution and heat transfer in rotating Rayleigh–Bénard convection. Phys. Rev. E 84, 056313.Google Scholar
Stewartson, K. 1957 On almost rigid rotations. J. Fluid Mech. 3, 17–26.CrossRefGoogle Scholar
Vorobieff, P. & Ecke, R. E. 1998a Transient states during spin-up of a Rayleigh–Bénard cell. Phys. Fluids 10, 2525–2538.CrossRefGoogle Scholar
Vorobieff, P. & Ecke, R. E. 1998b Vortex structure in rotating Rayleigh–Bénard convection. Physica D 123, 153–160.Google Scholar
Vorobieff, P. & Ecke, R. E. 2002 Turbulent rotating convection: an experimental study. J. Fluid Mech. 458, 191–218.CrossRefGoogle Scholar
Weiss, S. & Ahlers, G. 2011a Heat transport by turbulent rotating Rayleigh–Bénard convection and its dependence on the aspect ratio. J. Fluid Mech. 684, 407–426.Google Scholar
Weiss, S. & Ahlers, G. 2011b The large-scale flow structure in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 461–492.Google Scholar
Weiss, S., Stevens, R. J. A. M., Zhong, J.-Q., Clercx, H. J. H., Lohse, D. & Ahlers, G. 2010 Finite-size effects lead to supercritical bifurcations in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 105, 224501.Google Scholar
Zhong, F., Ecke, R. E. & Steinberg, V. 1991 Asymmetric modes and the transition to vortex structures in rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 67, 2473–2476.CrossRefGoogle ScholarPubMed
Zhong, F., Ecke, R. E. & Steinberg, V. 1993 Rotating Rayleigh–Bénard convection: asymmetric modes and vortex states. J. Fluid Mech. 249, 135–159.Google Scholar
Zhong, J.-Q. & Ahlers, G. 2010 Heat transport and the large-scale circulation in rotating turbulent Rayleigh–Bénard convection. J. Fluid Mech. 665, 300–333.Google Scholar
Zhong, J.-Q., Stevens, R. J. A. M., Clercx, H. J. H., Verzicco, R., Lohse, D. & Ahlers, G. 2009 Prandtl-, Rayleigh-, and Rossby-number dependence of heat transport in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 102, 044502.CrossRefGoogle ScholarPubMed