Hostname: page-component-848d4c4894-nmvwc Total loading time: 0 Render date: 2024-06-16T23:06:08.118Z Has data issue: false hasContentIssue false
  • English
  • Français

THE DIAGONAL CYCLE EULER SYSTEM FOR 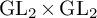$\mathrm {GL}_2\times \mathrm {GL}_2$

Part of: Arithmetic problems. Diophantine geometry Algebraic number theory: global fields Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  13 June 2023

Raúl Alonso Open the ORCID record for Raúl Alonso [Opens in a new window] ,
Francesc Castella Open the ORCID record for Francesc Castella [Opens in a new window]  and
Óscar Rivero Open the ORCID record for Óscar Rivero [Opens in a new window]
Raúl Alonso
Affiliation:
Department of Mathematics, Princeton University, Fine Hall, Princeton, NJ 08544-1000, USA (raular@math.princeton.edu)
Francesc Castella
Affiliation:
Department of Mathematics, University of California, Santa Barbara, CA 93106, USA (castella@ucsb.edu)
Óscar Rivero*
Affiliation:
Mathematics Institute, Zeeman Building, University of Warwick, Coventry CV4 7AL, UK
*
Oscar.Rivero-Salgado@warwick.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

We construct an anticyclotomic Euler system for the Rankin–Selberg convolutions of two modular forms, using p-adic families of generalised Gross–Kudla–Schoen diagonal cycles. As applications of this construction, we prove new results on the Bloch–Kato conjecture in analytic ranks zero and one, and a divisibility towards an Iwasawa main conjecture.

Keywords

Euler systemsdiagonal cyclesp-adic families of modular formsBloch–Kato conjectureIwasawa theory

MSC classification

Primary: 11R23: Iwasawa theory
Secondary: 11F85: $p$-adic theory, local fields 14G35: Modular and Shimura varieties
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , First View , pp. 1 - 63
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alonso, R., Castella, F. and Rivero, Ó., ‘An anticyclotomic Euler system for adjoint modular Galois representations’, to appear in Ann. Inst. Fourier (Grénoble). Accepted in 2023. https://arxiv.org/abs/2204.07658.Google Scholar
Agboola, A. and Howard, B., Anticyclotomic Iwasawa theory of CM elliptic curves, Ann. Inst. Fourier (Grenoble) 56(4) (2006), 1001–1048.CrossRefGoogle Scholar
Ash, A. and Stevens, G., Cohomology of arithmetic groups and congruences between systems of Hecke eigenvalues, J. Reine Angew. Math. 365 (1986), 192–220.Google Scholar
Ash, A. and Stevens, G., Modular forms in characteristic $l$ and special values of their $L$ -functions, Duke Math. J. 53(3) (1986), 849–868.CrossRefGoogle Scholar
Bertolini, M., Darmon, H. and Rotger, V., Beilinson-Flach elements and Euler systems I: Syntomic regulators and $p$ -adic Rankin $L$ -series, J. Algebraic Geom. 24(2) (2015), 355–378.CrossRefGoogle Scholar
Bertolini, M., Darmon, H. and Rotger, V., Beilinson-Flach elements and Euler systems II: The Birch-Swinnerton-Dyer conjecture for Hasse-Weil-Artin $L$ -series, J. Algebraic Geom. 24(3) (2015), 569–604.CrossRefGoogle Scholar
Beilinson, A. A., Higher regulators and values of $L$ -functions, in Current Problems in Mathematics, vol. 24, pp. 181–238 (Itogi Nauki i Tekhniki, Akad. Nauk SSSR, Vsesoyuz. Inst. Nauchn. i Tekhn. Inform., Moscow, 1984).Google Scholar
Bloch, S. and Kato, K., $\mathrm{L}$ -functions and Tamagawa numbers of motives, in The Grothendieck Festschrift, vol. I, Progress in Mathematics, vol. 86, pp. 333–400 (Birkhäuser Boston, Boston, MA, 1990).Google Scholar
Büyükboduk, K. and Lei, A., Anticyclotomic $p$ -ordinary Iwasawa theory of elliptic modular forms, Forum Math. 30(4) (2018), 887–913.CrossRefGoogle Scholar
Büyükboduk, K. and Lei, A., Iwasawa theory of elliptic modular forms over imaginary quadratic fields at non-ordinary primes, Int. Math. Res. Not. IMRN 2021(14) (2021), 10654–10730.CrossRefGoogle Scholar
Büyükboduk, K., Lei, A., Loeffler, D. and Venkat, G., Iwasawa theory for Rankin-Selberg products of $p$ -nonordinary eigenforms, Algebra Number Theory 13(4) (2019), 901–941.CrossRefGoogle Scholar
Bertolini, M., Seveso, M. A. and Venerucci, R., Reciprocity laws for balanced diagonal classes, Astérisque (434) (2022), 77–174.Google Scholar
Castella, F., $p$ -adic heights of Heegner points and Beilinson-Flach classes, J. Lond. Math. Soc. (2) 96(1) (2017), 156–180.CrossRefGoogle Scholar
Castella, F. and Do, K. T., Diagonal cycles and anticyclotomic Iwasawa theory of modular forms, Preprint, 2023, arXiv:2303.06751.Google Scholar
Do, K. T., Construction of an anticyclotomic Euler system with applications, Ph.D. thesis, Princeton University, 2022.Google Scholar
Darmon, H. and Rotger, V., Diagonal cycles and Euler systems I: A $p$ -adic Gross-Zagier formula, Ann. Sci. Éc. Norm. Supér. 47(4) (2014), 779–832.CrossRefGoogle Scholar
Darmon, H. and Rotger, V., Diagonal cycles and Euler systems II: The Birch and Swinnerton-Dyer conjecture for Hasse-Weil-Artin $L$ -functions, J. Amer. Math. Soc. 30(3) (2017), 601–672.CrossRefGoogle Scholar
Darmon, H. and Rotger, V., $p$ -adic families of diagonal cycles, Astérisque (434) (2022), 29–75.Google Scholar
Flach, M., A finiteness theorem for the symmetric square of an elliptic curve, Invent. Math. 109(2) (1992), 307–327.CrossRefGoogle Scholar
Gross, B. H. and Kudla, S. S., Heights and the central critical values of triple product $L$ -functions, Compositio Math. 81(2) (1992), 143–209.Google Scholar
Greenberg, R., Iwasawa theory and $p$ -adic deformations of motives, in Motives (Seattle, WA, 1991), Proceedings of Symposia in Pure Mathematics vol. 55, pp. 193–223 (American Mathematical Society, Providence, RI, 1994).Google Scholar
Greenberg, R. and Stevens, G., $p$ -adic $L$ -functions and $p$ -adic periods of modular forms, Invent. Math. 111(2) (1993), 407–447.CrossRefGoogle Scholar
Gross, B. H. and Schoen, C., The modified diagonal cycle on the triple product of a pointed curve, Ann. Inst. Fourier (Grenoble) 45(3) (1995), 649–679.CrossRefGoogle Scholar
Greenberg, M. and Seveso, M. A., Triple product $p$ -adic $L$ -functions for balanced weights, Math. Ann. 376(1–2) (2020), 103–176.CrossRefGoogle Scholar
Harris, M. and Kudla, S. S., The central critical value of a triple product $L$ -function, Ann. of Math. (2) 133(3) (1991), 605–672.CrossRefGoogle Scholar
Howard, B., The Heegner point Kolyvagin system, Compos. Math. 140(6) (2004), 1439–1472.CrossRefGoogle Scholar
Howard, B., Variation of Heegner points in Hida families, Invent. Math. 167(1) (2007), 91–128.CrossRefGoogle Scholar
Hsieh, M.-L., Hida families and $p$ -adic triple product $L$ -functions, Am. J. Math. 143(2) (2021), 411–532.CrossRefGoogle Scholar
Harris, M. and Tilouine, J., $p$ -adic measures and square roots of special values of triple product $L$ -functions, Math. Ann. 320(1) (2001), 127–147.CrossRefGoogle Scholar
Hsieh, M.-L. and Yamana, S., Derivatives of cyclotomic triple product $L$ -functions and $p$ -adic heights of diagonal cycles, in preparation.Google Scholar
Jannsen, U., Continuous Étale Cohomology. Math. Annal. 280(2), (1988), 207–246.CrossRefGoogle Scholar
Jetchev, D., Nekovář, J. and Skinner, C., preprint.Google Scholar
Kato, K., $p$ -adic Hodge theory and values of zeta functions of modular forms, in Cohomologies $p$ -adiques et applications arithmétiques. III. Astérisque 295 pp. ix, 117–290 (Societe Mathematique de France, Paris, 2004). http://www.numdam.org/item/AST_2004__295__117_0/ Google Scholar
Kings, G., Loeffler, D. and Zerbes, S. L., Rankin-Eisenstein classes and explicit reciprocity laws, Camb. J. Math. 5(1) (2017), 1–122.CrossRefGoogle Scholar
Kings, G., Loeffler, D. and Zerbes, S. L., Rankin-Eisenstein classes for modular forms, Amer. J. Math. 142(1) (2020), 79–138.CrossRefGoogle Scholar
Lei, A., Loeffler, D. and Zerbes, S. L., Euler systems for Rankin-Selberg convolutions of modular forms, Ann. of Math. (2) 180(2) (2014), 653–771.CrossRefGoogle Scholar
Lei, A., Loeffler, D. and Zerbes, S. L., Euler systems for modular forms over imaginary quadratic fields, Compos. Math. 151(9) (2015), 1585–1625.CrossRefGoogle Scholar
Loeffler, D., Images of adelic Galois representations for modular forms, Glasg. Math. J. 59(1) (2017), 11–25.CrossRefGoogle Scholar
Loeffler, D. and Zerbes, S. L., Iwasawa theory and $p$ -adic $L$ -functions over ${\mathbb{Z}}_p^2$ -extensions, Int. J. Number Theory 10(8) (2014), 2045–2095.CrossRefGoogle Scholar
Mazur, B. and Rubin, K., Kolyvagin systems, Mem. Amer. Math. Soc. 168(799) (2004), viii+96.Google Scholar
Nekovář, J., On $p$ -adic height pairings, in Séminaire de Théorie des Nombres, Paris, 1990–91, Progress in Mathematics vol. 108, pp. 127–202 (Birkhäuser Boston, Boston, MA, 1993).Google Scholar
Nekovář, J. and Nizioł, W., Syntomic cohomology and $p$ -adic regulators for varieties over $p$ -adic fields, Algebra Number Theory 10(8) (2016), 1695–1790.CrossRefGoogle Scholar
Perrin-Riou, B., Fonctions $Lp$ -adiques, théorie d’Iwasawa et points de Heegner, Bull. Soc. Math. France 115(4) (1987), 399–456.CrossRefGoogle Scholar
Perrin-Riou, B., $p$ -adic $L$ -functions and $p$ -adic representations, in SMF/AMS Texts and Monographs vol. 3 (American Mathematical Society, Providence, RI, 2000). Translated from the 1995 French original by Leila Schneps and revised by the author.Google Scholar
Pollack, R. and Weston, T., On anticyclotomic $\mu$ -invariants of modular forms, Compos. Math. 147(5) (2011), 1353–1381.CrossRefGoogle Scholar
Rubin, K., Euler systems, in Hermann Weyl lectures, The Institute for Advanced Study, Annals of Mathematics Studies vol. 147 (Princeton University Press, Princeton, NJ, 2000).Google Scholar
Wan, X., Heegner point Kolyvagin system and Iwasawa main conjecture, Acta Math. Sin. 37(1) (2020), 104–120.CrossRefGoogle Scholar
Yuan, X., Zhang, S. and Zhang, W., Triple product $L$ -series and Gross–Kudla–Schoen cycles, Preprint, https://math.mit.edu/~wz2113/math/online/triple.pdf.Google Scholar