Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
LUKKARINEN, JANI
and
PAKKANEN, MIKKO S.
2013.
ON THE POSITIVITY OF RIEMANN–STIELTJES INTEGRALS.
Bulletin of the Australian Mathematical Society,
Vol. 87,
Issue. 3,
p.
400.
Ahlgren, Scott
and
Andersen, Nickolas
2016.
Kloosterman Sums and Maass Cusp Forms of Half Integral Weight for the Modular Group.
International Mathematics Research Notices,
p.
rnw234.




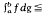 [inf
[inf 