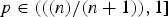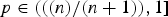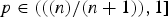Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Liu, Honghai
and
Wang, Hanbin
2020.
VARIATIONAL INEQUALITIES FOR BILINEAR AVERAGES.
Mathematika,
Vol. 66,
Issue. 3,
p.
622.
Wen, Yongming
and
Hou, Xianming
2021.
The boundedness of variation associated with the commutators of approximate identities.
Journal of Inequalities and Applications,
Vol. 2021,
Issue. 1,
Guo, Weichao
Wen, Yongming
Wu, Huoxiong
and
Yang, Dongyong
2022.
Variational characterizations of weighted Hardy spaces and weighted spaces.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 152,
Issue. 6,
p.
1613.
Demir, S.
2024.
Variation and λ-jump inequalities on H<sup>p</sup> spaces.
Izvestiya Vysshikh Uchebnykh Zavedenii. Matematika,
p.
15.
Zhang, Chao
2024.
Boundedness of Differential Transforms for Poisson Semigroups Generated by Bessel Operators.
The Quarterly Journal of Mathematics,
Vol. 75,
Issue. 1,
p.
277.