We show that for all 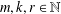$m,k,r\in \mathbb{N}$, there is an 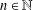$n\in \mathbb{N}$ such that whenever 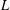$L$ is a Latin square of order $m$ and the Cartesian product 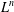$L^{n}$ of $n$ copies of 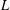$L$ is $r$-coloured, there is a monochrome Latin subsquare of 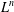$L^{n}$, isotopic to 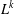$L^{k}$. In particular, for every prime 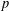$p$ and for all 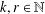$k,r\in \mathbb{N}$, there is an $n\in \mathbb{N}$ such that whenever the multiplication table 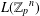$L({\mathbb{Z}_{p}}^{n})$ of the group 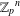${\mathbb{Z}_{p}}^{n}$ is $r$-coloured, there is a monochrome Latin subsquare of order 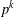$p^{k}$. On the other hand, we show that for every group $G$ of order $\leq 15$, there is a 2-colouring of $L(G)$ without a nontrivial monochrome Latin subsquare.