For a compact abelian group G, a corner in G × G is a triple of points (x, y), (x, y+d), (x+d, y). The classical corners theorem of Ajtai and Szemerédi implies that for every α > 0, there is some δ > 0 such that every subset A ⊂ G × G of density α contains a δ fraction of all corners in G × G, as x, y, d range over G.

Recently, Mandache proved a “popular differences” version of this result in the finite field case $G = {\mathbb{F}}_p^n$, showing that for any subset A ⊂ G × G of density α, one can fix d ≠ 0 such that A contains a large fraction, now known to be approximately α4, of all corners with difference d, as x, y vary over G. We generalise Mandache’s result to all compact abelian groups G, as well as the case of corners in 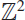$\mathbb{Z}^2$.