Hostname: page-component-8448b6f56d-m8qmq Total loading time: 0 Render date: 2024-04-19T06:24:47.126Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiplicity and concentration results for a fractional Schrödinger-Poisson type equation with magnetic field

Part of: Miscellaneous topics - Partial differential equations Partial differential equations Variational problems in infinite-dimensional spaces

Published online by Cambridge University Press:  23 January 2019

Vincenzo Ambrosio
Vincenzo Ambrosio*
Affiliation:
Dipartimento di Ingegneria Industriale e Scienze Matematiche, Università Politecnica delle Marche, via Brecce Bianche 12, 60131Ancona, Italy (vincenzo.ambrosio2@unina.it)
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper is devoted to the study of fractional Schrödinger-Poisson type equations with magnetic field of the type

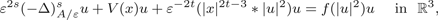$$\varepsilon^{2s}(-\Delta)_{A/\varepsilon}^{s}u + V(x)u + {\rm e}^{-2t}(\vert x \vert^{2t-3} \ast \vert u\vert ^{2})u = f(\vert u \vert^{2})u \quad \hbox{in} \ \open{R}^{3},$$
where ε > 0 is a parameter, s, t ∈ (0, 1) are such that 2s+2t>3, A:ℝ3 → ℝ3 is a smooth magnetic potential, (−Δ)As is the fractional magnetic Laplacian, V:ℝ3 → ℝ is a continuous electric potential and f:ℝ → ℝ is a C1 subcritical nonlinear term. Using variational methods, we obtain the existence, multiplicity and concentration of nontrivial solutions for e > 0 small enough.

Keywords

Fractional magnetic operatorsNehari manifoldLjusternick-Schnirelmann theory

MSC classification

Primary: 35A15: Variational methods
Secondary: 35R11: Fractional partial differential equations 35S05: Pseudodifferential operators 58E05: Abstract critical point theory (Morse theory, Ljusternik-Schnirelman (Lyusternik-Shnirel'man) theory, etc.)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 2 , April 2020 , pp. 655 - 694
Copyright
Copyright © Royal Society of Edinburgh 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Ackermann, N.. On a periodic Schrödinger equation with nonlocal superlinear part. Math. Z. 248 (2004), 423–443.CrossRefGoogle Scholar
2Alves, C. O. and Miyagaki, O. H.. Existence and concentration of solution for a class of fractional elliptic equation in ℝN via penalization method. Calc. Var. Partial Differ. Equ. 55 (2016), 19, art. 47.CrossRefGoogle Scholar
3Alves, C. O., Figueiredo, G. M. and Furtado, M. F.. Multiple solutions for a nonlinear Schrödinger equation with magnetic fields. Comm. Partial Differ. Equ. 36 (2011), 1565–1586.CrossRefGoogle Scholar
4Alves, C. O., Figueiredo, G. M. and Yang, M.. Multiple semiclassical solutions for a nonlinear Choquard equation with magnetic field. Asymptot. Anal. 96 (2016), 135–159.CrossRefGoogle Scholar
5Ambrosetti, A.. On Schrödinger-Poisson systems. Milan J. Math. 76 (2008), 257–274.CrossRefGoogle Scholar
6Ambrosetti, A. and Rabinowitz, P. H.. Dual variational methods in critical point theory and applications. J. Funct. Anal. 14 (1973), 349–381.CrossRefGoogle Scholar
7Ambrosio, V.. Multiplicity of positive solutions for a class of fractional Schrödinger equations via penalization method. Ann. Mat. Pura Appl. (4) 196 (2017), 2043–2062.CrossRefGoogle Scholar
8Ambrosio, V.. Concentrating solutions for a class of nonlinear fractional Schrödinger equations in ℝN, to appear in Rev. Mat. Iberoam., arXiv:1612.02388.Google Scholar
9Ambrosio, V.. Concentration phenomena for a fractional Choquard equation with magnetic field, to appear in Dyn. Partial Differ. Equ.Google Scholar
10Ambrosio, V.. Boundedness and decay of solutions for some fractional magnetic Schrödinger equations in ℝN. Milan J. Math. 86 (2018), no. 2, 125–136.CrossRefGoogle Scholar
11Ambrosio, V. and d'Avenia, P.. Nonlinear fractional magnetic Schrödinger equation: existence and multiplicity. J. Differ. Equ. 264 (2018), 3336–3368.CrossRefGoogle Scholar
12Arioli, G. and Szulkin, A.. A semilinear Schrödinger equation in the presence of a magnetic field. Arch. Ration. Mech. Anal. 170 (2003), 277–295.CrossRefGoogle Scholar
13Azzollini, A., d'Avenia, P. and Pomponio, A.. On the Schrödinger-Maxwell equations under the effect of a general nonlinear term. Ann. Inst. H. Poincaré Anal. Non Linéaire 27 (2010), 779–791.CrossRefGoogle Scholar
14Benci, V. and Cerami, G.. Multiple positive solutions of some elliptic problems via the Morse theory and the domain topology. Calc. Var. Partial Differ. Equ. 2 (1994), 29–48.CrossRefGoogle Scholar
15Benci, V. and Fortunato, D.. An eigenvalue problem for the Schrödinger-Maxwell equations. Topol. Methods Nonlinear Anal. 11 (1998), 283–293.CrossRefGoogle Scholar
16Benguria, R., Brezis, H. and Lieb, E. H.. The Thomas-Fermi-von Weizsäker theory of atoms and molecules. Comm. Math. Phys. 79 (1981), 167–180.CrossRefGoogle Scholar
17Brézis, H. and Lieb, E.. A relation between pointwise convergence of functions and convergence of functionals. Proc. Amer. Math. Soc. 88 (1983), 486–490.CrossRefGoogle Scholar
18Cingolani, S.. Semiclassical stationary states of nonlinear Schrödinger equations with an external magnetic field. J. Differ. Equ. 188 (2003), 52–79.CrossRefGoogle Scholar
19Cingolani, S. and Lazzo, M.. Multiple semiclassical standing waves for a class of nonlinear Schrödinger equations. Topol. Methods Nonlinear Anal. 10 (1997), 1–13.CrossRefGoogle Scholar
20Cingolani, S. and Secchi, S.. Semiclassical states for NLS equations with magnetic potentials having polynomial growths. J. Math. Phys. 46 (2005), 19.CrossRefGoogle Scholar
21Cingolani, S., Clapp, M. and Secchi, S.. Multiple solutions to a magnetic nonlinear Choquard equation. Z. Angew. Math. Phys. 63 (2012), 233–248.CrossRefGoogle Scholar
22D'Aprile, T. and Wei, J.. On bound states concentrating on spheres for the Maxwell-Schrödinger equation. SIAM J. Math. Anal. 37 (2005), 321–342.CrossRefGoogle Scholar
23Dávila, J., del Pino, M. and Wei, J.. Concentrating standing waves for the fractional nonlinear Schrödinger equation. J. Differ. Equ. 256 (2014), 858–892.CrossRefGoogle Scholar
24d'Avenia, P. and Squassina, M.. Ground states for fractional magnetic operators, to appear in ESAIM Control Optim. Calc. Var., doi: 10.1051/cocv/2016071.CrossRefGoogle Scholar
25Di Nezza, E., Palatucci, G. and Valdinoci, E.. Hitchhiker's guide to the fractional Sobolev spaces. Bull. Sci. math. 136 (2012), 521–573.CrossRefGoogle Scholar
26Dipierro, S., Medina, M. and Valdinoci, E.. Fractional elliptic problems with critical growth in the whole of ℝn, Appunti. Scuola Normale Superiore di Pisa (Nuova Serie) [Lecture Notes. Scuola Normale Superiore di Pisa (New Series)], 15. Edizioni della Normale, Pisa, 2017. viii+152 pp.Google Scholar
27Esteban, M. and Lions, P. L.. Stationary solutions of nonlinear Schrödinger equations with an external magnetic field. Partial differential equations and the calculus of variations, vol. I, pp. 401–449, Progr. nonlinear differential equations Appl., 1 (Boston, MA: Birkhäuser Boston, 1989).Google Scholar
28Felmer, P., Quaas, A. and Tan, J.. Positive solutions of the nonlinear Schrödinger equation with the fractional Laplacian. Proc. Roy. Soc. Edinburgh Sect. A 142 (2012), 1237–1262.CrossRefGoogle Scholar
29Fiscella, A., Pinamonti, A. and Vecchi, E.. Multiplicity results for magnetic fractional problems. J. Differ. Equ. 263 (2017), 4617–4633.CrossRefGoogle Scholar
30Giammetta, A. R.. Fractional Schrödinger-Poisson-Slater system in one dimension, preprint arXiv:1405.2796.Google Scholar
31He, X.. Multiplicity and concentration of positive solutions for the Schrödinger-Poisson equations. Z. Angew. Math. Phys. 62 (2011), 869–889.CrossRefGoogle Scholar
32He, Y. and Li, G.. Standing waves for a class of Schrödinger-Poisson equations in ℝ3 involving critical Sobolev exponents. Ann. Acad. Sci. Fenn. Math. 40 (2015), 729–766.CrossRefGoogle Scholar
33Ichinose, T.. Magnetic relativistic Schrödinger operators and imaginary-time path integrals. Mathematical physics, spectral theory and stochastic analysis, pp. 247–297, Oper. Theory Adv. Appl., vol. 232 (Basel: Birkhäuser/Springer Basel AG, 2013).Google Scholar
34Kato, T.. Schrödinger operators with singular potentials, Proceedings of the International Symposium on Partial Differential Equations and the Geometry of Normed Linear Spaces (Jerusalem, 1972), Israel J. Math., vol. 13, pp. 135–148 (1973).Google Scholar
35Kurata, K.. Existence and semi-classical limit of the least energy solution to a nonlinear Schrödinger equation with electromagnetic fields. Nonlinear Anal. 41 (2000), 763–778.CrossRefGoogle Scholar
36Laskin, N.. Fractional quantum mechanics and Lévy path integrals. Phys. Lett. A 268 (2000), 298–305.CrossRefGoogle Scholar
37Lieb, E. H. and Loss, M.. Analysis. Graduate studies in Mathematics, vol. 14 (Providence, RI: American Mathematical Society, 1997), xviii+278 pp.Google Scholar
38Liu, Z. and Zhang, J.. Multiplicity and concentration of positive solutions for the fractional Schrödinger-Poisson systems with critical growth. ESAIM Control Optim. Calc. Var. 23 (2017), 1515–1542.CrossRefGoogle Scholar
39Markowich, P., Ringhofer, C. and Schmeiser, C.. Semiconductor equations (New York: Springer-Verlag, 1990).CrossRefGoogle Scholar
40Mingqi, X., Pucci, P., Squassina, M. and Zhang, B.. Nonlocal Schrödinger-Kirchhoff equations with external magnetic field. Discrete Contin. Dyn. Syst. 37 (2017), 1631–1649.Google Scholar
41Molica Bisci, G., Rădulescu, V. and Servadei, R.. Variational methods for nonlocal fractional problems vol. 162 (Cambridge: Cambridge University Press, 2016).CrossRefGoogle Scholar
42Moroz, V. and Van Schaftingen, J.. Groundstates of nonlinear Choquard equations: existence, qualitative properties and decay asymptotics. J. Funct. Anal. 265 (2013), 153–184.CrossRefGoogle Scholar
43Moser, J.. A new proof of De Giorgi's theorem concerning the regularity problem for elliptic differential equations. Comm. Pure Appl. Math. 13 (1960), 457–468.CrossRefGoogle Scholar
44Murcia, E. and Siciliano, G.. Positive semiclassical states for a fractional Schrödinger-Poisson system. Differ. Integral Equ. 30 (2017), 231–258.Google Scholar
45Palatucci, G. and Pisante, A.. Improved Sobolev embeddings, profile decomposition, and concentration-compactness for fractional Sobolev spaces. Calc. Var. Partial Differ. Equ. 50 (2014), 799–829.CrossRefGoogle Scholar
46Pinamonti, A., Squassina, M. and Vecchi, E.. The Maz'ya-Shaposhnikova limit in the magnetic setting. J. Math. Anal. Appl. 449 (2017), 1152–1159.CrossRefGoogle Scholar
47Pinamonti, A., Squassina, M. and Vecchi, E.. Magnetic BV functions and the Bourgain-Brezis-Mironescu formula, To appear in Adv. Calc. Var. DOI: 10.1515/acv-2017-0019.CrossRefGoogle Scholar
48Rabinowitz, P. H.. On a class of nonlinear Schrödinger equations. Z. Angew. Math. Phys. 43 (1992), 270–291.CrossRefGoogle Scholar
49Ruiz, D.. The Schrödinger-Poisson equation under the effect of a nonlinear local term. J. Funct. Anal. 237 (2006), 655–674.CrossRefGoogle Scholar
50Secchi, S.. Ground state solutions for nonlinear fractional Schrödinger equations in ℝN. J. Math. Phys. 54 (2013), 031501.CrossRefGoogle Scholar
51Squassina, M. and Volzone, B.. Bourgain-Brezis-Mironescu formula for magnetic operators. C. R. Math. 354 (2016), 825–831.CrossRefGoogle Scholar
52Teng, K.. Existence of ground state solutions for the nonlinear fractional Schrödinger-Poisson system with critical Sobolev exponent. J. Differ. Equ. 261 (2016), 3061–3106.CrossRefGoogle Scholar
53Wang, J., Tian, L., Xu, J. and Zhang, F.. Existence and concentration of positive solutions for semilinear Schrödinger-Poisson systems in ℝ3. Calc. Var. Partial Differ. Equ. 48 (2013), 275–276.CrossRefGoogle Scholar
54Willem, M.. Minimax theorems. Progress in nonlinear differential equations and their applications vol. 24 (Boston, MA: Birkhäuser Boston, Inc., 1996).CrossRefGoogle Scholar
55Yang, M.. Concentration of positive ground state solutions for Schrödinger-Maxwell systems with critical growth. Adv. Nonlinear Stud. 16 (2016), 389–408.CrossRefGoogle Scholar
56Zhang, J., do Ó, M. and Squassina, M.. Fractional Schrödinger-Poisson Systems with a General Subcritical or Critical Nonlinearity. Adv. Nonlinear Stud. 16 (2016), 15–30.CrossRefGoogle Scholar
57Zhang, B., Squassina, M. and Zhang, X.. Fractional NLS equations with magnetic field, critical frequency and critical growth, to appear in Manuscripta Math., doi:10.1007/s00229-017-0937-4.CrossRefGoogle Scholar
58Zhao, L. and Zhao, F.. On the existence of solutions for the Schrödinger-Poisson equations. J. Math. Anal. Appl. 346 (2008), 155–169.CrossRefGoogle Scholar