Hostname: page-component-8448b6f56d-wq2xx Total loading time: 0 Render date: 2024-04-18T07:08:06.688Z Has data issue: false hasContentIssue false
  • English
  • Français

Bounding Sn(t) on the Riemann hypothesis

Published online by Cambridge University Press:  02 March 2017

EMANUEL CARNEIRO  and
ANDRÉS CHIRRE
EMANUEL CARNEIRO
Affiliation:
IMPA - Instituto Nacional de Matemática Pura e Aplicada, Estrada Dona Castorina, 110, Rio de Janeiro, RJ, Brazil22460-320. e-mail: carneiro@impa.br, achirre@impa.br
ANDRÉS CHIRRE
Affiliation:
IMPA - Instituto Nacional de Matemática Pura e Aplicada, Estrada Dona Castorina, 110, Rio de Janeiro, RJ, Brazil22460-320. e-mail: carneiro@impa.br, achirre@impa.br
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 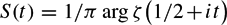$S(t) = {1}/{\pi} \arg \zeta \big(\hh + it \big)$ be the argument of the Riemann zeta-function at the point 1/2 + it. For n ⩾ 1 and t > 0 define its iterates

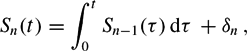$$\begin{equation*} S_n(t) = \int_0^t S_{n-1}(\tau) \,\d\tau\, + \delta_n\,, \end{equation*}$$
where δn is a specific constant depending on n and S0(t) ≔ S(t). In 1924, J. E. Littlewood proved, under the Riemann hypothesis (RH), that Sn(t) = O(log t/(log log t)n + 1). The order of magnitude of this estimate was never improved up to this date. The best bounds for S(t) and S1(t) are currently due to Carneiro, Chandee and Milinovich. In this paper we establish, under RH, an explicit form of this estimate
$$\begin{equation*} -\left( C^-_n + o(1)\right) \frac{\log t}{(\log \log t)^{n+1}} \ \leq \ S_n(t) \ \leq \ \left( C^+_n + o(1)\right) \frac{\log t}{(\log \log t)^{n+1}}\,, \end{equation*}$$
for all n ⩾ 2, with the constants C±n decaying exponentially fast as n → ∞. This improves (for all n ⩾ 2) a result of Wakasa, who had previously obtained such bounds with constants tending to a stationary value when n → ∞. Our method uses special extremal functions of exponential type derived from the Gaussian subordination framework of Carneiro, Littmann and Vaaler for the cases when n is odd, and an optimized interpolation argument for the cases when n is even. In the final section we extend these results to a general class of L-functions.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 164 , Issue 2 , March 2018 , pp. 259 - 283
Copyright
Copyright © Cambridge Philosophical Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Carneiro, E. and Chandee, V.. Bounding ζ(s) in the critical strip. J. Number Theory 131 (2011), 363–384.CrossRefGoogle Scholar
[2] Carneiro, E., Chandee, V., Littmann, F. and Milinovich, M. B.. Hilbert spaces and the pair correlation of zeros of the Riemann zeta-function. J. Reine Angew. Math. (Crelle's Journal) DOI 10.1515/crelle-2014-0078 (to appear).Google Scholar
[3] Carneiro, E., Chandee, V. and Milinovich, M. B.. Bounding S(t) and S 1(t) on the Riemann hypothesis. Math. Ann. 356 (2013), no. 3, 939–968.Google Scholar
[4] Carneiro, E., Chandee, V. and Milinovich, M. B.. A note on the zeros of zeta and L-functions. Math. Z. 281 (2015), 315–332.CrossRefGoogle Scholar
[5] Carneiro, E. and Finder, R.. On the argument of L-functions. Bull. Braz. Math. Soc. 46, no. 4 (2015), 601–620.Google Scholar
[6] Carneiro, E. and Littmann, F.. Bandlimited approximation to the truncated Gaussian and applications. Constr. Approx. 38, no. 1 (2013), 19–57.CrossRefGoogle Scholar
[7] Carneiro, E. and Littmann, F.. Extremal functions in de Branges and Euclidean spaces. Adv. Math. 260 (2014), 281–349.CrossRefGoogle Scholar
[8] Carneiro, E. and Littmann, F.. Extremal functions in de Branges and Euclidean spaces II. Amer. J. Math. (to appear).Google Scholar
[9] Carneiro, E., Littmann, F. and Vaaler, J. D.. Gaussian subordination for the Beurling–Selberg extremal problem. Trans. Amer. Math. Soc. 365, no. 7 (2013), 3493–3534.Google Scholar
[10] Carneiro, E. and Vaaler, J. D.. Some extremal functions in Fourier analysis, II. Trans. Amer. Math. Soc. 362 (2010), 5803–5843.Google Scholar
[11] Chandee, V. and Soundararajan, K.. Bounding |ζ(1/2 + it)| on the Riemann hypothesis. Bull. London Math. Soc. 43 (2011), no. 2, 243–250.Google Scholar
[12] Davenport, H.. Multiplicative number theory. Third edition, Graduate Texts in Mathematics 74 (Springer-Verlag, New York, 2000).Google Scholar
[13] Fujii, A.. On the zeros of the Riemann zeta function. Comment. Math. Univ. St. Pauli 51 (2002), no. 1, 1–17.Google Scholar
[14] Fujii, A.. An explicit estimate in the theory of the distribution of the zeros of the Riemann zeta function. Comment. Math. Univ. St. Pauli 53 (2004), 85–114.Google Scholar
[15] Fujii, A.. A note of the distribution of the argument of the Riemann zeta function. Comment. Math. Univ. St. Pauli 55 (2006), no. 2, 135–147.Google Scholar
[16] Gallagher, P. X.. Pair correlation of zeros of the zeta function. J. Reine Angew. Math. 362 (1985), 72–86.Google Scholar
[17] Goldston, D. A. and Gonek, S. M.. A note on S(t) and the zeros of the Riemann zeta-function. Bull. London Math. Soc. 39 (2007), 482–486.Google Scholar
[18] Gradshteyn, I. S. and Ryzhik, I. M.. Table of Integrals, Series and Products. 4th ed. (New York Academic Press, 1965).Google Scholar
[19] Holt, J. and Vaaler, J. D.. The Beurling–Selberg extremal functions for a ball in the Euclidean space. Duke Math. J. 83 (1996), 203–247.Google Scholar
[20] Iwaniec, H. and Kowalski, E.. Analytic Number Theory. American Mathematical Society Colloquium Publications, vol. 53 (2004).Google Scholar
[21] Karatsuba, A. A. and Korolëv, M. A.. The argument of the Riemann zeta function. Russian Math. Surveys 60 (2005), no. 3, 433–488 (in English), Uspekhi Mat. Nauk 60 (2005), no. 3, 41–96 (in Russian).Google Scholar
[22] Littlewood, J. E.. On the zeros of the Riemann zeta-function. Proc. Camb. Phil. Soc. 22 (1924), 295–318.Google Scholar
[23] Ramachandra, K. and Sankaranarayanan, A.. On some theorems of Littlewood and Selberg I. J. Number Theory 44 (1993), 281–291.Google Scholar
[24] Vaaler, J. D.. Some extremal functions in Fourier analysis. Bull. Amer. Math. Soc. 12 (1985), 183–215.CrossRefGoogle Scholar
[25] Wakasa, T.. The explicit upper bound of the multiple integrals of S(t) on the Riemann hypothesis. Comment. Math. Univ. St. Pauli 61 (2012), no. 2, 115–131.Google Scholar