Hostname: page-component-8448b6f56d-42gr6 Total loading time: 0 Render date: 2024-04-24T20:57:54.827Z Has data issue: false hasContentIssue false
  • English
  • Français

Regimes of two-dimensionality of decaying shallow axisymmetric swirl flows with background rotation

Published online by Cambridge University Press:  01 December 2011

M. Duran-Matute ,
L. P. J. Kamp ,
R. R. Trieling  and
G. J. F. van Heijst
M. Duran-Matute*
Affiliation:
Department of Applied Physics and J. M. Burgers Centre, Eindhoven University of Technology, PO Box 513, 5600 MB Eindhoven, The Netherlands
L. P. J. Kamp
Affiliation:
Department of Applied Physics and J. M. Burgers Centre, Eindhoven University of Technology, PO Box 513, 5600 MB Eindhoven, The Netherlands
R. R. Trieling
Affiliation:
Department of Applied Physics and J. M. Burgers Centre, Eindhoven University of Technology, PO Box 513, 5600 MB Eindhoven, The Netherlands
G. J. F. van Heijst
Affiliation:
Department of Applied Physics and J. M. Burgers Centre, Eindhoven University of Technology, PO Box 513, 5600 MB Eindhoven, The Netherlands
*
†Email address for correspondence: m.duran.matute@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Both background rotation and small depths are said to enforce the two-dimensionality of flows. In the current paper, we describe a systematic study of the two-dimensionality of a shallow monopolar vortex subjected to background rotation. Using a perturbation analysis of the Navier–Stokes equations for small aspect ratio 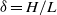(with 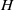the fluid depth and 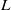a typical radial length scale of the vortex), we found nine different regimes in the parameter space where the flow is governed to lowest order by different sets of equations. From the properties of these sets of equations, it was determined that the flow can be considered as quasi-two-dimensional in only five of the nine regimes. The scaling of the velocity components as given by these sets of equations was compared with results from numerical simulations to find the actual boundaries of the different regimes in the parameter space (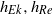), where 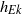is the Ekman boundary layer thickness and 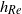is the equivalent boundary layer thickness for a monopolar vortex without background rotation. Even though background rotation and small depths do promote the two-dimensionality of flows independently, the combination of these two characteristics does not necessarily have that same effect.

JFM classification

Geophysical and Geological Flows: Rotating flows Geophysical and Geological Flows: Shallow water flows Vortex Flows: Vortex dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 691 , 25 January 2012 , pp. 214 - 244
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Afanasyev, Y. & Wells, J. 2005 Quasi-two-dimensional turbulence on the polar beta-plane: laboratory experiments. Geophys. Astrophys. Fluid Dyn. 99, 1–17.CrossRefGoogle Scholar
2. Akkermans, R. A. D., Cieslik, A. R., Kamp, L. P. J., Trieling, R. R., Clercx, H. J. H. & van Heijst, G. J. F. 2008a The three-dimensional structure of an electromagnetically generated dipolar vortex in a shallow fluid layer. Phys. Fluids 554, 116601.CrossRefGoogle Scholar
3. Akkermans, R. A. D., Kamp, L. P. J., Clercx, H. J. H. & van Heijst, G. J. F. 2008b Intrinsic three-dimensionality in electromagnetically driven shallow flows. Europhys. Lett. 83, 24001.CrossRefGoogle Scholar
4. Bödewadt, 1940 Die Drehströmung über festem Grunde. Z. Angew. Math. Mech. 20, 241–245.CrossRefGoogle Scholar
5. Brink, K. H. 1997 Time-dependent motions and the nonlinear bottom Ekman layer. J. Mar. Res. 55, 613–631.CrossRefGoogle Scholar
6. Carnevale, G. F., Briscolini, M., Kloosterziel, R. C. & Vallis, G. K. 1997 Three-dimensionally perturbed vortex tubes in a rotating flow. J. Fluid Mech. 341, 127–163.CrossRefGoogle Scholar
7. Davidson, P. A. 1989 The interaction between swirling and recirculating velocity components in unsteady, inviscid flow. J. Fluid Mech. 209, 35–55.CrossRefGoogle Scholar
8. Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
9. Dolzhanskii, F. V., Krymov, V. A. & Manin, D. Y. 1990 Stability and vortex structures of quasi-two-dimensional flows. Sov. Phys. Uspekhi 33, 495–520.CrossRefGoogle Scholar
10. Dolzhanskii, F. V., Krymov, V. A. & Manin, D. Y. 1992 An advanced experimental investigation of quasi-two-dimensional shear flows. J. Fluid Mech. 241, 705–722.CrossRefGoogle Scholar
11. Duran-Matute, M., Albagnac, J., Kamp, L. P. J. & van Heijst, G. J. F. 2010a Dynamics and structure of decaying shallow dipolar vortices. Phys. Fluids 22, 116606.CrossRefGoogle Scholar
12. Duran-Matute, M., Kamp, L. P. J., Trieling, R. R. & van Heijst, G. J. F. 2010b Scaling of decaying shallow axisymmetric swirl flows. J. Fluid Mech. 648, 471–484.CrossRefGoogle Scholar
13. Hart, J. E. 2000 A note on nonlinear correction to the Ekman layer pumping velocity. Phys. Fluids 12, 131–135.CrossRefGoogle Scholar
14. Hopfinger, E. J. 1982 Turbulence and waves in a rotating tank. J. Fluid Mech. 125, 505–534.CrossRefGoogle Scholar
15. Hopfinger, E. J. & van Heijst, G. J. F. 1993 Vortices in rotating fluids. Annu. Rev. Fluid Mech. 25, 241–289.CrossRefGoogle Scholar
16. Ishida, S. & Iwayama, T. 2006 A comprehensive analysis of nonlinear corrections to the classical Ekman pumping. J. Met. Soc. Japan 89, 839–851.CrossRefGoogle Scholar
17. von Kármán, T. 1921 Über laminare und turbulente Reibung. Z. Angew. Math. Mech. 1, 233–252.CrossRefGoogle Scholar
18. Kellay, H. & Goldburg, W. I. 2002 Two-dimensional turbulence: a review of some recent experiments. Rep. Prog. Phys. 65, 845–894.CrossRefGoogle Scholar
19. Kloosterziel, R. C. & van Heijst, G. J. F. 1991 An experimental study of unstable barotropic vortices in a rotating fluid. J. Fluid Mech. 223, 1–24.CrossRefGoogle Scholar
20. Kloosterziel, R. C. & van Heijst, G. J. F. 1992 The evolution of stable barotropic vortices in a rotating free-surface fluid. J. Fluid Mech. 239, 607–629.CrossRefGoogle Scholar
21. Morize, C., Moisy, F. & Rabaud, M. 2005 Decaying grid turbulence in a rotating tank. Phys. Fluids 17, 095105.CrossRefGoogle Scholar
22. Orlandi, P. & Carnevale, G. F. 1999 Evolution of isolated vortices in a rotating fluid of finite depth. J. Fluid Mech. 381, 239–269.CrossRefGoogle Scholar
23. Paret, J. & Tabeling, P. 1997 Experimental observation of the two-dimensional inverse energy cascade. Phys. Rev. Lett. 79, 4162–4165.CrossRefGoogle Scholar
24. Pedlosky, J. 1987 Geophysical Fluid Dynamics, 2nd edn. Springer.CrossRefGoogle Scholar
25. Satijn, M. P., Cense, A. W., Verzicco, R., Clercx, H. J. H. & van Heijst, G. J. F. 2001 Three-dimensional structure and decay properties of vortices in shallow fluid layers. Phys. Fluids 13, 1932–1945.CrossRefGoogle Scholar
26. Staplehurst, P. J., Davidson, P. A. & Dalziel, S. B. 2008 Structure formation in homogeneous freely decaying rotating turbulence. J. Fluid Mech. 598, 81–105.CrossRefGoogle Scholar
27. Tabeling, P. 2002 Two-dimensional turbulence: a physicist approach. Phys. Rep. 362, 1–62.CrossRefGoogle Scholar
28. Tabeling, P., Burkhart, S., Cardoso, O. & Willaime, H. 1991 Experimental study of freely decaying two-dimensional turbulence. Phys. Rev. Lett. 67, 3772–3775.CrossRefGoogle ScholarPubMed
29. Thomson, W. 1910 Vibrations of a columnar vortex. Math. Phys. Papers 4, 152–165.Google Scholar
30. Velasco Fuentes, O. U. 2009 Kelvin’s discovery of Taylor columns. Eur. J. Mech. B/Fluids 28, 469–472.CrossRefGoogle Scholar
31. Zavala Sansón, L. & van Heijst, G. J. F. 2000 Nonlinear Ekman effects in rotating barotropic flows. J. Fluid Mech. 412, 75–91.CrossRefGoogle Scholar