Hostname: page-component-8448b6f56d-t5pn6 Total loading time: 0 Render date: 2024-04-16T16:23:20.194Z Has data issue: false hasContentIssue false
  • English
  • Français

Heat/mass transport in shear flow over a reactive surface with inert defects

Published online by Cambridge University Press:  13 December 2016

Preyas N. Shah Open the ORCID record for Preyas N. Shah [Opens in a new window] ,
Tiras Y. Lin  and
Eric S. G. Shaqfeh
Preyas N. Shah*
Affiliation:
Department of Mechanical Engineering, Stanford University, Stanford, CA 94305, USA
Tiras Y. Lin*
Affiliation:
Department of Mechanical Engineering, Stanford University, Stanford, CA 94305, USA
Eric S. G. Shaqfeh*
Affiliation:
Department of Mechanical Engineering, Stanford University, Stanford, CA 94305, USA Department of Chemical Engineering, Stanford University, Stanford, CA 94305, USA Institute of Computational and Mathematical Engineering, Stanford University, Stanford, CA 94305, USA
*
†Email addresses for correspondence: preyas@stanford.edu, tiraslin@stanford.edu, esgs@stanford.edu
†Email addresses for correspondence: preyas@stanford.edu, tiraslin@stanford.edu, esgs@stanford.edu
†Email addresses for correspondence: preyas@stanford.edu, tiraslin@stanford.edu, esgs@stanford.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the problem of mass transport to surfaces with heterogeneous reaction rates in the presence of shear flow over these surfaces. The reactions are first order and the heterogeneity is due to the existence of inert regions on the surfaces. Such problems are ubiquitous in the field of heterogeneous catalysis, electrochemistry and even biological mass transport. In these problems, the microscale reaction rate is characterized by a Damköhler number $\unicode[STIX]{x1D705}$, while the Péclet number $P$ is the dimensionless ratio of the bulk shear rate to the inverse diffusion time scale. The area fraction of the reactive region is denoted by 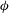$\unicode[STIX]{x1D719}$. The objective is to calculate the yield of reaction, which is directly related to the mass flux to the reactive region, denoted by the dimensionless Sherwood number 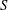$S$. Previously, we used boundary element simulations and examined the case of first-order reactive disks embedded in an inert surface (Shah & Shaqfeh J. Fluid Mech., vol. 782, 2015, pp. 260–299). Various correlations for the Sherwood number as a function of 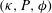$(\unicode[STIX]{x1D705},P,\unicode[STIX]{x1D719})$ were obtained. In particular, we demonstrated that the ‘method of additive resistances’ provides a good approximation for the Sherwood number for a wide range of values of 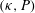$(\unicode[STIX]{x1D705},P)$ for $0<\unicode[STIX]{x1D719}<0.78$. When $\unicode[STIX]{x1D719}\approx 0.78$, the reactive disks are in a close packed configuration where the inert regions are essentially disconnected from each other. In this work, we develop an understanding of the physics when 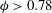$\unicode[STIX]{x1D719}>0.78$, by examining the inverse problem of inert disks on a reactive surface. We show that the method of resistances approach to obtain the Sherwood number fails in the limit as 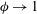$\unicode[STIX]{x1D719}\rightarrow 1$, i.e. in the dilute limit of periodic inert disks, due to the existence of a surface concentration boundary layer around each disk that scales with (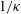$1/\unicode[STIX]{x1D705}$). This boundary layer controls the screening length between inert disks and allows us to introduce a new theory, thus providing new correlations for the Sherwood number that are highly accurate in the limit of 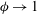$\unicode[STIX]{x1D719}\rightarrow 1$.

JFM classification

Low-Reynolds-number flows: Boundary integral methods Boundary Layers: Boundary Layers Low-Reynolds-number flows: Porous media
Type
Papers
Information
Journal of Fluid Mechanics , Volume 811 , 25 January 2017 , pp. 372 - 399
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bartholomew, C. H. & Farrauto, R. J. 2011 Fundamentals of Industrial Catalytic Processes. Wiley.Google Scholar
Churchill, S. W. & Usagi, R. 1972 A general expression for the correlation of rates of transfer and other phenomena. AIChE J. 18 (6), 1121–1128.Google Scholar
Córdoba, A. 1989 Dirac comb. Lett. Math. Phys. 17 (3), 191–196.CrossRefGoogle Scholar
Gladwell, G. M. L., Barber, J. R. & Olesiak, Z. 1983 Thermal problems with radiation boundary conditions. Q. J. Mech. Appl. Maths 36 (3), 387–401.CrossRefGoogle Scholar
Hasimoto, H. 1959 On the periodic fundamental solutions of the Stokes equations and their application to viscous flow past a cubic array of spheres. J. Fluid Mech. 5 (02), 317–328.Google Scholar
Sadhal, S. S. & Tio, K. 1991 Analysis of thermal constriction resistance with adiabatic circular gaps. J. Thermophys. Heat Transfer 5 (4), 550–559.Google Scholar
Sangani, A. S. & Behl, S. 1989 The planar singular solutions of Stokes and Laplace equations and their application to transport processes near porous surfaces. Phys. Fluids A 1 (1), 21–37.Google Scholar
Shah, P. N. & Shaqfeh, E. S. G. 2015 Heat/mass transport in shear flow over a heterogeneous surface with first-order surface-reactive domains. J. Fluid Mech. 782, 260–299.Google Scholar
Stone, H. A. 1989 Heat/mass transfer from surface films to shear flows at arbitrary Péclet numbers. Phys. Fluids A 1, 1112–1122.Google Scholar