Hostname: page-component-8448b6f56d-t5pn6 Total loading time: 0 Render date: 2024-04-23T10:11:01.871Z Has data issue: false hasContentIssue false
  • English
  • Français

LOCAL BOUNDEDNESS OF NONAUTONOMOUS SUPERPOSITION OPERATORS IN $BV[0,1]$

Part of: Real functions Nonlinear operators and their properties

Published online by Cambridge University Press:  08 July 2015

PIOTR KASPRZAK  and
PIOTR MAĆKOWIAK
PIOTR KASPRZAK*
Affiliation:
Optimization and Control Theory Department, Faculty of Mathematics and Computer Science, Adam Mickiewicz University, ul. Umultowska 87, 61-614 Poznań, Poland email kasp@amu.edu.pl
PIOTR MAĆKOWIAK
Affiliation:
Department of Mathematical Economics, Poznań University of Economics, Al. Niepodległości 10, 61-875 Poznań, Poland email p.mackowiak@ue.poznan.pl
*
✉kasp@amu.edu.pl
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The main goal of this paper is to give the answer to one of the main problems of the theory of nonautonomous superposition operators acting in the space of functions of bounded variation in the sense of Jordan. Namely, we prove that if the superposition operator maps the space 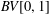$BV[0,1]$ into itself, then it is automatically locally bounded, provided its generator is a locally bounded function.

Keywords

acting conditionslocally bounded mapping(nonautonomous) superposition operatorvariation in the sense of Jordan

MSC classification

Primary: 47H30: Particular nonlinear operators (superposition, Hammerstein, Nemytskiĭ, Uryson, etc.)
Secondary: 26A45: Functions of bounded variation, generalizations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 92 , Issue 2 , October 2015 , pp. 325 - 341
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Ambrosio, L., Fusco, N. and Pallara, D., Functions of Bounded Variation and Free Discontinuity Problems, Oxford Mathematical Monographs (Oxford Science Publications, Clarendon Press, Oxford, 2000).CrossRefGoogle Scholar
Appell, J., Banaś, J. and Merentes, N., Bounded Variation and Around, De Gruyter Series in Nonlinear Analysis and Applications, 17 (De Gruyter, Berlin, 2014).Google Scholar
Appell, J. and Zabrejko, P. P., Nonlinear Superposition Operators (Cambridge University Press, Cambridge, 1990).CrossRefGoogle Scholar
Bugajewska, D., ‘On the superposition operator in the space of functions of bounded variation, revisited’, Math. Comput. Modelling 52(5–6) (2010), 791–796.CrossRefGoogle Scholar
Bugajewska, D., ‘A note on differential and integral equations in the spaces of functions ofΛ-bounded variation’, Nonlinear Anal. 75 (2012), 4213–4221.CrossRefGoogle Scholar
Bugajewski, D., ‘On BV-solutions of some nonlinear integral equations’, Integral Equations Operator Theory 46 (2003), 387–398.CrossRefGoogle Scholar
Čelidze, V. G. and Džvaršeĭšvili, A. G., The Theory of the Denjoy Integral and Some Applications, Series in Real Analysis, 3 (World Scientific Publishing Co. Inc., Teaneck, NJ, 1989), Translated from the Russian, with a preface and an appendix by P. S. Bullen.CrossRefGoogle Scholar
Chambolle, A., Caselles, V., Cremers, D., Novaga, M. and Pock, T., ‘An introduction to total variation for image analysis’, in: Theoretical Foundations and Numerical Methods for Sparse Recovery, Radon Series on Computational and Applied Mathematics, 9 (Walter de Gruyter, Berlin, 2010), 263–340.CrossRefGoogle Scholar
Chan, T. F. and Shen, J., Image Processing and Analysis, Variational, PDE, Wavelet, and Stochastic Methods (Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA, 2005).CrossRefGoogle Scholar
Hansen, P. C., Nagy, J. G. and O’Leary, D. P., Deblurring Images, Matrices, Spectra, and Filtering, Fundamentals of Algorithms, 3 (Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA, 2006).CrossRefGoogle Scholar
Harris, H. and Laibson, D., ‘Dynamic choices of hyperbolic consumers’, Econometrica 69 (2001), 935–957.CrossRefGoogle Scholar
Jordan, C., ‘Sur la série de Fourier’, C. R. Acad. Sci. Paris 2 (1881), 228–230; (in French).Google Scholar
Josephy, M., ‘Composing functions of bounded variation’, Proc. Amer. Math. Soc. 83(2) (1981), 354–356.CrossRefGoogle Scholar
Kim, Y. and Vese, L. A., ‘Image recovery using functions of bounded variation and Sobolev spaces of negative differentiability’, Inverse Probl. Imaging 3(1) (2009), 43–68.CrossRefGoogle Scholar
Maćkowiak, P., ‘A counterexample to Ljamin’s theorem’, Proc. Amer. Math. Soc. 142(5) (2014), 1773–1776.CrossRefGoogle Scholar
Mazya, V., Sobolev Spaces with Applications to Elliptic Partial Differential Equations, Grundlehren der mathematischen Wissenschaften, 342 (Springer, Heidelberg, 2011).CrossRefGoogle Scholar
Rudin, L., Osher, S. and Fatemi, E., ‘Nonlinear total variation based noise removal algorithms’, Phys. D 60(1–4) (1992), 259–268.CrossRefGoogle Scholar
Waterman, D., ‘On convergence of Fourier series of functions of generalized bounded variation’, Studia Math. 44 (1972), 107–117.CrossRefGoogle Scholar