No CrossRef data available.
Article contents
Density of mode-locking property for quasi-periodically forced Arnold circle maps
Published online by Cambridge University Press: 04 April 2024
Abstract
We show that the mode-locking region of the family of quasi-periodically forced Arnold circle maps with a topologically generic forcing function is dense. This gives a rigorous verification of certain numerical observations in [M. Ding, C. Grebogi and E. Ott. Evolution of attractors in quasiperiodically forced systems: from quasiperiodic to strange nonchaotic to chaotic. Phys. Rev. A 39(5) (1989), 2593–2598] for such forcing functions. More generally, under some general conditions on the base map, we show the density of the mode-locking property among dynamically forced maps (defined in [Z. Zhang. On topological genericity of the mode-locking phenomenon. Math. Ann. 376 (2020), 707–72]) equipped with a topology that is much stronger than the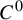 
$C^0$ topology, compatible with smooth fiber maps. For quasi-periodic base maps, our result generalizes the main results in [A. Avila, J. Bochi and D. Damanik. Cantor spectrum for Schrödinger operators with potentials arising from generalized skew-shifts. Duke Math. J. 146 (2009), 253–280], [J. Wang, Q. Zhou and T. Jäger. Genericity of mode-locking for quasiperiodically forced circle maps. Adv. Math. 348 (2019), 353–377] and Zhang (2020).
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
Avila, A. and Bochi, J.. A uniform dichotomy for generic
$SL(2,\mathbb{R})$
cocycles over a minimal base. Bull. Soc. Math. France 135 (2007), 407–417.CrossRefGoogle Scholar
$SL(2,\mathbb{R})$
cocycles over a minimal base. Bull. Soc. Math. France 135 (2007), 407–417.CrossRefGoogle ScholarAvila, A., Bochi, J. and Damanik, D.. Cantor spectrum for Schrödinger operators with potentials arising from generalized skew-shifts. Duke Math. J. 146 (2009), 253–280.CrossRefGoogle Scholar
Avila, A., Bochi, J. and Damanik, D.. Opening gaps in the spectrum of strictly ergodic Schrödinger operators. J. Eur. Math. Soc. (JEMS) 14 (2012), 61–106.Google Scholar
Bjerklöv, K. and Jäger, T.. Rotation numbers for quasiperiodically forced circle maps – mode-locking vs strict monotonicity. J. Amer. Math. Soc. 22(2) (2009), 353–362.CrossRefGoogle Scholar
Bochi, J.. Genericity of zero Lyapunov exponents. Ergod. Th. & Dynam. Sys. 22 (2002), 1667–1696.CrossRefGoogle Scholar
Bondeson, A., Ott, E. and Antonsen, T. M. Jr. Quasiperiodically forced damped pendula and Schrödinger equations with quasiperiodic potentials: implications of their equivalence. Phys. Rev. Lett. 55 (1985), 2103.CrossRefGoogle ScholarPubMed
Damanik, D.. Lyapunov exponents and spectral analysis of ergodic Schrödinger operators: a survey of Kotani theory and its applications. Spectral Theory and Mathematical Physics: A Festschrift in Honor of Barry Simon’s 60th Birthday (Proceedings of Symposia in Pure Mathematics, 76, Part 2). Ed. Gesztesy, F., Deift, P., Galvez, C., Perry, P. and Schlag, W.. American Mathematical Society, Providence, RI, 2007, pp. 539–563.CrossRefGoogle Scholar
Ding, M., Grebogi, C. and Ott, E.. Evolution of attractors in quasiperiodically forced systems: from quasiperiodic to strange nonchaotic to chaotic. Phys. Rev. A 39(5) (1989), 2593–2598.CrossRefGoogle ScholarPubMed
Dugundji, J.. An extension of Tietze’s theorem. Pacific J. Math. 1(3) (1951), 353–367.CrossRefGoogle Scholar
Eliasson, H.. Floquet solutions for the
$1$
-dimensional quasi-periodic Schrödinger equation. Comm. Math. Phys. 146 (1992), 447–482.CrossRefGoogle Scholar
$1$
-dimensional quasi-periodic Schrödinger equation. Comm. Math. Phys. 146 (1992), 447–482.CrossRefGoogle ScholarFayad, B. and Krikorian, R.. Some questions around quasi-periodic dynamics. Proceedings of the International Congress of Mathematicians (ICM 2018), Vol. III. Invited Lectures, 1909–1932. World Scientific Publishers, Hackensack, NJ, 2018, pp. 1909–1932.CrossRefGoogle Scholar
Grebogi, C., Ott, E., Pelikan, S. and Yorke, J. A.. Strange attractors that are not chaotic. Phys. D 13(1–2) (1984), 261–268.CrossRefGoogle Scholar
Herman, M.. Une méthode pour minorer les exposants de Lyapunov et quelques exemples montrant le caractère local d’un théorème d’Arnold et de Moser sur le tore de dimension 2. Comment. Math. Helv. 58 (1983), 453–502.CrossRefGoogle Scholar
Jäger, T.. Strange non-chaotic attractors in quasiperiodically forced circle maps. Comm. Math. Phys. 289 (2009), 253–289.CrossRefGoogle Scholar
Jäger, T.. Strange non-chaotic attractors in quasiperiodically forced circle maps: Diophantine forcing. Ergod. Th. & Dynam. Sys. 33(5) (2013), 1477–1501.CrossRefGoogle Scholar
Jäger, T. and Wang, J.. Abundance of mode-locking for quasiperiodically forced circle maps. Comm. Math. Phys., 353(1) (2017), 1–36.Google Scholar
Jitomirskaya, S.. Ergodic Schrödinger operators (on one foot). Spectral Theory and Mathematical Physics: A Festschrift in Honor of Barry Simon’s 60th Birthday (Proceedings of Symposia in Pure Mathematics, 76, Part 2). Ed. Gesztesy, F., Deift, P., Galvez, C., Perry, P. and Schlag, W.. American Mathematical Society, Providence, RI, 2007, pp. 613–647.CrossRefGoogle Scholar
Krikorian, R., Wang, J., You, J. and Zhou, Q.. Linearization of quasiperiodically forced circle flow beyond Brjuno condition. Comm. Math. Phys. 358 (2018), 81–100.CrossRefGoogle Scholar
Romeiras, F. J. and Ott, E.. Strange nonchaotic attractors of the damped pendulum with quasiperiodic forcing. Phys. Rev. A 35 (1987), 4404.CrossRefGoogle ScholarPubMed
Simon, B.. Almost periodic Schrödinger operators: a review. Adv. Math. 3(4) (1982), 463–490.CrossRefGoogle Scholar
Wang, J., Zhou, Q. and Jäger, T.. Genericity of mode-locking for quasiperiodically forced circle maps. Adv. Math. 348 (2019), 353–377.CrossRefGoogle Scholar
Zhang, Z.. On topological genericity of the mode-locking phenomenon. Math. Ann. 376 (2020), 707–728.CrossRefGoogle Scholar