Hostname: page-component-848d4c4894-wg55d Total loading time: 0 Render date: 2024-06-01T20:50:14.878Z Has data issue: false hasContentIssue false
  • English
  • Français

Generic homeomorphisms have full metric mean dimension

Part of: Special properties Smooth dynamical systems: general theory Connections with other structures, applications Topological dynamics

Published online by Cambridge University Press:  28 December 2020

MARIA CARVALHO Open the ORCID record for MARIA CARVALHO [Opens in a new window] ,
FAGNER B. RODRIGUES  and
PAULO VARANDAS Open the ORCID record for PAULO VARANDAS [Opens in a new window]
MARIA CARVALHO
Affiliation:
Departamento de Matemática, Universidade do Porto, Porto, Portugal (e-mail:mpcarval@fc.up.pt)
FAGNER B. RODRIGUES
Affiliation:
Departamento de Matemática, Universidade Federal do Rio Grande do Sul, Porto Alegre, Brazil (e-mail:fagnerbernardini@gmail.com)
PAULO VARANDAS*
Affiliation:
Departamento de Matemática e Estatística, Universidade Federal da Bahia, Salvador, Brazil Centro de Matemática da Universidade do Porto (CMUP), Porto, Portugal
*
e-mail:paulo.varandas@ufba.br
Get access Rights & Permissions [Opens in a new window]

Abstract

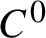We prove that for $C^0$ -generic homeomorphisms, acting on a compact smooth boundaryless manifold with dimension greater than one, the upper metric mean dimension with respect to the smooth metric coincides with the dimension of the manifold. As an application, we show that the upper box dimension of the set of periodic points of a $C^0$ -generic homeomorphism is equal to the dimension of the manifold. In the case of continuous interval maps, we prove that each level set for the metric mean dimension with respect to the Euclidean distance is 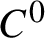$C^0$ -dense in the space of continuous endomorphisms of $[0,1]$ with the uniform topology. Moreover, the maximum value is attained at a 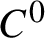$C^0$ -generic subset of continuous interval maps and a dense subset of metrics topologically equivalent to the Euclidean distance.

Keywords

metric mean dimensionpseudo-horseshoetopological dynamics

MSC classification

Primary: 37C45: Dimension theory of dynamical systems 54H20: Topological dynamics
Secondary: 37B40: Topological entropy 54F45: Dimension theory
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 42 , Issue 1 , January 2022 , pp. 40 - 64
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Carvalho, M., Rodrigues, F. and Varandas, P.. A variational formula for the metric mean dimension of free semigroup actions. Preprint, 2019. Ergod. Th. & Dynam. Sys. to appear.Google Scholar
Falconer, K.. Fractal Geometry: Mathematical Foundations and Applications, 2nd edn. John Wiley & Sons, Chichester, 2003.CrossRefGoogle Scholar
Gromov, M.. Topological invariants of dynamical systems and spaces of holomorphic maps I. Math. Phys. Anal. Geom. 2(4) (1999), 323–415.CrossRefGoogle Scholar
Gutman, Y.. Embedding ${\mathbb{Z}}^k$ -actions in cubical shifts and ${\mathbb{Z}}^k$ -symbolic extensions. Ergod. Th. & Dynam. Sys. 31 (2011), 383–403.CrossRefGoogle Scholar
Gutman, Y., Lindenstrauss, E. and Tsukamoto, M.. Mean dimension of ${\mathbb{Z}}^k$ -actions. Geom. Funct. Anal. 26(3) (2016), 778–817.CrossRefGoogle Scholar
Gutman, Y., Qiao, Y. and Szabó, G.. The embedding problem in topological dynamics and Takens’ theorem. Nonlinearity 31 (2018), 597–620.CrossRefGoogle Scholar
Gutman, Y., Qiao, Y. and Tsukamoto, M.. Application of signal analysis to the embedding problem of ${\mathbb{Z}}^k$ -actions. Geom. Funct. Anal. 29 (2019), 1440–1502.CrossRefGoogle Scholar
Hurley, M.. On proofs of the ${C}^0$ general density theorem. Proc. Amer. Math. Soc. 124(4) (1996), 1305–1309.CrossRefGoogle Scholar
Kennedy, J. and Yorke, J.. Topological horseshoes. Trans. Amer. Math. Soc. 353(6) (2001), 2513–2530.CrossRefGoogle Scholar
Lima, H. and Varandas, P.. On the rotation sets of generic homeomorphisms on the torus ${T}^d$ . Preprint, 2019, arXiv:1901.00396. Ergod. Th. & Dynam. Sys. to appear.CrossRefGoogle Scholar
Lindenstrauss, E.. Mean dimension, small entropy factors and embedding main theorem. Publ. Math. Inst. Hautes Études Sci. 89(1) (1999), 227–262.CrossRefGoogle Scholar
Lindenstrauss, E. and Tsukamoto, M.. From rate distortion theory to metric mean dimension: variational principle. IEEE Trans. Inform. Theory 64(5) (2018), 3590–3609.CrossRefGoogle Scholar
Lindenstrauss, E. and Weiss, B.. Mean topological dimension. Israel J. Math. 115 (2000), 1–24.CrossRefGoogle Scholar
Misiurewicz, M.. Horseshoes for continuous mappings of an interval. Dynamical Systems Lectures (CIME Summer Schools, 78). Ed. Marchioro, C.. Springer, Berlin, 2010, pp. 127–135.Google Scholar
Munkres, J.. Obstructions to the smoothing of piecewise-differentiable homeomorphisms. Ann. of Math. 72 (1960), 521–554.CrossRefGoogle Scholar
Pugh, C.. The closing lemma. Amer. J. Math. 89(4) (1967), 956–1009.CrossRefGoogle Scholar
Šalát, T., Tóth, J. and Zsilinszky, L.. Metric space of metrics defined on a given set. Real Anal. Exchange 18(1) (1992–1993), 225–231.CrossRefGoogle Scholar
Velozo, A. and Velozo, R.. Rate distortion theory, metric mean dimension and measure theoretic entropy. Preprint, 2019, arXiv:1707.05762.Google Scholar
Walters, P.. An Introduction to Ergodic Theory. Springer, New York, 1982.CrossRefGoogle Scholar
Yano, K.. A remark on the topological entropy of homeomorphisms. Invent. Math. 59 (1980), 215–220.CrossRefGoogle Scholar