Hostname: page-component-848d4c4894-ttngx Total loading time: 0 Render date: 2024-05-16T21:52:24.228Z Has data issue: false hasContentIssue false
  • English
  • Français

Substreetutions and more on trees

Part of: Topological dynamics Graph theory

Published online by Cambridge University Press:  08 April 2024

ALEXANDRE BARAVIERA Open the ORCID record for ALEXANDRE BARAVIERA [Opens in a new window]  and
RENAUD LEPLAIDEUR Open the ORCID record for RENAUD LEPLAIDEUR [Opens in a new window]
ALEXANDRE BARAVIERA
Affiliation:
Instituto de Matemática e Estatística - UFRGS, Avenida Bento Gonçalves, 9500, Porto Alegre CEP 91509-900, RS, Brazil (e-mail: baravi@mat.ufrgs.br)
RENAUD LEPLAIDEUR*
Affiliation:
ISEA, Université de la Nouvelle-Calédonie & LMBA CNRS UMR6205, Nouméa, New Caledonia
*
e-mail: renaud.leplaideur@unc.nc
Get access Rights & Permissions [Opens in a new window]

Abstract

We define a notion of substitution on colored binary trees that we call substreetution. We show that a point fixed by a substreetution may (or not) be almost periodic, and thus the closure of the orbit under the 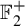$\mathbb {F}_{2}^{+}$-action may (or not) be minimal. We study one special example: we show that it belongs to the minimal case and that the number of preimages in the minimal set increases just exponentially fast, whereas it could be expected a super-exponential growth. We also give examples of periodic trees without invariant measures on their orbit. We use our construction to get quasi-periodic colored tilings of the hyperbolic disk.

Keywords

tree shiftentropygroup actionminimal systems

MSC classification

Primary: 05C05: Trees
Secondary: 37B05: Transformations and group actions with special properties (minimality, distality, proximality, etc.)
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , First View , pp. 1 - 55
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baraviera, A., Leplaideur, R. and Lopes, A.. The potential point of view for renormalization. Stoch. Dyn. 12(4) (2012), 1250005.CrossRefGoogle Scholar
Beckus, S., Hartnick, T. and Pogorzelski, F.. Symbolic substitution systems beyond abelian groups. Preprint, 2021, arXiv:2109.15210.Google Scholar
Bédaride, N. and Hilion, A.. Geometric realizations of 2-dimensional substitutive tilings. Q. J. Math. 64(4) (2013), 955–979.CrossRefGoogle Scholar
Benjamini, I. and Peres, Y.. Markov chains indexed by trees. Ann. Probab. 22(1) (1994), 219–243.CrossRefGoogle Scholar
Berstel, J., Boasson, L., Carton, O. and Fagnot, I.. A first investigation of Sturmian trees. STACS 2007: Proceedings 24th Annual Symposium on Theoretical Aspects of Computer Science (Aachen, Germany, February 22–24, 2007). Ed. Thomas, W. and Weil, P.. Springer, Berlin, 2007, pp. 73–84.CrossRefGoogle Scholar
Bruin, H. and Leplaideur, R.. Renormalization, thermodynamic formalism for quasi-crystals in subshifts. Comm. Math. Phys. 231 (2013), 209–247.CrossRefGoogle Scholar
Bruin, H. and Leplaideur, R.. Renormalization, freezing phase transition and Fibonacci quasicrystals. Ann. Sci. Éc. Norm. Supér. (4) 48(fascicule 3) (2015), 739–763.CrossRefGoogle Scholar
Dal’Bo, F.. Geodesic and Horocyclic Trajectories (Universitext). Springer-Verlag, London, 2011; EDP Sciences, Les Ulis.CrossRefGoogle Scholar
Damm, W.. The IO- and OI-hierarchies. Theoret. Comput. Sci. 20 (1982), 95–207.CrossRefGoogle Scholar
de Vries, J.. Elements of Topological Dynamics. Springer, Dordrecht, 1993.CrossRefGoogle Scholar
Ellis, D. B., Ellis, R. and Nerurkar, M.. The topological dynamics of semigroup actions. Trans. Amer. Math. Soc. 353(4) (2001), 1279–1320.CrossRefGoogle Scholar
Fogg, P.. Substitutions in Dynamics, Arithmetics and Combinatorics (Lecture Notes in Mathematics, 1794). Springer, Berlin, 2002.CrossRefGoogle Scholar
Furstenberg, H. and Weiss, B.. Markov processes and Ramsey theory for trees. Combin. Probab. Comput. 12 (2003), 547–563.CrossRefGoogle Scholar
Katok, A. and Hasselblatt, B.. Introduction to the Modern Theory of Dynamical Systems. Cambridge University Press, Cambridge, 2010.Google Scholar
Kim, D. H., Lee, B., Lim, S. and Sim, D.. Quasi–Sturmian colorings on regular trees. Ergod. Th. & Dynam. Sys. 40(12) (2020), 3403–3419.CrossRefGoogle Scholar
Krylov, N. and Bogolubov, N.. La théorie générale de la mesure dans son application à l’étude des systèmes dynamiques de la mécanique non linéaire. Ann. of Math. (2) 38 (1937), 65–113.CrossRefGoogle Scholar
Müllner, C. and Yassawi, R.. Automorphisms of automatic shifts. Ergod. Th. & Dynam. Sys. 41 (2021), 1530–1559.CrossRefGoogle Scholar
Nguyen, Q. V. and Huang, M. L.. Space optimized tree: a connection+enclosure approach for the visualization of large hierarchies. Inform. Vis. 2 (2003), 3–15.CrossRefGoogle Scholar
Petersen, K. and Salama, I.. Entropy on regular trees. Discrete Contin. Dyn. Syst. 40(7) (2020), 4453–4477.CrossRefGoogle Scholar
Przytycki, F.. Conical limit set and Poincaré exponent for iterations of rational functions. Trans. Amer. Math. Soc. 351(5) (1999), 2081–2099.CrossRefGoogle Scholar
Przytycki, F., Rivera-Letelier, J. and Smirnov, S.. Equality of pressures for rational functions. Ergod. Th. & Dynam. Sys. 24(3) (2004), 891–914.CrossRefGoogle Scholar
Queffelec, M.. Substitution Dynamical Systems—Spectral Analysis (Lecture Notes in Mathematics, 1294). Springer, Berlin, 2010.CrossRefGoogle Scholar
Rozikov, U. A.. Gibbs Measures on Cayley Trees. World Scientific, Singapore, 2013.CrossRefGoogle Scholar
Spitzer, F.. Markov random fields on an infinite tree. Ann. Probab. 3(5) (1975), 387–398.CrossRefGoogle Scholar