Hostname: page-component-848d4c4894-tn8tq Total loading time: 0 Render date: 2024-06-15T05:31:02.308Z Has data issue: false hasContentIssue false
  • English
  • Français

Fixed point sets and the fundamental group I: semi-free actions on G-CW-complexes

Published online by Cambridge University Press:  03 August 2023

Sylvain Cappell Open the ORCID record for Sylvain Cappell [Opens in a new window] ,
Shmuel Weinberger  and
Min Yan Open the ORCID record for Min Yan [Opens in a new window]
Sylvain Cappell
Affiliation:
Courant Institute of Mathematical Sciences, New York University, New York, NY, USA (cappell@cims.nyu.edu)
Shmuel Weinberger
Affiliation:
University of Chicago, Chicago, IL, USA (shmuel@math.uchicago.edu)
Min Yan
Affiliation:
Hong Kong University of Science and Technology, Hong Kong, People's Republic of China (mamyan@ust.hk)
Get access Rights & Permissions [Opens in a new window]

Abstract

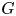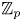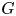Smith theory says that the fixed point set of a semi-free action of a group $G$ on a contractible space is ${\mathbb {Z}}_p$-acyclic for any prime factor $p$ of the order of $G$. Jones proved the converse of Smith theory for the case 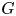$G$ is a cyclic group acting semi-freely on contractible, finite CW-complexes. We extend the theory to semi-free group actions on finite CW-complexes of given homotopy types, in various settings. In particular, the converse of Smith theory holds if and only if a certain $K$-theoretical obstruction vanishes. We also give some examples that show the geometrical effects of different types of $K$-theoretical obstructions.

Keywords

group actionsalgebraic K-theorySmith theory
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 22
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Assadi, A. H., Finite Group Actions on Simply-connected Manifolds and CW complexes. Memoirs AMS, Vol.35, no. 257 (AMS, 1982).CrossRefGoogle Scholar
Assadi, A. H. and Vogel, P.. Actions of finite groups on compact manifolds. Topology 26 (1987), 239–263.CrossRefGoogle Scholar
Bartels, A. C.. On the domain of the assembly map in the algebraic $K$-theory. Alg. Geom. Topology 3 (2003), 1037–1050.CrossRefGoogle Scholar
Bass, H.. Algebraic K-Theory (W. A. Benjamin, New York and Amsterdam, 1968).Google Scholar
Bass, H., Heller, A. and Swan, R.. The Whitehead group of a polynomial extension. Inst. Hautes Études Sci. Publ. Math. 22 (1964), 61–79.CrossRefGoogle Scholar
Bass, H. and Murthy, M. P.. Grothendieck groups and Picard groups of abelian group rings. Ann. Math. 86 (1967), 16–73.CrossRefGoogle Scholar
Cappell, S. and Shaneson, J.. Nonlinear similarity. Ann. Math. 113 (1981), 315–355.CrossRefGoogle Scholar
Cappell, S., Weinberger, S. and Yan, M., Fixed point sets and the fundamental group II: Euler characteristics. preprint, 2021.Google Scholar
Carter, D.. Lower K-theory of finite groups. Comm. Alg. 8 (1980), 1927–1937.CrossRefGoogle Scholar
Davis, J. and Lück, W.. Spaces over a category and assembly maps in isomorphism conjectures in K- and L-theory. K-Theory 15 (1998), 201–252.CrossRefGoogle Scholar
Farrell, T. and Jones, L.. Isomorphism conjectures in algebraic K-theory. JAMS 6 (1993), 249–297.Google Scholar
Jones, L.. The converse to the fixed point theorem of P.A. Smith: I. Ann. Math. 94 (1971), 52–68.CrossRefGoogle Scholar
Milnor, J., Introduction to Algebraic $K$-theory. Annals of Mathematics Studies, Vol.72 (Princeton University Press, 1972).CrossRefGoogle Scholar
Mislin, G., Wall's finiteness obstruction. In: Handbook of Algebraic Topology (Elsevier, 1995), pp. 1259–1291.CrossRefGoogle Scholar
Morimoto, M. and Iizuka, K.. Extendability of $G$-maps to pseudo-equivalences to finite $G$-CW-complexes whose fundamental groups are finite. Osaka J. Math. 21 (1984), 59–69.Google Scholar
Oliver, R.. Fixed-point sets of group actions on finite cyclic complexes. Comment. Math. Helvetici 50 (1975), 155–177.CrossRefGoogle Scholar
Oliver, R. and Petrie, T.. $G$-CW-surgery and $K_0(\mathbb {Z}G)$. Math. Z. 179 (1982), 11–42.CrossRefGoogle Scholar
Petrie, T., Pseudoequivalences of $G$-manifolds. In: Algebraic and geometric topology (Proc. Sympos. Pure Math., Stanford Univ., 1976), Part 1, 169–210, Proc. Sympos. Pure Math., 32 (Amer. Math. Soc., Providence, RI, 1978).CrossRefGoogle Scholar
Quinn, F.. Ends of maps II. Invent. Math. 68 (1982), 353–424.CrossRefGoogle Scholar
Quinn, F.. Homotopically stratified sets. JAMS 1 (1988), 441–499.Google Scholar
Rosenberg, J., Algebraic K-Theory and its Applications. Graduate Texts in Mathematics, Vol. 147 (Springer-Verlag, 1994).CrossRefGoogle Scholar
Smith, P. A.. Fixed-point theorems for periodic transformations. Amer. J. Math. 63 (1941), 1–8.CrossRefGoogle Scholar
Steinberger, M.. The equivariant $s$-cobordism theorem. Invent. Math. 91 (1988), 61–104.CrossRefGoogle Scholar
Steinberger, M. and West, J.. Equivariant $h$-cobordisms and finiteness obstructions. BAMS 12 (1985), 217–220.CrossRefGoogle Scholar
Swan, R.. Periodic resolutions for finite groups. Ann. Math. 72 (1960), 267–291.CrossRefGoogle Scholar
Swan, R. and Evans, E. G., $K$-Theory of Finite Groups and Orders. Lecture Notes in Math., Vol.149 (Springer, 1970).CrossRefGoogle Scholar
Ullom, S.. Nontrivial lower bounds for class groups of integral group rings. Illinois J. Math. 2 (1976), 361–371.Google Scholar
Wall, C. T. C.. Finiteness conditions for CW-complexes. Ann. Math. 81 (1965), 56–69.CrossRefGoogle Scholar
Weinberger, S., Constructions of group actions: a survey of some recent developments. Group actions on manifolds (Boulder, Colo., 1983), pp. 269–298 Contemp. Math., 36 (Amer. Math. Soc., Providence, RI, 1985).CrossRefGoogle Scholar
West, J.. Mapping Hilbert cube manifolds to ANR's: a solution of a conjecture of Borsuk. Ann. Math. 106 (1977), 1–18.CrossRefGoogle Scholar