Introduction
Permittivity and permeability are two important properties of natural material for characterising their electric and magnetic responses in electromagnetic (EM) waves. For natural materials, these two parameters are usually larger than or equal to those in free space, thus the incident EM waves obey the traditional Snell’s laws and show the ordinary EM reflections and refractions. Veselago (Reference Veselago1968) proposed theoretical analyses on the left-handed materials with negative permittivity and permeability, which exhibit many exotic physical phenomena such as negative refraction, negative Doppler effect, and backward Cherenkov diffraction. At the end of the 20th century, Sir John Pendry and co-workers have successively proposed a series of physical models and experimental verification methods for realising the first metamaterials (Pendry et al., Reference Pendry, Holden, Stewart and Youngs1996; Pendry, Reference Pendry2000; Smith et al., Reference Smith, Padilla, Vier, Nemat-Nasser and Schultz2000, Reference Smith, Pendry and Wiltshire2004; Shelby et al., Reference Shelby, Smith and Schultz2001), which later ignited the extensive researches on metamaterial.
Metamaterials are artificial EM materials and are composed of subwavelength-scale unit cells (namely meta-atoms) periodically or aperiodically distributed in space. The early metamaterials are usually designed in three-dimensional (3D) forms, in which the phases accumulate in the 3D space and change in the space gradually. Figure 1a shows a classical meta-atom composed of a dielectric plate with drilled hole (Ma and Cui, Reference Ma and Cui2010). When different materials and height are selected, the effective refractive index of such meta-atoms can be acquired via S-parameter retrieval method based on effective medium theory (Smith et al., Reference Smith, Schultz, Markoš and Soukoulis2002; Chen et al., Reference Chen, Grzegorczyk, Wu, Pacheco and Kong2004; Liu et al., Reference Liu, Cui, Huang, Zhao and Smith2007; Ma and Cui, Reference Ma and Cui2010). Such meta-atoms with desired effective permittivity and permeability are periodically arranged in the 3D space to realise different controls of EM wave. For example, the 3D broadband and broad-angle transformation-optics lens in Figure 1b can achieve the beam scanning function in a wide frequency band from 12.5 to 18 GHz (Figure 1c). The emergence of metamaterials has brought many exotic physical phenomena and engineering applications that cannot be realised with the naturally materials, such as perfect lenses, invisibility cloaking, zero-refractive-index phenomena, and so on (Schurig et al., Reference Schurig, Mock, Justice, Cummer, Pendry, Starr and Smith2006; Liu et al., Reference Liu, Ji, Mock, Chin, Cui and Smith2009; Kundtz and Smith, Reference Kundtz and Smith2010; Ma and Cui, Reference Ma and Cui2010; Ramaccia et al., Reference Ramaccia, Scattone, Bilotti and Toscano2013).

Figure 1. 3D broadband and broad-angle transformation-optics lens. Reproduced from Ma and Cui (Reference Ma and Cui2010). (a) Meta-atom design and the relationship between refractive index and diameter of the drilled hole. (b) Graph of the 3D transformation-optics lens. (c) Beam-scanning performances at 12.5, 15 and 18 GHz.
However, the 3D metamaterials have some drawbacks such as complicated design, bulky size and difficult integration with other devices. To address these disadvantages, two-dimensional (2D) metamaterials, namely metasurfaces, have been proposed with a low-profile property (Holloway et al., Reference Holloway, Kuester, Gordon, O'Hara, Booth and Smith2012; Ding et al., Reference Ding, Pors and Bozhevolnyi2017). As the thickness of metasurface is typically less than a tenth of a wavelength, traditional approaches for analysing 3D metamaterials are no longer valid for metasurfaces. Yu et al. (Reference Yu, Genevet, Kats, Aieta, Tetienne, Capasso and Gaburro2011) proposed the generalised Snell’s law as a rapid while efficient approach for designing metasurfaces, which utilises the abrupt phase changes at the metasurface to control the shape and direction of EM wavefront, realising excellent beam deflecting and vortex-beam radiations (Figure 2). By elaborately designing phase profiles on metasurfaces, the reflected or transmitted waves can be tailored at will to achieve designed EM functions such as beamforming, beam steering, random refraction, and imaging (Sun et al., Reference Sun, He, Xiao, Xu, Li and Zhou2012; Pfeiffer and Grbic, Reference Pfeiffer and Grbic2013; Zheng et al., Reference Zheng, Mühlenbernd, Kenney, Liu, Zentgraf and Zhang2015; Chen et al., Reference Chen, Feng, Monticone, Zhao, Zhu, Jiang, Zhang, Kim, Ding, Zhang, Alu and Qiu2017; Kim et al., Reference Kim, Yang, Badloe, Kim, Yoon and Rho2021; Zheng et al., Reference Zheng, Dai, Li, Ye, Xiong, Liu, Zheng and Zhang2021; Luo et al., Reference Luo, Hu, Ou, Li, Lai, Liu, Cheng, Pan and Duan2022). Besides phase control, many other attributes of EM waves such as amplitude, polarisation state and frequency have also been controlled to implement multi-dimensional EM controls (Liu et al., Reference Liu, Zhang, Kenney, Su, Xu, Ouyang, Shi, Han, Zhang and Zhang2014; Wan et al., Reference Wan, Jia, Cui and Zhou2016a; Xu et al., Reference Xu, Li, Wang and Han2018, Reference Xu, Hu, Wang, Wang, Wang, Wang, Huang, Genevet, Huang and Qiu2021; Xu et al.,(Reference Xu, Hu, Han, Jiang, Huang, Li, Yang, Ling, Chen, Zhao and Qi2019a, Reference Xu, Hu, Li, Han, Zhao, Sun, Yuan, Wang, Jiang, Ling, Cui and Qiu2019b); Wu et al.,(Reference Wu, Bao, Wu, Wang, Ma, Wu, Bai, Galdi and Cui2020b) Li et al., Reference Li, Han, Li, Liu, Huang, Li and Cao2022), realising more complicated and multi-functional devices and systems.

Figure 2. (a) Refraction schematics of the generalised Snell’s law. Reproduced from Yu et al. (Reference Yu, Genevet, Kats, Aieta, Tetienne, Capasso and Gaburro2011). (b) Gradient phase abrupt to achieve deflecting wave propagation. (c) Specific analysis of the generalised Snell’s law for anomalous reflection and transmission. (d) Realisations of vortex beams using spiral phase pattern.
With the vast designs of metamaterial having different structures and exotic EM properties, many attempts have also been devoted to achieve reconfigurable metamaterial with multiple functionalities integrated in a single metasurface. In the early times, researchers could adjust EM coefficients in a small range by loading varactors (Zhao et al., Reference Zhao, Cheng, Chen, Qi, Jiang and Cui2013). Then, more complicated active components and control approaches allow the EM manipulations to be reconfigurable among several functions (Oliveri et al., Reference Oliveri, Werner and Massa2015; Bang et al., Reference Bang, Kim, Yoon, Tanaka and Rho2018). But the controlling approaches are still analog, and the types and number of functions are still limited.
Cui et al. (Reference Cui, Qi, Wan, Zhao and Cheng2014) proposed the concept of coding and digital metamaterials/metasurfaces. Distinguished from the traditionally continuous phase representations, discrete digits are adopted here to represent the reflected or transmitted phases. Figure 3a shows a 1-bit coding metamaterial, in which the phase difference of the meta-atoms with digital states ‘0’ and ‘1’ is around 180°. Herein, these binary meta-atoms are arranged in a 2D plane with pre-designed coding patterns to achieve desired EM functionalities. Figure 3b shows three coding patterns ‘000000…/000000…’, ‘010101…/010101…’, ‘010101…/101010…’, and the realised single-beam, dual-beam, four-beam radiations. Due to the Fourier transform relationship between the far-field radiation pattern and the coding pattern of coding metamaterials, digital information embedded in coding patterns is directly manifested in the radiation patterns. In addition, some researches have also focused on the fundamental problems about the influence of digital and discrete properties in practical engineering. Shuang et al. (Reference Shuang, Zhao, Wei, Cheng, Jin, Cui, Hougne and Li2022) demonstrated that the 1-bit coding can satisfy the requirements in most practical scenarios compared to continuous phase control. One can even adopt optimised ways or extend 1-bit to multi-bit states for more precise manipulations and better performances (Cui et al., Reference Cui, Qi, Wan, Zhao and Cheng2014; Yang et al., Reference Yang, Xu, Yang, Cao, Xu, Gao and Li2017). Combined with the information science, some methods in signal processing such as convolution and addition theorems have been introduced to assist coding pattern design to achieve more flexible EM information manipulations (Liu et al., Reference Liu, Cui, Zhang, Xu, Wang, Wan, Gu, Tang, Qi, Han, Zhang, Zhou and Cheng2016b; Wu et al., Reference Wu, Shi, Liu, Wu and Cui2018). The concept of information entropy has also been introduced to measure the information capacity of coding metamaterials (Cui et al., Reference Cui, Liu and Li2016). Further, fast algorithm was adopted to optimise the coding pattern and achieve automatic pattern design (Moccia et al., Reference Moccia, Liu, Wu, Castaldi, Andreone, Cui and Galdi2017; Jing et al., Reference Jing, Li, Zhang, Wang, Feng, Sui, Qiu, Ma and Qu2018; Zhang et al., Reference Zhang, Liu, Wan, Zhang, Liu, Yang and Cui2019c). Besides phase coding, other important EM properties such as amplitude (Bao et al., Reference Bao, Ma, Bai, Jing, Wu, Fu, Yang, Wu and Cui2018; Wu et al.,(Reference Wu, Bao, Wu, Wang, Ma, Wu, Bai, Galdi and Cui2020b)), polarisation (Liu et al., Reference Liu, Cui, Xu, Bao, Du, Wan, Tang, Ouyang, Zhou, Yuan, Ma, Jiang, Han, Zhang and Cheng2016a; Ma et al., Reference Ma, Shi, Bai, Chen, Noor and Cui2017) and frequency (Liu et al. (Reference Liu, Zhang, Yang, Xu, Yang, Noor, Zhang, Iqbal, Wan, Tian, Tang, Cheng, Han, Zhang and Cui2016c); Wu et al., Reference Wu, Liu, Wan, Zhang, Wang, Li and Cui2017) have been jointly controlled to realise many other interesting functionalities, including novel multi-functional, power-controllable, broadband or multi-band coding metamaterials (Cui et al., Reference Cui, Wu, Wu, Shi and Li2017b; Wu et al., Reference Wu, Bao, Wu and Cui2020a). Due to the strong connection with information science, coding and digital metamaterials are also referred to as information metamaterials (Cui et al., Reference Cui, Liu and Zhang2017a; Liu and Cui, Reference Liu and Cui2017; Zhang et al., Reference Zhang, Liu and Cui2017; Cui, Reference Cui2018; Ma and Cui, Reference Ma and Cui2020; Wu and Cui, Reference Wu and Cui2020).

Figure 3. (a) Schematic of coding and digital metamaterials/metasurfaces and a typical 1-bit meta-atom. (b) Three coding patterns ‘000000…/000000…’, ‘010101…/010101…’, ‘010101…/101010…’, and corresponding single-beam, dual-beam, four-beam radiations. (c) Meta-atom of 1-bit programmable metamaterial. (d) Real-time control by programmable metamaterial. Reproduced from Cui et al. (Reference Cui, Qi, Wan, Zhao and Cheng2014).
More importantly, the digital and discrete properties of information metamaterials allow us to reach more effective and dynamic controls in the programmable manner. As shown in Figure 3c,d, by controlling the bias voltage across the loaded active components on the meta-atom, their digital state can be dynamically controlled. Coding patterns are loaded to the coding metamaterials via field programmable gate arrays (FPGA) to achieve real-time and digital manipulations on the far-field radiations (Cui et al., Reference Cui, Qi, Wan, Zhao and Cheng2014). Hence, the term ‘programmable metamaterials’ also emerges in some other contexts as ‘information metamaterials’ (Cui, Reference Cui2017; Li and Cui, Reference Li and Cui2019; Bao and Cui, Reference Bao and Cui2020; Cui et al., Reference Cui, Li, Liu, Ma, Zhang, Wan, Jiang and Cheng2020). The proposal of information metamaterials facilitates the development of the programmable metamaterials, which can provide more flexible and powerful EM manipulating abilities and information processing capacities.
In this article, we focus on programmable metamaterials, including their theoretical concepts, implementing methods, and applications in EM controls. First, we introduce the evolution of metamaterials and metasurfaces from the passive designs to digital coding representations, leading to the information and programmable metamaterials and metasurfaces. Then, space-domain, time-domain and space–time programmable metamaterials and metasurfaces are discussed in details, including the corresponding theories, functionalities, experimental implementations, and system-level applications. Several novel programmable technologies of meta-atom design and driven methods are emphatically analysed. Finally, we give a summary and a prospect of the programmable metamaterials and metasurfaces for the future studies and application potentials.
Programmable metamaterials for EM manipulations
A brief introduction of programmable metamaterial has been given in first section. In this section, we will explain the design methods and functionalities of the programmable metamaterials in the space domain, time domain, and space–time domain.
Space-domain programmable metamaterials
Space-domain programmable metamaterials are the most common form of programmable metamaterials, in which the coding pattern formed on the entire aperture is simultaneous in time, and the EM responses of each meta-atom in different positions are controlled by FPGA. For example, we can achieve real-time anomalous beam reflection through by loading coding patterns to programmable metamaterials based on the generalised Snell’s law (Cui et al., Reference Cui, Qi, Wan, Zhao and Cheng2014). Various space-domain programmable metamaterials have been designed that can realise many different EM manipulating functionalities, as illustrated in the following.
Phase programmable metamaterials
Phase is the most popular coefficient that is engineered in metamaterial designs. Figure 4a shows a typical phase programmable meta-atom from Wan et al. (Reference Wan, Jia, Cui and Zhou2016a), where a positive-intrinsic-negative (PIN) diode is loaded between two metallic patches. The working states of PIN diode are dynamically controlled by external voltages through the feeding lines. Equivalent circuits of the PIN diode at the ‘OFF’ and ‘ON’ state are shown in the inset of Figure 4a, which come from the datasheet of PIN diode and are verified by the co-simulation of EM wave and circuit. The reflecting performances of the meta-atom are shown in Figure 4b, showing that the meta-atom can perform digital state ‘0’ and ‘1’ with 180° phase difference with large reflection amplitude when the PIN diode is switched. The spatial coding pattern on the metamaterial composed of 400 binary meta-atoms can be modulated in different forms to achieve different radiation patterns with different deflecting angles (Figure 4c,d). Note that space-domain phase programmable scheme is not restricted to reflection type, Bai et al. (Reference Bai, Kong, Sun, Wang, Qian, Li, Cao, He, Liang, Jin and Zhu2020) and Chen et al. (Reference Chen, Ma, Nie, Hong, Cui, Ruan and Cui2021) show a series designs of transmission-type programmable metamaterials, which is achieved by stacking more layers to provide required phase changes for the transmitted wave. As displayed in Figure 4e, the PIN diodes are loaded on the radiating layer to modulate the incidence from the receiving layer with 1-bit phase difference. This type of meta-atom is more suitable for array applications due to the relatively independent feed-line design, which effectively decreases the complexity of the transmission-type programmable metamaterials.

Figure 4. (a) Detailed structure of the binary programmable meta-atom. (b) Reflected amplitude and phase responses of the binary meta-atom. (c,d) Different coding patterns and corresponding radiation patterns. Reproduced from Wan et al. (Reference Wan, Jia, Cui and Zhou2016a). (e) Transmission-type programmable meta-atom. Reproduced from Bai et al. (Reference Bai, Kong, Sun, Wang, Qian, Li, Cao, He, Liang, Jin and Zhu2020).
Amplitude and polarisation programmable metamaterials
Besides phase programmable metamaterials, amplitude and polarisation of EM waves can provide extra degree of freedom to EM manipulation. Hong et al. (Reference Hong, Ma, Gao, Liu, Xiao, Iqbal and Cui2021) proposed an amplitude-programmable metamaterial with multi-frequency modulations, as illustrated in Figure 5a. Two PIN diodes and chip attenuators are integrated in the meta-atom. When the diodes are respectively in ‘On’ and ‘OFF’ states, the meta-atom is encoded as ‘11’, ‘10/01’ and ‘00’, indicating the 1-bit amplitude modulations (high amplitude as ‘1’ and low amplitude as ‘0’) at 2.98, 4.11 and 5.73 GHz, respectively. For experimental verification, a metamaterial array with 10 × 10 elements is fabricated. By changing different coding patterns in real-time, the powers of radiating beams at different frequencies can be controlled independently. Further, a new wireless communication system is designed based on the amplitude programmable metamaterial (Hong et al., Reference Hong, Ma, Gao, Liu, Xiao, Iqbal and Cui2021).

Figure 5. (a) Amplitude-coding programmable metamaterial with multifrequency modulations. Reproduced from Hong et al. (Reference Hong, Ma, Gao, Liu, Xiao, Iqbal and Cui2021). (b) Amplitude-phase-joint-coding programmable metamaterial. Reproduced from Wang et al. (Reference Wu, Ma, Wu, Xiao, Gou, Wang, Wang, Bao, Wang, Qing and Cui2022d). (c) Polarisation-controlled dual-programmable metamaterial. Reproduced from Zhang et al. (Reference Zhang, Yu, Jiang, Sun, Bai, Wang, Qiu and Cui2020b). (d) Programmable metamaterial for digital polarisation conversion. Reproduced from Ma et al. (Reference Ma, Hong, Bai, Jing, Wu, Bao, Cheng and Cui2020a).
Amplitude coding scheme is also able to join with phase coding to achieve more complicated functionalities (Liao et al., Reference Liao, Guo, Yuan, Ji, Huang and Luo2022; Wang et al., Reference Wang, Zhang, Zhang, Ma and Cui2022a). Figure 5b shows a novel amplitude-phase-joint-coding programmable metamaterial using a PIN diode loaded on a single-layer reflection-type meta-atom, which can be equivalent to a rheostat (Wang et al., Reference Wang, Zhang, Zhang, Ma and Cui2022a). When the bias voltage changes from 0 V to 1 V, the effective resistance changes from 1 Ω to 10,000 Ω, enabling the realisation of 1-bit phase coding with continuous amplitude control in a wide frequency range of 8–13 GHz. With such techniques, both the radiation patterns and power can be controlled simultaneously, allowing even more complicated functionalities such as diffraction order control and side-lobe suppression.
For polarisation-programmable metamaterials, dynamic manipulations of EM waves can be performed on differently polarised states (Yang et al., Reference Yang, Cao, Yang, Gao, Xu, Li, Chen, Zhao, Zheng and Li2016; Ma et al., Reference Ma, Hong, Gao, Jing, Liu, Bai, Cheng and Cui2020b; Zhang et al., Reference Zhang, Yu, Jiang, Sun, Bai, Wang, Qiu and Cui2020b). Zhang et al., Reference Zhang, Yu, Jiang, Sun, Bai, Wang, Qiu and Cui2020b demonstrate a polarisation-controlled dual-programmable metamaterial, which can be applied in exclusive-OR operation, wide-angle dual-beam scanning and dual-polarised aperture sharing, as shown in Figure 5c. Four PIN diodes are centrally arranged in the meta-atom to achieve independent 1-bit-phase controls of x- and y-polarizations. Ma et al. (Reference Ma, Hong, Gao, Jing, Liu, Bai, Cheng and Cui2020b) give another design of the polarisation programmable metamaterial to achieve polarisation conversion. Polarisation state of each meta-atom is determined by the PIN diode, which can be represented as ‘0’ and ‘1’ for two orthogonal polarizations. By changing the 1-bit coding sequence, namely, the percentage of x- or y-polarised meta-atoms, real-time polarisation conversions are achieved, as shown in Figure 5d.
Full-space programmable metamaterials
The abovementioned reflection- or transmission-type programmable metamaterials can only conduct EM manipulations in half space. Hence, full-space controllable metasurfaces are proposed by considering the reflection and transmission spaces together (Li et al., Reference Li, Dai, Mehmood, Hu, Yanchuk, Tao, Hao, Kim, Jeong, Zheng, Yu, Alu, Rho and Qiu2018; Wu et al., Reference Wu, Zhang, Bao, Wu, Ma, Bai, Wu and Cui2019; Shang et al., Reference Shang, Hu, Guan, Wang, Zhang, Wu, Liu, Ding, Burokur, Li, Ding and Qiu2022), which are then promoted to the programmable cases. The first design is illustrated in Figure 6a, which can realise the real-time switching of working space (i.e., radiation space). The meta-atom is composed of multiple square-patch layers for phase manipulation, together with a slot layer with a loaded PIN diode for space switching (Figure 6b). When the PIN diode is switched to the ‘ON’ and ‘OFF’ states with different equivalent circuits, the meta-atom performs as 1-bit reflection-type phase coding and 2-bit transmission-type phase coding, respectively. By designing the corresponding phase-coding patterns, the full-space programmable metamaterial can achieve real-time switching between transmitting beam deflection and random reflection (Wu et al., Reference Wu, Zhang, Bao, Wu, Ma, Bai, Wu and Cui2019).

Figure 6. (a,b) Illustration of full-space reflection-transmission amplitude programmable metamaterial and the structure of adopted meta-atom. Reproduced from Wu et al. (Reference Wu, Zhang, Bao, Wu, Ma, Bai, Wu and Cui2019). (c,d) Illustration of totally full-space programmable metamaterial and the structure of adopted meta-atom. Reproduced from Bao et al. (Reference Bao, Ma, Wu, Fu, Wu and Cui2021).
Inspired by this work (Wu et al., Reference Wu, Zhang, Bao, Wu, Ma, Bai, Wu and Cui2019), more novel programmable full-space metamaterials have been proposed, such as anisotropic designs, absorbing-transmitting combining designs, and so on (Wu et al., Reference Wu, Ma, Wu, Xiao, Gou, Wang, Wang, Bao, Wang, Qing and Cui2020d; Bao et al., Reference Bao, Ma, Wu, Fu, Wu and Cui2021; Wang et al., Reference Wang, Ma, Chen, Sun and Cui2021; Hu et al., Reference Hu, Chen, Zhang, Zhao, Jiang, Zhao and Feng2022; Ma et al., Reference Ma, Hong, Gao, Xiao, Chen and Cui2022). In 2021, Bao et al. (Reference Bao, Ma, Wu, Fu, Wu and Cui2021) proposed to combine the real-time manipulations on beam reflection and beam transmission. By elaborately designing the feed-line network, a full-space phase programmable metamaterial is achieved by controlling the coding patterns on reflection side and transmission side independently, leading to an independent while real-time control of reflecting and transmitting waves (Figure 6c,d). Hu et al. (Reference Hu, Zhao, Chen, Qu, Yang, Zhao, Jiang and Feng2022) proposed the novel concept of programmable omni-metasurface, which can integrate the reflection mode, transmission mode, and duplex modes of simultaneous reflection and transmission. It can also provide the full-space EM controls and indicate a multiuser wireless communication system for two separate spaces.
Time-domain and space–time programmable metamaterials
Space-domain programmable metamaterials allow the dynamic manipulations on coding patterns and corresponding EM functions, but stable in time domain. According to Fourier transform theory, introduction of time-modulated signals with periodic variations can control EM waves in time domain and thus generate responses in the frequency domain, even simultaneous controls of EM directions in space domain and spectrum in frequency domain together. Hence, time-domain and space–time programmable metamaterials are introduced to achieve multi-dimensional complicated manipulations of the EM waves.
Theory and examples of space–time programmable metamaterials
In Zhao et al. (Reference Zhao, Yang, Dai, Cheng, Li, Qi, Ke, Bai, Liu, Jin, Alu and Cui2018), the theoretical analysis of time-domain programmable metamaterials is formulated as:
 $$\begin{align} {E}_r(f) = \boldsymbol{\varGamma} \left(f-{f}_c\right) = \sum \limits_{k = -\infty}^{\infty}\mathrm{PF}\times \mathrm{TF}\cdot \delta \left(\kern2pt f-{f}_c-{kf}_0\right), \end{align}$$
$$\begin{align} {E}_r(f) = \boldsymbol{\varGamma} \left(f-{f}_c\right) = \sum \limits_{k = -\infty}^{\infty}\mathrm{PF}\times \mathrm{TF}\cdot \delta \left(\kern2pt f-{f}_c-{kf}_0\right), \end{align}$$
where Er (f) is the reflected waves, f 0 and fc are modulation frequency and incident frequency, respectively, PF and TF represent pulse factor and time factor, respectively. In this case, spectrum of the reflection coefficient is shifted to the frequency of the incident wave. Therefore, the spectrum of the reflected wave can be controlled by designing different coding sequences in time-domain of the time-domain programmable metamaterials.
Furthermore, time-domain coding scheme can be combined with space-domain coding scheme to achieve more complex functionalities (Zhang et al., Reference Zhang, Chen, Liu, Zhang, Zhao, Dai, Bai, Wan, Cheng, Castaldi, Galdi and Cui2018). Now, each programmable meta-atom on space–time programmable metamaterials has a unique time coding sequence with period T 0, whose digital state switches periodically in time domain. According to Fourier transform theory, a periodic function in time domain produces a discrete harmonic spectrum distribution in frequency domain. When a monochromatic plane wave with frequency fc is incident on the space–time programmable metamaterials modulated by a signal with period T 0 in the time domain, the reflected energy will be distributed to the fundamental wave fc and the harmonic waves fc + mf 0, where f 0 = 1/T 0. The spatial distribution of the reflected waves at different harmonic frequencies can be manipulated independently by combining the time-domain modulation signal with the space-domain coding patterns.
Figure 7a gives an example of an 8 × 8 space–time programmable metamaterial to illustrate the working mechanism. The variation in space domain and time domain is represented by a 3D space–time coding matrix whose dimension is set as (M, N, L). M and N represent the length of the metamaterial in the spatial dimension, and L represents the length in the temporal dimension. A 3D space–time coding matrix with dimension of (8, 8, 8) can be generated as desired, as shown in Figure 7b. Green and red dots represent ‘0’ and ‘1’ digits, respectively, which correspond to 0° and 180° phase responses. For any programmable metamaterials represented by a 3D space–time coding matrix, the scattering pattern at any harmonic frequency can be calculated. Hence, we can achieve harmonic beam scanning functionality by optimising the matrix as shown in Figure 7c,d.
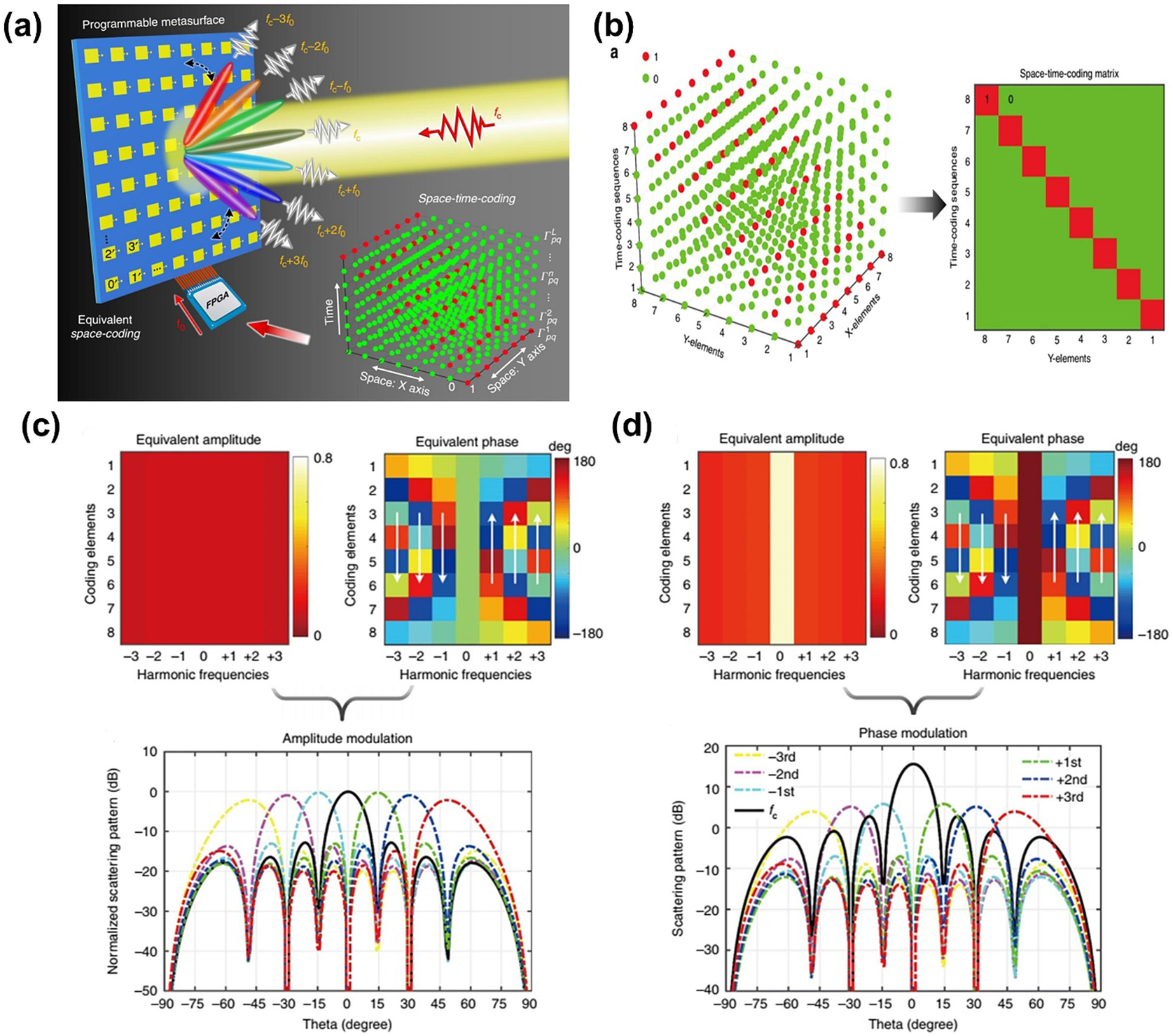
Figure 7. (a) Conceptual illustration of space–time programmable metamaterials. (b) 3D space–time coding matrix and corresponding 2D case, respectively. The red and green dots represent ‘1’ and ‘0’ states, respectively. (c) Harmonic beam scanning by amplitude modulation. (d) Harmonic beam scanning by phase modulation. Reproduced from Zhang et al. (Reference Zhang, Chen, Liu, Zhang, Zhao, Dai, Bai, Wan, Cheng, Castaldi, Galdi and Cui2018).
To give a theoretical analysis, Wu et al. (Reference Wu, Gao, Zhang, Bai, Cheng, Li and Cui2020c) studied two kinds of mechanisms of space–time programmable metamaterials from the view of information theory and analysed their unique advantages in processing EM information. The study also reveals the information conversion efficiency of the space–time programmable metamaterials, which provides theoretical guidance for information processing applications based on space–time coding programmable metamaterial, such as wireless communication, computational imaging and mathematical operations.
The structural design of meta-atom of space–time programmable metamaterials is basically similar to that of the space domain programmable metamaterials, but the time-varying properties requires faster switching speed of digital states of the meta-atom (Castaldi et al., Reference Castaldi, Zhang, Moccia, Hathaway, Tang, Cui and Galdi2021; Zhang and Cui, Reference Zhang and Cui2021).
Harmonic amplitude and phase modulations
Harmonic manipulations have been deployed in many interesting regions such as the wireless communications, and biological monitoring. Traditional harmonic manipulations are achieved by additional amplifiers and phase shifters to accurately control the harmonic amplitude and phase after the mixing process, which leads to issues such as high costs and difficult system integration. Here, a method with independent control of harmonic amplitudes and phases via a time-domain programmable metamaterial is proposed by Dai et al. (Reference Dai, Zhao, Cheng and Cui2018, Reference Dai, Yang, Tang, Chen, Ke, Cheng, Jin and Cui2020). In this method, the frequency, amplitude and phase responses of a specific harmonic wave are independently controlled, which allows us to manipulate the shape and intensity of the harmonic spectrum of the reflected wave by controlling the bias voltages V1/V2 and modulating periods T, as schematically shown in Figure 8.

Figure 8. (a) Schematic diagram of the time-domain programmable metamaterial with harmonic amplitude and phase controls. (b) The measured harmonic amplitude/phase distributions of the time-domain programmable metamaterial at 3.7 GHz with different bias voltages V1/V2 and modulating periods T. Reproduced from Dai et al. (Reference Dai, Zhao, Cheng and Cui2018).
This method serves as a guidance for time-domain programmable metamaterial to accurately control the spectrum distribution of EM waves, which extends the potential applications of time-domain programmable metamaterial and lay the foundation of time-domain programmable metamaterials for their future application in new wireless communication systems.
Multi-bit phase coding via time-domain programmable metamaterial
Generally, the higher the number of coding states of a programmable metamaterial, the smaller the phase quantization error and the more precise the EM wave can be achieved. However, the design of multi-bit (greater than 2-bit) programmable metamaterial based on active components (e.g., PIN diodes) is very challenging because total number of n PIN diodes are needed to be integrated on a single meta-atom to achieve 2n coding states, which inevitably result in complex design of structure, DC bias circuit layout and control system. An alternate approach to realise multi-bit coding functionality is to use varactor diodes, but it suffers from larger loss and larger reverse bias voltages. In 2019, a method of multi-bit phase coding based on 2-bit time-domain programmable metamaterial was presented (Zhang et al., Reference Zhang, Wang, Shao, Shen, Chen, Wan, Cheng and Cui2019b). Through vector synthesis analysis in Figure 9a, the EM incidence can be modulated to the outgoing wave with arbitrary phase bit as shown in Figure 9a,b. Such a working scheme is also applied in arbitrary polarisation construction (Ke et al., Reference Ke, Dai, Chen, Wang, Zhang, Tang, Yang, Liu, Li, Lu, Cheng, Jin and Cui2021; Hu et al., Reference Hu, Zhao, Chen, Qu, Yang, Zhao, Jiang and Feng2022).

Figure 9. (a) Theoretical vector synthesis of multi-bit phase coding construction. (b) Schematic of multi-bit phase coding via space–time programmable metamaterials. Reproduced from Zhang et al. (Reference Zhang, Wang, Shao, Shen, Chen, Wan, Cheng and Cui2019b). (c) Nonreciprocal reflection based on space–time programmable metamaterial. Reproduced from Zhang et al. (Reference Zhang, Chen, Shao, Dai, Cheng, Castaldi, Galdi and Cui2019a).
In the future, multi-bit phase coding using time-domain programmable metamaterials are expected favourable for realising novel phased-array antennas without traditional multi-bit phase shifters, saving the cost and reducing the design complexity.
Nonreciprocal reflection based on space–time programmable metamaterial
Breaking reciprocity has always been an interesting topic in the fields of electromagnetism, physics and information science, and have played an important role in communication, energy harvesting, and heat radiation. For example, in a wireless communication system, a nonreciprocal antenna transmitter can radiate a highly directional beam without receiving a reflected echo in the same direction. Conventional approaches are to break time inversion by using magnetic or nonlinear materials, which are bulky, expensive, and difficult to integrate. A new method is proposed to break the reciprocity by using space–time programmable metamaterial (Zhang et al., Reference Zhang, Chen, Shao, Dai, Cheng, Castaldi, Galdi and Cui2019a), where a 2-bit meta-atom is used to design an appropriate space–time gradient coding matrix to achieve nonreciprocal anomalous reflection accompanied by efficient frequency conversion. Figure 9c shows by switching between different space–time coding matrices, the angle and frequency of reflected waves can be dynamically controlled in real-time under temporal inversion, leading to the programmable non-reciprocal effect. Due to this excellent performance, space–time programmable metamaterials are expected to find wide applications in isolators, mixers, duplexers, one-way transmission, wireless communication and radar systems in future.
Asynchronous space–time programmable metamaterial
As previously mentioned, space–time programmable metamaterials function as a powerful tool for reaching more flexible EM manipulations beyond the space-domain programmable metamaterials. However, all the above studies on space–time programmable metamaterials are carried out in a synchronous framework, that is, all the meta-atoms on the aperture share the same modulation frequency.
Hence, an asynchronous space–time programmable metamaterial is proposed, where the meta-atoms are manipulated by different time coding periods (Wang et al., (Reference Wang, Chen, Ke, Cheng and Cui2022b)). It is shown that when the metamaterial is operated under asynchronous conditions (all meta-atoms no longer share the same manipulating frequency), the reflected waves will generate dynamic scattered wavefronts in space due to the scattering echoes of each element. Therefore, the phase gradient between the meta-atoms is no longer constant with time, resulting in the time-varying characteristics of the scattered EM waves. As shown in Figure 10a, the proposed asynchronous space–time programmable metamaterial extends the traditional concept of ‘phase gradients’ further to ‘frequency gradients’, thus the scattering pattern is not only a function of angle, but also a function of the distance and time. By designing the spatial distribution of the manipulating frequency of meta-atoms, researchers can realise various radiation patterns for beam scanning and dynamic radar cross-section (RCS) applications (Figure 10b,c), providing more freedom for EM manipulations.

Figure 10. (a) Schematic of asynchronous space–time programmable metamaterial. (b) Automatic spatial beam scanning. (c) Dynamic RCS manipulations. Reproduced from Wang et al., (Reference Wang, Chen, Ke, Cheng and Cui2022b).
New implementations of programmable metamaterials
Examples in Part 2 show that varactors or PIN diodes are the most commonly used components to provide real-time controls for programmable metamaterials. They bring rapid state switching, but at the cost of complex feeding network designs and high-power consumption. In this part, we will show some new implementations of programmable metamaterials with different advantages.
Light-driven programmable metamaterials
Feeding line network is one of the necessary designs in electrically-driven programmable metamaterials, which may deteriorate the radiating performances. To solve this issue, optically-driven programmable is proposed to achieve microwave reflection phase control using an optical interrogation network (OIN) (Zhang et al., Reference Zhang, Tang, Jiang, Bai, Tang, Bai, Qiu and Cui2018, Reference Zhang, Jiang, Jiang, Wang, Tiao, Bai, Luo, Sun, Luo, Qiu and Cui2020a), as illustrated in Figure 11a. The Meta-atom layer is composed of metallic structures with loaded varactors to provide phase variation. The required voltage change comes from the OIN, which is composed of a series photodiode array to receive optical signals and convert to voltages for the varactors. The overall thickness of the whole structure is only ~0.085λ at 6 GHz, much more compact than the electrically-driven programmable metamaterials. Hence, for illuminating light with different patterns, the programmable metamaterial can perform different functionalities such as external cloaking, illusion, and vortex beams (Figure 11b–d). Simulations and experiments have verified all the functionalities, indicating this light-driven approach can offer us a hybrid and integrating manner to achieve more flexible and complicated functions (Zhang et al., Reference Zhang, Jiang, Jiang, Wang, Tiao, Bai, Luo, Sun, Luo, Qiu and Cui2020a).

Figure 11. (a) Schematic of light-driven programmable metamaterial and EM functionalities. (b) Actual experiment setup and environment. (c) Performances of cloaking and illusion. (d) Measured results of the planar metamaterial and target ladder. Reproduced from Zhang et al. (Reference Zhang, Tang, Jiang, Bai, Tang, Bai, Qiu and Cui2018).
Programmable metamaterials based on liquid crystal
Liquid crystal (LC) can be introduced to the programmable metamaterials to realise terahertz-wave manipulations in real-time (Wu et al., Reference Wu, Shen, Ge, Chen, Shen, Wang, Zhang, Hu, Fan, Padilla, Lu, Jin, Chen and Wu2020e; Fu et al., Reference Fu, Shi, Yang, Fu, Liu, Wu, Yang, Bao and Cui2022). Specially, 1-bit phase modulation of a reflection-type meta-atom is achieved by controlling the bias voltage across LC. As LC needs to be bounded in the structure, the meta-atom contains seven layers, including silicon substrate, metallic back plate, polyimide (PI), LC, PI, complementary split ring resonator (CSRR), and quartz, as shown in Figure 12a. Silicon substrate and ultra-thin quartz are used as supporting layers, PI layers are pre-processed with parallel grooves for fix the pre-orientation of the LC, metallic back plate and CSRR also act as electrodes for applying the bias voltage. Hence, the 1-bit phase responses of the LC-driven meta-atom is controlled by changing voltages as shown in Figure 12b, and the phase coding pattern can be controlled in row for the programmable metamaterial to achieve anomalous reflection and wide-angle beam steering (Figure 12c).

Figure 12. (a) Illustration of the beam steering-based terahertz programmable metamaterials, and the topological structure of the LC programmable meta-atom. (b) 1-bit performance of the meta-atom. (c) Dual-beam scattering pattern of the metamaterial at different frequencies, where the curves 1–5 represent the different coding sequences. Reproduced from Fu et al. (Reference Fu, Shi, Yang, Fu, Liu, Wu, Yang, Bao and Cui2022).
Passive programmable metamaterials
In industrial community, power consumption is one of the critical measures of real systems. Hence, some low-power-consumption programmable metamaterials are proposed, which commonly use motors to rotate the meta-atom or metamaterial to reconstruct the EM control functionalities (Yang et al., Reference Yang, Chen, Zheng, Zhao, Hu, Qu, Jiang, Zhao and Feng2021; Xu et al., Reference Xu, Su, Zhang, Dong, Liu, Shi, Wang, Kang and Alu2022; Jeong et al., Reference Jeong, Park, Phon and Lim2022; Liu et al., Reference Liu, Ma, Shao, Zhang, Yan, Ma, Zhang and Cui2022), or introduce the Kirigami technology to mechanically reconfigure the EM performance (Phon et al., Reference Phon, Jeong and Lim2022; Zheng et al., Reference Zheng, Chen, Yang, Wu, Qu, Zhao, Jiang and Feng2022).
Researchers proposed a mechanical metamaterial platform to implement continuous and real-time Pancharatnam–Berry (PB) phase control of circularly polarised EM waves (Xu et al., Reference Xu, Su, Zhang, Dong, Liu, Shi, Wang, Kang and Alu2022). Different from the conventional varactor-driven programmable metamaterial, the introduced PB meta-atoms are rotated through a stepping motor and a series of gears (Figure 13a). With the change of the rotation angle, the reflected phase can be modulated for 0 to 2π with a large reflected amplitude at 7 GHz. To prove the flexible EM manipulating capacity, researchers first conduct a meta-lens to realise the real-time scanning of focal point, as shown in Figure 13b. Then, four vortex beams with different modes are also achieved by constructing corresponding phase patterns on the same aperture. Finally, reprogrammable hologram imaging is achieved to show two different images as shown in Figure 13c.

Figure 13. (a) Conceptional illustration of motor-driven PB phase programmable metamaterial. (b) 1-bit performance of the meta-atom. (b,c) Performances of real-time moving of focal point and reprogrammable hologram imaging. Reproduced from Xu et al. (Reference Xu, Su, Zhang, Dong, Liu, Shi, Wang, Kang and Alu2022).
System-level applications of programmable metamaterials
In addition to the powerful EM manipulating functionalities and devices that can be achieved with programmable metamaterials, they can play more important role in system-level applications. Here, we will present some applications of programmable information metamaterials in information, imaging and smart systems.
Information systems via programmable metamaterials
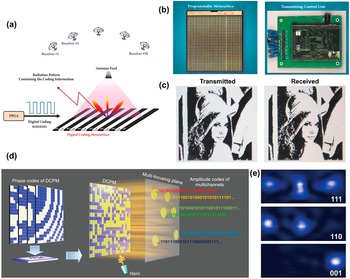
In Part. 1, we have mentioned that the coding pattern determines the radiated beam pattern, thus the information embedded in the coding pattern can be demodulated from the detected beams. Owing to this unique property, Cui et al. (Reference Cui, Liu, Bai and Ma2019) proposed a novel direct information transmitting system. Without traditional radio-frequency (RF) parts, programmable metamaterial entirely achieves the information processing on the wave transmission directly. As shown in Figure 14a, the system major comprises of a 1-bit reflection-type programmable metamaterial and a feed horn antenna. By changing the coding pattern with an FPGA, the programmable metamaterial can radiate different radiation patterns in far-field region, which can then be captured by some of the receiving antennas. This communication scheme ensures that all the transmitted information can only be correctly received by the receivers placed at the designed locations, which provide an encryption communication at the physical level. The results are shown in Figure 14c, in which the transmitted picture can be received in a low error rate.

Figure 14. (a) Principle of direct information transmitting system via programmable metamaterials. (b) The adopted programmable metamaterial and transmitting control unit. (c) The measured performance of picture transmission. Reproduced from Cui et al. (Reference Cui, Liu, Bai and Ma2019). (d) Schematic of multi-channel information transmitting system in near-field region. (e) Results of acquired signal of ‘111’, ‘110’ and ‘001’ in near field. Reproduced from Wan et al. (Reference Wan, Zhang, Chen, Zhang, Xu, Huang, Xiao, Xiao and Cui2019).
Wan et al. (Reference Wan, Zhang, Chen, Zhang, Xu, Huang, Xiao, Xiao and Cui2019) introduced a multi-channel information transmitting system in the near-field region. As shown in Figure 14d, the programmable metamaterial applies various phase coding patterns controlled by the FPGA, constructing tri-channel information output in the near-field through multi-focusing technology to mimic digital states of ‘111’, ‘110’, ‘001’ and any other cases (Figure 14e). The transmitted digital states can be distinguished by weak and strong power, namely the amplitude code ‘0’ and ‘1’. As there are almost no interaction among the three channels, a brief space division multiplexing information modulator can thus be achieved, implying highly potential application in near-field information communication and processing.
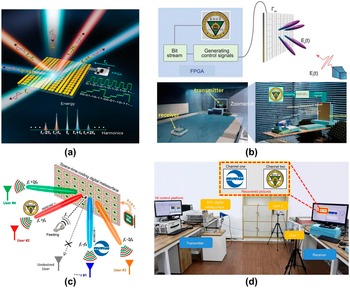
In particular, space–time programmable metamaterial can further manipulate the propagating direction and harmonic power distribution of EM wave, which make it more suitable for space and frequency division multiplexing (Zhao et al., Reference Zhao, Yang, Dai, Cheng, Li, Qi, Ke, Bai, Liu, Jin, Alu and Cui2018; Dai et al., Reference Dai, Tang, Yang, Li, Chen, Ke, Cheng, Jin and Cui2019; Zhang et al., Reference Zhang, Chen, Tang, Dai, Miao, Zhou, Jin, Cheng and Cui2021). A dynamic time-domain programmable metamaterial that enables efficient manipulations on spectral harmonic distributions is presented by Zhao et al. (Reference Zhao, Yang, Dai, Cheng, Li, Qi, Ke, Bai, Liu, Jin, Alu and Cui2018). By dynamically manipulating the local phase of reflectance, the precise control of different harmonics in a highly programmable manner can be achieved, which enable unusual responses such as velocity illusions. As a related application, a novel binary frequency-shift keying (BFSK) wireless communication system based on time-domain programmable metamaterial is also proposed in Figure 15a,b, which largely simplifies the modern communication system architecture and exhibiting excellent real-time signal transmission performance.

Figure 15. (a) Information processing of the time-domain programmable metamaterial. (b) Schematic of the proposed BFSK wireless communication system based on the time-domain programmable metamaterial. Reproduced from Zhao et al. (Reference Zhao, Yang, Dai, Cheng, Li, Qi, Ke, Bai, Liu, Jin, Alu and Cui2018). (c) A direct information-transmitting wireless communication system based on space–time programmable metamaterial with space division and frequency division multiplexing. (d) Prototype of dual-channel wireless communication system based on space–time programmable metamaterial, which can transmit different pictures to two users simultaneously and independently. Reproduced from Zhang et al. (Reference Zhang, Chen, Tang, Dai, Miao, Zhou, Jin, Cheng and Cui2021).
Further, a wireless communication scheme is proposed by Zhang et al. (Reference Zhang, Chen, Tang, Dai, Miao, Zhou, Jin, Cheng and Cui2021) that uses space–time programmable metamaterial for both space division multiplexing and frequency division multiplexing, as shown in Figure 15c. The programmable metamaterial is vertically excited by a single-tone plane wave with frequency fc , which is periodically switched according to the corresponding space–time coding matrix with a time modulation period T 0 = 1/f 0. Using a 2-bit space–time programmable metamaterial, a two-channel direct information transmission system is built to transmit two different photos to two users at different locations in space, as exhibited in Figure 15d. It also shows that the space–time programmable metamaterial can realise the information modulation and energy radiation simultaneously, and can regulate the spatial and frequency spectrum characteristics of EM waves. The system greatly extends the application scope of programmable metamaterial, suggesting potential applications in wireless communication, secure communication, radar systems, even on-chip designs in the future (Imani et al. (Reference Imani, Abadal and Del Hougne2022)).
Imaging systems via programmable metamaterials
Figure 16a shows a single-sensor and single-frequency passive imaging system based on transmission-type 2-bit programmable metamaterial. For this system, multiple measuring patterns are necessary to compose the generalised system response matrix G = (Gpj), p = 1, 2,…,P, j = 1, 2,…,N (P is indicates the total number of metamaterial patterns for the whole imaging, N is the number of sub-areas of the image scene). Along with the original object-area vector σ = (σ), i = 1, 2,…,N, the measurement data V = (V(p)i), p = 1, 2,…,P, is expressed as:
 $$\begin{align}\left[\begin{array}{c}{V}^{(1)}\\ {}{V}^{(2)}\\ {}\vdots \\ {}{V}^{(P)}\end{array}\right] = \left[\begin{array}{cccc}{G}_{11}& {G}_{12}& \cdots & {G}_{1N}\\ {}{G}_{21}& {G}_{22}& & \\ {}\vdots & & \ddots & \\ {}{G}_{P1}& & & {G}_{PN}\end{array}\right]\left[\begin{array}{c}{\sigma}_1\\ {}{\sigma}_2\\ {}\vdots \\ {}{\sigma}_N\end{array}\right]. \end{align}$$
$$\begin{align}\left[\begin{array}{c}{V}^{(1)}\\ {}{V}^{(2)}\\ {}\vdots \\ {}{V}^{(P)}\end{array}\right] = \left[\begin{array}{cccc}{G}_{11}& {G}_{12}& \cdots & {G}_{1N}\\ {}{G}_{21}& {G}_{22}& & \\ {}\vdots & & \ddots & \\ {}{G}_{P1}& & & {G}_{PN}\end{array}\right]\left[\begin{array}{c}{\sigma}_1\\ {}{\sigma}_2\\ {}\vdots \\ {}{\sigma}_N\end{array}\right]. \end{align}$$

Figure 16. (a) Single-radar-single-frequency passive imaging system via programmable metamaterial. Reproduced from Li et al. (Reference Li, Li, Xu, Wu, Wu, Wan, Cheng and Cui2016). (b) The reprogrammable hologram imaging. Reproduced from Li et al. (Reference Li, Cui, Ji, Liu, Ding, Wan, Li, Jiang, Qiu and Zhang2017). (c) Intelligent recognizer. Reproduced from Li et al. (Reference Li, Shuang, Ma, Li, Zhao, Wei, Liu, Hao, Qiu and Cui2019b). (d) machine-learning metamaterial imager. Reproduced from Li et al. (Reference Li, Ruan, Liu, Li, Shuang, Alu, Qiu and Cui2019a).
Hence, this equation can be solved by multiple programmable radiation and measurements illustrated in Figure 16a. First, a random coding pattern is generated by computer and stored in the FPGA to encode the metamaterial. Second, the plane EM wave emitted by the horn antenna is vertically incident on the metamaterial, and the corresponding transmission mode is generated by the metamaterial modulation. In the third step, the modulated radiation pattern impinges on the target, and the scattered signal is collected by the same antenna. By repeating this process, a series of random coding patterns are generated and the corresponding scattered signals are detected and recorded in order. Finally, the target can be reconstructed by inverse scattering imaging algorithm, accomplishing the imaging of the unknown target (Li et al., Reference Li, Li, Xu, Wu, Wu, Wan, Cheng and Cui2016).
Programmable metamaterials can also be used to compose active imaging system (Li et al., Reference Li, Cui, Ji, Liu, Ding, Wan, Li, Jiang, Qiu and Zhang2017). As shown in Figure 16b, through setting different hologram coding patterns via modified Gerchberg–Saxton (GS) algorithm, the programmable metamaterial can achieve different letters in real-time. With the help of dynamic EM control, researcher have accomplished more complicated functionalities and systems such as intelligent recognizer and machine-learning metamaterial imager (Li et al., Reference Li, Ruan, Liu, Li, Shuang, Alu, Qiu and Cui2019a, Reference Li, Shuang, Ma, Li, Zhao, Wei, Liu, Hao, Qiu and Cui2019b), as shown in Figure 16c,d.
Smart system via programmable metamaterials
Combined with artificial intelligence (AI) technology, programmable metamaterials can achieve self-adaptive and recognised systems (Ma et al., Reference Ma, Bai, Jing, Yang, Li and Cui2019, Reference Ma, Hong, Bai, Jing, Wu, Bao, Cheng and Cui2020; Liu et al., Reference Liu, Yu, Ma, Li and Cui2021, Reference Liu, Ma, Luo, Hong, Xiao, Zhang, Miao, Yu, Cheng, Li and Cui2022a). Further, researchers adopt 5-layer transmission-type programmable metamaterial to construct a programmable artificially intelligent machine (PAIM) (Liu et al., Reference Liu, Ma, Luo, Hong, Xiao, Zhang, Miao, Yu, Cheng, Li and Cui2022a), which can control EM wave propagation and interaction features (as shown in Figure 17). Each layer of PAIM has 8 × 8 meta-atoms, and each of them can be regarded as neuron node of artificial neural network (ANN). Hence, the whole PAIM is a physical implementation of ANN.

Figure 17. (a) Schematic of the intelligent system via programmable metamaterials. (b, c) Theoretical and physical model of PAIM. Reproduced from Liu et al. (Reference Liu, Ma, Luo, Hong, Xiao, Zhang, Miao, Yu, Cheng, Li and Cui2022a).
Specifically, when the incident EM wave is incident on a meta-atom, the amplitude and phase of the transmitted wave can be directly manipulated by FPGA. When the modulated EM wave passes through the meta-atom, it turns into a new source which radiates EM wave in all directions. Thus, the output of the fifth metamaterial is taken as the final output of the whole PAIM. The whole forward process can be regarded as the process of adjusting the EM energy distribution in the layer-by-layer manner in free space. In this way, PAIM can accomplish various functionalities such as automatic image recognition, reinforcement learning and communication coding and encoding, laying the foundation of totally intelligent programmable metamaterial designs. Furthermore, this scheme can be used for signal processing by elaborately designing the network of PAIM, and even for realising a completely automatic information-receiving and operating system.
Conclusions
In this article, we comprehensively review the developments of programmable metamaterials and metasurfaces, which inherit the flexible and real-time EM manipulations and digital information processing. The proposal of space-domain, time-domain and space–time-domain programmable metamaterials and metasurfaces further enable more flexible EM functionalities and facilitate the realisation of new-scheme and new-architecture information systems. We envision that the future programmable metamaterials and metasurfaces should advance towards more programmable, adaptive, and intelligent capabilities, along the following possible directions.
-
• More efficient programmable approaches. For the existing designs, electrically-driven diodes or varactors are still the most common components for modulation. Hence, more new modulation approaches and components such as phase-change materials and chips should be introduced to programmable metamaterials to provide more efficient and powerful controls.
-
• Interactions with the information science. There having been several preliminary studies proposed on the qualitative and quantitative analyses of the digital coding and programmable metamaterials regarding their information processing capabilities. More theories should be studied and developed for programmable metamaterial to further unleash its potential for information processing.
-
• Advanced programmable metamaterials. With the introduction of intelligent concept, programmable metamaterials are no longer simple EM devices but an advanced information processing system. Hence, more self-adaptive and self-feedback components should be integrated with programmable metamaterials to accomplish more advanced intelligent functionalities.
-
• Actual programmable metamaterial applications. Programmable metamaterials have shown great potential in many communication and information processing applications, which plays important roles in Reconfigurable Intelligent Surface (RIS). In the next stage, more engineering requirements and constraints need to be considered during the programmable metamaterial designs, aiming at real applications in wireless communications or beam-controlling radar systems.
In conclusion, the programmable metamaterials have shown strong EM manipulating powers, and will perform more important roles in the modern information technology, bringing out more functionalities beyond imagination. We remark that this review focuses more details on the programmable features of metamaterials than the previously published reviews on digital coding metamaterials and information metamaterials (Cui, Reference Cui2017, Reference Cui2018; Cui et al., Reference Cui, Liu and Zhang2017, Reference Cui, Li, Liu, Ma, Zhang, Wan, Jiang and Cheng2020; Zhang et al., Reference Zhang, Liu and Cui2017; Li and Cui, Reference Li and Cui2019; Bao and Cui, Reference Bao and Cui2020; Ma and Cui, Reference Ma and Cui2020; Wu and Cui, Reference Wu and Cui2020). Especially, this work emphasises the phase, amplitude and polarisation programmable capabilities of a variety of reflection-type, transmission-type, and full-space metamaterials and metasurfaces, and several new experimental realisations of the programmable features such as liquid crystal and passive manners.
Funding Statement
This project was supported by the Basic Scientific Center of Information Metamaterials of the National Natural Science Foundation of China (62288101), the National Key Research and Development Program of China (2017YFA0700201, 2017YFA0700202, 2017YFA0700203), the China National Postdoctoral Program for Innovative Talents (BX20200080), the China National Postdoctoral Science Foundation (2021M690603), the Natural Science Foundation of Jiangsu Province (BK20210210, BK20212002), the 111 Project (111-2-05) and the Fundamental Research Funds for the Central Universities (2242021R20022).
Competing Interests
The authors declare no competing interests exist.
Authorship Contributions
R.Y.W. wrote the article. L.W.W. contributed to the subsections of time-domain and space–time programmable metamaterials. S.H. contributed to the subsections of space-domain programmable metamaterials. S.L. and T.J.C. suggested the proposal, supervised the work and wrote the article.