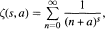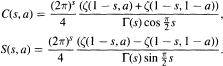Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Williams, Kenneth S.
and
Zhang, Nan Yue
1993.
Special values of the Lerch zeta function and the evaluation of certain integrals.
Proceedings of the American Mathematical Society,
Vol. 119,
Issue. 1,
p.
35.
Espinosa, Olivier
and
Moll, Victor H.
2002.
On Some Integrals Involving the Hurwitz Zeta Function: Part 1.
The Ramanujan Journal,
Vol. 6,
Issue. 2,
p.
159.
Yun-Fei, Wu
2004.
A New Integral Representation of the Riemann Zeta Function.
Rocky Mountain Journal of Mathematics,
Vol. 34,
Issue. 3,
Medina, Luis A.
and
Moll, Victor H.
2009.
A class of logarithmic integrals.
The Ramanujan Journal,
Vol. 20,
Issue. 1,
p.
91.
2012.
Zeta and q-Zeta Functions and Associated Series and Integrals.
p.
603.
Choi, Junesang
2014.
EVALUATION OF CERTAIN ALTERNATING SERIES.
Honam Mathematical Journal,
Vol. 36,
Issue. 2,
p.
263.
Choi, Junesang
2014.
Rapidly Converging Series forζ(2n+1)from Fourier Series.
Abstract and Applied Analysis,
Vol. 2014,
Issue. ,
p.
1.
Lord, Nick
2014.
Beyond the Basel problem: Euler’s derivation of the general formula for ζ (2n).
The Mathematical Gazette,
Vol. 98,
Issue. 543,
p.
459.