Article contents
VAUGHT’S CONJECTURE FOR ALMOST CHAINABLE THEORIES
Published online by Cambridge University Press: 13 August 2021
Abstract
A structure  ${\mathbb Y}$ of a relational language L is called almost chainable iff there are a finite set
${\mathbb Y}$ of a relational language L is called almost chainable iff there are a finite set 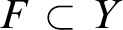 $F \subset Y$ and a linear order
$F \subset Y$ and a linear order  $\,<$ on the set
$\,<$ on the set 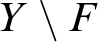 $Y\setminus F$ such that for each partial automorphism
$Y\setminus F$ such that for each partial automorphism 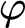 $\varphi $ (i.e., local automorphism, in Fraïssé’s terminology) of the linear order
$\varphi $ (i.e., local automorphism, in Fraïssé’s terminology) of the linear order  $\langle Y\setminus F, <\rangle $ the mapping
$\langle Y\setminus F, <\rangle $ the mapping  $\mathop {\mathrm {id}}\nolimits _F \cup \varphi $ is a partial automorphism of
$\mathop {\mathrm {id}}\nolimits _F \cup \varphi $ is a partial automorphism of 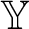 ${\mathbb Y}$. By theorems of Fraïssé and Pouzet, an infinite structure
${\mathbb Y}$. By theorems of Fraïssé and Pouzet, an infinite structure 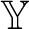 ${\mathbb Y}$ is almost chainable iff the profile of
${\mathbb Y}$ is almost chainable iff the profile of  ${\mathbb Y}$ is bounded; namely, iff there is a positive integer m such that
${\mathbb Y}$ is bounded; namely, iff there is a positive integer m such that  ${\mathbb Y}$ has
${\mathbb Y}$ has 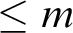 $\leq m$ non-isomorphic substructures of size n, for each positive integer n. A complete first order L-theory
$\leq m$ non-isomorphic substructures of size n, for each positive integer n. A complete first order L-theory 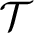 ${\mathcal T}$ having infinite models is called almost chainable iff all models of
${\mathcal T}$ having infinite models is called almost chainable iff all models of 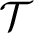 ${\mathcal T}$ are almost chainable and it is shown that the last condition is equivalent to the existence of one countable almost chainable model of
${\mathcal T}$ are almost chainable and it is shown that the last condition is equivalent to the existence of one countable almost chainable model of 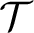 ${\mathcal T}$. In addition, it is proved that an almost chainable theory has either one or continuum many non-isomorphic countable models and, thus, the Vaught conjecture is confirmed for almost chainable theories.
${\mathcal T}$. In addition, it is proved that an almost chainable theory has either one or continuum many non-isomorphic countable models and, thus, the Vaught conjecture is confirmed for almost chainable theories.
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © Association for Symbolic Logic 2021
References
REFERENCES
- 1
- Cited by


