Hostname: page-component-76fb5796d-22dnz Total loading time: 0 Render date: 2024-04-26T16:24:50.548Z Has data issue: false hasContentIssue false
  • English
  • Français

Water waves for small surface tension: an approach via normal form

Published online by Cambridge University Press:  14 November 2011

Gérard Iooss  and
Klaus Kirchgässner
Gérard Iooss
Affiliation:
Institut Non Lineaire de Nice, Université de Nice, Pare Valrose, 06108 Nice Cedex 2, France
Klaus Kirchgässner
Affiliation:
Department of Mathematics, University of Stuttgart, Pfaffenwaldring 57, 7000 Stuttgart, Germany
Get access Rights & Permissions [Opens in a new window]

Synopsis

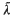In this paper we determine the possible crest-forms of permanent waves of small amplitude which exist on the free surface of a two-dimensional fluid layer under the influence of gravity and surface tension when the Froude number is close to 1. The Bond number b, measuring surface tension, is assumed to satisfy b < ⅓. We find one-parameter families of periodic waves of two different types, quasiperiodic waves and solitary waves with oscillations at infinity. The existence of true solitary waves is established for a sequence of systems approximating the full Euler equations in every algebraic order of 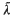− 1.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 122 , Issue 3-4 , 1992 , pp. 267 - 299
Copyright
Copyright © Royal Society of Edinburgh 1992

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Amick, C. J. and Kirchgässner, K.. Solitary water-waves in the presence of surface tension. In Dynamical Problems in Continuum Physics, I.M.A. Volumes in Mathematics and Its Applications 4 (Berlin: Springer, 1986).Google Scholar
2Amick, C. J. and Kirchgässner, K.. A theory of solitary water-waves in the presence of surface tension. Arch. Rational Mech. Anal. 105 (1989), 1–49.CrossRefGoogle Scholar
3Amick, C. J. and McLeod, J. B.. A singular perturbation problem in water waves (manuscript).Google Scholar
4Amick, C. J. and Toland, J. F.. Trajectories homoclinic to period orbits in four dimensions (manuscript).Google Scholar
5Beale, J. T.. Exact solitary water waves with capillary ripples at infinity. Comm. Pure Appl. Math. 44 (1991), 211–257.CrossRefGoogle Scholar
6Coullet, P. and Spiegel, M. E.. Amplitude equations for systems with competing instabilities. SIAM J. Appl. Math. 43 (1983), 774–819.CrossRefGoogle Scholar
7Dias, F., Iooss, G. and Vanden-Broeck, J. M.. Capillary-gravity solitary waves with damped oscillations (manuscript).Google Scholar
8Eckhaus, W.. Singular perturbations of homoclinic orbits in ℝ4 (University of Utrecht, Preprint, Nr. 642, 1991).Google Scholar
9Elphick, C., Tirapegui, E., Brachet, M. E., Coullet, P. and Iooss, G.. A simple global characterization for normal forms of singular vector fields. Physica D 29 (1987), 95–127.CrossRefGoogle Scholar
10Hamilton, R. S.. The inverse function theorem of Nash-Moser. Bull. Amer. Math. Soc. 7 (1982), 65–222.CrossRefGoogle Scholar
11Hammersley, J. M. and Mazzarino, G.. Computational aspects of some autonomous differential equations. Proc. Roy. Soc. London Ser. A 424 (1989), 19–37.Google Scholar
12Hunter, J. K. and Scheurle, J.. Existence of perturbed solitary wave solutions to a model equation for water waves. Physica D 32 (1988), 253–268.CrossRefGoogle Scholar
13Iooss, G. and Adelmeyer, M.. Topics in bifurcation theory and applications (manuscript).Google Scholar
14Iooss, G. and Kirchgässner, K.. Bifurcations d'ondes solitaires en présense d'une faible tension superficielle. Note C.R. Acad. Sci. Paris 311 1 (1990), 265–268.Google Scholar
15Iooss, G. and Los, J.. Bifurcation of spatially quasi-periodic solutions in hydrodynamic stability problems. Nonlinearity 3 (1990), 851–871.CrossRefGoogle Scholar
16Iooss, G. and Peroueme, M. C.. Perturbed homoclinic solution in reversible 1:1 resonance vector fields. J. Differential Equations (to appear).Google Scholar
17Kato, T.. Perturbation theory for linear operators, Grundlehren der mathematischen Wissenschaften (New York: Springer, 1966).Google Scholar
18Kirchgässner, K.. Wave solutions of reversible systems and applications. J. Differential Equations 45 (1982), 113–127.CrossRefGoogle Scholar
19Kirchgässner, K.. Nonlinearly resonant surface waves and homoclinic bifurcation. Adv. in Appl. Mech. 26 (1988), 135–181.CrossRefGoogle Scholar
20Mielke, A.. Reduction of quasilinear elliptic equations in cylindrical domains with applications. Math. Methods Appl. Sci. 10 (1988), 51–66.CrossRefGoogle Scholar
21Sun, S. M.. Existence of a generalized solitary wave solution for water with positive Bond number less than 1/3. J. Math. Anal. Appl. 156 (1991), 471–504.CrossRefGoogle Scholar
22Whitham, G. B.. Linear and nonlinear waves (New York: J. Wiley, 1974).Google Scholar
23Zeidler, E.. Existenzbeweis für cnoidal waves unter Berücksichtigung der Oberflächenspannung. Arch. Rational Mech. Anal. 41 (1971), 81–107.CrossRefGoogle Scholar