Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Rudnick, Zeév
2018.
A METRIC THEORY OF MINIMAL GAPS.
Mathematika,
Vol. 64,
Issue. 3,
p.
628.
Bloom, Thomas F.
Chow, Sam
Gafni, Ayla
and
Walker, Aled
2018.
ADDITIVE ENERGY AND THE METRIC POISSONIAN PROPERTY.
Mathematika,
Vol. 64,
Issue. 3,
p.
679.
Hinrichs, Aicke
Kaltenböck, Lisa
Larcher, Gerhard
Stockinger, Wolfgang
and
Ullrich, Mario
2019.
On a multi-dimensional Poissonian pair correlation concept and uniform distribution.
Monatshefte für Mathematik,
Vol. 190,
Issue. 2,
p.
333.
Lachmann, Thomas
and
Technau, Niclas
2019.
On exceptional sets in the metric Poissonian pair correlations problem.
Monatshefte für Mathematik,
Vol. 189,
Issue. 1,
p.
137.
Aistleitner, Christoph
Lachmann, Thomas
and
Technau, Niclas
2019.
THERE IS NO KHINTCHINE THRESHOLD FOR METRIC PAIR CORRELATIONS.
Mathematika,
Vol. 65,
Issue. 4,
p.
929.
Marklof, Jens
2020.
Pair correlation and equidistribution on manifolds.
Monatshefte für Mathematik,
Vol. 191,
Issue. 2,
p.
279.
Bloom, Thomas F.
and
Walker, Aled
2020.
GCD sums and sum-product estimates.
Israel Journal of Mathematics,
Vol. 235,
Issue. 1,
p.
1.
Rudnick, Zeév
and
Technau, Niclas
2020.
The metric theory of the pair correlation function of real-valued lacunary sequences.
Illinois Journal of Mathematics,
Vol. 64,
Issue. 4,
Steinerberger, Stefan
2020.
Poissonian pair correlation in higher dimensions.
Journal of Number Theory,
Vol. 208,
Issue. ,
p.
47.
Cohen, Alex
2021.
Poissonian correlation of higher order differences.
Journal of Number Theory,
Vol. 229,
Issue. ,
p.
463.
RAMÍREZ, FELIPE A.
2022.
Remarks about inhomogeneous pair correlations.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 173,
Issue. 2,
p.
369.
 $\unicode[STIX]{x1D6FC}$, the sequence of fractional parts
$\unicode[STIX]{x1D6FC}$, the sequence of fractional parts 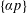 $\{\unicode[STIX]{x1D6FC}p\}$ is equidistributed in
$\{\unicode[STIX]{x1D6FC}p\}$ is equidistributed in 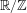 $\mathbb{R}/\mathbb{Z}$ as
$\mathbb{R}/\mathbb{Z}$ as  $p$ ranges over primes. There is a natural second-order equidistribution property, a pair correlation of such fractional parts, which has recently received renewed interest, in particular regarding its relation to additive combinatorics. In this paper we show that the primes do not enjoy this stronger equidistribution property.
$p$ ranges over primes. There is a natural second-order equidistribution property, a pair correlation of such fractional parts, which has recently received renewed interest, in particular regarding its relation to additive combinatorics. In this paper we show that the primes do not enjoy this stronger equidistribution property.



