Global blow-up for a semilinear heat equation on a subspace
Published online by Cambridge University Press: 24 August 2015
Abstract
We study the asymptotic behaviour as t → T–, near a finite blow-up time T > 0, of decreasing-in-x solutions to the following semilinear heat equation with a non-local term:
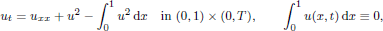
with Neumann boundary conditions and strictly decreasing initial function u0(x) with zero mass. We prove sharp estimates for u(x, t) as t → T–, revealing a non-uniform global blow-up:

uniformly on any compact set [δ, 1], δ ∈ (0, 1).
MSC classification
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 145 , Issue 5 , October 2015 , pp. 893 - 923
- Copyright
- Copyright © Royal Society of Edinburgh 2015
- 3
- Cited by


