Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T04:28:17.210Z Has data issue: false hasContentIssue false
  • English
  • Français

Higher ℓ2-Betti Numbers of Universal Quantum Groups

Published online by Cambridge University Press:  20 November 2018

Julien Bichon ,
David Kyed  and
Sven Raum
Julien Bichon
Affiliation:
Laboratoire de Mathématiques Blaise Pascal, Université Clermont Auvergne, Campus universitaire des Cézeaux, 3 place Vasarely, 63178 Aubière cedex, Francejulien.bichon@uca.fr
David Kyed
Affiliation:
Department of Mathematics and Computer Science, University of Southern Denmark, Campusvej 22, DK-5230 Odense M, Denmarkdkyed@imada.sdu.dk
Sven Raum
Affiliation:
EPFL SB SMA, Station 8, CH-1015 Lausanne, Switzerlandsven.raum@epfl.ch
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We calculate all 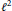${{\ell }^{2}}$-Betti numbers of the universal discrete Kac quantum groups 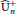$\widehat{\text{U}}_{n}^{+}$ as well as their half-liberated counterparts 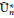$\widehat{\text{U}}_{n}^{*}$.

Keywords

16T0546L6520G42ℓ2-Betti numberfree unitary quantum grouphalf-liberated unitary quantum groupfree product formulaextension
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 61 , Issue 2 , 01 June 2018 , pp. 225 - 235
Copyright
Copyright © Canadian Mathematical Society 2018

References

[Ban97a] Banica, T., Legroupe quantique compact libre U(n). Comm. Math. Phys. 190(1997), no. 1, 143–172.http://dx.doi.Org/10.1007/s002200050237 Google Scholar
[BS09] Banica, T. and Speicher, R., Liberation orthogonal Lie groups. Adv. Math. 222(2009), no. 4, 1461–1501.http://dx.doi.Org/10.1016/j.aim.2009.06.009 Google Scholar
[BV10] Banica, T. and Vergnioux, R., Invariants ofthe half-liberated orthogonal group. Ann. Inst. Fourier 60(2010), no. 6, 2137–2164.http://dx.doi.org/10.5802/aif.2579 Google Scholar
[BMT01] Bédos, E., Murphy, G. J., and Tuset, L., Co-amenability of compact quantum groups. J. Geom. Phys. 40(2001), no. 2, 130–153. http://dx.doi.org/10.1016/S0393-0440(01)00024-9 Google Scholar
[vdB98] van den Bergh, M., A relation between Hochschild homology and cohomology for Gorenstei rings. Proc. Amer. Math. Soc. 126(1998), no. 5,1345–1348.http://dx.doi.org/10.1090/S0002-9939-98-04210-5 Google Scholar
[BDD11] Bhowmick, J., D'Andrea, F., and Dabrowski, L., Quantum isometries ofthe finite noncommutative geometry of the Standard model. Comm. Math. Phys. 307(2011), 101–131.http://dx.doi.Org/10.1007/s00220-011-1301-2 Google Scholar
[Bicl6] Bichon, J., Cohomological dimensions of universal cosoverign Hopf algebras. Publicacions Matemàtiques, to appear. arxiv:1611.02069Google Scholar
[BNY15] Bichon, J., Neshveyev, S., and Yamashita, M., Graded twisting of categories and quantum groups by group actions. Ann. Inst. Fourier 66(2016), no. 6, 2299–2338.http://dx.doi.org/10.5802/aif.3064 Google Scholar
[BNY16] Bichon, J., Neshveyev, S., and Yamashita, M., Graded twisting of comodule algebras and module categories. arxiv:1 604.02078Google Scholar
[Bral2] Brannan, M., Approximation properties for free orthogonal andfree unitary quantum groups. J. Reine Angew. Math. 672(2012), 223–251.Google Scholar
[Chil4] Chirvasitu, A., Cosemisimple Hopf algebras are faithfully flat over Hopf subalgebras. Algebra Number Theory 8(2014), no. 5,1179–1199.http://dx.doi.Org/10.214O/ant.2O14.8.1179 Google Scholar
[CHT09] Collins, B., Härtel, Johannes, and Thom, A., Homology offree quantum groups. C. R. Math. Acad. Sei. Paris 347(2009), no. 5-6, 271–276.http://dx.doi.Org/10.1016/j.crma.2009.01.021 Google Scholar
[FimlO] Fima, P. Kazhdan's property T for discrete quantum groups. Internat. J. Math. 221(2010), no. 1, 47–65. http://dx.doi.Org/10.1142/S0129167X1000591X Google Scholar
[KVOO] Kustermans, J. and Vaes, S., Locally compact quantum groups. Ann. Sei. École Norm. Sup. (4) 33(2000), no. 6, 837–934. http://dx.doi.Org/10.1016/S0012-9593(00)01055-7Google Scholar
[KyeO8a] Kyed, D., L2-Betti numbers of coamenable quantum groups. Münster J. Math. 1(2008), 143–179.Google Scholar
[KyeO8b] Kyed, D., L2-homology for compact quantum groups. Math. Scand. 103(2008), no. 1,111–129.http://dx.doi.org/10.7146/math.scand.a-15072 Google Scholar
[Kyell] Kyed, D., On the zeroth L2-homology of a quantum group. Münster J. Math. 4(2011), 119–127.Google Scholar
[Kyel2] Kyed, D., An L2-Kunneth formula for tracial algebras. J. Operator Theory 67(2012), 317–327.Google Scholar
[KR16] Kyed, D. and Raum, S., On the l2-Betti numbers of universal quantum groups. Math. Ann., to appear. arxiv:1 610.05474Google Scholar
[LücO2] Lück, W., L2-invariants: theory and applications to geometry and K-theory. Ergebnisse der Mathematik und ihrer Grenzgebiete, 44, Folge, A Series of Modern Surveys in Mathematics, Springer-Verlag, Berlin, 2002.Google Scholar
[MN06] Meyer, R. and Nest, R., The Baum-Connes conjeeture via localisation of categories. Topology 45(2006), no. 2, 209–259. http://dx.doi.Org/10.1016/j.top.2005.07.001Google Scholar
[ReiOl] Reich, H., On the K-and L-theory ofthe algebra of Operators affiliated to a finite von Neumann algebra. Jf-Theory 24, no. 4, 303–326.http://dx.doi.Org/10.1023/A:1014078228859 Google Scholar
[SauO2] Sauer, R., L2-invariants of groups and discrete measured groupoids. Ph.D. Dissertation, University of Münster, 2002.Google Scholar
[Tho08] Thom, A., L2 *-cohomology for von Neumann algebras. Geom. Funct. Anal. 18(2008), no. 1, 251–270. http://dx.doi.org/10.1007/s00039-007-0634-7 Google Scholar
[VW96] Van Daele, A. and Wang, S., Universal quantum groups. Internat. J. Math. 7(1996), no. 2, 255–263. http://dx.doi.Org/10.1142/S0129167X96000153 Google Scholar
[VerO7] Vergnioux, R., The property of rapid decayfor discrete quantum groups. J. Operator Theory 57(2007), 303–324.Google Scholar
[Verl2] Vergnioux, R., Paths in quantum Cayley trees and L2-cohomology. Adv. Math. 229(2012), 2686–2711.http://dx.doi.Org/10.1016/j.aim.2O12.01.011 Google Scholar
[Voill] Voigt, C., The Baum-Connes conjeeture for free orthogonal quantum groups. Adv. Math. 227(2011), no. 5, 1873–1913.http://dx.doi.Org/10.1016/j.aim.2O11.04.008 Google Scholar
[Wan95] Wang, S., Free produets ofcompact quantum groups. Comm. Math. Phys. 167(1995), no. 3, 671–692.http://dx.doi.Org/10.1007/BF02101540 Google Scholar
[Wan98] Wang, S., Quantum symmetry groups of finite Spaces. Comm. Math. Phys. 195(1998), 195–211. http://dx.doi.org/10.1007/s002200050385 Google Scholar
[Wei94] Weibel, C. A., An introduction to homological algebra. Cambridge Studies in Advanced Mathematics, 38, Cambridge University Press, Cambridge, 1994.http://dx.doi.Org/10.1017/CBO9781139644136 Google Scholar
[Wor87] Woronowicz, S. L., Twisted SU(2) group. An example ofa noncommutative differential calculus. Publ. Res. Inst. Math. Sei. 23(1987), 117–181. http://dx.doi.org/10.2977/prims/1195176848 Google Scholar
[Wor98] Woronowicz, S. L., Compact quantum groups. In: Symetries quantiques (Les Houches, 1995), North-Holland, Amsterdam, 1998, pp. 845–884.Google Scholar