Hostname: page-component-76fb5796d-9pm4c Total loading time: 0 Render date: 2024-04-28T02:00:07.424Z Has data issue: false hasContentIssue false
  • English
  • Français

A non-singular version of the Oseledeč ergodic theorem

Part of: Ergodic theory

Published online by Cambridge University Press:  24 February 2022

ANTHONY H. DOOLEY Open the ORCID record for ANTHONY H. DOOLEY [Opens in a new window]  and
JIE JIN
ANTHONY H. DOOLEY*
Affiliation:
School of Mathematical and Physical Sciences, University of Technology Sydney, Ultimo 2007, Australia (e-mail: Jie.Jin-3@student.uts.edu.au)
JIE JIN
Affiliation:
School of Mathematical and Physical Sciences, University of Technology Sydney, Ultimo 2007, Australia (e-mail: Jie.Jin-3@student.uts.edu.au)
*
e-mail: anthony.dooley@uts.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

Kingman’s subadditive ergodic theorem is traditionally proved in the setting of a measure-preserving invertible transformation T of a measure space 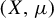$(X, \mu )$ . We use a theorem of Silva and Thieullen to extend the theorem to the setting of a not necessarily invertible transformation, which is non-singular under the assumption that 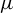$\mu $ and $\mu \circ T$ have the same null sets. Using this, we are able to produce versions of the Furstenberg–Kesten theorem and the Oseledeč ergodic theorem for products of random matrices without the assumption that the transformation is either invertible or measure-preserving.

Keywords

non-singular ergodic theoryrandom ergodic theoremcritical dimension

MSC classification

Primary: 37A40: Nonsingular (and infinite-measure preserving) transformations 37A30: Ergodic theorems, spectral theory, Markov operators
Secondary: 37A50: Relations with probability theory and stochastic processes
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 43 , Issue 3 , March 2023 , pp. 873 - 886
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aaronson, J.. An Introduction to Infinite Ergodic Theory. American Mathematical Society, Providence, RI, 1997.CrossRefGoogle Scholar
Arnold, L.. Random Dynamical Systems. Springer, Berlin, 2003.Google Scholar
Avila, A. and Bochi, J.. On the subadditive ergodic theorem. Preprint, 2009, http://www.mat.uc.cl/~jairo.bochi/docs/kingbirk.pdf.Google Scholar
Birkhoff, G. D.. Proof of the ergodic theorem. Proc. Natl. Acad. Sci. USA 17 (1931), 656–660.CrossRefGoogle ScholarPubMed
Dooley, A. H. and Mortiss, G.. On the critical dimension of product odometers. Ergod. Th. & Dynam. Sys. 29 (2008), 475–485.CrossRefGoogle Scholar
Furstenberg, H. and Kesten, H.. Products of random matrices. Ann. Math. Stat. 31 (1960), 457–469.CrossRefGoogle Scholar
Hurewicz, W.. Ergodic theorem without invariant measure. Ann. of Math. (2) 45 (1944), 192–206.CrossRefGoogle Scholar
Jones, R. L.. New proofs for the maximal ergodic theorem and the Hardy–Littlewood maximal theorem. Proc. Amer. Math. Soc. 87 (1983), 681–684.CrossRefGoogle Scholar
Katznelson, Y. and Weiss, B.. A simple proof of some ergodic theorems. Israel J. Math. 42 (1982), 291–296.CrossRefGoogle Scholar
Kingman, J. F. C.. The ergodic theory of subadditive stochastic processes. J. R. Stat. Soc. Ser. B. 30 (1968), 499–510.Google Scholar
Oseledets, I.. A multiplicative ergodic theorem: Lyapunov characteristic numbers for dynamical systems. Trans. Moscow Math. Soc. 19 (1968), 197–231.Google Scholar
Raghunathan, M. S.. A proof of Oseledec’s multiplicative ergodic theorem. Israel J. Math. 32 (1979), 356–362.CrossRefGoogle Scholar
Schmidt, K.. Algebraic Ideas in Ergodic Theory (CBMS Regional Conference Series in Mathematics, 76). American Mathematical Society, Providence, RI, 1990.CrossRefGoogle Scholar
Silva, C. E. and Thieullen, P.. The subadditive ergodic theorem and recurrence properties of Markovian transformations. J. Math. Anal. Appl. 154 (1991), 83–99.CrossRefGoogle Scholar
Steel, J. M.. Kingman’s subadditive ergodic theorem. Ann. Inst. Henri Poincaré Probab. Stat. 25 (1989), 93–98.Google Scholar