Article contents
Inviscid and viscous global stability of vortex rings
Published online by Cambridge University Press: 04 September 2020
Abstract
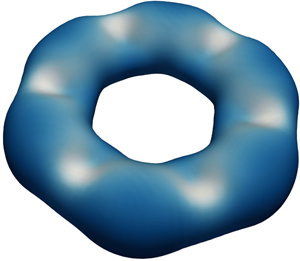
We perform inviscid and viscous, global, linear stability analyses of vortex rings which are compared with asymptotic theories and numerical simulations. We find growth rates of rings to be very sensitive to the details of vorticity distribution, in a way not accounted for in asymptotic theories, clearly demonstrated in our analyses of equilibrated rings–ring base flows initially obtained from Gaussian rings evolved to a quasi-steady state before any instabilities set in. Such equilibrated rings with the same  $\epsilon = a/R$, the ratio of core radius
$\epsilon = a/R$, the ratio of core radius  $a$ to ring radius
$a$ to ring radius  $R$, but evolved with different viscosities, have inviscid growth rates differing by up to 9 %, though the differences in vorticity at any point are small. In contrast, the growth rates of rings with a Gaussian vorticity distribution are found to be up to 33 % smaller than the inviscid asymptotic theories over
$R$, but evolved with different viscosities, have inviscid growth rates differing by up to 9 %, though the differences in vorticity at any point are small. In contrast, the growth rates of rings with a Gaussian vorticity distribution are found to be up to 33 % smaller than the inviscid asymptotic theories over  $0.4 > \epsilon > 0.05$. We attribute these differences to the nature of velocity fields at
$0.4 > \epsilon > 0.05$. We attribute these differences to the nature of velocity fields at  $O(\epsilon ^2)$, between equilibrated and Gaussian rings, where the former shows a good quantitative match with the asymptotic theories. Additionally, there are some differences with previous direct numerical simulations (DNS), but in very close quantitative agreement with our DNS results. Our calculations provide a new relation capturing the near-linear dependence of growth rates on the reciprocal of a strain rate-based Reynolds number
$O(\epsilon ^2)$, between equilibrated and Gaussian rings, where the former shows a good quantitative match with the asymptotic theories. Additionally, there are some differences with previous direct numerical simulations (DNS), but in very close quantitative agreement with our DNS results. Our calculations provide a new relation capturing the near-linear dependence of growth rates on the reciprocal of a strain rate-based Reynolds number  $\widehat Re$. Importantly, our equilibrated ring calculations do tend to the inviscid limit of asymptotic theories, once corrections for ring radius evolution and equilibrated distribution are imposed, unlike for Gaussian rings.
$\widehat Re$. Importantly, our equilibrated ring calculations do tend to the inviscid limit of asymptotic theories, once corrections for ring radius evolution and equilibrated distribution are imposed, unlike for Gaussian rings.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 2
- Cited by





