Hostname: page-component-76fb5796d-qxdb6 Total loading time: 0 Render date: 2024-04-26T03:48:49.767Z Has data issue: false hasContentIssue false
  • English
  • Français

Evolution of shock-accelerated heavy gas layer

Published online by Cambridge University Press:  08 January 2020

Yu Liang Open the ORCID record for Yu Liang [Opens in a new window] ,
Lili Liu ,
Zhigang Zhai Open the ORCID record for Zhigang Zhai [Opens in a new window] ,
Ting Si Open the ORCID record for Ting Si [Opens in a new window]  and
Chih-Yung Wen Open the ORCID record for Chih-Yung Wen [Opens in a new window]
Yu Liang
Affiliation:
Advanced Propulsion Laboratory, Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China Department of Mechanical Engineering and Interdisplinary Division of Aeronautical and Aviation Engineering, The Hong Kong Polytechnic University, Kowloon, Hong Kong
Lili Liu
Affiliation:
Advanced Propulsion Laboratory, Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China Department of Mechanical Engineering and Interdisplinary Division of Aeronautical and Aviation Engineering, The Hong Kong Polytechnic University, Kowloon, Hong Kong
Zhigang Zhai*
Affiliation:
Advanced Propulsion Laboratory, Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
Ting Si
Affiliation:
Advanced Propulsion Laboratory, Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
Chih-Yung Wen
Affiliation:
Department of Mechanical Engineering and Interdisplinary Division of Aeronautical and Aviation Engineering, The Hong Kong Polytechnic University, Kowloon, Hong Kong
*
†Email address for correspondence: sanjing@ustc.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

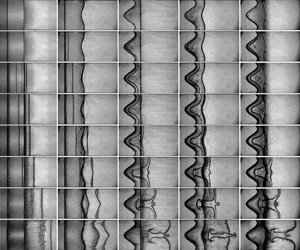
Richtmyer–Meshkov instability of the SF6 gas layer surrounded by air is experimentally investigated. Using the soap film technique, five kinds of gas layer with two sharp interfaces are generated such that the development of each individual interface is highlighted. The flow patterns are determined by the amplitudes and phases of two corrugated interfaces. For a layer with both interfaces planar, the interface velocity shows that the reflected rarefaction waves from the second interface accelerate the first interface motion. For a layer with the second interface corrugated but the first interface planar, the reflected rarefaction waves make the first interface develop with the same phase as the second interface. For a layer with the first interface corrugated but the second interface planar, the rippled shock seeded from the first interface makes the second interface develop with the same phase as the first interface and the layer evolves into an ‘upstream mushroom’ shape. For two interfaces corrugated with opposite (the same) phase but a larger amplitude for the first interface, the layer evolves into ‘sinuous’ shape (‘bow and arrow’ shape, which has never been observed previously). For the interface amplitude growth in the linear stage, the waves’ effects are considered in the model to give a better prediction. In the nonlinear stage, the effect of the rarefaction waves on the first interface evolution is quantitatively evaluated, and the nonlinear growth is well predicted. It is the first time in experiments to quantify the interfacial instability induced by the rarefaction waves inside the heavy gas layer.

JFM classification

Compressible Flows: Shock waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 886 , 10 March 2020 , A7
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arnett, W. D., Bahcall, J. N., Kirshner, R. P. & Woosley, S. E. 1989 Supernova 1987A. Annu. Rev. Astron. Astrophys. 27 (1), 629–700.CrossRefGoogle Scholar
Bai, J. S., Zou, L. Y., Wang, T., Liu, K., Huang, W. B., Liu, J. H., Li, P., Tan, D. W. & Liu, C. L. 2010 Experimental and numerical study of shock-accelerated elliptic heavy gas cylinders. Phys. Rev. E 82 (5), 056318.Google ScholarPubMed
Bai, X., Deng, X. L. & Jiang, L. 2018 A comparative study of the single-mode Richtmyer–Meshkov instability. Shock Waves 28 (4), 795–813.CrossRefGoogle Scholar
Balakumar, B. J., Orlicz, G. C., Ristorcelli, J. R., Balasubramanian, S., Prestridge, K. P. & Tomkins, C. D. 2012 Turbulent mixing in a Richtmyer–Meshkov fluid layer after reshock: velocity and density statistics. J. Fluid Mech. 696, 67–93.CrossRefGoogle Scholar
Bates, J. W. 2004 Initial value problem solution for isolated rippled shock fronts in arbitrary fluid media. Phys. Rev. E 69 (5), 056313.Google ScholarPubMed
Bell, G. I.1951 Taylor instability on cylinders and spheres in the small amplitude approximation. Report No. LA-1321, LANL 1321.Google Scholar
Brouillette, M. 2002 The Richtmyer–Meshkov instability. Annu. Rev. Fluid Mech. 34 (1), 445–468.CrossRefGoogle Scholar
Budzinski, J. M., Benjamin, R. F. & Jacobs, J. W. 1994 Influence of initial conditions on the flow patters of a shock-accelerated thin fluid layer. Phys. Fluids 6 (11), 3510–3512.CrossRefGoogle Scholar
Buttler, W. T., Oró, D. M., Preston, D. L., Mikaelian, K. O., Cherne, F. J., Hixson, R. S., Mariam, F. G., Morris, C., Stone, J. B., Terrones, G. et al. 2012 Unstable Richtmyer–Meshkov growth of solid and liquid metals in vacuum. J. Fluid Mech. 703, 60–84.CrossRefGoogle Scholar
Collins, B. D. & Jacobs, J. W. 2002 PLIF flow visualization and measurements of the Richtmyer–Meshkov instability of an air/SF6 interface. J. Fluid Mech. 464, 113–136.CrossRefGoogle Scholar
Dell, Z., Stellingwerf, R. F. & Abarzhi, S. I. 2015 Effect of initial perturbation amplitude on Richtmyer–Meshkov flows induced by strong shocks. Phys. Plasmas 22 (9), 092711.CrossRefGoogle Scholar
Ding, J., Li, J., Sun, R., Zhai, Z. & Luo, X. 2019 Convergent Richtmyer–Meshkov instability of a heavy gas layer with perturbed outer interface. J. Fluid Mech. 878, 277–291.CrossRefGoogle Scholar
Ding, J., Si, T., Chen, M., Zhai, Z., Lu, X. & Luo, X. 2017 On the interaction of a planar shock with a three-dimensional light gas cylinder. J. Fluid Mech. 828, 289–317.CrossRefGoogle Scholar
de Frahan, M. T. H., Movahed, P. & Johnsen, E. 2015 Numerical simulations of a shock interacting with successive interfaces using the discontinuous Galerkin method: the multilayered Richtmyer–Meshkov and Rayleigh–Taylor instabilities. Shock Waves 25 (4), 329–345.CrossRefGoogle Scholar
Hahn, M., Drikakis, D., Youngs, D. L. & Williams, R. J. R. 2011 Richtmyer–Meshkov turbulent mixing arising from an inclined material interface with realistic surface perturbations and reshocked flow. Phys. Fluids 23 (4), 046101.CrossRefGoogle Scholar
Holmes, R. L., Dimonte, G., Fryxell, B., Gittings, M. L., Grove, J. W., Schneider, M., Sharp, D. H., Velikovich, A. L., Weaver, R. P. & Zhang, Q. 1999 Richtmyer–Meshkov instability growth: experiment, simulation and theory. J. Fluid Mech. 389, 55–79.CrossRefGoogle Scholar
Ishizaki, R., Nishihara, K., Sakagami, H. & Ueshima, Y. 1996 Instability of a contact surface driven by a nonuniform shock wave. Phys. Rev. E 53 (6), R5592.Google ScholarPubMed
Ishizaki, R., Nishihara, K., Wouchuk, J. G., Shigemori, K., Nakai, M., Miyanaga, N., Azechi, H. & Mima, K. 1999 Rippled shock propagation and hydrodynamic perturbation growth in laser implosion. J Mater. Process Tech. 85 (1), 34–38.CrossRefGoogle Scholar
Jacobs, J. W., Jenkins, D. G., Klein, D. L. & Benjamin, R. F. 1995 Nonlinear growth of the shock-accelerated instability of a thin fluid layer. J. Fluid Mech. 295, 23–42.CrossRefGoogle Scholar
Jacobs, J. W., Klein, D. L., Jenkins, D. G. & Benjamin, R. F. 1993 Instability growth patterns of a shock-accelerated thin fluid layer. Phys. Rev. Lett. 70 (5), 583–586.CrossRefGoogle ScholarPubMed
Jourdan, G. & Houas, L. 2005 High-amplitude single-mode perturbation evolution at the Richtmyer–Meshkov instability. Phys. Rev. Lett. 95 (20), 204502.CrossRefGoogle ScholarPubMed
Liang, Y., Ding, J., Zhai, Z., Si, T. & Luo, X. 2017 Interaction of cylindrically converging diffracted shock with uniform interface. Phys. Fluids 29 (8), 086101.CrossRefGoogle Scholar
Liang, Y., Zhai, Z., Ding, J. & Luo, X. 2019 Richtmyer–Meshkov instability on a quasi-single-mode interface. J. Fluid Mech. 872, 729–751.CrossRefGoogle Scholar
Liao, S., Zhang, W., Chen, H., Zou, L., Liu, J. & Zheng, X. 2019 Atwood number effects on the instability of a uniform interface driven by a perturbed shock wave. Phys. Rev. E 99 (1), 013103.Google ScholarPubMed
Lindl, J. D., Amendt, P., Berger, R. L., Glendinning, S. G., Glenzer, S. H., Haan, S. W., Kauffman, R. L., Landen, O. L. & Suter, L. J. 2004 The physics basis for ignition using indirect-drive targets on the National Ignition Facility. Phys. Plasmas 11 (2), 339–491.CrossRefGoogle Scholar
Liu, L., Liang, Y., Ding, J., Liu, N. & Luo, X. 2018a An elaborate experiment on the single-mode Richtmyer–Meshkov instability. J. Fluid Mech. 853, R2.CrossRefGoogle Scholar
Liu, W., Li, X., Yu, C., Fu, Y., Wang, P., Wang, L. & Ye, W. 2018b Theoretical study on finite-thickness effect on harmonics in Richtmyer–Meshkov instability for arbitrary atwood numbers. Phys. Plasmas 25 (12), 122103.CrossRefGoogle Scholar
Luo, X., Dong, P., Si, T. & Zhai, Z. 2016 The Richtmyer–Meshkov instability of a ‘V’ shaped air/SF6 interface. J. Fluid Mech. 802, 186–202.CrossRefGoogle Scholar
Luo, X., Liang, Y., Si, T. & Zhai, Z. 2019 Effects of non-periodic portions of interface on Richtmyer–Meshkov instability. J. Fluid Mech. 861, 309–327.CrossRefGoogle Scholar
Luo, X., Wang, M., Si, T. & Zhai, Z. 2015 On the interaction of a planar shock with an SF6 polygon. J. Fluid Mech. 773, 366–394.CrossRefGoogle Scholar
Luo, X., Zhang, F., Ding, J., Si, T., Yang, J., Zhai, Z. & Wen, C. Y. 2018 Long-term effect of Rayleigh–Taylor stabilization on converging Richtmyer–Meshkov instability. J. Fluid Mech. 849, 231–244.CrossRefGoogle Scholar
Mariani, C., Vandenboomgaerde, M., Jourdan, G., Souffland, D. & Houas, L. 2008 Investigation of the Richtmyer–Meshkov instability with stereolithographed interfaces. Phys. Rev. Lett. 100 (25), 254503.CrossRefGoogle ScholarPubMed
Meshkov, E. E. 1969 Instability of the interface of two gases accelerated by a shock wave. Fluid Dyn. 4 (5), 101–104.CrossRefGoogle Scholar
Meyer, K. A. & Blewett, P. J. 1972 Numerical investigation of the stability of a shock-accelerated interface between two fluids. Phys. Fluids 15 (5), 753–759.CrossRefGoogle Scholar
Mikaelian, K. O. 1985 Richtmyer–Meshkov instabilities in stratified fluids. Phys. Rev. A 31 (1), 410–419.CrossRefGoogle ScholarPubMed
Mikaelian, K. O. 1990 Rayleigh–Taylor and Richtmyer–Meshkov instabilities in multilayer fluids with surface tension. Phys. Rev. A 42 (12), 7211.CrossRefGoogle ScholarPubMed
Mikaelian, K. O. 1995 Rayleigh–Taylor and Richtmyer–Meshkov instabilities in finite-thickness fluid layers. Phys. Fluids 7 (4), 888–890.CrossRefGoogle Scholar
Mikaelian, K. O. 1996 Numerical simulations of Richtmyer–Meshkov instabilities in finite-thickness fluid layers. Phys. Fluids 8 (5), 1269–1292.CrossRefGoogle Scholar
Morgan, R. V., Likhachev, O. A. & Jacobs, J. W. 2016 Rarefaction-driven Rayleigh–Taylor instability. Part 1. Diffuse-interface linear stability measurements and theory. J. Fluid Mech. 791, 34–60.CrossRefGoogle Scholar
Niederhaus, C. E. & Jacobs, J. W. 2003 Experimental study of the Richtmyer–Meshkov instability of incompressible fluids. J. Fluid Mech. 485, 243–277.CrossRefGoogle Scholar
Orlicz, G. C., Balakumar, B. J., Tomkins, C. D. & Prestridge, K. P. 2009 A Mach number study of the Richtmyer–Meshkov instability in a varicose, heavy-gas curtain. Phys. Fluids 21 (6), 064102.CrossRefGoogle Scholar
Ott, E. 1972 Nonlinear evolution of the Rayleigh–Taylor instability of a thin layer. Phys. Rev. Lett. 29 (21), 1429.CrossRefGoogle Scholar
Plesset, M. S. 1954 On the stability of fluid flows with spherical symmetry. J. Appl. Phys. 25 (1), 96–98.CrossRefGoogle Scholar
Prestridge, K. 2018 Experimental adventures in variable-density mixing. Phys. Rev. Fluids 3 (11), 110501.CrossRefGoogle Scholar
Prestridge, K., Vorobieff, P., Rightley, P. M. & Benjamin, R. F. 2000 Validation of an instability growth model using Particle Image Velocimtery measurement. Phys. Rev. Lett. 84 (19), 4353–4356.CrossRefGoogle Scholar
Ranjan, D., Oakley, J. & Bonazza, R. 2011 Shock-bubble interactions. Annu. Rev. Fluid Mech. 43, 117–140.CrossRefGoogle Scholar
Rayleigh, Lord 1883 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc. Lond. Math. Soc. 14, 170–177.Google Scholar
Richtmyer, R. D. 1960 Taylor instability in shock acceleration of compressible fluids. Commun. Pure Appl. Maths 13 (2), 297–319.CrossRefGoogle Scholar
Rightley, P. M., Vorobieff, P., Martin, R. & Benjamin, R. F. 1999 Experimental observations of the mixing transition in a shock-accelerated gas curtain. Phys. Fluids 11 (1), 186–200.CrossRefGoogle Scholar
Rikanati, A., Oron, D., Sadot, O. & Shvarts, D. 2003 High initial amplitude and high Mach number effects on the evolution of the single-mode Richtmyer–Meshkov instability. Phys. Rev. E 67, 026307.Google ScholarPubMed
Sadot, O., Erez, L., Alon, U., Oron, D., Levin, L. A., Erez, G., Ben-Dor, G. & Shvarts, D. 1998 Study of nonlinear evolution of single-mode and two-bubble interaction under Richtmyer–Meshkov instability. Phys. Rev. Lett. 80 (8), 1654–1657.CrossRefGoogle Scholar
Shimoda, J., Inoue, T., Ohira, Y., Yamazaki, R., Bamba, A. & Vink, J. 2015 On cosmic-ray production efficiency at Supernova remnant shocks propagating into realistic diffuse interstellar medium. Astrophys. J. 803 (2), 98–103.CrossRefGoogle Scholar
Taylor, G. 1950 The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond. A 201 (1065), 192–196.Google Scholar
Tomkins, C., Kumar, S., Orlicz, G. & Prestridge, K. 2008 An experimental investigation of mixing mechanisms in shock-accelerated flow. J. Fluid Mech. 611, 131–150.CrossRefGoogle Scholar
Tomkins, C. D., Balakumar, B. J., Orlicz, G., Prestridge, K. P. & Ristorcelli, J. R. 2013 Evolution of the density self-correlation in developing Richtmyer–Meshkov turbulence. J. Fluid Mech. 735, 288–306.CrossRefGoogle Scholar
Vandenboomgaerde, M., Rouzier, P., Souffland, D., Biamino, L., Jourdan, G., Houas, L. & Mariani, C. 2018 Nonlinear growth of the converging Richtmyer–Meshkov instability in a conventional shock tube. Phys. Rev. Fluids 3 (1), 014001.CrossRefGoogle Scholar
Zhai, Z., Liang, Y., Liu, L., Ding, J., Luo, X. & Zou, L. 2018a Interaction of rippled shock wave with flat fast–slow interface. Phys. Fluids 30 (4), 046104.CrossRefGoogle Scholar
Zhai, Z., Zou, L., Wu, Q. & Luo, X. 2018b Review of experimental Richtmyer–Meshkov instability in shock tube: from simple to complex. Proc. Inst. Mech. Engrs, Part C 232 (16), 2830–2849.CrossRefGoogle Scholar
Zhang, Q., Deng, S. & Guo, W. 2018 Quantitative theory for the growth rate and amplitude of the compressible Richtmyer–Meshkov instability at all density ratios. Phys. Rev. Lett. 121 (17), 174502.CrossRefGoogle ScholarPubMed
Zhang, Q. & Guo, W. 2016 Universality of finger growth in two-dimensional Rayleigh–Taylor and Richtmyer–Meshkov instabilities with all density ratios. J. Fluid Mech. 786, 47–61.CrossRefGoogle Scholar
Zhou, Y. 2017a Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 720–722, 1–136.Google Scholar
Zhou, Y. 2017b Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 723–725, 1–160.Google Scholar
Zou, L., Liu, J., Liao, S., Zheng, X., Zhai, Z. & Luo, X. 2017 Richtmyer–Meshkov instability of a flat interface subjected to a rippled shock wave. Phys. Rev. E 95 (1), 013107.Google ScholarPubMed