Hostname: page-component-76fb5796d-2lccl Total loading time: 0 Render date: 2024-04-27T14:58:09.234Z Has data issue: false hasContentIssue false
  • English
  • Français

CONSERVATION THEOREMS ON SEMI-CLASSICAL ARITHMETIC

Part of: Proof theory and constructive mathematics General logic

Published online by Cambridge University Press:  17 March 2022

MAKOTO FUJIWARA Open the ORCID record for MAKOTO FUJIWARA [Opens in a new window]  and
TAISHI KURAHASHI Open the ORCID record for TAISHI KURAHASHI [Opens in a new window]
MAKOTO FUJIWARA*
Affiliation:
SCHOOL OF SCIENCE AND TECHNOLOGY MEIJI UNIVERSITY 1-1-1 HIGASHI-MITA, TAMA-KU, KAWASAKI-SHI KANAGAWA 214-8571, JAPAN
TAISHI KURAHASHI
Affiliation:
GRADUATE SCHOOL OF SYSTEM INFORMATICS KOBE UNIVERSITY 1-1 ROKKODAI, NADA, KOBE 657-8501, JAPAN E-mail: kurahashi@people.kobe-u.ac.jp
*
E-mail: makotofujiwara@meiji.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

We systematically study conservation theorems on theories of semi-classical arithmetic, which lie in-between classical arithmetic 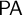$\mathsf {PA}$ and intuitionistic arithmetic $\mathsf {HA}$. Using a generalized negative translation, we first provide a structured proof of the fact that 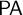$\mathsf {PA}$ is 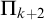$\Pi _{k+2}$-conservative over 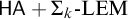$\mathsf {HA} + {\Sigma _k}\text {-}\mathrm {LEM}$ where 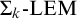${\Sigma _k}\text {-}\mathrm {LEM}$ is the axiom scheme of the law-of-excluded-middle restricted to formulas in 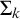$\Sigma _k$. 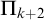In addition, we show that this conservation theorem is optimal in the sense that for any semi-classical arithmetic T, if $\mathsf {PA}$ is $\Pi _{k+2}$-conservative over T, then 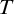${T}$ proves ${\Sigma _k}\text {-}\mathrm {LEM}$. In the same manner, we also characterize conservation theorems for other well-studied classes of formulas by fragments of classical axioms or rules. This reveals the entire structure of conservation theorems with respect to the arithmetical hierarchy of classical principles.

Keywords

conservation theoremssemi-classical arithmeticintuitionistic arithmetic

MSC classification

Primary: 03B20: Subsystems of classical logic (including intuitionistic logic)
Secondary: 03F03: Proof theory, general 03F30: First-order arithmetic and fragments 03F50: Metamathematics of constructive systems
Type
Article
Information
The Journal of Symbolic Logic , Volume 88 , Issue 4 , December 2023 , pp. 1469 - 1496
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akama, Y., Berardi, S., Hayashi, S., and Kohlenbach, U., An arithmetical hierarchy of the law of excluded middle and related principles. Proceedings of the 19th Annual IEEE Symposium on Logic in Computer Science (LICS’04) , 2004, pp. 192–301.Google Scholar
Boolos, G., The Logic of Provability , Cambridge University Press, Cambridge, 1993.Google Scholar
van Dalen, D., Logic and Structure , fifth ed., Universitext, Springer, London, 2013.Google Scholar
Friedman, H., Classically and intuitionistically provably recursive functions , Higher Set Theory (Müller, G. H. and Scott, D. S., editors), Springer, Berlin; Heidelberg, 1978, pp. 21–27.Google Scholar
Fujiwara, M., Ishihara, H., Nemoto, T., Suzuki, N.-Y., and Yokoyama, K., Extended frames and separations of logical principles, submitted, 2021. Available at https://researchmap.jp/makotofujiwara/misc/30348506.Google Scholar
Fujiwara, M. and Kurahashi, T., Refining the arithmetical hierarchy of classical principles, submitted, 2020. Available at https://arxiv.org/abs/2010.11527.Google Scholar
Fujiwara, M. and Kurahashi, T., Prenex normal form theorems in semi-classical arithmetic, Journal of Symbolic Logic, vol. 86 (2021), no. 3, pp. 1124–1153.Google Scholar
Hayashi, S. and Nakata, M., Towards limit computable mathematics , Types for Proofs and Programs (Callaghan, P., Luo, Z., McKinna, J., Pollack, R., and Pollack, R., editors), Springer, Berlin; Heidelberg, 2002, pp. 125–144.Google Scholar
Ishihara, H., A note on the Gödel–Gentzen translation . Mathematical Logic Quarterly , vol. 46 (2000), no. 1, pp. 135–137.Google Scholar
Ishihara, H., Some conservative extension results on classical and intuitionistic sequent calculi , Logic, Construction, Computation (Berger, U., Diener, H., Schuster, P., and Monika, S., editors), Ontos Mathematical Logic, De Gruyter, Berlin; Boston, 2012, pp. 289–304.CrossRefGoogle Scholar
Kaye, R., Paris, J., and Dimitracopoulos, C., On parameter free induction schemas, Journal of Symbolic Logic, vol. 53 (1988), no. 4, pp. 1082–1097.Google Scholar
Kohlenbach, U. and Safarik, P., Fluctuations, effective learnability and metastability in analysis. Annals of Pure and Applied Logic , vol. 165 (2014), no. 1, pp. 266–304.CrossRefGoogle Scholar
Troelstra, A. S. (editor), Metamathematical Investigation of Intuitionistic Arithmetic and Analysis , Lecture Notes in Mathematics, 344, Springer, Berlin; New York, 1973.Google Scholar
Troelstra, A. S. and van Dalen, D., Constructivism in Mathematics, An Introduction , vol. I, Studies in Logic and the Foundations of Mathematics, 121, North-Holland, Amsterdam, 1988.Google Scholar