Hostname: page-component-848d4c4894-xfwgj Total loading time: 0 Render date: 2024-06-29T06:30:30.487Z Has data issue: false hasContentIssue false
  • English
  • Français

The statistics of Weil's trigonometric sums

Published online by Cambridge University Press:  24 October 2008

R. W. K. Odoni
R. W. K. Odoni
Affiliation:
University of Glasgow
Get access Rights & Permissions [Opens in a new window]

Extract

Let F be the finite field of q = pn elements and let F0 be its prime subfield; thus, card F0 = p. For polynomials f ∈ F[x] and non-principal additive characters η of F A. Weil (1) proved the estimate

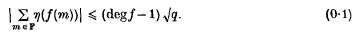
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 74 , Issue 3 , November 1973 , pp. 467 - 471
Copyright
Copyright © Cambridge Philosophical Society 1973

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Weil, A. Sur les courbes algébriques, et les variétés qui s'en déduisent. Act. Sci. Indust. 1041 (Paris, Hermann, 1948).Google Scholar
(2)Stepanov, S. A.Bounds for Weil's sums, by elementary methods [Russian]. Izv. Akad. Nauk SSSR. Ser. Mat. 34 (1970), 1015–1037.Google Scholar
(3)Postnikov, A. G.Ergodic properties of measures in the theory of Diophantine approximation. Steklov Inst. Monograph, no. 82 (1966).Google Scholar
(4)Gnedenko, B. V.Theory of probability (Moscow, Mir, 1969).Google Scholar
(5)Bowman, F.Introduction to Bessel functions (Dover, New York, 1958), pp. 113–114.Google Scholar
(6)Rayleigh, Lord. Collected papers (Cambridge University Press, 1920), vol. 6, 604–626.Google Scholar
(7)Kluyver, J. C.A local probability problem. Proceedings of the Section of Science, Koningslijk Akad. van Wetensk. Amsterdam 8 (1906), 341–350.Google Scholar
(8)Breiman, L.Probability (Addison-Wesley, 1968).Google Scholar