Hostname: page-component-76fb5796d-5g6vh Total loading time: 0 Render date: 2024-04-26T02:06:16.969Z Has data issue: false hasContentIssue false
  • English
  • Français

Decay at infinity for solutions to some fractional parabolic equations

Part of: Partial differential equations Parabolic equations and systems

Published online by Cambridge University Press:  14 March 2024

Agnid Banerjee  and
Abhishek Ghosh
Agnid Banerjee
Affiliation:
Department of Mathematics, TIFR CAM, Bangalore 560065, India (agnidban@gmail.com)
Abhishek Ghosh
Affiliation:
Department of Functional Analysis, Institute of Mathematics, Polish Academy of Sciences, Ul. Śniadeckich 8, Warsaw 00-656, Poland (abhi170791@gmail.com;agosh@impan.pl)
Get access Rights & Permissions [Opens in a new window]

Abstract

For $s\in [\tfrac {1}{2},\, 1)$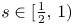, let $u$ 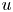solve $(\partial _t - \Delta )^s u = Vu$ 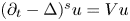in $\mathbb {R}^{n} \times [-T,\, 0]$ 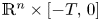for some $T>0$ where $||V||_{ C^2(\mathbb {R}^n \times [-T, 0])} < \infty$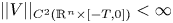. We show that if for some $0<\mathfrak {K} < T$ 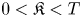and $\epsilon >0$

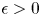\[ {\unicode{x2A0D}}-_{[-\mathfrak{K},\, 0]} u^2(x, t) {\rm d}t \leq Ce^{-|x|^{2+\epsilon}}\ \forall x \in \mathbb{R}^n, \]
then $u \equiv 0$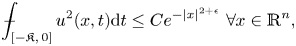 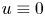in $\mathbb {R}^{n} \times [-T,\, 0]$.

Keywords

non-local parabolic equationsLandis conjecturefractional heatstrong unique continuationCarleman estimates

MSC classification

Primary: 35A02: Uniqueness problems 35B60: Continuation and prolongation of solutions 35K05: Heat equation
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 37
Check for updates
Copyright
Copyright © The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aronszajn, N., Krzywicki, A. and Szarski, J.. A unique continuation theorem for exterior differential forms on Riemannian manifolds. Ark. Mat. 4 (1962), 417–453.CrossRefGoogle Scholar
Arya, V. and Banerjee, A.. Quantitative uniqueness for fractional heat type operators. Calc. Var. 62 (2023), 47. https://doi.org/10.1007/s00526-023-02535-1.CrossRefGoogle Scholar
Arya, V., Banerjee, A., Danielli, D. and Garofalo, N.. Space-like strong unique continuation for some fractional parabolic equations. J. Funct. Anal. 284 (2023), 109723.CrossRefGoogle Scholar
Audrito, A. and Terracini, S.. On the nodal set of solutions to a class of nonlocal parabolic reaction-diffusion equations, arXiv:1807.10135, to appear in Memoirs of AMS.Google Scholar
Banerjee, A. and Garofalo, N.. Monotonicity of generalized frequencies and the strong unique continuation property for fractional parabolic equations. Adv. Math. 336 (2018), 149–241.CrossRefGoogle Scholar
Banerjee, A. and Garofalo, N.. On the space-like analyticity in the extension problem for nonlocal parabolic equations. Proc. Amer. Math. Soc. 151 (2023), 1235–1246.CrossRefGoogle Scholar
Banerjee, A. and Garofalo, N.. On the forward in time propagation of zeros in fractional heat type problems, eprint arXiv:2301.12239, to appear in Archiv der Mathematik.Google Scholar
Banerjee, A. and Ghosh, A.. Sharp asymptotic of solutions to some nonlocal parabolic equations, eprint arXiv:2306.00341.Google Scholar
Banerjee, A. and Senapati, S.. The Calderón problem for space-time fractional parabolic operators with variable coefficients, eprint arXiv:2205.12509.Google Scholar
Banerjee, A. and Senapati, S.. Extension problem for the fractional parabolic Lamé operator and unique continuation, eprint arXiv:2208.11598.Google Scholar
Bellova, K. and Lin, F.. Nodal sets of Steklov eigenfunctions. Calc. Var. Partial Differ. Equ. 54 (2015), 2239–2268.CrossRefGoogle Scholar
Bourgain, J. and Kenig, C.. On localization in the continuous Anderson-Bernoulli model in higher dimension. Invent. Math. 161 (2005), 389–426.CrossRefGoogle Scholar
Chen, X.. A strong unique continuation theorem for parabolic equations. Math. Ann. 311 (1996), 603–630.CrossRefGoogle Scholar
Escauriaza, L. and Fernández, F. J.. Unique continuation for parabolic operators. Ark. Mat. 41 (2003), 35–60.CrossRefGoogle Scholar
Escauriaza, L., Fernández, F. J. and Vessella, S.. Doubling properties of caloric functions. Appl. Anal. 85 (2006), 205–223.CrossRefGoogle Scholar
Escauriaza, L., Kenig, C. E., Ponce, G. and Vega, L.. Hardy uncertainty principle, convexity and parabolic evolutions. Commun. Math. Phys. 346 (2016), 667–678.CrossRefGoogle Scholar
Escauriaza, L., Kenig, C., Ponce, G. and Vega, L.. Decay at infinity of caloric functions within characteristic hyperplanes. Math. Res. Lett. 13 (2006), 441–453.CrossRefGoogle Scholar
Escauriaza, L., Kenig, C., Ponce, G. and Vega, L.. Hardy's uncertainty principle, convexity and Schrödinger evolutions. J. Eur. Math. Soc. (JEMS) 10 (2008), 883–907.Google Scholar
Escauriaza, L., Seregin, G. and Sverak, V.. Backward uniqueness for parabolic equations. Arch. Ration. Mech. Anal. 169 (2003), 147–157.CrossRefGoogle Scholar
Fall, M. and Felli, V.. Unique continuation property and local asymptotics of solutions to fractional elliptic equations. Commun. Partial Differ. Equ. 39 (2014), 354–397.CrossRefGoogle Scholar
Felli, V., Primo, A. and Siclari, G.. On fractional parabolic equations with Hardy-type potentials, eprint arXiv:2212.03744v2.Google Scholar
Garofalo, N. (2020) Two classical properties of the Bessel quotient $I_{\nu +1}/I_\nu$ and their implications in PDE's, Advances in harmonic analysis and partial differential equations, Contemp. Math., Vol. 748, (Amer. Math. Soc., Providence, RI), pp. 57–97.Google Scholar
Garofalo, N. and Lin, F.. Monotonicity properties of variational integrals, $A_p$ weights and unique continuation. Indiana Univ. Math. J. 35 (1986), 245–268.CrossRefGoogle Scholar
Jones, B. F.. Lipschitz spaces and the heat equation. J. Math. Mech. 18 (1968/69), 379–409.Google Scholar
Kenig, C., Silvestre, L. and Wang, J.. On Landis’ conjecture in the plane. Commun. Partial Differ. Equ. 40 (2015), 766–789.CrossRefGoogle Scholar
Kondratev, V. and Landis, E., Qualitative theory of second-order linear partial differential equations (Russian). Partial Differ. Equ. 3 99–215.Google Scholar
Lai, R., Lin, Y. and Rüland, A.. The Calderón problem for a space-time fractional parabolic equation. SIAM J. Math. Anal. 52 (2020), 2655–2688.CrossRefGoogle Scholar
Landis, E. and Oleinik, O.. Generalized analyticity and some related properties of solutions of elliptic and parabolic equations. Russian Math. Surv. 29 (1974), 195–212.CrossRefGoogle Scholar
Lieberman, G.. Second order parabolic differential equations (World Scientific Publishing Co., Inc., River Edge, NJ, 1996).CrossRefGoogle Scholar
Logunov, A., Malinnikova, E., Nadirashvili, N. and Nazarov, F.. The Landis conjecture on exponential decay, eprint arXiv:2007.07034.Google Scholar
Meshkov, V.. Weighted differential inequalities and their application for estimates of the decrease at infinity of the solutions of second-order elliptic equations. (Russian), Trans. Proc. Steklov Inst. Math. 1 (1992)145–166.Google Scholar
Micu, S. and Zuazua, E.. On the lack of null-controllability of the heat equation in the half space. Port. Math. 58 (2001), 1–24.Google Scholar
Nyström, K. and Sande, O.. Extension properties and boundary estimates for a fractional heat operator. Nonlinear Anal. 140 (2016), 29–37.CrossRefGoogle Scholar
Poon, C.. Unique continuation for parabolic equations. Commun. Partial Differ. Equ. 21 (1996), 521–539.CrossRefGoogle Scholar
Rüland, A. (2013) On some rigidity properties in PDEs, Dissertation, Rheinischen Friedrich-Wilhelms-Universität Bonn.Google Scholar
Rüland, A.. Unique continuation for fractional Schrödinger equations with rough potentials. Commun. Partial Differ. Equ. 40 (2015), 77–114.CrossRefGoogle Scholar
Rüland, A.. On quantitative unique continuation properties of fractional Schrödinger equations: doubling, vanishing order and nodal domain estimates. Trans. Amer. Math. Soc. 369 (2017), 2311–2362.CrossRefGoogle Scholar
Rüland, A. and Salo, Mikko. Quantitative approximation properties for the fractional heat equation. Math. Control Relat. Fields 10 (2020), 1–26.CrossRefGoogle Scholar
Rüland, A. and Wang, J. N.. On the fractional Landis conjecture. J. Funct. Anal. 277 (2019), 3236–3270.CrossRefGoogle Scholar
Roncal, L., Stan, D. and Vega, L.. Carleman type inequalities for fractional relativistic operators. Rev. Mat. Complut. 36 (2023), 301–332.CrossRefGoogle Scholar
Samko, S. (2002) Hypersingular integrals and their applications, Vol. 5 of Analytical Methods and Special Functions (Taylor & Francis Group, London).CrossRefGoogle Scholar
Seregin, G. and Sverak, V. (2002) The Navier-Stokes equations and backwards uniqueness, Nonlinear Problems of Mathematical Physics and Related Topics, Vol. 2, (Kluwer Acad./Plenum Publ.) pp. 359–370.Google Scholar
Stinga, P. and Torrea, J.. Regularity theory and extension problem for fractional nonlocal parabolic equations and the master equation. SIAM J. Math. Anal. 49 (2017), 3893–3924.CrossRefGoogle Scholar
Yu, H.. Unique continuation for fractional orders of elliptic equations. Ann. PDE. 3 (2017), 16.CrossRefGoogle Scholar
Zhu, J.. Doubling property and vanishing order of Steklov eigenfunctions. Commun. Partial Differ. Equ. 40 (2015), 1498–1520.CrossRefGoogle Scholar