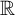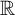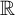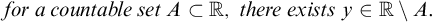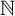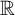1 Introduction
1.1 Summary
Like Hilbert [Reference Hilbert34], we believe the infinite to be a central object of study in mathematics. That the infinite comes in ‘different sizes’ is a relatively new insight, due to Cantor around 1874 [Reference Cantor19], in the guise of the uncountability of
![]() ${\mathbb R}$
, also known simply as Cantor’s theorem. We have previously studied the uncountability of
${\mathbb R}$
, also known simply as Cantor’s theorem. We have previously studied the uncountability of
![]() ${\mathbb R}$
in the guise of the following third-order
Footnote
1
principles.
${\mathbb R}$
in the guise of the following third-order
Footnote
1
principles.
-
•
 $\mathsf {NIN}$
: there is no injection from
$\mathsf {NIN}$
: there is no injection from
 $[0,1]$
to
$[0,1]$
to
 ${\mathbb N}$
.
${\mathbb N}$
. -
•
 $\mathsf {NBI}$
: there is no bijection from
$\mathsf {NBI}$
: there is no bijection from
 $[0,1]$
to
$[0,1]$
to
 ${\mathbb N}$
.
${\mathbb N}$
.
In particular, as shown in [Reference Normann and Sanders69–Reference Normann and Sanders71], the principles
![]() $\mathsf {NBI}$
and
$\mathsf {NBI}$
and
![]() $\mathsf {NIN}$
are hard to prove in terms of conventionalFootnote
2
comprehension, while the objects claimed to exist are hard to compute in terms of the other data, in the sense of Kleene’s computability theory based on S1–S9 [Reference Kleene46, Reference Longley and Normann58]. As shown in [Reference Sanders79], this hardness remains if we restrict the mappings in
$\mathsf {NIN}$
are hard to prove in terms of conventionalFootnote
2
comprehension, while the objects claimed to exist are hard to compute in terms of the other data, in the sense of Kleene’s computability theory based on S1–S9 [Reference Kleene46, Reference Longley and Normann58]. As shown in [Reference Sanders79], this hardness remains if we restrict the mappings in
![]() $\mathsf {NIN}$
and
$\mathsf {NIN}$
and
![]() $\mathsf {NBI}$
to well-known function classes, e.g., based on bounded variation, Borel, upper semi-continuity, and quasi-continuity. Moreover, many basic third-order theorems imply
$\mathsf {NBI}$
to well-known function classes, e.g., based on bounded variation, Borel, upper semi-continuity, and quasi-continuity. Moreover, many basic third-order theorems imply
![]() $\mathsf {NIN}$
or
$\mathsf {NIN}$
or
![]() $\mathsf {NBI}$
, and the same at the computational level (see [Reference Normann and Sanders68–Reference Normann and Sanders71, Reference Sanders77–Reference Sanders80]). Finally,
$\mathsf {NBI}$
, and the same at the computational level (see [Reference Normann and Sanders68–Reference Normann and Sanders71, Reference Sanders77–Reference Sanders80]). Finally,
![]() $\mathsf {NIN}$
and
$\mathsf {NIN}$
and
![]() $\mathsf {NBI}$
seem to be the weakest natural third-order principles that boast all the aforementioned properties.
$\mathsf {NBI}$
seem to be the weakest natural third-order principles that boast all the aforementioned properties.
For all these reasons, the study of the uncountability of
![]() ${\mathbb R}$
in Reverse Mathematics (RM for short; see Section 1.3.1) seems like a natural enterprise. However, try as we might we have not managed to obtain elegant equivalences for
${\mathbb R}$
in Reverse Mathematics (RM for short; see Section 1.3.1) seems like a natural enterprise. However, try as we might we have not managed to obtain elegant equivalences for
![]() $\mathsf {NIN}$
and
$\mathsf {NIN}$
and
![]() $\mathsf {NBI}$
, working in Kohlenbach’s higher-order Reverse Mathematics (see Section 1.3.1).
$\mathsf {NBI}$
, working in Kohlenbach’s higher-order Reverse Mathematics (see Section 1.3.1).
As argued in detail in Section 2, our main problem is that countable sets that occur ‘in the wild’ do not have injections (let alone bijections) to
![]() ${\mathbb N}$
that can be defined in weak logical systems. By contrast, the (equivalent over
${\mathbb N}$
that can be defined in weak logical systems. By contrast, the (equivalent over
![]() $\mathsf {ZF}$
and weaker systems) definition of countable set as in Definitions 1.1 and 1.2 is much more suitable for the development of higher-order RM and is central to this paper.
$\mathsf {ZF}$
and weaker systems) definition of countable set as in Definitions 1.1 and 1.2 is much more suitable for the development of higher-order RM and is central to this paper.
Definition 1.1. A set
![]() $A\subset {\mathbb R}$
is height countable if there is a height function
$A\subset {\mathbb R}$
is height countable if there is a height function
![]() $H:{\mathbb R}\rightarrow {\mathbb N}$
for A, i.e., for all
$H:{\mathbb R}\rightarrow {\mathbb N}$
for A, i.e., for all
![]() $n\in {\mathbb N}$
,
$n\in {\mathbb N}$
,
![]() $A_{n}:= \{ x\in A: H(x)<n\}$
is finite.
$A_{n}:= \{ x\in A: H(x)<n\}$
is finite.
Definition 1.2 (Finite set)
Any
![]() $X\subset {\mathbb R}$
is finite if there is
$X\subset {\mathbb R}$
is finite if there is
![]() $N\in {\mathbb N}$
such that for any finite sequence
$N\in {\mathbb N}$
such that for any finite sequence
![]() $(x_{0}, \dots , x_{N})$
of distinct reals, there is
$(x_{0}, \dots , x_{N})$
of distinct reals, there is
![]() $i\leq N$
such that
$i\leq N$
such that
![]() $x_{i}\not \in X$
.
$x_{i}\not \in X$
.
The notion of ‘height function’ can be found in the literature in connection to countability [Reference Huxley40, Reference Kolmogorov and Fomin49, Reference Moll59, Reference Royden75, Reference Vatssa97], while ‘height countable’ essentially amounts to union over
![]() ${\mathbb N}$
of finite sets. By contrast, we believe Definition 1.2 has not been studied in the literature. Our move away from injections/bijections towards height functions and finite sets constitutes a ‘shift of definition’ which has ample historical precedent in RM and constructive mathematics, as discussed in Remark 2.1. We note that Kleene’s quantifier
${\mathbb N}$
of finite sets. By contrast, we believe Definition 1.2 has not been studied in the literature. Our move away from injections/bijections towards height functions and finite sets constitutes a ‘shift of definition’ which has ample historical precedent in RM and constructive mathematics, as discussed in Remark 2.1. We note that Kleene’s quantifier
![]() $(\exists ^{2})$
from Section 1.3.2 is needed to make Definition 1.2 well-behaved, as discussed in more detail in Remark 3.5.
$(\exists ^{2})$
from Section 1.3.2 is needed to make Definition 1.2 well-behaved, as discussed in more detail in Remark 3.5.
In more detail, we shall establish a large number of equivalences for the following principle, which is based on Definition 1.1 and expresses that
![]() $[0,1]$
is uncountable:
$[0,1]$
is uncountable:
-
•
 $\mathsf {NIN}_{\mathsf {alt}}$
: the unit interval is not height countable.
$\mathsf {NIN}_{\mathsf {alt}}$
: the unit interval is not height countable.
In particular, we show in Section 3 that
![]() $\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to the following natural principles, working in Kohlenbach’s higher-order Reverse Mathematics, introduced in Section 1.3.1. Recall that a regulated function has left and right limits everywhere, as studied by Bourbaki for Riemann integration (see Section 1.3.3).
$\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to the following natural principles, working in Kohlenbach’s higher-order Reverse Mathematics, introduced in Section 1.3.1. Recall that a regulated function has left and right limits everywhere, as studied by Bourbaki for Riemann integration (see Section 1.3.3).
-
(i) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a point
$f:[0,1]\rightarrow {\mathbb R}$
, there is a point
 $x\in [0,1]$
where f is continuous (or quasi-continuous, or lower semi-continuous, or Darboux).
$x\in [0,1]$
where f is continuous (or quasi-continuous, or lower semi-continuous, or Darboux). -
(ii) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, the set of continuity points is dense in
$f:[0,1]\rightarrow {\mathbb R}$
, the set of continuity points is dense in
 $[0,1]$
.
$[0,1]$
. -
(iii) For regulated
 $f:[0,1]\rightarrow [0,1]$
with Riemann integral
$f:[0,1]\rightarrow [0,1]$
with Riemann integral
 $\int _{0}^{1}f(x)dx=0$
, there is
$\int _{0}^{1}f(x)dx=0$
, there is
 $x\in [0,1]$
with
$x\in [0,1]$
with
 $f(x)=0$
(Bourbaki [Reference Bourbaki10, p. 61, Corollary 1]).
$f(x)=0$
(Bourbaki [Reference Bourbaki10, p. 61, Corollary 1]). -
(iv) (Volterra [Reference Volterra98]) For regulated
 $f,g:[0,1]\rightarrow {\mathbb R}$
, there is
$f,g:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
such that f and g are both continuous or both discontinuous at x.
$x\in [0,1]$
such that f and g are both continuous or both discontinuous at x. -
(v) (Volterra [Reference Volterra98]) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is either
$f:[0,1]\rightarrow {\mathbb R}$
, there is either
 $q\in {\mathbb Q}\cap [0,1]$
where f is discontinuous, or
$q\in {\mathbb Q}\cap [0,1]$
where f is discontinuous, or
 $x\in [0,1]\setminus {\mathbb Q}$
where f is continuous.
$x\in [0,1]\setminus {\mathbb Q}$
where f is continuous. -
(vi) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $y\in (0,1)$
where
$y\in (0,1)$
where
 $F(x):=\lambda x.\int _{0}^{x}f(t)dt$
is differentiable with derivative equal to
$F(x):=\lambda x.\int _{0}^{x}f(t)dt$
is differentiable with derivative equal to
 $f(y)$
.
$f(y)$
. -
(vii) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there are
$f:[0,1]\rightarrow {\mathbb R}$
, there are
 $a, b\in [0,1]$
such that
$a, b\in [0,1]$
such that
 $\{ x\in [0,1]:f(a)\leq f(x)\leq f(b)\}$
is infinite.
$\{ x\in [0,1]:f(a)\leq f(x)\leq f(b)\}$
is infinite. -
(viii) Blumberg’s theorem [Reference Blumberg8] restricted to regulated functions.
A full list of equivalences may be found in Section 3.3, while we obtain similar (but also very different) results for functions of bounded variation in Section 3.4. We introduce all required definitions in Section 1.3. Some of the above theorems, including items (iv) and (v) in the list, stem from Volterra’s early work (1881) in the spirit of-but predating-the Baire category theorem, as discussed in Section 1.2.
Now, comparing items (i) and (ii) suggests that our results are robust as follows:
A system is robust if it is equivalent to small perturbations of itself. [Reference Montalbán60, p. 432; emphasis in original]
Most of our results shall be seen to exhibit a similar (or stronger) level of robustness. In this light, we feel that the uncountability of
![]() ${\mathbb R}$
deserves the moniker ‘big’ system in the way this notion is used in second-order RM, namely as boasting many equivalences from various different fields of mathematics.
${\mathbb R}$
deserves the moniker ‘big’ system in the way this notion is used in second-order RM, namely as boasting many equivalences from various different fields of mathematics.
Next, items (i)–(viii) above imply
![]() $\mathsf {NIN}$
and are therefore hard to prove in the sense of Footnote 2. By contrast, we show in Section 3.5 that adding the extra condition ‘Baire 1’, makes these items provable from (essentially) arithmetical comprehension. While regulated functions are of course Baire 1, say over
$\mathsf {NIN}$
and are therefore hard to prove in the sense of Footnote 2. By contrast, we show in Section 3.5 that adding the extra condition ‘Baire 1’, makes these items provable from (essentially) arithmetical comprehension. While regulated functions are of course Baire 1, say over
![]() $\mathsf {ZF}$
, there is no contradiction here as the statement a regulated function on the unit interval is Baire 1 already implies
$\mathsf {ZF}$
, there is no contradiction here as the statement a regulated function on the unit interval is Baire 1 already implies
![]() $\mathsf {NIN}$
(see [Reference Normann and Sanders72, Section 2.8]). Other restrictions of items (i)–(viii), e.g., involving semi-continuity or Baire 2, are still equivalent to
$\mathsf {NIN}$
(see [Reference Normann and Sanders72, Section 2.8]). Other restrictions of items (i)–(viii), e.g., involving semi-continuity or Baire 2, are still equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, as shown in Section 3.3.3.
$\mathsf {NIN}_{\mathsf {alt}}$
, as shown in Section 3.3.3.
Finally, this paper deals with the RM of the uncountability of
![]() ${\mathbb R}$
, while stronger ‘completeness’ properties of the reals, namely related to measure and category, are studied in [Reference Sanders83]. In particular, the latter paper develops the higher-order RM of the Baire category theorem and Tao’s pigeon hole principle for measure spaces [Reference Tao93]. We do not currently know of a principle weaker than the uncountability of
${\mathbb R}$
, while stronger ‘completeness’ properties of the reals, namely related to measure and category, are studied in [Reference Sanders83]. In particular, the latter paper develops the higher-order RM of the Baire category theorem and Tao’s pigeon hole principle for measure spaces [Reference Tao93]. We do not currently know of a principle weaker than the uncountability of
![]() ${\mathbb R}$
that yields (interesting) RM-equivalences.
${\mathbb R}$
that yields (interesting) RM-equivalences.
1.2 Volterra’s early work and related results
We introduce Volterra’s early work from [Reference Volterra98] as it pertains to this paper, as well as related results.
First of all, the Riemann integral was groundbreaking for a number of reasons, including its ability to integrate functions with infinitely many points of discontinuity, as shown by Riemann himself [Reference Riemann74]. A natural question is then ‘how discontinuous’ a Riemann integrable function can be. In this context, Thomae introduced the function
![]() $T:{\mathbb R}\rightarrow {\mathbb R}$
around 1875 in [Reference Thomae95, p. 14, Section 20]:
$T:{\mathbb R}\rightarrow {\mathbb R}$
around 1875 in [Reference Thomae95, p. 14, Section 20]:
 $$ \begin{align} T(x):= \begin{cases} 0, & \mathrm{if} \ x\in {\mathbb R}\setminus{\mathbb Q},\\ \frac{1}{q}, & \mathrm{if} \ x=\frac{p}{q} \ \mathrm{and} \ p, q \ \mathrm{are \ co}\text{-}\mathrm{prime}. \end{cases} \end{align} $$
$$ \begin{align} T(x):= \begin{cases} 0, & \mathrm{if} \ x\in {\mathbb R}\setminus{\mathbb Q},\\ \frac{1}{q}, & \mathrm{if} \ x=\frac{p}{q} \ \mathrm{and} \ p, q \ \mathrm{are \ co}\text{-}\mathrm{prime}. \end{cases} \end{align} $$
Thomae’s function T is integrable on any interval, but has a dense set of points of discontinuity, namely
![]() ${\mathbb Q}$
, and a dense set of points of continuity, namely
${\mathbb Q}$
, and a dense set of points of continuity, namely
![]() ${\mathbb R}\setminus {\mathbb Q}$
.
${\mathbb R}\setminus {\mathbb Q}$
.
The perceptive student, upon seeing Thomae’s function as in (1.1), will ask for a function continuous at each rational point and discontinuous at each irrational one. Such a function cannot exist, as is generally proved using the Baire category theorem. However, Volterra in [Reference Volterra98] already established this negative result about 20 years before the publication of the Baire category theorem.
Secondly, as to the content of Volterra’s paper [Reference Volterra98], we find the following theorem on the first page, where a function is pointwise discontinuous if it has a dense set of continuity points.
Theorem 1.3 (Volterra, 1881)
There do not exist pointwise discontinuous functions defined on an interval for which the continuity points of one are the discontinuity points of the other, and vice versa.
Volterra then states two corollaries, of which the following is perhaps well-known in ‘popular mathematics’ and constitutes the aforementioned negative result.
Corollary 1.4 (Volterra, 1881)
There is no
![]() ${\mathbb R}\rightarrow {\mathbb R}$
-function that is continuous on
${\mathbb R}\rightarrow {\mathbb R}$
-function that is continuous on
![]() ${\mathbb Q}$
and discontinuous on
${\mathbb Q}$
and discontinuous on
![]() ${\mathbb R}\setminus {\mathbb Q}$
.
${\mathbb R}\setminus {\mathbb Q}$
.
Thirdly, we shall study Volterra’s theorem and corollary restricted to regulated functions (see Section 1.3.3). The latter kinds of functions are automatically ‘pointwise discontinuous’ in the sense of Volterra.
Fourth, Volterra’s results from [Reference Volterra98] are generalised in [Reference Gauld30, Reference Silva and Wu86]. The following theorem is immediate from these generalisations.
Theorem 1.5. For any countable dense set
![]() $D\subset [0,1]$
and
$D\subset [0,1]$
and
![]() $f:[0,1]\rightarrow {\mathbb R}$
, f is either discontinuous at some point in D or continuous at some point in
$f:[0,1]\rightarrow {\mathbb R}$
, f is either discontinuous at some point in D or continuous at some point in
![]() $[0,1]\setminus D$
.
$[0,1]\setminus D$
.
Perhaps surprisingly, this generalisation (restricted to bounded variation or regulated functions) is still equivalent to the uncountability of
![]() ${\mathbb R}$
. The same holds for the related Blumberg’s theorem with the same restrictions.
${\mathbb R}$
. The same holds for the related Blumberg’s theorem with the same restrictions.
Theorem 1.6 (Blumberg’s theorem [Reference Blumberg8])
For any
![]() $f:{\mathbb R}\rightarrow {\mathbb R}$
, there is a dense subset
$f:{\mathbb R}\rightarrow {\mathbb R}$
, there is a dense subset
![]() $D\subset {\mathbb R}$
such that the restriction of f to D, usually denoted
$D\subset {\mathbb R}$
such that the restriction of f to D, usually denoted
![]() $f_{\upharpoonright D}$
, is continuous.
$f_{\upharpoonright D}$
, is continuous.
To be absolutely clear, the conclusion of Blumberg’s theorem means that
where the underlined quantifier marks the difference with ‘usual’ continuity.
1.3 Preliminaries and definitions
We briefly introduce Reverse Mathematics in Section 1.3.1. We introduce some essential axioms (Section 1.3.2) and definitions (Section 1.3.3). A full introduction may be found in, e.g., [Reference Normann and Sanders69, Section 2].
1.3.1 Reverse Mathematics
Reverse Mathematics (RM hereafter) is a program in the foundations of mathematics initiated around 1975 by Friedman [Reference Friedman28, Reference Friedman29] and developed extensively by Simpson [Reference Simpson89]. The aim of RM is to identify the minimal axioms needed to prove theorems of ordinary, i.e., non-set theoretical, mathematics.
We refer to [Reference Stillwell91] for a basic introduction to RM and to [Reference Dzhafarov and Mummert26, Reference Simpson88, Reference Simpson89] for an overview of RM. We expect basic familiarity with RM, in particular Kohlenbach’s higher-order RM [Reference Kohlenbach48] essential to this paper, including the base theory
![]() $\mathsf {RCA}_{0}^{\omega }$
. An extensive introduction can be found in, e.g., [Reference Normann and Sanders65, Reference Normann and Sanders67–Reference Normann and Sanders69]. All undefined notions may be found in [Reference Normann and Sanders68, Reference Normann and Sanders69], while we do point out here that we shall sometimes use common notations from the type theory. For instance, the natural numbers are type
$\mathsf {RCA}_{0}^{\omega }$
. An extensive introduction can be found in, e.g., [Reference Normann and Sanders65, Reference Normann and Sanders67–Reference Normann and Sanders69]. All undefined notions may be found in [Reference Normann and Sanders68, Reference Normann and Sanders69], while we do point out here that we shall sometimes use common notations from the type theory. For instance, the natural numbers are type
![]() $0$
objects, denoted
$0$
objects, denoted
![]() $n^{0}$
or
$n^{0}$
or
![]() $n\in {\mathbb N}$
. Similarly, elements of Baire space are type
$n\in {\mathbb N}$
. Similarly, elements of Baire space are type
![]() $1$
objects, denoted
$1$
objects, denoted
![]() $f\in {\mathbb N}^{{\mathbb N}}$
or
$f\in {\mathbb N}^{{\mathbb N}}$
or
![]() $f^{1}$
. Mappings from Baire space
$f^{1}$
. Mappings from Baire space
![]() ${\mathbb N}^{{\mathbb N}}$
to
${\mathbb N}^{{\mathbb N}}$
to
![]() ${\mathbb N}$
are denoted
${\mathbb N}$
are denoted
![]() $Y:{\mathbb N}^{{\mathbb N}}\rightarrow {\mathbb N}$
or
$Y:{\mathbb N}^{{\mathbb N}}\rightarrow {\mathbb N}$
or
![]() $Y^{2}$
.
$Y^{2}$
.
1.3.2 Some comprehension functionals
In second-order RM, the logical hardness of a theorem is measured via what fragment of the comprehension axiom is needed for a proof. For this reason, we introduce some axioms and functionals related to higher-order comprehension in this section. We are mostly dealing with conventional comprehension here, i.e., only parameters over
![]() ${\mathbb N}$
and
${\mathbb N}$
and
![]() ${\mathbb N}^{{\mathbb N}}$
are allowed in formula classes like
${\mathbb N}^{{\mathbb N}}$
are allowed in formula classes like
![]() $\Pi _{k}^{1}$
and
$\Pi _{k}^{1}$
and
![]() $\Sigma _{k}^{1}$
.
$\Sigma _{k}^{1}$
.
First of all, the functional
![]() $\varphi $
in
$\varphi $
in
![]() $(\exists ^{2})$
is also Kleene’s quantifier
$(\exists ^{2})$
is also Kleene’s quantifier
![]() $\exists ^{2}$
and is clearly discontinuous at
$\exists ^{2}$
and is clearly discontinuous at
![]() $f=11\dots $
in Cantor space:
$f=11\dots $
in Cantor space:
In fact,
![]() $(\exists ^{2})$
is equivalent to the existence of
$(\exists ^{2})$
is equivalent to the existence of
![]() $F:{\mathbb R}\rightarrow {\mathbb R}$
such that
$F:{\mathbb R}\rightarrow {\mathbb R}$
such that
![]() $F(x)=1$
if
$F(x)=1$
if
![]() $x>_{{\mathbb R}}0$
, and
$x>_{{\mathbb R}}0$
, and
![]() $0$
otherwise (see [Reference Kohlenbach48, Proposition 3.12]). Related to
$0$
otherwise (see [Reference Kohlenbach48, Proposition 3.12]). Related to
![]() $(\exists ^{2})$
, the functional
$(\exists ^{2})$
, the functional
![]() $\mu ^{2}$
in
$\mu ^{2}$
in
![]() $(\mu ^{2})$
is called Feferman’s
$(\mu ^{2})$
is called Feferman’s
![]() $\mu $
(see [Reference Avigad and Feferman2]) and may be found—with the same symbol—in Hilbert-Bernays’ Grundlagen [Reference Hilbert and Bernays35, Supplement IV]:
$\mu $
(see [Reference Avigad and Feferman2]) and may be found—with the same symbol—in Hilbert-Bernays’ Grundlagen [Reference Hilbert and Bernays35, Supplement IV]:
 $$\begin{align} (\exists \mu^{2})(\forall f^{1})\big[ (\exists n)(f(n)=0) \rightarrow [f(\mu(f))=0&\wedge (\forall i<\mu(f))(f(i)\ne 0) ]\\ & \wedge [ (\forall n)(f(n)\ne0)\rightarrow \mu(f)=0] \big]. \end{align}$$
$$\begin{align} (\exists \mu^{2})(\forall f^{1})\big[ (\exists n)(f(n)=0) \rightarrow [f(\mu(f))=0&\wedge (\forall i<\mu(f))(f(i)\ne 0) ]\\ & \wedge [ (\forall n)(f(n)\ne0)\rightarrow \mu(f)=0] \big]. \end{align}$$
We have
![]() $(\exists ^{2})\leftrightarrow (\mu ^{2})$
over
$(\exists ^{2})\leftrightarrow (\mu ^{2})$
over
![]() $\mathsf {RCA}_{0}^{\omega }$
(see [Reference Kohlenbach48, Section 3]) and
$\mathsf {RCA}_{0}^{\omega }$
(see [Reference Kohlenbach48, Section 3]) and
![]() $\mathsf {ACA}_{0}^{\omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\exists ^{2})$
proves the same sentences as
$\mathsf {ACA}_{0}^{\omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\exists ^{2})$
proves the same sentences as
![]() $\mathsf {ACA}_{0}$
by [Reference Hunter38, Theorem 2.5].
$\mathsf {ACA}_{0}$
by [Reference Hunter38, Theorem 2.5].
Secondly, the functional
![]() $\mathsf {S}^{2}$
in
$\mathsf {S}^{2}$
in
![]() $(\mathsf {S}^{2})$
is called the Suslin functional [Reference Kohlenbach48]:
$(\mathsf {S}^{2})$
is called the Suslin functional [Reference Kohlenbach48]:
The system
![]() $\Pi _{1}^{1}\text {-}\mathsf {CA}_{0}^{\omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\mathsf {S}^{2})$
proves the same
$\Pi _{1}^{1}\text {-}\mathsf {CA}_{0}^{\omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\mathsf {S}^{2})$
proves the same
![]() $\Pi _{3}^{1}$
-sentences as
$\Pi _{3}^{1}$
-sentences as
![]() $\Pi _{1}^{1}\text {-}\mathsf {CA}_{0}$
by [Reference Sakamoto and Yamazaki76, Theorem 2.2]. By definition, the Suslin functional
$\Pi _{1}^{1}\text {-}\mathsf {CA}_{0}$
by [Reference Sakamoto and Yamazaki76, Theorem 2.2]. By definition, the Suslin functional
![]() $\mathsf {S}^{2}$
can decide whether a
$\mathsf {S}^{2}$
can decide whether a
![]() $\Sigma _{1}^{1}$
-formula as in the left-hand side of
$\Sigma _{1}^{1}$
-formula as in the left-hand side of
![]() $(\mathsf {S}^{2})$
is true or false. We similarly define the functional
$(\mathsf {S}^{2})$
is true or false. We similarly define the functional
![]() $\mathsf {S}_{k}^{2}$
which decides the truth or falsity of
$\mathsf {S}_{k}^{2}$
which decides the truth or falsity of
![]() $\Sigma _{k}^{1}$
-formulas from
$\Sigma _{k}^{1}$
-formulas from
![]() $\mathsf {L}_{2}$
; we also define the system
$\mathsf {L}_{2}$
; we also define the system
![]() $\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
as
$\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
as
![]() $\mathsf {RCA}_{0}^{\omega }+(\mathsf {S}_{k}^{2})$
, where
$\mathsf {RCA}_{0}^{\omega }+(\mathsf {S}_{k}^{2})$
, where
![]() $(\mathsf {S}_{k}^{2})$
expresses that
$(\mathsf {S}_{k}^{2})$
expresses that
![]() $\mathsf {S}_{k}^{2}$
exists. We note that the operators
$\mathsf {S}_{k}^{2}$
exists. We note that the operators
![]() $\nu _{n}$
from [Reference Buchholz, Feferman, Pohlers and Sieg18, p. 129] are essentially
$\nu _{n}$
from [Reference Buchholz, Feferman, Pohlers and Sieg18, p. 129] are essentially
![]() $\mathsf {S}_{n}^{2}$
strengthened to return a witness (if existent) to the
$\mathsf {S}_{n}^{2}$
strengthened to return a witness (if existent) to the
![]() $\Sigma _{n}^{1}$
-formula at hand.
$\Sigma _{n}^{1}$
-formula at hand.
Thirdly, full second-order arithmetic
![]() $\mathsf {Z}_{2}$
is readily derived from
$\mathsf {Z}_{2}$
is readily derived from
![]() $\cup _{k}\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
, or from:
$\cup _{k}\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
, or from:
and we therefore define
![]() $\mathsf {Z}_{2}^{\Omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\exists ^{3})$
and
$\mathsf {Z}_{2}^{\Omega }\equiv \mathsf {RCA}_{0}^{\omega }+(\exists ^{3})$
and
![]() $\mathsf {Z}_{2}^\omega \equiv \cup _{k}\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
, which are conservative over
$\mathsf {Z}_{2}^\omega \equiv \cup _{k}\Pi _{k}^{1}\text {-}\mathsf {CA}_{0}^{\omega }$
, which are conservative over
![]() $\mathsf {Z}_{2}$
by [Reference Hunter38, Corollary 2.6]. Despite this close connection,
$\mathsf {Z}_{2}$
by [Reference Hunter38, Corollary 2.6]. Despite this close connection,
![]() $\mathsf {Z}_{2}^{\omega }$
and
$\mathsf {Z}_{2}^{\omega }$
and
![]() $\mathsf {Z}_{2}^{\Omega }$
can behave quite differently, as discussed in, e.g., [Reference Normann and Sanders65, Section 2.2]. The functional from
$\mathsf {Z}_{2}^{\Omega }$
can behave quite differently, as discussed in, e.g., [Reference Normann and Sanders65, Section 2.2]. The functional from
![]() $(\exists ^{3})$
is also called ‘
$(\exists ^{3})$
is also called ‘
![]() $\exists ^{3}$
’, and we use the same convention for other functionals.
$\exists ^{3}$
’, and we use the same convention for other functionals.
1.3.3 Some basic definitions
We introduce some definitions needed in the below, mostly stemming from mainstream mathematics. We note that subsets of
![]() ${\mathbb R}$
are given by their characteristic functions as in Definition 1.7, well-known from measure and probability theory.
${\mathbb R}$
are given by their characteristic functions as in Definition 1.7, well-known from measure and probability theory.
Zeroth of all, we make use the usual definition of (open) set, where
![]() $B(x, r)$
is the open ball with radius
$B(x, r)$
is the open ball with radius
![]() $r>0$
centred at
$r>0$
centred at
![]() $x\in {\mathbb R}$
.
$x\in {\mathbb R}$
.
Definition 1.7 (Sets)
-
• A subset
 $A\subset {\mathbb R}$
is given by its characteristic function
$A\subset {\mathbb R}$
is given by its characteristic function
 $F_{A}:{\mathbb R}\rightarrow \{0,1\}$
, i.e., we write
$F_{A}:{\mathbb R}\rightarrow \{0,1\}$
, i.e., we write
 $x\in A$
for
$x\in A$
for
 $ F_{A}(x)=1$
, for any
$ F_{A}(x)=1$
, for any
 $x\in {\mathbb R}$
.
$x\in {\mathbb R}$
. -
• A subset
 $O\subset {\mathbb R}$
is open in case
$O\subset {\mathbb R}$
is open in case
 $x\in O$
implies that there is
$x\in O$
implies that there is
 $k\in {\mathbb N}$
such that
$k\in {\mathbb N}$
such that
 $B(x, \frac {1}{2^{k}})\subset O$
.
$B(x, \frac {1}{2^{k}})\subset O$
. -
• A subset
 $C\subset {\mathbb R}$
is closed if the complement
$C\subset {\mathbb R}$
is closed if the complement
 ${\mathbb R}\setminus C$
is open.
${\mathbb R}\setminus C$
is open.
As discussed in Remark 2.7, the study of functions of bounded variation already gives rise to open sets that do not come with additional representation beyond Definition 1.7.
First of all, we shall study the following notions of weak continuity, all of which hark back to the days of Baire, Darboux, and Volterra [Reference Baire3, Reference Baire4, Reference Darboux22, Reference Volterra98].
Definition 1.8. For
![]() $f:[0,1]\rightarrow {\mathbb R}$
, we have the following definitions:
$f:[0,1]\rightarrow {\mathbb R}$
, we have the following definitions:
-
• f is upper semi-continuous at
 $x_{0}\in [0,1]$
if
$x_{0}\in [0,1]$
if
 $f(x_{0})\geq _{{\mathbb R}}\lim \sup _{x\rightarrow x_{0}} f(x)$
.
$f(x_{0})\geq _{{\mathbb R}}\lim \sup _{x\rightarrow x_{0}} f(x)$
. -
• f is lower semi-continuous at
 $x_{0}\in [0,1]$
if
$x_{0}\in [0,1]$
if
 $f(x_{0})\leq _{{\mathbb R}}\lim \inf _{x\rightarrow x_{0}} f(x)$
.
$f(x_{0})\leq _{{\mathbb R}}\lim \inf _{x\rightarrow x_{0}} f(x)$
. -
• f is quasi-continuous at
 $x_{0}\in [0, 1]$
if for
$x_{0}\in [0, 1]$
if for
 $ \epsilon> 0$
and an open neighbourhood U of
$ \epsilon> 0$
and an open neighbourhood U of
 $x_{0}$
, there is a non-empty open
$x_{0}$
, there is a non-empty open
 ${ G\subset U}$
with
${ G\subset U}$
with
 $(\forall x\in G) (|f(x_{0})-f(x)|<\varepsilon )$
.
$(\forall x\in G) (|f(x_{0})-f(x)|<\varepsilon )$
. -
•
 $f:{\mathbb R}\rightarrow {\mathbb R}$
symmetrically continuous at
$f:{\mathbb R}\rightarrow {\mathbb R}$
symmetrically continuous at
 $x\in {\mathbb R}$
if
$x\in {\mathbb R}$
if  $$\begin{align*}(\forall \varepsilon> 0)(\exists \delta>0)(\forall z\in {\mathbb R} )(|z|<\delta\rightarrow |f(x+z)-f(x-z)|<\varepsilon ). \end{align*}$$
$$\begin{align*}(\forall \varepsilon> 0)(\exists \delta>0)(\forall z\in {\mathbb R} )(|z|<\delta\rightarrow |f(x+z)-f(x-z)|<\varepsilon ). \end{align*}$$
-
• f is Baire 1 if it is the pointwise limit of a sequence of continuous functions.
-
• f is Baire
 $2$
if it is the pointwise limit of a sequence of Baire
$2$
if it is the pointwise limit of a sequence of Baire
 $1$
functions.
$1$
functions. -
• f is Baire 1
 $^{*}$
ifFootnote
3
there is a sequence of closed sets
$^{*}$
ifFootnote
3
there is a sequence of closed sets
 $(C_{n})_{n\in {\mathbb N}}$
such
$(C_{n})_{n\in {\mathbb N}}$
such
 $[0,1]=\cup _{n\in {\mathbb N}}C_{n}$
and
$[0,1]=\cup _{n\in {\mathbb N}}C_{n}$
and
 $f_{\upharpoonright C_{m}}$
is continuous for all
$f_{\upharpoonright C_{m}}$
is continuous for all
 $m\in {\mathbb N}$
.
$m\in {\mathbb N}$
.
The first two items are often abbreviated as ‘usco’ and ‘lsco’.
Secondly, we also need the notion of ‘intermediate value property’, also called the ‘Darboux property’ in light of Darboux’s work in [Reference Darboux22].
Definition 1.9 (Darboux property)
Let
![]() $f:[0,1]\rightarrow {\mathbb R}$
be given.
$f:[0,1]\rightarrow {\mathbb R}$
be given.
-
• A real
 $y\in {\mathbb R}$
is a left (resp. right) cluster value of f at
$y\in {\mathbb R}$
is a left (resp. right) cluster value of f at
 $x\in [0,1]$
if there is
$x\in [0,1]$
if there is
 $(x_{n})_{n\in {\mathbb N}}$
such that
$(x_{n})_{n\in {\mathbb N}}$
such that
 $y=\lim _{n\rightarrow \infty } f(x_{n})$
and
$y=\lim _{n\rightarrow \infty } f(x_{n})$
and
 $x=\lim _{n\rightarrow \infty }x_{n}$
and
$x=\lim _{n\rightarrow \infty }x_{n}$
and
 $(\forall n\in {\mathbb N})(x_{n}\leq x)$
(resp.
$(\forall n\in {\mathbb N})(x_{n}\leq x)$
(resp.
 $(\forall n\in {\mathbb N})(x_{n}\geq x)$
).
$(\forall n\in {\mathbb N})(x_{n}\geq x)$
). -
• A point
 $x\in [0,1]$
is a Darboux point of
$x\in [0,1]$
is a Darboux point of
 $f:[0,1]\rightarrow {\mathbb R}$
if for any
$f:[0,1]\rightarrow {\mathbb R}$
if for any
 $\delta>0$
and any left (resp. right) cluster value y of f at x and
$\delta>0$
and any left (resp. right) cluster value y of f at x and
 $z\in {\mathbb R}$
strictly between y and
$z\in {\mathbb R}$
strictly between y and
 $f(x)$
, there is
$f(x)$
, there is
 $w\in (x-\delta , x)$
(resp.
$w\in (x-\delta , x)$
(resp.
 $w\in ( x, x+\delta )$
) such that
$w\in ( x, x+\delta )$
) such that
 $f(w)=y$
.
$f(w)=y$
.
By definition, a point of continuity is also a Darboux point, but not vice versa.
Thirdly, we introduce the ‘usual’ definitions of countable set (Definitions 1.10 and 1.11).
Definition 1.10 (Enumerable sets of reals)
A set
![]() $A\subset {\mathbb R}$
is enumerable if there exists a sequence
$A\subset {\mathbb R}$
is enumerable if there exists a sequence
![]() $(x_{n})_{n\in {\mathbb N}}$
such that
$(x_{n})_{n\in {\mathbb N}}$
such that
![]() $(\forall x\in {\mathbb R})(x\in A\rightarrow (\exists n\in {\mathbb N})(x=_{{\mathbb R}}x_{n}))$
.
$(\forall x\in {\mathbb R})(x\in A\rightarrow (\exists n\in {\mathbb N})(x=_{{\mathbb R}}x_{n}))$
.
This definition reflects the RM-notion of ‘countable set’ from [Reference Simpson89, Theorem V.4.2]. We note that given
![]() $\mu ^{2}$
from Section 1.3.2, we may replace the final implication in Definition 1.10 by an equivalence.
$\mu ^{2}$
from Section 1.3.2, we may replace the final implication in Definition 1.10 by an equivalence.
Definition 1.11 (Countable subset of
 ${\mathbb R}$
)
${\mathbb R}$
)
A set
![]() $A\subset {\mathbb R}$
is countable if there exists
$A\subset {\mathbb R}$
is countable if there exists
![]() $Y:{\mathbb R}\rightarrow {\mathbb N}$
such that
$Y:{\mathbb R}\rightarrow {\mathbb N}$
such that
![]() $(\forall x, y\in A)(Y(x)=_{0}Y(y)\rightarrow x=_{{\mathbb R}}y)$
. The mapping
$(\forall x, y\in A)(Y(x)=_{0}Y(y)\rightarrow x=_{{\mathbb R}}y)$
. The mapping
![]() $Y:{\mathbb R}\rightarrow {\mathbb N}$
is called an injection from A to
$Y:{\mathbb R}\rightarrow {\mathbb N}$
is called an injection from A to
![]() ${\mathbb N}$
or injective on A. If
${\mathbb N}$
or injective on A. If
![]() $Y:{\mathbb R}\rightarrow {\mathbb N}$
is also surjective, i.e.,
$Y:{\mathbb R}\rightarrow {\mathbb N}$
is also surjective, i.e.,
![]() $(\forall n\in {\mathbb N})(\exists x\in A)(Y(x)=n)$
, we call A strongly countable.
$(\forall n\in {\mathbb N})(\exists x\in A)(Y(x)=n)$
, we call A strongly countable.
The first part of Definition 1.11 is from Kunen’s set theory textbook [Reference Kunen54, p. 63] and the second part is taken from Hrbacek–Jech’s set theory textbook [Reference Hrbacek and Jech36] (where the term ‘countable’ is used instead of ‘strongly countable’). For the rest of this paper, ‘strongly countable’ and ‘countable’ shall exclusively refer to Definition 1.11, except when explicitly stated otherwise.
Finally, the uncountability of
![]() ${\mathbb R}$
can be studied in numerous guises in higher-order RM. For instance, the following are from [Reference Normann and Sanders68, Reference Normann and Sanders69], where it is also shown that many extremely basic theorems imply these principles, while
${\mathbb R}$
can be studied in numerous guises in higher-order RM. For instance, the following are from [Reference Normann and Sanders68, Reference Normann and Sanders69], where it is also shown that many extremely basic theorems imply these principles, while
![]() $\mathsf {Z}_{2}^{\omega }$
cannot prove them.
$\mathsf {Z}_{2}^{\omega }$
cannot prove them.
-
• For a countable set
 $A\subset [0,1]$
, there is
$A\subset [0,1]$
, there is
 $y\in [0,1]\setminus A$
.
$y\in [0,1]\setminus A$
. -
•
 $\mathsf {NIN}$
: there is no injection from
$\mathsf {NIN}$
: there is no injection from
 $[0,1]$
to
$[0,1]$
to
 ${\mathbb N}$
.
${\mathbb N}$
. -
• For a strongly countable set
 $A\subset [0,1]$
, there is
$A\subset [0,1]$
, there is
 $y\in [0,1]\setminus A$
.
$y\in [0,1]\setminus A$
. -
•
 $\mathsf {NBI}$
: there is no bijection from
$\mathsf {NBI}$
: there is no bijection from
 $[0,1]$
to
$[0,1]$
to
 ${\mathbb N}$
.
${\mathbb N}$
.
The reader will verify that the first two and last two items are (trivially) equivalent. Besides these and similar variations in [Reference Sanders79], we have not been able to obtain elegant or natural equivalences involving the uncountability of
![]() ${\mathbb R}$
try as we might. As discussed in Section 2, this is because the above items are formulated using the ‘set theoretic’ definition of countability as in Definition 1.11. In Section 3, we obtain many equivalences involving the uncountability of
${\mathbb R}$
try as we might. As discussed in Section 2, this is because the above items are formulated using the ‘set theoretic’ definition of countability as in Definition 1.11. In Section 3, we obtain many equivalences involving the uncountability of
![]() ${\mathbb R}$
, based on the alternative (but equivalent over
${\mathbb R}$
, based on the alternative (but equivalent over
![]() $\mathsf {ZF}$
) notion of ‘height countable’ introduced in Section 1.1.
$\mathsf {ZF}$
) notion of ‘height countable’ introduced in Section 1.1.
1.3.4 Some advanced definitions: bounded variation and around
We formulate the definitions of bounded variation and regulated functions, and some background.
Firstly, the notion of bounded variation (often abbreviated
![]() $BV$
below) was first explicitlyFootnote
4
introduced by Jordan around 1881 [Reference Jordan42] yielding a generalisation of Dirichlet’s convergence theorems for Fourier series. Indeed, Dirichlet’s convergence results are restricted to functions that are continuous except at a finite number of points, while
$BV$
below) was first explicitlyFootnote
4
introduced by Jordan around 1881 [Reference Jordan42] yielding a generalisation of Dirichlet’s convergence theorems for Fourier series. Indeed, Dirichlet’s convergence results are restricted to functions that are continuous except at a finite number of points, while
![]() $BV$
-functions can have infinitely many points of discontinuity, as already studied by Jordan, namely in [Reference Jordan42, p. 230]. Nowadays, the total variation of a function
$BV$
-functions can have infinitely many points of discontinuity, as already studied by Jordan, namely in [Reference Jordan42, p. 230]. Nowadays, the total variation of a function
![]() $f:[a, b]\rightarrow {\mathbb R}$
is defined as follows:
$f:[a, b]\rightarrow {\mathbb R}$
is defined as follows:
If this quantity exists and is finite, one says that f has bounded variation on
![]() $[a,b]$
. Now, the notion of bounded variation is defined in [Reference Nies, Triplett and Yokoyama64] without mentioning the supremum in (1.2); this approach can also be found in [Reference Bridges11, Reference Bridges and Mahalanobis12, Reference Kreuzer53]. Hence, we shall distinguish between the two notions in Definition 1.12. As it happens, Jordan seems to use item (a) of Definition 1.12 in [Reference Jordan42, pp. 228–229]. This definition suggests a twofold variation for any result on functions of bounded variation, namely depending on whether the supremum (1.2) is given, or only an upper bound on the latter.
$[a,b]$
. Now, the notion of bounded variation is defined in [Reference Nies, Triplett and Yokoyama64] without mentioning the supremum in (1.2); this approach can also be found in [Reference Bridges11, Reference Bridges and Mahalanobis12, Reference Kreuzer53]. Hence, we shall distinguish between the two notions in Definition 1.12. As it happens, Jordan seems to use item (a) of Definition 1.12 in [Reference Jordan42, pp. 228–229]. This definition suggests a twofold variation for any result on functions of bounded variation, namely depending on whether the supremum (1.2) is given, or only an upper bound on the latter.
Definition 1.12 (Variations on variation)
-
(a) The function
 $f:[a,b]\rightarrow {\mathbb R}$
has bounded variation on
$f:[a,b]\rightarrow {\mathbb R}$
has bounded variation on
 $[a,b]$
if there is
$[a,b]$
if there is
 $k_{0}\in {\mathbb N}$
such that
$k_{0}\in {\mathbb N}$
such that
 $k_{0}\geq \sum _{i=0}^{n} |f(x_{i})-f(x_{i+1})|$
for any partition
$k_{0}\geq \sum _{i=0}^{n} |f(x_{i})-f(x_{i+1})|$
for any partition
 $x_{0}=a <x_{1}< \dots < x_{n-1}<x_{n}=b $
.
$x_{0}=a <x_{1}< \dots < x_{n-1}<x_{n}=b $
. -
(b) The function
 $f:[a,b]\rightarrow {\mathbb R}$
has a variation on
$f:[a,b]\rightarrow {\mathbb R}$
has a variation on
 $[a,b]$
if the supremum in (1.2) exists and is finite.
$[a,b]$
if the supremum in (1.2) exists and is finite.
Secondly, the fundamental theorem about
![]() $BV$
-functions is as follows; this theorem is proved by Jordan in [Reference Jordan42].
$BV$
-functions is as follows; this theorem is proved by Jordan in [Reference Jordan42].
Theorem 1.13 (Jordan decomposition theorem [Reference Jordan42, p. 229])
A
![]() $BV$
-function
$BV$
-function
![]() $f : [0, 1] \rightarrow {\mathbb R}$
is the difference of two non-decreasing functions
$f : [0, 1] \rightarrow {\mathbb R}$
is the difference of two non-decreasing functions
![]() $g, h:[0,1]\rightarrow {\mathbb R}$
.
$g, h:[0,1]\rightarrow {\mathbb R}$
.
Theorem 1.13 has been studied via second-order representations in [Reference Greenberg, Miller and Nies31, Reference Kreuzer53, Reference Nies, Triplett and Yokoyama64, Reference Zheng and Rettinger105]. The same holds for constructive analysis by [Reference Bridges11, Reference Bridges and Mahalanobis12, Reference Heyting33, Reference Richman73], involving different (but related) constructive enrichments. Now,
![]() $\mathsf {ACA}_{0}$
suffices to derive Theorem 1.13 for various kinds of second-order representations of
$\mathsf {ACA}_{0}$
suffices to derive Theorem 1.13 for various kinds of second-order representations of
![]() $BV$
-functions in [Reference Kreuzer53, Reference Nies, Triplett and Yokoyama64]. By contrast, our results in [Reference Normann and Sanders68] imply that the third-order version of Theorem 1.13 is hard to prove in terms of conventional comprehension.
$BV$
-functions in [Reference Kreuzer53, Reference Nies, Triplett and Yokoyama64]. By contrast, our results in [Reference Normann and Sanders68] imply that the third-order version of Theorem 1.13 is hard to prove in terms of conventional comprehension.
Thirdly, Jordan proves in [Reference Jordan43, Section 105] that
![]() $BV$
-functions are exactly those for which the notion of ‘length of the graph of the function’ makes sense. In particular,
$BV$
-functions are exactly those for which the notion of ‘length of the graph of the function’ makes sense. In particular,
![]() $f\in BV$
if and only if the ‘length of the graph of f’, defined as follows:
$f\in BV$
if and only if the ‘length of the graph of f’, defined as follows:
exists and is finite by [Reference Appell, Bana’s and Merentes1, Theorem 3.28(c)]. In case the supremum in (1.3) exists (and is finite), f is also called rectifiable. Rectifiable curves predate
![]() $BV$
-functions: in [Reference Scheeffer84, Section 1 and 2], it is claimed that (1.3) is essentially equivalent to Duhamel’s 1866 approach from [Reference Dunham25, Chapter VI]. Around 1833, Dirksen, the PhD supervisor of Jacobi and Heine, already provides a definition of arc length that is (very) similar to (1.3) (see [Reference Dirksen24, Section 2, p. 128], but with some conceptual problems as discussed in [Reference Coolidge20, Section 3].
$BV$
-functions: in [Reference Scheeffer84, Section 1 and 2], it is claimed that (1.3) is essentially equivalent to Duhamel’s 1866 approach from [Reference Dunham25, Chapter VI]. Around 1833, Dirksen, the PhD supervisor of Jacobi and Heine, already provides a definition of arc length that is (very) similar to (1.3) (see [Reference Dirksen24, Section 2, p. 128], but with some conceptual problems as discussed in [Reference Coolidge20, Section 3].
Fourth, a function is regulated (called ‘regular’ in [Reference Appell, Bana’s and Merentes1]) if for every
![]() $x_{0}$
in the domain, the ‘left’ and ‘right’ limits
$x_{0}$
in the domain, the ‘left’ and ‘right’ limits
![]() $f(x_{0}-)=\lim _{x\rightarrow x_{0}-}f(x)$
and
$f(x_{0}-)=\lim _{x\rightarrow x_{0}-}f(x)$
and
![]() $f(x_{0}+)=\lim _{x\rightarrow x_{0}+}f(x)$
exist. Scheeffer studies discontinuous regulated functions in [Reference Scheeffer84] (without using the term ‘regulated’), while Bourbaki develops Riemann integration based on regulated functions in [Reference Bourbaki9]. We note that
$f(x_{0}+)=\lim _{x\rightarrow x_{0}+}f(x)$
exist. Scheeffer studies discontinuous regulated functions in [Reference Scheeffer84] (without using the term ‘regulated’), while Bourbaki develops Riemann integration based on regulated functions in [Reference Bourbaki9]. We note that
![]() $BV$
-functions are regulated, while Weierstrass’ ‘monster’ function is a natural example of a regulated function not in
$BV$
-functions are regulated, while Weierstrass’ ‘monster’ function is a natural example of a regulated function not in
![]() $BV$
.
$BV$
.
Finally, an interesting observation about regulated functions is as follows.
Remark 1.14 (Continuity and regulatedness)
First of all, as discussed in [Reference Kohlenbach48, Section 3], the local equivalence for functions on Baire space between sequential and ‘epsilon-delta’ continuity cannot be proved in
![]() $\mathsf {ZF}$
. By [Reference Normann and Sanders68, Theorem 3.32], this equivalence for regulated functions is provable in
$\mathsf {ZF}$
. By [Reference Normann and Sanders68, Theorem 3.32], this equivalence for regulated functions is provable in
![]() $\mathsf {ZF}$
(and actually just
$\mathsf {ZF}$
(and actually just
![]() $\mathsf {ACA}_{0}^{\omega }$
).
$\mathsf {ACA}_{0}^{\omega }$
).
Secondly,
![]() $\mu ^{2}$
readily computes the left and right limits of regulated
$\mu ^{2}$
readily computes the left and right limits of regulated
![]() $f:[0,1]\rightarrow {\mathbb R}$
. In this way, the formula ‘f is continuous at
$f:[0,1]\rightarrow {\mathbb R}$
. In this way, the formula ‘f is continuous at
![]() $x\in [0,1]$
’ is decidable using
$x\in [0,1]$
’ is decidable using
![]() $\mu ^{2}$
, namely equivalent to the formula ‘
$\mu ^{2}$
, namely equivalent to the formula ‘
![]() $f(x+)=f(x)=f(x-)$
’. The usual ‘epsilon-delta’ definition of continuity involves quantifiers over
$f(x+)=f(x)=f(x-)$
’. The usual ‘epsilon-delta’ definition of continuity involves quantifiers over
![]() ${\mathbb R}$
, i.e., the previous equality is much simpler and more elementary.
${\mathbb R}$
, i.e., the previous equality is much simpler and more elementary.
By the previous remark, the basic notions needed for the study of regulated and
![]() $BV$
-functions make sense in
$BV$
-functions make sense in
![]() $\mathsf {ACA}_{0}^{\omega }$
.
$\mathsf {ACA}_{0}^{\omega }$
.
2 Countability by any other name
We show that the ‘standard’ set-theoretic definitions of countability-from Section 1.3.3 and based on injections and bijections to
![]() ${\mathbb N}$
-are not suitable for the RM-study of regulated functions (see Section 2.1) and
${\mathbb N}$
-are not suitable for the RM-study of regulated functions (see Section 2.1) and
![]() $BV$
-functions (Section 2.2). We also formulate an alternative-more suitable for RM-notion of countability (Definitions 2.3 and 2.6), which amounts to ‘unions over
$BV$
-functions (Section 2.2). We also formulate an alternative-more suitable for RM-notion of countability (Definitions 2.3 and 2.6), which amounts to ‘unions over
![]() ${\mathbb N}$
of finite sets’ and which can also be found in the mathematical literature. This kind of ‘shift of definition’ has historical precedent as follows.
${\mathbb N}$
of finite sets’ and which can also be found in the mathematical literature. This kind of ‘shift of definition’ has historical precedent as follows.
Remark 2.1. First of all, the correct choice of definition for a given mathematical notion is crucial to the development of RM, as can be gleaned from the following quote from [Reference Brown and Simpson15, p. 129].
Under the old definition [of real number from [Reference Simpson87]], it would be consistent with
![]() $\mathsf {RCA}_{0}$
that there exists a sequence of real numbers
$\mathsf {RCA}_{0}$
that there exists a sequence of real numbers
![]() $(x_{n})_{n\in {\mathbb N}}$
such that
$(x_{n})_{n\in {\mathbb N}}$
such that
![]() $(x_{n}+\pi )_{n\in {\mathbb N}}$
is not a sequence of real numbers. We thank Ian Richards for pointing out this defect of the old definition. Our new definition [of real number from [Reference Brown and Simpson15]], given above, is adopted in order to remove this defect. All of the arguments and results of [Reference Simpson87] remain correct under the new definition.
$(x_{n}+\pi )_{n\in {\mathbb N}}$
is not a sequence of real numbers. We thank Ian Richards for pointing out this defect of the old definition. Our new definition [of real number from [Reference Brown and Simpson15]], given above, is adopted in order to remove this defect. All of the arguments and results of [Reference Simpson87] remain correct under the new definition.
In short, the early definition of ‘real number’ from [Reference Simpson87] was not suitable for the development of RM, highlighting the importance of the ‘right’ choice of definition.
Secondly, we stress that RM is not unique in this regard: the early definition of ‘continuous function’ in Bishop’s constructive analysis [Reference Bishop7] was also deemed problematic and changed to a new definition to be found in [Reference Bridges13]; the (substantial) problems with both definitions are discussed in some detail in [Reference Bridges14, Reference Waaldijk99], including elementary properties such as the concatenation of continuous functions and the continuity of
![]() $\frac {1}{x}$
for
$\frac {1}{x}$
for
![]() $x>0$
.
$x>0$
.
In short, the development of mathematics in logical systems with ‘restricted’ resources, like RM or constructive mathematics, seems to hinge on the ‘right’ choice of definition. In this section, we argue that the ‘right’ definition of countability for higher-order RM is given by height functions as in Section 1.1. To be absolutely clear, the background theory for this section is
![]() $\mathsf {ZFC}$
, i.e., a statement like ‘
$\mathsf {ZFC}$
, i.e., a statement like ‘
![]() $A\subset {\mathbb R}$
is countable’ means that the latter is provable in the former; most arguments (should) go through in
$A\subset {\mathbb R}$
is countable’ means that the latter is provable in the former; most arguments (should) go through in
![]() $\mathsf {Z}_{2}^{\Omega }$
.
$\mathsf {Z}_{2}^{\Omega }$
.
2.1 Regulated functions and countability
As suggested in Section 1.1, the set-theoretic definition of countable set is not suitable for the RM-study of regulated functions. We first provide some motivation for this claim in Remark 2.2. Inspired by the latter, we can then present our alternative notion in Definition 2.3, which amounts to ‘unions over
![]() ${\mathbb N}$
of finite sets’.
${\mathbb N}$
of finite sets’.
Remark 2.2 (Countable sets by any other name)
First of all, we have previously investigated the RM of regulated functions in [Reference Normann and Sanders68]. As part of this study, the following sets—definable via
![]() $\exists ^{2}$
—present themselves, where
$\exists ^{2}$
—present themselves, where
![]() $f:[0,1]\rightarrow {\mathbb R}$
is regulated:
$f:[0,1]\rightarrow {\mathbb R}$
is regulated:
 $$ \begin{align}\textstyle &A:= \big\{x\in (0,1): f(x+)\ne f(x) \vee f(x-)\ne f(x)\big\}, \notag \\ &A_{n}:=\big\{x\in (0,1): |f(x+)- f(x)|>\tfrac1{2^{n}} \vee |f(x-)- f(x)|>\tfrac1{2^{n}}\big\}. \end{align} $$
$$ \begin{align}\textstyle &A:= \big\{x\in (0,1): f(x+)\ne f(x) \vee f(x-)\ne f(x)\big\}, \notag \\ &A_{n}:=\big\{x\in (0,1): |f(x+)- f(x)|>\tfrac1{2^{n}} \vee |f(x-)- f(x)|>\tfrac1{2^{n}}\big\}. \end{align} $$
Clearly,
![]() $A=\cup _{n\in {\mathbb N}}A_{n}$
collects all points in
$A=\cup _{n\in {\mathbb N}}A_{n}$
collects all points in
![]() $(0,1)$
where f is discontinuous; this set is central to many proofs involving regulated functions (see, e.g., [Reference Appell, Bana’s and Merentes1, Theorem 0.36]). Now, that
$(0,1)$
where f is discontinuous; this set is central to many proofs involving regulated functions (see, e.g., [Reference Appell, Bana’s and Merentes1, Theorem 0.36]). Now, that
![]() $A_{n}$
is finite follows by a standardFootnote
5
compactness argument. However, while A is then countable, we are unable to construct an injection from A to
$A_{n}$
is finite follows by a standardFootnote
5
compactness argument. However, while A is then countable, we are unable to construct an injection from A to
![]() ${\mathbb N}$
(let alone a bijection), even working in
${\mathbb N}$
(let alone a bijection), even working in
![]() $\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7 for details).
$\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7 for details).
In short, one readily finds countable sets ‘in the wild’, namely pertaining to regulated functions, for which the associated injections to
![]() ${\mathbb N}$
cannot be constructed in reasonably weak logical systems.
${\mathbb N}$
cannot be constructed in reasonably weak logical systems.
Secondly, in light of (2.1), regulated functions give rise to countable sets given only as the union over
![]() ${\mathbb N}$
of finite sets (i.e., without information about an injection to
${\mathbb N}$
of finite sets (i.e., without information about an injection to
![]() ${\mathbb N}$
). To see that the ‘reverse’ is also true, consider the following function:
${\mathbb N}$
). To see that the ‘reverse’ is also true, consider the following function:
 $$ \begin{align} h(x):= \begin{cases} 0, & x\not \in \cup_{m\in {\mathbb N}}X_{m}, \\ \frac{1}{2^{n+1}}, & x\in X_{n} \text{ and } n \text{ is the least such number,} \end{cases} \end{align} $$
$$ \begin{align} h(x):= \begin{cases} 0, & x\not \in \cup_{m\in {\mathbb N}}X_{m}, \\ \frac{1}{2^{n+1}}, & x\in X_{n} \text{ and } n \text{ is the least such number,} \end{cases} \end{align} $$
where
![]() $(X_{n})_{n\in {\mathbb N}}$
is a sequence of finite sets in
$(X_{n})_{n\in {\mathbb N}}$
is a sequence of finite sets in
![]() $[0,1]$
. One readily shows that h is regulated using
$[0,1]$
. One readily shows that h is regulated using
![]() $\exists ^{2}$
. For general closed sets, (2.2) is crucial to the study of Baire 1 functions (see [Reference Myerson63, p. 238]). Hence, regulated functions yield countable sets given (only) as unions over
$\exists ^{2}$
. For general closed sets, (2.2) is crucial to the study of Baire 1 functions (see [Reference Myerson63, p. 238]). Hence, regulated functions yield countable sets given (only) as unions over
![]() ${\mathbb N}$
of finite sets, namely via
${\mathbb N}$
of finite sets, namely via
![]() $A=\cup _{n\in {\mathbb N}}A_{n}$
from (2.1), and vice versa, namely via
$A=\cup _{n\in {\mathbb N}}A_{n}$
from (2.1), and vice versa, namely via
![]() $h:[0,1]\rightarrow {\mathbb R}$
as in (2.2).
$h:[0,1]\rightarrow {\mathbb R}$
as in (2.2).
In summary, we observe that the usual definition of countable set (involving injections/bijections to
![]() ${\mathbb N}$
) is not suitable for the RM-study of regulated functions. Luckily, (2.1) and (2.2) suggest an alternative approach via the fundamental connection between regulated functions on one hand, and countable sets given as
${\mathbb N}$
) is not suitable for the RM-study of regulated functions. Luckily, (2.1) and (2.2) suggest an alternative approach via the fundamental connection between regulated functions on one hand, and countable sets given as
on the other hand. In conclusion, the RM-study of regulated functions should be based on the centred notion of countability and not injections/bijections to
![]() ${\mathbb N}$
.
${\mathbb N}$
.
Motivated by Remark 2.2, we introduce our alternative definition of countability, which is exactly the same as Definition 1.1 in Section 1.1.
Definition 2.3. A set
![]() $A\subset {\mathbb R}$
is height countable if there is a height function
$A\subset {\mathbb R}$
is height countable if there is a height function
![]() $H:{\mathbb R}\rightarrow {\mathbb N}$
for A, i.e., for all
$H:{\mathbb R}\rightarrow {\mathbb N}$
for A, i.e., for all
![]() $n\in {\mathbb N}$
,
$n\in {\mathbb N}$
,
![]() $A_{n}:= \{ x\in A: H(x)<n\}$
is finite.
$A_{n}:= \{ x\in A: H(x)<n\}$
is finite.
The previous notion of ‘height’ is mentioned in the context of countability in, e.g., [Reference Huxley40, Reference Kolmogorov and Fomin49, Reference Moll59, Reference Royden75, Reference Vatssa97]. Definition 2.3 amounts to ‘union over
![]() ${\mathbb N}$
of finite sets’, as is readily shown in
${\mathbb N}$
of finite sets’, as is readily shown in
![]() $\mathsf {ACA}_{0}^{\omega }$
.
$\mathsf {ACA}_{0}^{\omega }$
.
Finally, the observations from Remark 2.2 regarding countable sets also apply mutatis mutandis to finite sets. Indeed, finite as each
![]() $A_{n}$
from (2.1) may be, we are unable to construct an injection to a finite subset of
$A_{n}$
from (2.1) may be, we are unable to construct an injection to a finite subset of
![]() ${\mathbb N}$
, even assuming
${\mathbb N}$
, even assuming
![]() $\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7 for details). By contrast, the definition of finite set from Section 1.1 is more suitable: one readilyFootnote
6
shows that
$\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7 for details). By contrast, the definition of finite set from Section 1.1 is more suitable: one readilyFootnote
6
shows that
![]() $A_{n}$
from (2.1) is finite as in Definition 2.4, which is exactly the same as Definition 1.2 in Section 1.1.
$A_{n}$
from (2.1) is finite as in Definition 2.4, which is exactly the same as Definition 1.2 in Section 1.1.
Definition 2.4 (Finite set)
Any
![]() $X\subset {\mathbb R}$
is finite if there is
$X\subset {\mathbb R}$
is finite if there is
![]() $N\in {\mathbb N}$
such that for any finite sequence
$N\in {\mathbb N}$
such that for any finite sequence
![]() $(x_{0}, \dots , x_{N})$
of distinct reals, there is
$(x_{0}, \dots , x_{N})$
of distinct reals, there is
![]() $i\leq N$
such that
$i\leq N$
such that
![]() $x_{i}\not \in X$
.
$x_{i}\not \in X$
.
The number N from Definition 2.4 is call a size bound for the finite set
![]() $X\subset {\mathbb R}$
. Analogous to countable sets, the RM-study of regulated functions should be based on Definition 2.4 and not on the set-theoretic definition based on injections/bijections to finite subsets of
$X\subset {\mathbb R}$
. Analogous to countable sets, the RM-study of regulated functions should be based on Definition 2.4 and not on the set-theoretic definition based on injections/bijections to finite subsets of
![]() ${\mathbb N}$
or similar constructs.
${\mathbb N}$
or similar constructs.
2.2 Bounded variation functions and countability
We discuss the observations from Section 2.1 for the particular case of functions of bounded variation (which are regulated by Theorem 3.3). In particular, while the same observations apply, they have to be refined to yield elegant equivalences.
Remark 2.5 (Countable by another name)
First of all, we consider (2.1), but formulated for a
![]() $BV$
-function
$BV$
-function
![]() $g:[0,1]\rightarrow {\mathbb R}$
, as follows:
$g:[0,1]\rightarrow {\mathbb R}$
, as follows:
 $$ \begin{align}\textstyle &B:= \big\{x\in (0,1): g(x+)\ne g(x) \vee g(x-)\ne g(x)\big\}, \notag \\ &B_{n}:=\big\{x\in (0,1): |g(x+)- g(x)|>\tfrac1{2^{n}} \vee |g(x-)- g(x)|>\tfrac1{2^{n}}\big\}. \end{align} $$
$$ \begin{align}\textstyle &B:= \big\{x\in (0,1): g(x+)\ne g(x) \vee g(x-)\ne g(x)\big\}, \notag \\ &B_{n}:=\big\{x\in (0,1): |g(x+)- g(x)|>\tfrac1{2^{n}} \vee |g(x-)- g(x)|>\tfrac1{2^{n}}\big\}. \end{align} $$
Similar to
![]() $A=\cup _{n\in }A_{n}$
as in (2.3),
$A=\cup _{n\in }A_{n}$
as in (2.3),
![]() $B=\cup _{n\in {\mathbb N}}B_{n}$
collects all points in
$B=\cup _{n\in {\mathbb N}}B_{n}$
collects all points in
![]() $(0,1)$
where g is discontinuous and this set is central to many proofs involving
$(0,1)$
where g is discontinuous and this set is central to many proofs involving
![]() $BV$
-functions (see [Reference Appell, Bana’s and Merentes1]). Similar to A from (2.3), B is countable but we are unable to construct an injection from B to
$BV$
-functions (see [Reference Appell, Bana’s and Merentes1]). Similar to A from (2.3), B is countable but we are unable to construct an injection from B to
![]() ${\mathbb N}$
(let alone a bijection), even assuming
${\mathbb N}$
(let alone a bijection), even assuming
![]() $\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7).
$\mathsf {Z}_{2}^{\omega }$
(see Remark 2.7).
Secondly, there is a crucial difference between (2.1) and (2.3): we know that the set
![]() $B_{n}$
is finite and has at most
$B_{n}$
is finite and has at most
![]() $2^{n}V_{0}^{1}(f)$
elements; indeed, each element of
$2^{n}V_{0}^{1}(f)$
elements; indeed, each element of
![]() $B_{n}$
contributes at least
$B_{n}$
contributes at least
![]() $1/2^{n}$
to the total variation
$1/2^{n}$
to the total variation
![]() $V_{0}^{1}(f)$
as in (1.2). By contrast, we have no extra information about the size of
$V_{0}^{1}(f)$
as in (1.2). By contrast, we have no extra information about the size of
![]() $A_{n}$
from (2.1). However, this extra information is crucial if we wish to deal with
$A_{n}$
from (2.1). However, this extra information is crucial if we wish to deal with
![]() $BV$
-functions (only). Indeed, the function h from (2.2) is not in
$BV$
-functions (only). Indeed, the function h from (2.2) is not in
![]() $BV$
, e.g., in the trivial case where each
$BV$
, e.g., in the trivial case where each
![]() $X_{n}$
has at least
$X_{n}$
has at least
![]() $2^{n+1}$
elements. By contrast, consider the following nicer function:
$2^{n+1}$
elements. By contrast, consider the following nicer function:
 $$ \begin{align} k(x):= \begin{cases} 0, & x\not \in \cup_{m\in {\mathbb N}}Y_{m}, \\ \frac{1}{2^{n+1}} \frac{1}{g(n)+1},& x\in Y_{n} \text{ and } n \text{ is the least such number}, \end{cases} \end{align} $$
$$ \begin{align} k(x):= \begin{cases} 0, & x\not \in \cup_{m\in {\mathbb N}}Y_{m}, \\ \frac{1}{2^{n+1}} \frac{1}{g(n)+1},& x\in Y_{n} \text{ and } n \text{ is the least such number}, \end{cases} \end{align} $$
where
![]() $g\in {\mathbb N}^{{\mathbb N}}$
is a width function
Footnote
7
for
$g\in {\mathbb N}^{{\mathbb N}}$
is a width function
Footnote
7
for
![]() $(Y_{n})_{n\in {\mathbb N}}$
. One readily verifies that
$(Y_{n})_{n\in {\mathbb N}}$
. One readily verifies that
![]() $k:[0,1]\rightarrow {\mathbb R}$
is in
$k:[0,1]\rightarrow {\mathbb R}$
is in
![]() $BV$
with total variation bounded by
$BV$
with total variation bounded by
![]() $1$
. Hence,
$1$
. Hence,
![]() $BV$
-functions yield countable sets given (only) as in the following description:
$BV$
-functions yield countable sets given (only) as in the following description:
namely via
![]() $B=\cup _{n\in {\mathbb N}}B_{n}$
from (2.3), and vice versa, namely via
$B=\cup _{n\in {\mathbb N}}B_{n}$
from (2.3), and vice versa, namely via
![]() $k:[0,1]\rightarrow {\mathbb R}$
as in (2.4). The generalisations of bounded variation from Remark 3.23 have a similar property, as evidenced by the final part of the proof of Theorem 3.20.
$k:[0,1]\rightarrow {\mathbb R}$
as in (2.4). The generalisations of bounded variation from Remark 3.23 have a similar property, as evidenced by the final part of the proof of Theorem 3.20.
Motivated by Remark 2.5, we introduce our alternative (equivalent over
![]() $\mathsf {ZF}$
) definition of countability for the RM-study of
$\mathsf {ZF}$
) definition of countability for the RM-study of
![]() $BV$
-functions.
$BV$
-functions.
Definition 2.6. A set
![]() $B\subset {\mathbb R}$
is height–width countable if there is a height function
$B\subset {\mathbb R}$
is height–width countable if there is a height function
![]() $H:{\mathbb R}\rightarrow {\mathbb N}$
and width function
$H:{\mathbb R}\rightarrow {\mathbb N}$
and width function
![]() $g:{\mathbb N}\rightarrow {\mathbb N}$
, i.e., for all
$g:{\mathbb N}\rightarrow {\mathbb N}$
, i.e., for all
![]() $n\in {\mathbb N}$
, the set
$n\in {\mathbb N}$
, the set
![]() $B_{n}:= \{ x\in B: H(x)<n\}$
is finite with size bound
$B_{n}:= \{ x\in B: H(x)<n\}$
is finite with size bound
![]() $g(n)$
.
$g(n)$
.
Finally, the following technical remark makes the claims in Remarks 2.2 and 2.5 more precise in terms of logical systems.
Remark 2.7. As discussed above, the sets
![]() $A_{n}$
from (2.1) and
$A_{n}$
from (2.1) and
![]() $B_{n}$
from (2.3) are finite, while the unions
$B_{n}$
from (2.3) are finite, while the unions
![]() $A=\cup _{n\in {\mathbb N}}A_{n}$
and
$A=\cup _{n\in {\mathbb N}}A_{n}$
and
![]() $B=\cup _{n\in {\mathbb N}}B_{n}$
are countable. Hence, working in
$B=\cup _{n\in {\mathbb N}}B_{n}$
are countable. Hence, working in
![]() $\mathsf {ZF}$
(or even
$\mathsf {ZF}$
(or even
![]() $\mathsf {Z}_{2}^{\Omega }$
from Section 1.3.2), the following objects can be constructed:
$\mathsf {Z}_{2}^{\Omega }$
from Section 1.3.2), the following objects can be constructed:
-
• for
 $n\in {\mathbb N}$
, an injection
$n\in {\mathbb N}$
, an injection
 $Y_{n}$
from
$Y_{n}$
from
 $A_{n}$
to some
$A_{n}$
to some
 $\{0, 1, \dots , k\}$
with
$\{0, 1, \dots , k\}$
with
 $k\in {\mathbb N}$
,
$k\in {\mathbb N}$
, -
• for
 $m\in {\mathbb N}$
, an RM-code
$m\in {\mathbb N}$
, an RM-code
 $C_{m}$
(see [Reference Simpson89, II.5.6]) for the closed sets
$C_{m}$
(see [Reference Simpson89, II.5.6]) for the closed sets
 $A_{m}$
or
$A_{m}$
or
 $B_{m}$
.
$B_{m}$
.
However, it is shown in [Reference Sanders81, Reference Sanders82] that neither
![]() $Y_{n}$
nor
$Y_{n}$
nor
![]() $C_{n}$
are computable (in the sense of Kleene S1–S9; see [Reference Longley and Normann58]) in terms of any
$C_{n}$
are computable (in the sense of Kleene S1–S9; see [Reference Longley and Normann58]) in terms of any
![]() $\mathsf {S}_{m}^{2}$
and the other data. As a result, even
$\mathsf {S}_{m}^{2}$
and the other data. As a result, even
![]() $\mathsf {Z}_{2}^{\omega }$
cannot prove the general existence of
$\mathsf {Z}_{2}^{\omega }$
cannot prove the general existence of
![]() $Y_{n}$
and
$Y_{n}$
and
![]() $C_{n}$
as in the previous items. By contrast, the system
$C_{n}$
as in the previous items. By contrast, the system
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
(and even fragments) suffice to show that Definitions 2.3, 2.6, and 2.4 apply to
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
(and even fragments) suffice to show that Definitions 2.3, 2.6, and 2.4 apply to
![]() $A,A_{n}$
from (2.1) and
$A,A_{n}$
from (2.1) and
![]() $B,B_{n}$
from (2.3).
$B,B_{n}$
from (2.3).
In conclusion, we have introduced ‘new’—but equivalent over
![]() $\mathsf {ZF}$
and the weaker
$\mathsf {ZF}$
and the weaker
![]() $\mathsf {Z}_{2}^{\Omega }$
—definitions of finite and countable set with the following properties.
$\mathsf {Z}_{2}^{\Omega }$
—definitions of finite and countable set with the following properties.
-
• Our ‘new’ definitions capture the notion of finite and countable set as it occurs ‘in the wild’, namely in the study of
 $BV$
or regulated functions. This holds over relatively weak systems by Remark 2.7.
$BV$
or regulated functions. This holds over relatively weak systems by Remark 2.7. -
• One finds our ‘new’ definitions, in particular the notion of ‘height’, in the literature (see [Reference Huxley40, Reference Kolmogorov and Fomin49, Reference Moll59, Reference Royden75, Reference Vatssa97]).
-
• These ‘new’ definitions shall be seen to yield many equivalences in the RM of the uncountability of
 ${\mathbb R}$
(Sections 3.3 and 3.4).
${\mathbb R}$
(Sections 3.3 and 3.4).
We believe that the previous items justify our adoption of our ‘new’ definitions of finite and countable set. Moreover, Remark 2.1 creates some historical precedent based on second-order RM and constructive mathematics.
3 Main results: regulated and
 $BV$
-functions
$BV$
-functions
3.1 Introduction
In this section, we establish the equivalences sketched in Section 1.1 pertaining to the uncountability of
![]() ${\mathbb R}$
and properties of regulated functions (Section 3.3) and
${\mathbb R}$
and properties of regulated functions (Section 3.3) and
![]() $BV$
-functions (Section 3.4). In Section 3.2, we establish some basic properties of
$BV$
-functions (Section 3.4). In Section 3.2, we establish some basic properties of
![]() $BV$
and regulated functions in weak systems.
$BV$
and regulated functions in weak systems.
As noted in Section 1.1, we shall show that the uncountability of
![]() ${\mathbb R}$
as in
${\mathbb R}$
as in
![]() $\mathsf {NIN}_{\mathsf {alt}}$
is robust, i.e., equivalent to small perturbations of itself [Reference Montalbán60, p. 432]. Striking examples of this claimed robustness may be found in Theorem 3.7, where the perturbations are given by considering either one point of continuity, a dense set of such points, or various uncountability criteria for
$\mathsf {NIN}_{\mathsf {alt}}$
is robust, i.e., equivalent to small perturbations of itself [Reference Montalbán60, p. 432]. Striking examples of this claimed robustness may be found in Theorem 3.7, where the perturbations are given by considering either one point of continuity, a dense set of such points, or various uncountability criteria for
![]() $C_{f}$
, the set of continuity points.
$C_{f}$
, the set of continuity points.
Finally, the content of Section 3.5 is explained in the following remark.
Remark 3.1 (When more is less)
As noted in Section 1.1,
![]() $\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to well-known theorems from analysis restricted to regulated functions, with similar results for
$\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to well-known theorems from analysis restricted to regulated functions, with similar results for
![]() $BV$
-functions. These (restricted) theorems thus imply
$BV$
-functions. These (restricted) theorems thus imply
![]() $\mathsf {NIN}$
and are not provable in
$\mathsf {NIN}$
and are not provable in
![]() $\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
as a result (see [Reference Normann and Sanders69]). We show in Section 3.5 that adding the extra condition ‘Baire 1’ to these theorems makes them provable from (essentially) arithmetical comprehension. While regulated and
$\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
as a result (see [Reference Normann and Sanders69]). We show in Section 3.5 that adding the extra condition ‘Baire 1’ to these theorems makes them provable from (essentially) arithmetical comprehension. While regulated and
![]() $BV$
-functions are Baire 1, say over
$BV$
-functions are Baire 1, say over
![]() $\mathsf {ZF}$
or
$\mathsf {ZF}$
or
![]() $\mathsf {Z}_{2}^{\Omega }$
, there is no contradiction here as the statement
$\mathsf {Z}_{2}^{\Omega }$
, there is no contradiction here as the statement
already implies
![]() $\mathsf {NIN}$
by [Reference Normann and Sanders72, Theorem 2.34]. We stress that ‘Baire 1’ is special in this regard: other restrictions, e.g., involving semi-continuity or Baire 2, yield theorems that are still equivalent to
$\mathsf {NIN}$
by [Reference Normann and Sanders72, Theorem 2.34]. We stress that ‘Baire 1’ is special in this regard: other restrictions, e.g., involving semi-continuity or Baire 2, yield theorems that are still equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, as shown in Section 3.3.3. We have no explanation for this phenomenon.
$\mathsf {NIN}_{\mathsf {alt}}$
, as shown in Section 3.3.3. We have no explanation for this phenomenon.
3.2 Preliminary results
We collect some preliminary results pertaining to regulated and
![]() $BV$
-functions, and
$BV$
-functions, and
![]() $\mathsf {NIN}_{\mathsf {alt}}$
from Section 1.1.
$\mathsf {NIN}_{\mathsf {alt}}$
from Section 1.1.
First of all, to allow for a smooth treatment of finite sets, we shall adopt the following principle that collects the most basic properties of finite sets.
Principle 3.2 (
 $\mathsf {FIN}$
)
$\mathsf {FIN}$
)
-
• Finite union theorem: for a sequence of finite sets
 $(X_{n})_{n\in {\mathbb N}}$
and any
$(X_{n})_{n\in {\mathbb N}}$
and any
 $k\in {\mathbb N}$
,
$k\in {\mathbb N}$
,
 $\cup _{n\leq k}X_{n}$
is finite.
$\cup _{n\leq k}X_{n}$
is finite. -
• For any finite
 $X\subset {\mathbb R}$
, there is a finite sequence of reals
$X\subset {\mathbb R}$
, there is a finite sequence of reals
 $(x_{0}, \dots , x_{k})$
that includes all elements of X.
$(x_{0}, \dots , x_{k})$
that includes all elements of X. -
• Finite Axiom of Choice: for
 $Y^{2}, k^{0}$
with
$Y^{2}, k^{0}$
with
 $(\forall n\leq k)(\exists f\in 2^{{\mathbb N}})(Y(f, n)=0)$
, there is a finite sequence
$(\forall n\leq k)(\exists f\in 2^{{\mathbb N}})(Y(f, n)=0)$
, there is a finite sequence
 $(f_{0}, \dots , f_{k})$
in
$(f_{0}, \dots , f_{k})$
in
 $2^{{\mathbb N}}$
with
$2^{{\mathbb N}}$
with
 $(\forall n\leq k)(Y(f_{n}, n)=0)$
.
$(\forall n\leq k)(Y(f_{n}, n)=0)$
.
One can readily derive
![]() $\mathsf {FIN}$
from a sufficiently general fragment of the induction axiom; the RM of the latter is well-known (see, e.g., [Reference Simpson89, X.4.4]) and the RM of (fragments of)
$\mathsf {FIN}$
from a sufficiently general fragment of the induction axiom; the RM of the latter is well-known (see, e.g., [Reference Simpson89, X.4.4]) and the RM of (fragments of)
![]() $\mathsf {FIN}$
is therefore a matter of future research. We note that in [Reference Normann and Sanders68], we could derive (fragments of)
$\mathsf {FIN}$
is therefore a matter of future research. We note that in [Reference Normann and Sanders68], we could derive (fragments of)
![]() $\mathsf {FIN}$
from the principles under study, like the fact that (height) countable sets of reals can be enumerated. Hence, we could mostly avoid the use of fragments of
$\mathsf {FIN}$
from the principles under study, like the fact that (height) countable sets of reals can be enumerated. Hence, we could mostly avoid the use of fragments of
![]() $\mathsf {FIN}$
in the base theory in [Reference Normann and Sanders68], which does not seem possible for this paper.
$\mathsf {FIN}$
in the base theory in [Reference Normann and Sanders68], which does not seem possible for this paper.
Secondly, we need some some basic properties of
![]() $BV$
and regulated functions, all of which have been established in [Reference Normann and Sanders68] already.
$BV$
and regulated functions, all of which have been established in [Reference Normann and Sanders68] already.
Theorem 3.3 (
 $\mathsf {ACA}_{0}^{\omega }$
)
$\mathsf {ACA}_{0}^{\omega }$
)
-
• Assuming
 $\mathsf {FIN}$
, any
$\mathsf {FIN}$
, any
 $BV$
-function
$BV$
-function
 $f:[0,1]\rightarrow {\mathbb R}$
is regulated.
$f:[0,1]\rightarrow {\mathbb R}$
is regulated. -
• Any monotone function
 $f:[0,1]\rightarrow {\mathbb R}$
has bounded variation.
$f:[0,1]\rightarrow {\mathbb R}$
has bounded variation. -
• For any monotone function
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a sequence
$f:[0,1]\rightarrow {\mathbb R}$
, there is a sequence
 $(x_{n})_{n\in {\mathbb N}}$
that enumerates all
$(x_{n})_{n\in {\mathbb N}}$
that enumerates all
 $x\in [0,1]$
such that f is discontinuous at x.
$x\in [0,1]$
such that f is discontinuous at x. -
• For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
and
$f:[0,1]\rightarrow {\mathbb R}$
and
 $x\in [0,1]$
, f is sequentially continuous at x if and only if f is epsilon-delta continuous at x.
$x\in [0,1]$
, f is sequentially continuous at x if and only if f is epsilon-delta continuous at x. -
• For finite
 $X\subset [0,1]$
, the function
$X\subset [0,1]$
, the function
 $\mathbb {1}_{X}$
has bounded variation.
$\mathbb {1}_{X}$
has bounded variation.
Proof Proofs may be found in [Reference Normann and Sanders68, Section 3.3].
The fourth item of Theorem 3.3 is particularly interesting as the local equivalence between sequential and epsilon-delta continuity for general
![]() ${\mathbb R}\rightarrow {\mathbb R}$
-functions is not provable in
${\mathbb R}\rightarrow {\mathbb R}$
-functions is not provable in
![]() $\mathsf {ZF}$
, while
$\mathsf {ZF}$
, while
![]() $\mathsf {RCA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
suffices, as discussed in Remark 1.14.
$\mathsf {RCA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
suffices, as discussed in Remark 1.14.
Thirdly, we discuss some ‘obvious’ equivalences for
![]() $\mathsf {NIN}$
and
$\mathsf {NIN}$
and
![]() $\mathsf {NBI}$
.
$\mathsf {NBI}$
.
Remark 3.4. Now,
![]() $\mathsf {NIN}$
and
$\mathsf {NIN}$
and
![]() $\mathsf {NBI}$
are formulated for mappings from
$\mathsf {NBI}$
are formulated for mappings from
![]() $[0,1]$
to
$[0,1]$
to
![]() ${\mathbb N}$
, but we can equivalently replace the unit interval by, e.g.,
${\mathbb N}$
, but we can equivalently replace the unit interval by, e.g.,
![]() ${\mathbb R}$
,
${\mathbb R}$
,
![]() $2^{{\mathbb N}}$
, and
$2^{{\mathbb N}}$
, and
![]() ${\mathbb N}^{{\mathbb N}}$
, as shown in [Reference Sanders79, Section 2.1]. An important observation in this context, and readily formalised in
${\mathbb N}^{{\mathbb N}}$
, as shown in [Reference Sanders79, Section 2.1]. An important observation in this context, and readily formalised in
![]() $\mathsf {ACA}_{0}^{\omega }$
, is that the (rescaled) tangent function provides a bijection from any open interval to
$\mathsf {ACA}_{0}^{\omega }$
, is that the (rescaled) tangent function provides a bijection from any open interval to
![]() ${\mathbb R}$
; the inverse of tangent, called arctangent, yields a bijection in the other direction (also with rescaling). Moreover, using these bijections, one readily shows that
${\mathbb R}$
; the inverse of tangent, called arctangent, yields a bijection in the other direction (also with rescaling). Moreover, using these bijections, one readily shows that
![]() $\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to the following:
$\mathsf {NIN}_{\mathsf {alt}}$
is equivalent to the following:
-
• there is no height function from
 ${\mathbb R}$
to
${\mathbb R}$
to
 ${\mathbb N}$
.
${\mathbb N}$
.
Similarly, if we can show that there is no height function from some fixed open interval to
![]() ${\mathbb N}$
, then
${\mathbb N}$
, then
![]() $\mathsf {NIN}_{\mathsf {alt}}$
follows. We will tacitly make use of this fact in the proof of Theorems 3.6 and 3.7.
$\mathsf {NIN}_{\mathsf {alt}}$
follows. We will tacitly make use of this fact in the proof of Theorems 3.6 and 3.7.
Fourth, while we choose to use (at least) the system
![]() $\mathsf {ACA}_{0}^{\omega }$
as our base theory, one can replace the latter by
$\mathsf {ACA}_{0}^{\omega }$
as our base theory, one can replace the latter by
![]() $\mathsf {RCA}_{0}^{\omega }$
using the following trick.
$\mathsf {RCA}_{0}^{\omega }$
using the following trick.
Remark 3.5 (Excluded middle trick)
The law of excluded middle as in
![]() $(\exists ^{2})\vee \neg (\exists ^{2})$
is quite useful as follows: suppose we are proving
$(\exists ^{2})\vee \neg (\exists ^{2})$
is quite useful as follows: suppose we are proving
![]() $T\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
over
$T\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
over
![]() $\mathsf {RCA}_{0}^{\omega }$
. Now, in case
$\mathsf {RCA}_{0}^{\omega }$
. Now, in case
![]() $\neg (\exists ^{2})$
, all functions on
$\neg (\exists ^{2})$
, all functions on
![]() ${\mathbb R}$
are continuous by [Reference Kohlenbach48, Proposition 3.12] and
${\mathbb R}$
are continuous by [Reference Kohlenbach48, Proposition 3.12] and
![]() $\mathsf {NIN}_{\mathsf {alt}}$
then triviallyFootnote
8
holds. Hence, what remains is to establish
$\mathsf {NIN}_{\mathsf {alt}}$
then triviallyFootnote
8
holds. Hence, what remains is to establish
![]() $T\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
in case we have
$T\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
in case we have
![]() $(\exists ^{2})$
. However, the latter axiom, e.g., implies
$(\exists ^{2})$
. However, the latter axiom, e.g., implies
![]() $\mathsf {ACA}_{0}$
and can uniformly convert reals to their binary representations. In this way, finding a proof in
$\mathsf {ACA}_{0}$
and can uniformly convert reals to their binary representations. In this way, finding a proof in
![]() $\mathsf {RCA}_{0}^{\omega }+(\exists ^{2})$
is ‘much easier’ than finding a proof in
$\mathsf {RCA}_{0}^{\omega }+(\exists ^{2})$
is ‘much easier’ than finding a proof in
![]() $\mathsf {RCA}_{0}^{\omega }$
. In a nutshell, we may without loss of generality assume
$\mathsf {RCA}_{0}^{\omega }$
. In a nutshell, we may without loss of generality assume
![]() $(\exists ^{2})$
when proving theorems that are trivial (or readily proved) when all functions (on
$(\exists ^{2})$
when proving theorems that are trivial (or readily proved) when all functions (on
![]() ${\mathbb R}$
or
${\mathbb R}$
or
![]() ${\mathbb N}^{{\mathbb N} }$
) are continuous, like
${\mathbb N}^{{\mathbb N} }$
) are continuous, like
![]() $\mathsf {NIN}_{\mathsf {alt}}$
. Moreover, we can replace
$\mathsf {NIN}_{\mathsf {alt}}$
. Moreover, we can replace
![]() $2^{{\mathbb N}}$
by
$2^{{\mathbb N}}$
by
![]() $[0,1]$
at will, which is convenient sometimes.
$[0,1]$
at will, which is convenient sometimes.
While the previous trick is useful, it should be used sparingly: the axiom
![]() $(\exists ^{2})$
is required to guarantee that basic sets like the unit interval are sets in our sense (Definition 1.7) or that finite sets (Definition 1.2) are well-behaved. For this reason, we only mention Remark 3.5 in passing and shall generally work over
$(\exists ^{2})$
is required to guarantee that basic sets like the unit interval are sets in our sense (Definition 1.7) or that finite sets (Definition 1.2) are well-behaved. For this reason, we only mention Remark 3.5 in passing and shall generally work over
![]() $\mathsf {ACA}_{0}^{\omega }$
.
$\mathsf {ACA}_{0}^{\omega }$
.
3.3 Regulated functions and the uncountability of
 ${\mathbb R}$
${\mathbb R}$
We establish the equivalences sketched in Section 1.1 pertaining to the uncountability of
![]() ${\mathbb R}$
and properties of regulated functions.
${\mathbb R}$
and properties of regulated functions.
3.3.1 Volterra’s early work
In this section, we connect the uncountability of
![]() ${\mathbb R}$
to Volterra’s early results from Section 1.2. In particular, we establish the following theorem where the final two items exhibit some nice robustness properties of
${\mathbb R}$
to Volterra’s early results from Section 1.2. In particular, we establish the following theorem where the final two items exhibit some nice robustness properties of
![]() $\mathsf {NIN}_{\mathsf {alt}}$
and Volterra’s results, as promised in Section 1.1.
$\mathsf {NIN}_{\mathsf {alt}}$
and Volterra’s results, as promised in Section 1.1.
Theorem 3.6 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
The following are equivalent.
-
(a) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}$
.
$\mathsf {NIN}_{\mathsf {alt}}$
. -
(b) Volterra’s theorem for regulated functions: there do not exist two regulated functions defined on the unit interval for which the continuity points of one are the discontinuity points of the other, and vice versa.
-
(c) Volterra’s corollary for regulated functions: there is no regulated function that is continuous on
 ${\mathbb Q}\cap [0,1]$
and discontinuous on
${\mathbb Q}\cap [0,1]$
and discontinuous on
 $[0,1]\setminus {\mathbb Q}$
.
$[0,1]\setminus {\mathbb Q}$
. -
(d) Generalised Volterra’s corollary (Theorem 1.5) for regulated functions and height countable D (or: countable D, or: strongly countable D).
-
(e) For a sequence
 $(X_{n})_{n\in {\mathbb N}}$
of finite sets in
$(X_{n})_{n\in {\mathbb N}}$
of finite sets in
 $[0,1]$
, the set
$[0,1]$
, the set
 $[0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
is dense (or: not height countable, or: not countable, or: not strongly countable).
$[0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
is dense (or: not height countable, or: not countable, or: not strongly countable).
Proof First of all, Volterra’s theorem implies Volterra’s corollary (both restricted to regulated functions), as Thomae’s function T from (1.1) is readily defined using
![]() $\exists ^{2}$
, while the latter also shows that T is regulated and continuous exactly on
$\exists ^{2}$
, while the latter also shows that T is regulated and continuous exactly on
![]() ${\mathbb R}\setminus {\mathbb Q}$
.
${\mathbb R}\setminus {\mathbb Q}$
.
Secondly, we now derive
![]() $\mathsf {NIN}_{\mathsf {alt}}$
from Volterra’s corollary as in item (c). To this end, let
$\mathsf {NIN}_{\mathsf {alt}}$
from Volterra’s corollary as in item (c). To this end, let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets such that
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets such that
![]() $[0,1]=\cup _{n\in {\mathbb N}}X_{n}$
. Now use
$[0,1]=\cup _{n\in {\mathbb N}}X_{n}$
. Now use
![]() $\mu ^{2}$
to define the following function:
$\mu ^{2}$
to define the following function:
 $$ \begin{align} g(x):= \begin{cases} 0, & x\in {\mathbb Q},\\ \frac{1}{2^{n+1}}, & x \in {\mathbb R}\setminus {\mathbb Q} \wedge x\in X_{n} \text{ and } n \text{ is the least such number.} \end{cases} \end{align} $$
$$ \begin{align} g(x):= \begin{cases} 0, & x\in {\mathbb Q},\\ \frac{1}{2^{n+1}}, & x \in {\mathbb R}\setminus {\mathbb Q} \wedge x\in X_{n} \text{ and } n \text{ is the least such number.} \end{cases} \end{align} $$
We have
![]() $0=g(0+)=g(0-)=g(x+)=g(x-)$
for any
$0=g(0+)=g(0-)=g(x+)=g(x-)$
for any
![]() $x\in (0,1)$
, i.e., g is regulated. To establish this fact in our base theory, note that
$x\in (0,1)$
, i.e., g is regulated. To establish this fact in our base theory, note that
![]() $\cup _{k\leq n}X_{k}$
is finite for any
$\cup _{k\leq n}X_{k}$
is finite for any
![]() $n\in {\mathbb N}$
and can be enumerated, both thanks to
$n\in {\mathbb N}$
and can be enumerated, both thanks to
![]() $\mathsf {FIN}$
. As a result, g is continuous at any
$\mathsf {FIN}$
. As a result, g is continuous at any
![]() $x\in {\mathbb Q}\cap [0,1]$
and discontinuous at any
$x\in {\mathbb Q}\cap [0,1]$
and discontinuous at any
![]() $y\in [0,1]\setminus {\mathbb Q}$
. This contradicts Volterra’s corollary (for regulated functions), and
$y\in [0,1]\setminus {\mathbb Q}$
. This contradicts Volterra’s corollary (for regulated functions), and
![]() $\mathsf {NIN}_{\mathsf {alt}}$
follows.
$\mathsf {NIN}_{\mathsf {alt}}$
follows.
Thirdly, we derive Volterra’s corollary (for regulated functions) from
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, by contraposition. To this end, let f be regulated, continuous on
$\mathsf {NIN}_{\mathsf {alt}}$
, by contraposition. To this end, let f be regulated, continuous on
![]() $[0,1]\cap {\mathbb Q}$
, and discontinuous on
$[0,1]\cap {\mathbb Q}$
, and discontinuous on
![]() $[0,1]\setminus {\mathbb Q}$
. Now consider the following set
$[0,1]\setminus {\mathbb Q}$
. Now consider the following set
where we note that, e.g., the right limit
![]() $f(x+)$
for
$f(x+)$
for
![]() $x\in (0,1)$
equals
$x\in (0,1)$
equals
![]() $\lim _{k\rightarrow \infty }f(x+\frac {1}{2^{k}})$
; the latter limit is arithmetical and hence
$\lim _{k\rightarrow \infty }f(x+\frac {1}{2^{k}})$
; the latter limit is arithmetical and hence
![]() $\mu ^{2}$
readily obtains it. Hence, the set
$\mu ^{2}$
readily obtains it. Hence, the set
![]() $X_{n}$
from (3.2) can be defined in
$X_{n}$
from (3.2) can be defined in
![]() $\mathsf {ACA}_{0}^{\omega }$
. To show that
$\mathsf {ACA}_{0}^{\omega }$
. To show that
![]() $X_{n}$
is finite, suppose not and apply
$X_{n}$
is finite, suppose not and apply
![]() $\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
to find a sequence of reals in
$\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
to find a sequence of reals in
![]() $X_{n}$
. By the Bolzano–Weierstrass theorem from [Reference Simpson89, III.2], this sequence has a convergent sub-sequence, say with limit
$X_{n}$
. By the Bolzano–Weierstrass theorem from [Reference Simpson89, III.2], this sequence has a convergent sub-sequence, say with limit
![]() $c\in [0,1]$
; then either
$c\in [0,1]$
; then either
![]() $f(c-)$
or
$f(c-)$
or
![]() $f(c+)$
does not exist (using the usual epsilon-delta definition), a contradiction. Hence,
$f(c+)$
does not exist (using the usual epsilon-delta definition), a contradiction. Hence,
![]() $X_{n}$
is finite and by the assumptions on f, we have
$X_{n}$
is finite and by the assumptions on f, we have
![]() $D_{f}=\cup _{n\in {\mathbb N}}X_{n}= [0,1]\setminus {\mathbb Q}$
. Then
$D_{f}=\cup _{n\in {\mathbb N}}X_{n}= [0,1]\setminus {\mathbb Q}$
. Then
![]() $[0,1]=D_{f}\cup {\mathbb Q}=\big (\cup _{n\in {\mathbb N}}X_{n})\cup {\mathbb Q}$
shows that the unit interval is a union over
$[0,1]=D_{f}\cup {\mathbb Q}=\big (\cup _{n\in {\mathbb N}}X_{n})\cup {\mathbb Q}$
shows that the unit interval is a union over
![]() ${\mathbb N}$
of finite sets, i.e.,
${\mathbb N}$
of finite sets, i.e.,
![]() $\neg \mathsf {NIN}_{\mathsf {alt}}$
follows. One derives item (b) from
$\neg \mathsf {NIN}_{\mathsf {alt}}$
follows. One derives item (b) from
![]() $\mathsf {NIN}_{\mathsf {alt}}$
in the same way; indeed:
$\mathsf {NIN}_{\mathsf {alt}}$
in the same way; indeed:
![]() $[0,1]=D_{f}\cup D_{g}$
in case item (b) is false for regulated
$[0,1]=D_{f}\cup D_{g}$
in case item (b) is false for regulated
![]() $f, g:[0,1]\rightarrow {\mathbb R}$
, showing that the unit interval is the union over
$f, g:[0,1]\rightarrow {\mathbb R}$
, showing that the unit interval is the union over
![]() ${\mathbb N}$
of finite sets, yielding
${\mathbb N}$
of finite sets, yielding
![]() $\neg \mathsf {NIN}_{\mathsf {alt}}$
.
$\neg \mathsf {NIN}_{\mathsf {alt}}$
.
Fourth, we only need to show that
![]() $\mathsf {NIN}_{\mathsf {alt}}$
implies item (d), as
$\mathsf {NIN}_{\mathsf {alt}}$
implies item (d), as
![]() ${\mathbb Q}$
is trivially (height) countable and dense. Hence, let f be regulated, continuous on
${\mathbb Q}$
is trivially (height) countable and dense. Hence, let f be regulated, continuous on
![]() $[0,1]\cap D$
, and discontinuous on
$[0,1]\cap D$
, and discontinuous on
![]() $[0,1]\setminus D$
, where D is height countable and dense. In particular, assume
$[0,1]\setminus D$
, where D is height countable and dense. In particular, assume
![]() $D=\cup _{n\in {\mathbb N}}D_{n}$
where
$D=\cup _{n\in {\mathbb N}}D_{n}$
where
![]() $D_{n}$
is finite for
$D_{n}$
is finite for
![]() $n\in {\mathbb N}$
. Now consider
$n\in {\mathbb N}$
. Now consider
![]() $X_{n}$
as in (3.2) from the above and note that
$X_{n}$
as in (3.2) from the above and note that
![]() $[0,1]\setminus D=\cup _{n\in {\mathbb N}}X_{n}$
. Hence,
$[0,1]\setminus D=\cup _{n\in {\mathbb N}}X_{n}$
. Hence,
![]() $[0,1]=\cup _{n\in {\mathbb N}}Y_{n}$
where
$[0,1]=\cup _{n\in {\mathbb N}}Y_{n}$
where
![]() $Y_{n}=X_{n}\cup D_{n}$
is finite (as the components are), i.e.,
$Y_{n}=X_{n}\cup D_{n}$
is finite (as the components are), i.e.,
![]() $\neg \mathsf {NIN}_{\mathsf {alt}}$
follows.
$\neg \mathsf {NIN}_{\mathsf {alt}}$
follows.
Finally, we only need to show that
![]() $\mathsf {NIN}_{\mathsf {alt}}$
implies the final item (e). For the terms in brackets in the latter, this is trivial as (strongly) countable sets are height countable. For the density claim, let
$\mathsf {NIN}_{\mathsf {alt}}$
implies the final item (e). For the terms in brackets in the latter, this is trivial as (strongly) countable sets are height countable. For the density claim, let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets and suppose
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets and suppose
![]() $x_{0}\in [0,1]$
and
$x_{0}\in [0,1]$
and
![]() $N_{0}\in {\mathbb N}$
are such that
$N_{0}\in {\mathbb N}$
are such that
![]() $B(x_{0}, \frac {1}{2^{N_{0}}})\cap \big ([0,1]\setminus \cup _{n\in {\mathbb N}}X_{n} \big )$
is empty. Hence,
$B(x_{0}, \frac {1}{2^{N_{0}}})\cap \big ([0,1]\setminus \cup _{n\in {\mathbb N}}X_{n} \big )$
is empty. Hence,
![]() $B(x_{0}, \frac {1}{2^{N_{0}}})\subset \cup _{n\in {\mathbb N}}X_{n}$
and define the finite sets
$B(x_{0}, \frac {1}{2^{N_{0}}})\subset \cup _{n\in {\mathbb N}}X_{n}$
and define the finite sets
![]() $Y_{n}:= X_{n}\cap B(x_{0}, \frac {1}{2^{N_{0}}})$
using
$Y_{n}:= X_{n}\cap B(x_{0}, \frac {1}{2^{N_{0}}})$
using
![]() $\exists ^{2}$
. This implies
$\exists ^{2}$
. This implies
![]() $B(x_{0}, \frac {1}{2^{N_{0}}})= \cup _{n\in {\mathbb N}}Y_{n}$
, which contradicts
$B(x_{0}, \frac {1}{2^{N_{0}}})= \cup _{n\in {\mathbb N}}Y_{n}$
, which contradicts
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, modulo the rescaling discussed in Remark 3.4.
$\mathsf {NIN}_{\mathsf {alt}}$
, modulo the rescaling discussed in Remark 3.4.
The final item of the theorem essentially expresses the Baire category theorem restricted to the complement of finite sets, which are automatically open and dense.
3.3.2 Continuity and Riemann integration
We connect the uncountability of
![]() ${\mathbb R}$
to properties of regulated functions like continuity and Riemann integration.
${\mathbb R}$
to properties of regulated functions like continuity and Riemann integration.
First of all, we shall need the set of (dis)continuity points of regulated
![]() $f:[0,1]\rightarrow {\mathbb R}$
, definable via
$f:[0,1]\rightarrow {\mathbb R}$
, definable via
![]() $\exists ^{2}$
as follows:
$\exists ^{2}$
as follows:
These sets occupy a central spot in the study of regulated functions. We have the following theorem, where most items exhibit some kind of robustness.
Theorem 3.7 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
The following are equivalent.
-
(i) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}$
.
$\mathsf {NIN}_{\mathsf {alt}}$
. -
(ii) For any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous (or: quasi-continuous, or: lower semi-continuous).
$x\in [0,1]$
where f is continuous (or: quasi-continuous, or: lower semi-continuous). -
(iii) Any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
is pointwise discontinuous, i.e., the set
$f:[0,1]\rightarrow {\mathbb R}$
is pointwise discontinuous, i.e., the set
 $C_{f}$
is dense in the unit interval.
$C_{f}$
is dense in the unit interval. -
(iv) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, the set
$f:[0,1]\rightarrow {\mathbb R}$
, the set
 $C_{f}$
is not height countable (or: not countable, or: not strongly countable, or: not enumerable).
$C_{f}$
is not height countable (or: not countable, or: not strongly countable, or: not enumerable). -
(v) For regulated
 $f:[0,1]\rightarrow [0,1]$
such that the Riemann integral
$f:[0,1]\rightarrow [0,1]$
such that the Riemann integral
 $\int _{0}^{1}f(x)dx$
exists and is
$\int _{0}^{1}f(x)dx$
exists and is
 $0$
, there is
$0$
, there is
 $x\in [0,1]$
with
$x\in [0,1]$
with
 $f(x)=0$
Bourbaki ([Reference Bourbaki10, p. 61]).
$f(x)=0$
Bourbaki ([Reference Bourbaki10, p. 61]). -
(vi) For regulated
 $f:[0,1]\rightarrow [0,1]$
such that the Riemann integral
$f:[0,1]\rightarrow [0,1]$
such that the Riemann integral
 $\int _{0}^{1}f(x)dx$
exists and equals
$\int _{0}^{1}f(x)dx$
exists and equals
 $0$
, the set
$0$
, the set
 $\{x \in [0,1]: f(x)=0\}$
is dense ([Reference Bourbaki10, p. 61]).
$\{x \in [0,1]: f(x)=0\}$
is dense ([Reference Bourbaki10, p. 61]). -
(vii) Blumberg’s theorem [Reference Blumberg8] restricted to regulated functions on
 $[0,1]$
.
$[0,1]$
. -
(viii) Measure theoretic Blumberg’s theorem [Reference Brown16]: for regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a dense and uncountable (or: not strongly countable, or: not height countable) subset
$f:[0,1]\rightarrow {\mathbb R}$
, there is a dense and uncountable (or: not strongly countable, or: not height countable) subset
 $D\subset [0,1]$
such that
$D\subset [0,1]$
such that
 $f_{\upharpoonright D}$
is pointwise discontinuous.
$f_{\upharpoonright D}$
is pointwise discontinuous. -
(ix) For regulated
 $f:[0,1]\rightarrow (0, 1]$
, there exist
$f:[0,1]\rightarrow (0, 1]$
, there exist
 $N\in {\mathbb N}, x\in [0,1]$
such that
$N\in {\mathbb N}, x\in [0,1]$
such that
 $(\forall y\in B(x, \frac {1}{2^{N}}))(f(y)\geq \frac {1}{2^{N}})$
.
$(\forall y\in B(x, \frac {1}{2^{N}}))(f(y)\geq \frac {1}{2^{N}})$
. -
(x) For regulated
 $f:[0,1]\rightarrow (0, 1]$
, there exist a dense set D such that
$f:[0,1]\rightarrow (0, 1]$
, there exist a dense set D such that
 $f_{\upharpoonright D}$
is locally bounded away from zeroFootnote
9
(on D).
$f_{\upharpoonright D}$
is locally bounded away from zeroFootnote
9
(on D). -
(xi) (FTC) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
such that
$f:[0,1]\rightarrow {\mathbb R}$
such that
 $F(x):=\lambda x.\int _{0}^{x}f(t)dt$
exists, there is
$F(x):=\lambda x.\int _{0}^{x}f(t)dt$
exists, there is
 $x_{0}\in (0,1)$
where
$x_{0}\in (0,1)$
where
 $F(x)$
is differentiable with derivative
$F(x)$
is differentiable with derivative
 $f(x_{0})$
.
$f(x_{0})$
. -
(xii) For any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a Darboux point.
$f:[0,1]\rightarrow {\mathbb R}$
, there is a Darboux point. -
(xiii) For any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, its Darboux points are dense.
$f:[0,1]\rightarrow {\mathbb R}$
, its Darboux points are dense. -
(xiv) For any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
with only removable discontinuities, there is
$f:[0,1]\rightarrow {\mathbb R}$
with only removable discontinuities, there is
 $x\in [0,1]$
which is not a strictFootnote
10
local maximum.
$x\in [0,1]$
which is not a strictFootnote
10
local maximum.
Proof First of all, we prove item (ii) from
![]() $\mathsf {NIN}_{\mathsf {alt}}$
; we may use Volterra’s corollary as in Theorem 3.6. Fix regulated
$\mathsf {NIN}_{\mathsf {alt}}$
; we may use Volterra’s corollary as in Theorem 3.6. Fix regulated
![]() $f:[0,1]\rightarrow {\mathbb R}$
and consider this case distinction:
$f:[0,1]\rightarrow {\mathbb R}$
and consider this case distinction:
-
• If there is
 $q\in {\mathbb Q}\cap [0,1]$
with
$q\in {\mathbb Q}\cap [0,1]$
with
 $f(q+)= f(q-)=f(q)$
, item (ii) follows.
$f(q+)= f(q-)=f(q)$
, item (ii) follows. -
• If there is no such rational, then Volterra’s corollary guarantees there is
 $x\in [0,1]\setminus {\mathbb Q}$
such that f is continuous at x.
$x\in [0,1]\setminus {\mathbb Q}$
such that f is continuous at x.
In each case, there is a point of continuity for f, i.e., item (ii) follows. To prove that item (ii) implies
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, let
$\mathsf {NIN}_{\mathsf {alt}}$
, let
![]() $X:=\cup _{n\in {\mathbb N}}X_{n}$
be the union of finite sets
$X:=\cup _{n\in {\mathbb N}}X_{n}$
be the union of finite sets
![]() $X_{n}\subset [0,1]$
and define h as in (2.2). As for g from (3.1) in Theorem 3.6, h is regulated. Item (ii) then provides a point of continuity
$X_{n}\subset [0,1]$
and define h as in (2.2). As for g from (3.1) in Theorem 3.6, h is regulated. Item (ii) then provides a point of continuity
![]() $y\in [0,1]$
of h, which by definition must be such that
$y\in [0,1]$
of h, which by definition must be such that
![]() $y\not \in X$
. The same holds for quasi- and lower semi-continuity.
$y\not \in X$
. The same holds for quasi- and lower semi-continuity.
The implication (iii)
![]() $\rightarrow $
(ii) is immediate (as the empty set is not dense in
$\rightarrow $
(ii) is immediate (as the empty set is not dense in
![]() $[0,1]$
). To prove (i)
$[0,1]$
). To prove (i)
![]() $\rightarrow $
(iii), let f be regulated and such that
$\rightarrow $
(iii), let f be regulated and such that
![]() $C_{f}$
is not dense. To derive
$C_{f}$
is not dense. To derive
![]() $\neg \mathsf {NIN}_{\mathsf {alt}}$
, consider
$\neg \mathsf {NIN}_{\mathsf {alt}}$
, consider
![]() $X_{n}$
as in (3.2), which is finite for all
$X_{n}$
as in (3.2), which is finite for all
![]() $n\in {\mathbb N}$
(as is proved using
$n\in {\mathbb N}$
(as is proved using
![]() $\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
and the Bolzano–Weierstrass theorem). Since
$\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
and the Bolzano–Weierstrass theorem). Since
![]() $C_{f}$
is not dense in
$C_{f}$
is not dense in
![]() $[0,1]$
, there is
$[0,1]$
, there is
![]() $y\in [0,1]$
and
$y\in [0,1]$
and
![]() $N\in {\mathbb N}$
such that
$N\in {\mathbb N}$
such that
![]() $B(y, \frac {1}{2^{N}})\cap C_{f}=\emptyset $
. By definition, the set
$B(y, \frac {1}{2^{N}})\cap C_{f}=\emptyset $
. By definition, the set
![]() $D_{f}=\cup _{n\in {\mathbb N}}X_{n}$
collects all points where f is discontinuous. Hence,
$D_{f}=\cup _{n\in {\mathbb N}}X_{n}$
collects all points where f is discontinuous. Hence,
![]() $[0,1]\setminus C_{f}=D_{f}$
, yielding
$[0,1]\setminus C_{f}=D_{f}$
, yielding
![]() $B(y, \frac {1}{2^{N}})\subset D_{f}$
. Now define
$B(y, \frac {1}{2^{N}})\subset D_{f}$
. Now define
![]() $Y_{n}=X_{n}\cap B(y, \frac {1}{2^{N}})$
, which is finite since
$Y_{n}=X_{n}\cap B(y, \frac {1}{2^{N}})$
, which is finite since
![]() $X_{n}$
is finite. Hence,
$X_{n}$
is finite. Hence,
![]() $\cup _{n\in {\mathbb N}}Y_{n}=B(y, \frac {1}{2^{N}})$
, i.e., an interval can be expressed as the union over
$\cup _{n\in {\mathbb N}}Y_{n}=B(y, \frac {1}{2^{N}})$
, i.e., an interval can be expressed as the union over
![]() ${\mathbb N}$
of finite sets, which readily yields
${\mathbb N}$
of finite sets, which readily yields
![]() $\neg \mathsf {NIN}_{\mathsf {alt}}$
after rescaling as in Remark 3.4.
$\neg \mathsf {NIN}_{\mathsf {alt}}$
after rescaling as in Remark 3.4.
Regarding item (iv), it suffices to derive the latter from
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, which is immediate as
$\mathsf {NIN}_{\mathsf {alt}}$
, which is immediate as
![]() $D_{f}$
is height countable. Indeed, if
$D_{f}$
is height countable. Indeed, if
![]() $C_{f}$
is also height countable, then
$C_{f}$
is also height countable, then
![]() $[0,1]=C_{f}\cup D_{f}$
is height countable, contradicting
$[0,1]=C_{f}\cup D_{f}$
is height countable, contradicting
![]() $\mathsf {NIN}_{\mathsf {alt}}$
. In case
$\mathsf {NIN}_{\mathsf {alt}}$
. In case
![]() $A\subset [0,1]$
is countable, then any
$A\subset [0,1]$
is countable, then any
![]() $Y:[0,1]\rightarrow {\mathbb N}$
injective on A is also a height function, i.e., A is also height countable. The same holds for strongly countable and enumerable sets.
$Y:[0,1]\rightarrow {\mathbb N}$
injective on A is also a height function, i.e., A is also height countable. The same holds for strongly countable and enumerable sets.
Regarding items (v) and (vi), the latter immediately follow from items (ii) and (iii). Indeed, in case
![]() $f(x)>0$
for
$f(x)>0$
for
![]() $x\in C_{f}$
, then by continuity there are
$x\in C_{f}$
, then by continuity there are
![]() $k, N\in {\mathbb N}$
such that
$k, N\in {\mathbb N}$
such that
![]() $f(y)>\frac {1}{2^{k}}$
for
$f(y)>\frac {1}{2^{k}}$
for
![]() $y\in B(x, \frac {1}{2^{N+1}})$
, implying
$y\in B(x, \frac {1}{2^{N+1}})$
, implying
![]() $\int _{0}^{1}f(x)dx>\frac {1}{2^{k}2^{N}}>0$
. Now assume item (v), let
$\int _{0}^{1}f(x)dx>\frac {1}{2^{k}2^{N}}>0$
. Now assume item (v), let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets, and let h be as in (2.2). The latter is Riemann integrable with
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets, and let h be as in (2.2). The latter is Riemann integrable with
![]() $\int _{0}^{1}h(x)dx=0$
, which one shows via the usual ‘epsilon-delta’ definition and
$\int _{0}^{1}h(x)dx=0$
, which one shows via the usual ‘epsilon-delta’ definition and
![]() $\mathsf {FIN}$
. Any
$\mathsf {FIN}$
. Any
![]() $y\in [0,1]$
such that
$y\in [0,1]$
such that
![]() $h(y)=0$
, also satisfies
$h(y)=0$
, also satisfies
![]() $y\not \in \cup _{n\in {\mathbb N}} X_{n}$
, i.e.,
$y\not \in \cup _{n\in {\mathbb N}} X_{n}$
, i.e.,
![]() $ \mathsf {NIN}_{\mathsf {alt}}$
follows.
$ \mathsf {NIN}_{\mathsf {alt}}$
follows.
Next, we clearly have (iii)
![]() $\rightarrow $
(vii) and (iv)
$\rightarrow $
(vii) and (iv)
![]() $\rightarrow$
(viii) since
$\rightarrow$
(viii) since
![]() $D=C_{f}$
is as required. To prove (vii)
$D=C_{f}$
is as required. To prove (vii)
![]() $\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
, let
$\rightarrow \mathsf {NIN}_{\mathsf {alt}}$
, let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets in
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets in
![]() $[0,1]$
and consider the regulated function h from (2.2). Let D be the dense set provided by item (vii) and consider
$[0,1]$
and consider the regulated function h from (2.2). Let D be the dense set provided by item (vii) and consider
![]() $y\in D$
. In case
$y\in D$
. In case
![]() $h(y)\ne 0$
, say
$h(y)\ne 0$
, say
![]() $h(y)>\frac {1}{2^{k_{0}}}$
, use
$h(y)>\frac {1}{2^{k_{0}}}$
, use
![]() $\mathsf {FIN}$
to enumerate
$\mathsf {FIN}$
to enumerate
![]() $\cup _{n\leq k_{0}+1} X_{n}$
. Hence, we can find
$\cup _{n\leq k_{0}+1} X_{n}$
. Hence, we can find
![]() $N\in {\mathbb N}$
such that for
$N\in {\mathbb N}$
such that for
![]() $z\in B(x, \frac {1}{2^{N}})$
,
$z\in B(x, \frac {1}{2^{N}})$
,
![]() $h(z)<\frac {1}{2^{k_{0}+1}}$
, i.e.,
$h(z)<\frac {1}{2^{k_{0}+1}}$
, i.e.,
![]() $h_{\upharpoonright D}$
is not continuous (on D). This contradiction implies that
$h_{\upharpoonright D}$
is not continuous (on D). This contradiction implies that
![]() $h(y)=0$
, meaning
$h(y)=0$
, meaning
![]() $y\in [0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
, i.e.,
$y\in [0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
, i.e.,
![]() $\mathsf {NIN}_{\mathsf {alt}}$
follows. A similar proof works for item (viii) by considering a point from the dense set of continuity points of
$\mathsf {NIN}_{\mathsf {alt}}$
follows. A similar proof works for item (viii) by considering a point from the dense set of continuity points of
![]() $f_{\upharpoonright D}$
.
$f_{\upharpoonright D}$
.
Next, (ii) (resp. (iii)) clearly implies (ix) (resp. (x)). To show that (ix) and (x) imply
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, one proceeds as in the previous paragraphs. Similarly, (ii) (resp. (iii)) implies (xii) (resp. (xiii)), as any continuity point is a Darboux point, by definition. To show that (xii) implies
$\mathsf {NIN}_{\mathsf {alt}}$
, one proceeds as in the previous paragraphs. Similarly, (ii) (resp. (iii)) implies (xii) (resp. (xiii)), as any continuity point is a Darboux point, by definition. To show that (xii) implies
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, one considers the function h as in (2.2) and notes that a Darboux point of h is not in
$\mathsf {NIN}_{\mathsf {alt}}$
, one considers the function h as in (2.2) and notes that a Darboux point of h is not in
![]() $\cup _{n\in {\mathbb N}}X_{n}$
.
$\cup _{n\in {\mathbb N}}X_{n}$
.
For item (xi), the usual epsilon-delta proof establishes that
![]() $F(x):=\int _{0}^{x}f(t)dt$
is continuous and that
$F(x):=\int _{0}^{x}f(t)dt$
is continuous and that
![]() $F'(y)=f(y)$
in case f is continuous at y, i.e., item (ii) implies item (xi). As noted above, h as in (2.2) satisfies
$F'(y)=f(y)$
in case f is continuous at y, i.e., item (ii) implies item (xi). As noted above, h as in (2.2) satisfies
![]() $H(x):=\int _{0}^{x}h(x)dt=0$
for any
$H(x):=\int _{0}^{x}h(x)dt=0$
for any
![]() $x\in [0,1]$
, i.e., any
$x\in [0,1]$
, i.e., any
![]() $y\in (0,1)$
such that
$y\in (0,1)$
such that
![]() $H'(y)=0=h(y)$
is such that
$H'(y)=0=h(y)$
is such that
![]() $y\not \in \cup _{n\in {\mathbb N}}X_{n}$
, yielding
$y\not \in \cup _{n\in {\mathbb N}}X_{n}$
, yielding
![]() $\mathsf {NIN}_{\mathsf {alt}}$
as required.
$\mathsf {NIN}_{\mathsf {alt}}$
as required.
Finally, assume item (xiv), let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets, and note that h as in (2.2) is regulated with only removable discontinuities. Now, the set
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets, and note that h as in (2.2) is regulated with only removable discontinuities. Now, the set
![]() $X=\cup _{n\in {\mathbb N}}X_{n}$
consists of the local strict maxima of h, i.e., item (xiv) yields
$X=\cup _{n\in {\mathbb N}}X_{n}$
consists of the local strict maxima of h, i.e., item (xiv) yields
![]() $\mathsf {NIN}_{\mathsf {alt}}$
. For the reversal,
$\mathsf {NIN}_{\mathsf {alt}}$
. For the reversal,
![]() $\exists ^{2}$
computes a functional M such that
$\exists ^{2}$
computes a functional M such that
![]() $M(g, a, b)$
is a maximum of
$M(g, a, b)$
is a maximum of
![]() $g\in C([0,1])$
on
$g\in C([0,1])$
on
![]() $[a,b]\subset [0,1]$
(see [Reference Kohlenbach48, Section 3]), i.e.,
$[a,b]\subset [0,1]$
(see [Reference Kohlenbach48, Section 3]), i.e.,
![]() $(\forall y\in [a,b])(g(y)\leq g(M(g, a,b)))$
. Using the functional M, one readily shows that ‘x is a strict local maximum of g’ is decidableFootnote
11
given
$(\forall y\in [a,b])(g(y)\leq g(M(g, a,b)))$
. Using the functional M, one readily shows that ‘x is a strict local maximum of g’ is decidableFootnote
11
given
![]() $\exists ^{2}$
, for g continuous on
$\exists ^{2}$
, for g continuous on
![]() $[0,1]$
. Now let
$[0,1]$
. Now let
![]() $f:[0,1]\rightarrow {\mathbb R}$
be regulated and with only removable discontinuities. Use
$f:[0,1]\rightarrow {\mathbb R}$
be regulated and with only removable discontinuities. Use
![]() $\exists ^{2}$
to define
$\exists ^{2}$
to define
![]() $\tilde {f}:[0,1]\rightarrow {\mathbb R}$
as follows:
$\tilde {f}:[0,1]\rightarrow {\mathbb R}$
as follows:
![]() $\tilde {f}(x):= f(x+)$
for
$\tilde {f}(x):= f(x+)$
for
![]() $x\in [0, 1)$
and
$x\in [0, 1)$
and
![]() $\tilde {f}(1)=f(1-)$
. By definition,
$\tilde {f}(1)=f(1-)$
. By definition,
![]() $\tilde {f}$
is continuous on
$\tilde {f}$
is continuous on
![]() $[0,1]$
, and
$[0,1]$
, and
![]() $\exists ^{2}$
computes a (continuous) modulus of continuity, which follows in the same way as for Baire space (see, e.g., [Reference Kohlenbach47, Section 4]). In case f is discontinuous at
$\exists ^{2}$
computes a (continuous) modulus of continuity, which follows in the same way as for Baire space (see, e.g., [Reference Kohlenbach47, Section 4]). In case f is discontinuous at
![]() $x\in [0,1]$
, the latter point is a strict local maximum of f if and only if
$x\in [0,1]$
, the latter point is a strict local maximum of f if and only if
![]() $f(x)>f(x+)$
(or
$f(x)>f(x+)$
(or
![]() $f(x)>f(x-)$
in case
$f(x)>f(x-)$
in case
![]() $x=1$
). Note that
$x=1$
). Note that
![]() $\mu ^{2}$
(together with a modulus of continuity for
$\mu ^{2}$
(together with a modulus of continuity for
![]() $\tilde {f}$
) readily yields
$\tilde {f}$
) readily yields
![]() $N_{f, x}\in {\mathbb N}$
such that
$N_{f, x}\in {\mathbb N}$
such that
![]() $(\forall y\in B(x, \frac {1}{2^{N_{f,x}}}))( f(y)<f(x))$
, in case x is a strict local maximum of f. In case f is continuous at
$(\forall y\in B(x, \frac {1}{2^{N_{f,x}}}))( f(y)<f(x))$
, in case x is a strict local maximum of f. In case f is continuous at
![]() $x\in [0,1]$
, we can use
$x\in [0,1]$
, we can use
![]() $\exists ^{2}$
to decide whether x is a local strict maximum of
$\exists ^{2}$
to decide whether x is a local strict maximum of
![]() $\tilde {f}$
. By Footnote 11,
$\tilde {f}$
. By Footnote 11,
![]() $\mu ^{2}$
again yields
$\mu ^{2}$
again yields
![]() $N_{f, x}\in {\mathbb N}$
such that
$N_{f, x}\in {\mathbb N}$
such that
![]() $(\forall y\in B(x, \frac {1}{2^{N}}))( \tilde {f}(y)<\tilde {f}(x)))$
, in case x is a strict local maximum of
$(\forall y\in B(x, \frac {1}{2^{N}}))( \tilde {f}(y)<\tilde {f}(x)))$
, in case x is a strict local maximum of
![]() $\tilde {f}$
. Now consider the following set:
$\tilde {f}$
. Now consider the following set:
Then
![]() $A_{n}$
is finite as strict local maxima cannot be ‘too close’. Hence,
$A_{n}$
is finite as strict local maxima cannot be ‘too close’. Hence,
![]() $\mathsf {NIN}_{\mathsf {alt}}$
yields
$\mathsf {NIN}_{\mathsf {alt}}$
yields
![]() $y \in [0,1]\setminus \cup _{n\in {\mathbb N}}A_{n}$
, which is not a local maximum of f by definition, i.e., item (xiv) follows, and we are done.
$y \in [0,1]\setminus \cup _{n\in {\mathbb N}}A_{n}$
, which is not a local maximum of f by definition, i.e., item (xiv) follows, and we are done.
We may view item (i) as an extremely basic version of the connectedness of
![]() $[0,1]$
, as defined by Jordan in [Reference Jordan43, pp. 24–28]. Similarly, item (xi) is an extremely basic version of the fundamental theorem of calculus (FTC) and item (ii) is an extremely basic version of the Lebesgue criterion for Riemann integrability. Moreover, it seems necessary to formulate items (v), (vi), and (xi) with the extra condition that the functions at hand be Riemann integrable. As an exercise, the reader should prove that
$[0,1]$
, as defined by Jordan in [Reference Jordan43, pp. 24–28]. Similarly, item (xi) is an extremely basic version of the fundamental theorem of calculus (FTC) and item (ii) is an extremely basic version of the Lebesgue criterion for Riemann integrability. Moreover, it seems necessary to formulate items (v), (vi), and (xi) with the extra condition that the functions at hand be Riemann integrable. As an exercise, the reader should prove that
![]() $h:[0,1]\rightarrow {\mathbb R}$
as in (2.2) is effectively Riemann integrable, i.e., there is a functional that outputs the ‘
$h:[0,1]\rightarrow {\mathbb R}$
as in (2.2) is effectively Riemann integrable, i.e., there is a functional that outputs the ‘
![]() $\delta>0$
’ on input the ‘
$\delta>0$
’ on input the ‘
![]() $\varepsilon>0$
’ as in the usual epsilon-delta definition of Riemann integrability.
$\varepsilon>0$
’ as in the usual epsilon-delta definition of Riemann integrability.
Moreover, the notion of ‘left and right limits’ gives rise to the notion of ‘left and right derivatives’; following item (xi), the left (resp. right) limit of regulated
![]() $f:[0,1]\rightarrow {\mathbb R}$
equals the left (resp. right) derivative of F at every
$f:[0,1]\rightarrow {\mathbb R}$
equals the left (resp. right) derivative of F at every
![]() $x_{0}\in (0,1)$
via a completely elementary proof (say in
$x_{0}\in (0,1)$
via a completely elementary proof (say in
![]() $\mathsf {ACA}_{0}^{\omega }$
). We could also formulate item (ii) with approximate continuity (see, e.g., [Reference Bruckner17, II.5]) in the conclusion, but this notion seems to involve a lot of measure theory.
$\mathsf {ACA}_{0}^{\omega }$
). We could also formulate item (ii) with approximate continuity (see, e.g., [Reference Bruckner17, II.5]) in the conclusion, but this notion seems to involve a lot of measure theory.
3.3.3 Restrictions of regulated functions
In this section, we show that the above equivalences for
![]() $\mathsf {NIN}_{\mathsf {alt}}$
remain valid if we impose certain natural restrictions.
$\mathsf {NIN}_{\mathsf {alt}}$
remain valid if we impose certain natural restrictions.
First of all, the above results show that we have to be careful with intuitive statements like regulated functions are ‘close to continuous’. Indeed, by Theorem 3.7,
![]() $\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
is consistent with the existence of regulated functions that are discontinuous everywhere. Similarly,
$\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
is consistent with the existence of regulated functions that are discontinuous everywhere. Similarly,
![]() $\mathsf {NIN}$
follows from the statement a regulated function is Baire 1 by [Reference Normann and Sanders72, Theorem 2.34]. Hence,
$\mathsf {NIN}$
follows from the statement a regulated function is Baire 1 by [Reference Normann and Sanders72, Theorem 2.34]. Hence,
![]() $\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
cannot prove the latter basic fact and the restriction in item (ii) in Theorem 3.8 is therefore non-trivial. Similar results hold for items items (iii)–(iv) in Theorem 3.8 following [Reference Normann and Sanders72, Section 2.8].
$\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
cannot prove the latter basic fact and the restriction in item (ii) in Theorem 3.8 is therefore non-trivial. Similar results hold for items items (iii)–(iv) in Theorem 3.8 following [Reference Normann and Sanders72, Section 2.8].
Theorem 3.8 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
The following are equivalent.
-
(i) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}$
.
$\mathsf {NIN}_{\mathsf {alt}}$
. -
(ii) For any regulated and Baire 2 function
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous. -
(iii) For any regulated and Baire 1
 $^{*}$
function
$^{*}$
function
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous. -
(iv) For any regulated and usco (or: lsco) function
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous.
Proof In light of Theorem 3.7, we only need to show that items (ii)–(iv) imply
![]() $\mathsf {NIN}_{\mathsf {alt}}$
. The function
$\mathsf {NIN}_{\mathsf {alt}}$
. The function
![]() $h:[0,1]\rightarrow {\mathbb R}$
from (2.2) is central in the proof of the former theorem. For item (ii), h is Baire 2 as follows: define
$h:[0,1]\rightarrow {\mathbb R}$
from (2.2) is central in the proof of the former theorem. For item (ii), h is Baire 2 as follows: define
![]() $h_{n}(x)$
as
$h_{n}(x)$
as
![]() $h(x)$
in case
$h(x)$
in case
![]() $x\in \cup _{m\leq n}X_{n}$
, and
$x\in \cup _{m\leq n}X_{n}$
, and
![]() $0$
otherwise. By definition, h is the pointwise limit of
$0$
otherwise. By definition, h is the pointwise limit of
![]() $(h_{n})_{n\in {\mathbb N}}$
and
$(h_{n})_{n\in {\mathbb N}}$
and
![]() $\mathsf {FIN}$
allows us to enumerate
$\mathsf {FIN}$
allows us to enumerate
![]() $\cup _{m\leq n_{0}}X_{n}$
for fixed
$\cup _{m\leq n_{0}}X_{n}$
for fixed
![]() $n_{0}\in {\mathbb N}$
. Hence, for fixed
$n_{0}\in {\mathbb N}$
. Hence, for fixed
![]() $n_{0}\in {\mathbb N}$
,
$n_{0}\in {\mathbb N}$
,
![]() $h_{n_{0}}$
has at most finitely many points of discontinuity. In particular, there is an obvious sequence of continuous function with pointwise limit
$h_{n_{0}}$
has at most finitely many points of discontinuity. In particular, there is an obvious sequence of continuous function with pointwise limit
![]() $h_{n_{0}}$
, i.e., the latter is Baire 1. For item (iv), the function h is also usco as follows: in case
$h_{n_{0}}$
, i.e., the latter is Baire 1. For item (iv), the function h is also usco as follows: in case
![]() $h(x_{0})=0$
for
$h(x_{0})=0$
for
![]() $x_{0}\in [0,1]$
, the definition of usco is trivially satisfied. In case
$x_{0}\in [0,1]$
, the definition of usco is trivially satisfied. In case
![]() $h(x_{0})=\frac {1}{2^{n+1}}$
, then there is
$h(x_{0})=\frac {1}{2^{n+1}}$
, then there is
![]() $N\in {\mathbb N}$
such that
$N\in {\mathbb N}$
such that
![]() $(\forall y\in B(x, \frac {1}{2^{N}}))( y\not \in \cup _{m\leq n-1}X_{m} )$
as in the proof of Theorem 3.7. In this case, the definition of usco is also satisfied. The function
$(\forall y\in B(x, \frac {1}{2^{N}}))( y\not \in \cup _{m\leq n-1}X_{m} )$
as in the proof of Theorem 3.7. In this case, the definition of usco is also satisfied. The function
![]() $\lambda x. (1-h(x))$
is lsco. For item (iii), define the closed sets
$\lambda x. (1-h(x))$
is lsco. For item (iii), define the closed sets
![]() $C_{n}:= \cup _{m\leq n}X_{m}$
and note that the restriction of h to
$C_{n}:= \cup _{m\leq n}X_{m}$
and note that the restriction of h to
![]() $C_{n}$
is continuous for each n, i.e., h is also Baire 1
$C_{n}$
is continuous for each n, i.e., h is also Baire 1
![]() $^{*}$
.
$^{*}$
.
Secondly, we point out one subtlety in the previous proof: it is only shown that
![]() $h:[0,1]\rightarrow {\mathbb R}$
from (2.2) is Baire 2. In particular, we cannotFootnote
12
construct a double sequence of continuous functions with iterated limit equal to h. As it happens, Baire himself notes in [Reference Baire3, p. 69] that Baire 2 functions can be represented by such double sequences. We could generalise item (ii) in Theorem 3.8 to any higher Baire class and beyond, i.e., the latter theorem constitutes robustness in the flesh.
$h:[0,1]\rightarrow {\mathbb R}$
from (2.2) is Baire 2. In particular, we cannotFootnote
12
construct a double sequence of continuous functions with iterated limit equal to h. As it happens, Baire himself notes in [Reference Baire3, p. 69] that Baire 2 functions can be represented by such double sequences. We could generalise item (ii) in Theorem 3.8 to any higher Baire class and beyond, i.e., the latter theorem constitutes robustness in the flesh.
Moreover, going against our intuitions, we cannot replace ‘Baire 2’ by ‘Baire 1’ in Theorem 3.8 as the latter condition renders items (ii)–(iv) provable in
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
by the results in Section 3.5. In particular, while Baire
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
by the results in Section 3.5. In particular, while Baire
![]() $1^{*}$
and usco are subclasses of Baire 1, say in
$1^{*}$
and usco are subclasses of Baire 1, say in
![]() $\mathsf {ZF}$
or
$\mathsf {ZF}$
or
![]() $\mathsf {Z}_{2}^{\Omega }$
, these inclusions do not necessarily hold in weaker systems. For instance, it is consistent with
$\mathsf {Z}_{2}^{\Omega }$
, these inclusions do not necessarily hold in weaker systems. For instance, it is consistent with
![]() $\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
that there are totally discontinuous usco and regulated functions (see Theorem 3.7). An interesting RM-question would be to calibrate the strength of some of the well-known inclusions, like a regulated function on the unit interval is Baire 1.
$\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
that there are totally discontinuous usco and regulated functions (see Theorem 3.7). An interesting RM-question would be to calibrate the strength of some of the well-known inclusions, like a regulated function on the unit interval is Baire 1.
Thirdly, the supremum principle for regulated functions implies
![]() $\mathsf {NIN}$
by [Reference Normann and Sanders72, Theorem 2.32] where the former principle states the existence of
$\mathsf {NIN}$
by [Reference Normann and Sanders72, Theorem 2.32] where the former principle states the existence of
![]() $F:{\mathbb Q}^{2}\rightarrow {\mathbb R}$
such that
$F:{\mathbb Q}^{2}\rightarrow {\mathbb R}$
such that
![]() $F(p, q)=\sup _{x\in [p,q]}f(x)$
for any
$F(p, q)=\sup _{x\in [p,q]}f(x)$
for any
![]() $p, q\subset [0,1]\cap {\mathbb Q}$
. Indeed, using the well-known interval-halving technique, a supremum functional for
$p, q\subset [0,1]\cap {\mathbb Q}$
. Indeed, using the well-known interval-halving technique, a supremum functional for
![]() $h:[0,1]\rightarrow {\mathbb N}$
as in (2.2) would allow us to enumerate the associated union
$h:[0,1]\rightarrow {\mathbb N}$
as in (2.2) would allow us to enumerate the associated union
![]() $\cup _{n\in {\mathbb N}}X_{n}$
, i.e.,
$\cup _{n\in {\mathbb N}}X_{n}$
, i.e.,
![]() $\mathsf {NIN}_{\mathsf {alt}}$
readily follows. Perhaps surprisingly, the equivalences for
$\mathsf {NIN}_{\mathsf {alt}}$
readily follows. Perhaps surprisingly, the equivalences for
![]() $\mathsf {NIN}_{\mathsf {alt}}$
from the previous sections still go through if we restrict to regulated functions with a supremum functional. Regarding item (iv), the Heaviside function is regulated but not symmetrically continuous, where the latter notion goes back to Hausdorff [Reference Hausdorff32].
$\mathsf {NIN}_{\mathsf {alt}}$
from the previous sections still go through if we restrict to regulated functions with a supremum functional. Regarding item (iv), the Heaviside function is regulated but not symmetrically continuous, where the latter notion goes back to Hausdorff [Reference Hausdorff32].
Theorem 3.9 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
)
The following are equivalent.
-
(i) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}$
.
$\mathsf {NIN}_{\mathsf {alt}}$
. -
(ii) For regulated
 $f:[0,1]\rightarrow {\mathbb R}$
with a supremum functional, there is
$f:[0,1]\rightarrow {\mathbb R}$
with a supremum functional, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous. -
(iii) For regulated and lsco
 $f:[0,1]\rightarrow {\mathbb R}$
with a supremum functional, there is
$f:[0,1]\rightarrow {\mathbb R}$
with a supremum functional, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous. -
(iv) For regulated and symmetrically continuous
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous.
Proof The first item implies the other items by Theorem 3.7. Now assume the second item and let
![]() $Y:[0,1]\rightarrow {\mathbb N}$
be an injection and define
$Y:[0,1]\rightarrow {\mathbb N}$
be an injection and define
![]() $e(x):= \sum _{n=0}^{Y(x)+1}\frac {x^{n}}{n!} $
. By definition, we have
$e(x):= \sum _{n=0}^{Y(x)+1}\frac {x^{n}}{n!} $
. By definition, we have
![]() $e(x)<e^{x}$
for
$e(x)<e^{x}$
for
![]() $x\in [0,1]$
. Using the second item of
$x\in [0,1]$
. Using the second item of
![]() $\mathsf {FIN}$
, we have
$\mathsf {FIN}$
, we have
![]() $e(x+)=e(x-)=e^{x}$
for
$e(x+)=e(x-)=e^{x}$
for
![]() $x\in (0,1)$
. Indeed, for small enough neighbourhoods U of
$x\in (0,1)$
. Indeed, for small enough neighbourhoods U of
![]() $x\in (0,1)$
, Y is arbitrarily large on
$x\in (0,1)$
, Y is arbitrarily large on
![]() $U\setminus \{x\}$
, while
$U\setminus \{x\}$
, while
![]() $e^{x}$
is uniformly continuous on
$e^{x}$
is uniformly continuous on
![]() $[0,1]$
. Hence,
$[0,1]$
. Hence,
![]() $\lambda x.e(x)$
is regulated (and lsco) and
$\lambda x.e(x)$
is regulated (and lsco) and
![]() $\sup _{x\in [p,q]}e(x)=e^{q}$
also follows. Since the former function is totally discontinuous, we obtain a contradiction. To show that
$\sup _{x\in [p,q]}e(x)=e^{q}$
also follows. Since the former function is totally discontinuous, we obtain a contradiction. To show that
![]() $\lambda x.e(x)$
is also symmetrically continuous, note that the second item of
$\lambda x.e(x)$
is also symmetrically continuous, note that the second item of
![]() $\mathsf {FIN}$
implies that
$\mathsf {FIN}$
implies that
![]() $|e(x+h)-e(x-h)|$
is arbitrarily small for small enough
$|e(x+h)-e(x-h)|$
is arbitrarily small for small enough
![]() $h\in {\mathbb R}$
.
$h\in {\mathbb R}$
.
Finally, there are a number of equivalent definitions of ‘Baire 1’ on the reals [Reference Baire4, Reference Koumoullis52, Reference Lee, Tang and Zhao57], including the following ones by [Reference Koumoullis52, Theorem 2.3] and [Reference Kuratowski55, Section 34, VII].
Definition 3.10.
-
• Any
 $f:[0,1]\rightarrow {\mathbb R}$
is fragmented if for any
$f:[0,1]\rightarrow {\mathbb R}$
is fragmented if for any
 $\varepsilon>0$
and closed
$\varepsilon>0$
and closed
 $C\subset [0,1]$
, there is non-empty relativelyFootnote
13
open
$C\subset [0,1]$
, there is non-empty relativelyFootnote
13
open
 $O\subset C$
such that
$O\subset C$
such that
 $\mathsf {diam}(f(O))<\varepsilon $
.
$\mathsf {diam}(f(O))<\varepsilon $
. -
• Any
 $f:[0,1]\rightarrow {\mathbb R}$
is B-measurable of class 1 if for every open
$f:[0,1]\rightarrow {\mathbb R}$
is B-measurable of class 1 if for every open
 $Y\subset {\mathbb R}$
, the set
$Y\subset {\mathbb R}$
, the set
 $f^{-1}(Y)$
is
$f^{-1}(Y)$
is
 $\textbf {F}_{\sigma }$
, i.e., a union over
$\textbf {F}_{\sigma }$
, i.e., a union over
 ${\mathbb N}$
of closed sets.
${\mathbb N}$
of closed sets.
The diameter of a set X of reals is defined as usual, namely
![]() $\mathsf {diam}(X):=\sup _{x, y \in X}|x-y|$
, where the latter supremum need not exist for Definition 3.10. We have the following theorem, similar to Theorem 3.8.
$\mathsf {diam}(X):=\sup _{x, y \in X}|x-y|$
, where the latter supremum need not exist for Definition 3.10. We have the following theorem, similar to Theorem 3.8.
Theorem 3.11 (
 $\mathsf {ACA}_{0}^{\omega }+ \mathsf {IND}_{0}$
)
$\mathsf {ACA}_{0}^{\omega }+ \mathsf {IND}_{0}$
)
The following are equivalent.
-
(a) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}$
.
$\mathsf {NIN}_{\mathsf {alt}}$
. -
(b) For fragmented and regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a point
$f:[0,1]\rightarrow {\mathbb R}$
, there is a point
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous. -
(c) For B-measurable of class 1 and regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is
$f:[0,1]\rightarrow {\mathbb R}$
, there is
 $x\in [0,1]$
where f is continuous.
$x\in [0,1]$
where f is continuous.
Proof In light of Theorem 3.7, it suffices to prove that h from (2.2) is fragmented and B-measurable of class
![]() $1$
. For the former notion, for fixed
$1$
. For the former notion, for fixed
![]() $k\in {\mathbb N}$
,
$k\in {\mathbb N}$
,
![]() $\mathsf {FIN}$
can enumerate the (finitely many)
$\mathsf {FIN}$
can enumerate the (finitely many)
![]() $x\in [0,1]$
such that
$x\in [0,1]$
such that
![]() $h(x)\geq \frac {1}{2^{k}}$
. Any open set O not including these points is such that
$h(x)\geq \frac {1}{2^{k}}$
. Any open set O not including these points is such that
![]() $\textsf {diam}(h(O))<\frac {1}{2^{k}}$
, showing that h is fragmented.
$\textsf {diam}(h(O))<\frac {1}{2^{k}}$
, showing that h is fragmented.
For the B-measurability (of first class), in case
![]() $(X_{n})_{n\in {\mathbb N}}$
is a sequence of finite sets such that
$(X_{n})_{n\in {\mathbb N}}$
is a sequence of finite sets such that
![]() $[0,1]=\cup _{n\in {\mathbb N}}X_{n}$
, note that for any
$[0,1]=\cup _{n\in {\mathbb N}}X_{n}$
, note that for any
![]() $Z\subset {\mathbb R}$
, the set
$Z\subset {\mathbb R}$
, the set
![]() $h^{-1}(Z)$
is the union of those
$h^{-1}(Z)$
is the union of those
![]() $X_{n}$
such that
$X_{n}$
such that
![]() $\frac {1}{2^{n+1}}\in Z$
, i.e.,
$\frac {1}{2^{n+1}}\in Z$
, i.e.,
![]() $\textbf {F}_{\sigma }$
by definition.
$\textbf {F}_{\sigma }$
by definition.
We show in Section 3.5 that most of the above statements that are equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}$
, become provable in the much weaker system
$\mathsf {NIN}_{\mathsf {alt}}$
, become provable in the much weaker system
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
if we additionally require the functions to be Baire 1 as in Definition 1.8.
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
if we additionally require the functions to be Baire 1 as in Definition 1.8.
3.4 Bounded variation and the uncountability of
 ${\mathbb R}$
${\mathbb R}$
3.4.1 Introduction
In this section, we establish the equivalences sketched in Section 1.1 pertaining to the uncountability of
![]() ${\mathbb R}$
and properties of
${\mathbb R}$
and properties of
![]() $BV$
-functions. In particular, we study the following weakening of
$BV$
-functions. In particular, we study the following weakening of
![]() $\mathsf {NIN}_{\mathsf {alt}}$
involving the notion of height-width countability from Definition 2.6.
$\mathsf {NIN}_{\mathsf {alt}}$
involving the notion of height-width countability from Definition 2.6.
Principle 3.12 (
 $\mathsf {NIN}_{\mathsf {alt}}'$
)
$\mathsf {NIN}_{\mathsf {alt}}'$
)
The unit interval is not height–width countable.
Equivalences for
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
will involve some (restrictions of) items from Theorems 3.6 and 3.7, but also a number of theorems from analysis that hold for
$\mathsf {NIN}_{\mathsf {alt}}'$
will involve some (restrictions of) items from Theorems 3.6 and 3.7, but also a number of theorems from analysis that hold for
![]() $BV$
-functions and not for regulated ones. For the latter, we need some additional definitions, found in Section 3.4.2, while the equivalences are in Section 3.4.3
$BV$
-functions and not for regulated ones. For the latter, we need some additional definitions, found in Section 3.4.2, while the equivalences are in Section 3.4.3
Finally, we first establish Theorem 3.15, which is interesting because we are unable to derive
![]() $\mathsf {NIN}_{\mathsf {alt}}$
from the items listed therein. The exact definitions of
$\mathsf {NIN}_{\mathsf {alt}}$
from the items listed therein. The exact definitions of
![]() $\mathsf {HBU}$
and
$\mathsf {HBU}$
and
![]() $\mathsf {WHBU}$
are below, where the former expresses that the uncountable covering
$\mathsf {WHBU}$
are below, where the former expresses that the uncountable covering
![]() $\cup _{x\in [0,1]}B(x, \Psi (x))$
has a finite sub-covering, i.e., the Heine-Borel theorem or Cousin lemma [Reference Cousin21]. The principle
$\cup _{x\in [0,1]}B(x, \Psi (x))$
has a finite sub-covering, i.e., the Heine-Borel theorem or Cousin lemma [Reference Cousin21]. The principle
![]() $\mathsf {WHBU}$
is the combinatorial essence of the Vitali covering theorem, as studied in [Reference Normann and Sanders66].
$\mathsf {WHBU}$
is the combinatorial essence of the Vitali covering theorem, as studied in [Reference Normann and Sanders66].
Principle 3.13 (
 $\mathsf {HBU}$
[Reference Normann and Sanders65])
$\mathsf {HBU}$
[Reference Normann and Sanders65])
For any
![]() $ \Psi :{\mathbb R}\rightarrow {\mathbb R}^{+}$
, there are
$ \Psi :{\mathbb R}\rightarrow {\mathbb R}^{+}$
, there are
![]() $ y_{0}, \dots , y_{k}\in [0,1]$
such that
$ y_{0}, \dots , y_{k}\in [0,1]$
such that
![]() $ \cup _{i\leq k}B(y_{i}, \Psi (y_{i}))$
covers
$ \cup _{i\leq k}B(y_{i}, \Psi (y_{i}))$
covers
![]() $[0,1]$
.
$[0,1]$
.
Principle 3.14 (
 $\mathsf {WHBU}$
[Reference Normann and Sanders66])
$\mathsf {WHBU}$
[Reference Normann and Sanders66])
For any
![]() $\Psi :{\mathbb R}\rightarrow {\mathbb R}^{+} $
and
$\Psi :{\mathbb R}\rightarrow {\mathbb R}^{+} $
and
![]() $ \varepsilon>_{{\mathbb R}}0$
, there are
$ \varepsilon>_{{\mathbb R}}0$
, there are
![]() $ y_{0}, \dots , y_{k}\in [0,1]$
such that
$ y_{0}, \dots , y_{k}\in [0,1]$
such that
![]() $\cup _{i\leq k}B(y_{i}, \Psi (y_{i}))$
has measure at least
$\cup _{i\leq k}B(y_{i}, \Psi (y_{i}))$
has measure at least
![]() $1-\varepsilon $
.
$1-\varepsilon $
.
We note that
![]() $\mathsf {WHBU}$
can be formulated without using the Lebesgue measure, as discussed at length in e.g., [Reference Normann and Sanders66] or [Reference Simpson89, X.1]. We conjecture that
$\mathsf {WHBU}$
can be formulated without using the Lebesgue measure, as discussed at length in e.g., [Reference Normann and Sanders66] or [Reference Simpson89, X.1]. We conjecture that
![]() $\mathsf {NIN}_{\mathsf {alt}}$
is not provable in
$\mathsf {NIN}_{\mathsf {alt}}$
is not provable in
![]() $\mathsf {ACA}_{0}^{\omega }+ \mathsf {HBU}$
.
$\mathsf {ACA}_{0}^{\omega }+ \mathsf {HBU}$
.
Theorem 3.15 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
The following theorems imply
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
:
$\mathsf {NIN}_{\mathsf {alt}}'$
:
-
• Jordan decomposition theorem for
 $[0,1]$
,
$[0,1]$
, -
• the principle
 $\mathsf {HBU}$
restricted to
$\mathsf {HBU}$
restricted to
 $BV$
-functions,
$BV$
-functions, -
• the principle
 $\mathsf {WHBU}$
restricted to
$\mathsf {WHBU}$
restricted to
 $BV$
-functions.
$BV$
-functions.
Proof For the first item, let
![]() $(Y_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets with width bound
$(Y_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets with width bound
![]() $g\in {\mathbb N}^{{\mathbb N}}$
. The function
$g\in {\mathbb N}^{{\mathbb N}}$
. The function
![]() $k:[0,1]\rightarrow {\mathbb R}$
from (2.4) has bounded variation, with upper bound 1 by definition, for which we need
$k:[0,1]\rightarrow {\mathbb R}$
from (2.4) has bounded variation, with upper bound 1 by definition, for which we need
![]() $\mathsf {FIN}$
. By [Reference Normann and Sanders70, Lemma 7],
$\mathsf {FIN}$
. By [Reference Normann and Sanders70, Lemma 7],
![]() $\mu ^{2}$
can enumerate the points of discontinuity of a monotone function, i.e., the Jordan decomposition theorem provides a sequence
$\mu ^{2}$
can enumerate the points of discontinuity of a monotone function, i.e., the Jordan decomposition theorem provides a sequence
![]() $(x_{n})_{n\in {\mathbb N}}$
that enumerates the points of discontinuity of a
$(x_{n})_{n\in {\mathbb N}}$
that enumerates the points of discontinuity of a
![]() $BV$
-function. Using the usual diagonal argument (see, e.g., [Reference Simpson89, II.4.9]), we can find a point not in this sequence, yielding
$BV$
-function. Using the usual diagonal argument (see, e.g., [Reference Simpson89, II.4.9]), we can find a point not in this sequence, yielding
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
.
For the remaining items, let
![]() $(Y_{n})_{n\in {\mathbb N}}$
again be a sequence of finite sets with width bound
$(Y_{n})_{n\in {\mathbb N}}$
again be a sequence of finite sets with width bound
![]() $g\in {\mathbb N}^{{\mathbb N}}$
. Suppose
$g\in {\mathbb N}^{{\mathbb N}}$
. Suppose
![]() $[0,1]=\cup _{n\in {\mathbb N}}Y_{n}$
and define
$[0,1]=\cup _{n\in {\mathbb N}}Y_{n}$
and define
![]() $\Psi :[0,1]\rightarrow {\mathbb R}^{+}$
as follows
$\Psi :[0,1]\rightarrow {\mathbb R}^{+}$
as follows
![]() $\Psi (x):= \frac {1}{2^{n+5}}\frac {1}{g(n)+1}$
where
$\Psi (x):= \frac {1}{2^{n+5}}\frac {1}{g(n)+1}$
where
![]() $x\in Y_{n}$
and n is the least such number. For
$x\in Y_{n}$
and n is the least such number. For
![]() $x_{0}, \dots , x_{k}\in [0,1]$
, the measure of
$x_{0}, \dots , x_{k}\in [0,1]$
, the measure of
![]() $\cup _{i\leq k} B(x_{i}, \Psi (x_{i}))$
is at most
$\cup _{i\leq k} B(x_{i}, \Psi (x_{i}))$
is at most
![]() $1/2$
by construction, contradicting
$1/2$
by construction, contradicting
![]() $\mathsf {HBU}$
and
$\mathsf {HBU}$
and
![]() $\mathsf {WHBU}$
. Using
$\mathsf {WHBU}$
. Using
![]() $\mathsf {FIN}$
, one readily shows that
$\mathsf {FIN}$
, one readily shows that
![]() $\Psi $
is in
$\Psi $
is in
![]() $BV$
.
$BV$
.
The principle
![]() $\mathsf {HBU}$
is studied in [Reference Barrett5, Reference Barrett, Downey and Greenberg6] for
$\mathsf {HBU}$
is studied in [Reference Barrett5, Reference Barrett, Downey and Greenberg6] for
![]() $\Psi $
represented by, e.g., second-order Borel codes. This ‘coded’ version is provable in
$\Psi $
represented by, e.g., second-order Borel codes. This ‘coded’ version is provable in
![]() $\mathsf {ATR}_{0}$
extended with some induction. By contrast and Theorem 3.15,
$\mathsf {ATR}_{0}$
extended with some induction. By contrast and Theorem 3.15,
![]() $\mathsf {HBU}$
restricted to
$\mathsf {HBU}$
restricted to
![]() $BV$
-functions, which are definitely Borel, implies
$BV$
-functions, which are definitely Borel, implies
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
, which in turn is not provable in
$\mathsf {NIN}_{\mathsf {alt}}'$
, which in turn is not provable in
![]() $\mathsf {Z}_{2}^{\omega }$
. Thus, the use of codes fundamentally changes the logical strength of
$\mathsf {Z}_{2}^{\omega }$
. Thus, the use of codes fundamentally changes the logical strength of
![]() $\mathsf {HBU}$
. A similar argument can be made for the Jordan decomposition theorem, studied for second-order codes in [Reference Nies, Triplett and Yokoyama64].
$\mathsf {HBU}$
. A similar argument can be made for the Jordan decomposition theorem, studied for second-order codes in [Reference Nies, Triplett and Yokoyama64].
3.4.2 Definitions
We introduce some extra definitions needed for the RM-study of
![]() $BV$
-functions as in Section 3.4.3.
$BV$
-functions as in Section 3.4.3.
First of all, we shall study unordered sums, which are a device for bestowing meaning upon ‘uncountable sums’
![]() $\sum _{x\in I}f(x)$
for any index set I and
$\sum _{x\in I}f(x)$
for any index set I and
![]() $f:I\rightarrow {\mathbb R}$
. A central result is that if
$f:I\rightarrow {\mathbb R}$
. A central result is that if
![]() $\sum _{x\in I}f(x)$
somehow exists, it must be a ‘normal’ series of the form
$\sum _{x\in I}f(x)$
somehow exists, it must be a ‘normal’ series of the form
![]() $\sum _{i\in {\mathbb N}}f(y_{i})$
, i.e.,
$\sum _{i\in {\mathbb N}}f(y_{i})$
, i.e.,
![]() $f(x)=0$
for all but countably many
$f(x)=0$
for all but countably many
![]() $x\in [0,1]$
; Tao mentions this theorem in [Reference Tao94, p. xii].
$x\in [0,1]$
; Tao mentions this theorem in [Reference Tao94, p. xii].
By way of motivation, there is considerable historical and conceptual interest in this topic: Kelley notes in [Reference Kelley44, p. 64] that Moore’s study of unordered sums in [Reference Moore61] led to the concept of net with his student Smith [Reference Moore and Smith62]. Unordered sums can be found in (self-proclaimed) basic or applied textbooks [Reference Hunter and Nachtergaele39, Reference Sohrab90] and can be used to develop measure theory [Reference Kelley44, p. 79]. Moreover, Tukey shows in [Reference Tukey96] that topology can be developed using phalanxes, which are nets with the same index sets as unordered sums.
Now, unordered sums are just a special kind of net and
![]() $a:[0,1]\rightarrow {\mathbb R}$
is therefore written
$a:[0,1]\rightarrow {\mathbb R}$
is therefore written
![]() $(a_{x})_{x\in [0,1]} $
in this context to suggest the connection to nets. The associated notation
$(a_{x})_{x\in [0,1]} $
in this context to suggest the connection to nets. The associated notation
![]() $\sum _{x\in [0,1]}a_{x}$
is purely symbolic. We only need the following notions in the below. Let
$\sum _{x\in [0,1]}a_{x}$
is purely symbolic. We only need the following notions in the below. Let
![]() $\mathsf {fin}({\mathbb R})$
be the set of all finite sequences of reals without repetitions.
$\mathsf {fin}({\mathbb R})$
be the set of all finite sequences of reals without repetitions.
Definition 3.16. Let
![]() $a:[0,1]\rightarrow {\mathbb R}$
be any mapping, also denoted
$a:[0,1]\rightarrow {\mathbb R}$
be any mapping, also denoted
![]() $(a_{x})_{x\in [0,1]}$
.
$(a_{x})_{x\in [0,1]}$
.
-
• We say that
 $\sum _{x\in [0,1]} a_{x}$
is Cauchy if there is
$\sum _{x\in [0,1]} a_{x}$
is Cauchy if there is
 $\Phi :{\mathbb R}\rightarrow \mathsf {fin}({\mathbb R})$
such that for
$\Phi :{\mathbb R}\rightarrow \mathsf {fin}({\mathbb R})$
such that for
 $\varepsilon>0$
and all
$\varepsilon>0$
and all
 $J\in \mathsf {fin}({{\mathbb R}})$
with
$J\in \mathsf {fin}({{\mathbb R}})$
with
 $J\cap \Phi (\varepsilon )=\emptyset $
, we have
$J\cap \Phi (\varepsilon )=\emptyset $
, we have
 $|\sum _{x\in J}a_{x}|<\varepsilon $
.
$|\sum _{x\in J}a_{x}|<\varepsilon $
. -
• We say that
 $\sum _{x\in [0,1]}a_{x} $
is bounded if there is
$\sum _{x\in [0,1]}a_{x} $
is bounded if there is
 $N_{0}\in {\mathbb N}$
such that for any
$N_{0}\in {\mathbb N}$
such that for any
 $J\in \mathsf {fin}({\mathbb R})$
,
$J\in \mathsf {fin}({\mathbb R})$
,
 $N_{0}>|\sum _{x\in J}a_{x}|$
.
$N_{0}>|\sum _{x\in J}a_{x}|$
.
Note that in the first item,
![]() $\Phi $
is called a Cauchy modulus. For simplicity, we focus on positive unordered sums, i.e.,
$\Phi $
is called a Cauchy modulus. For simplicity, we focus on positive unordered sums, i.e.,
![]() $(a_{x})_{x\in [0,1]}$
such that
$(a_{x})_{x\in [0,1]}$
such that
![]() $a_{x}\geq 0$
for
$a_{x}\geq 0$
for
![]() $x\in [0,1]$
.
$x\in [0,1]$
.
Secondly, there are many spaces between the regulated and
![]() $BV$
-functions, as discussed in Remark 3.23. We shall study one particular construct, called Waterman variation, defined as follows.
$BV$
-functions, as discussed in Remark 3.23. We shall study one particular construct, called Waterman variation, defined as follows.
Definition 3.17. A decreasing sequence of positive reals
![]() $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
is a Waterman sequence if
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
is a Waterman sequence if
![]() $\lim _{n\rightarrow \infty }\lambda _{n}=0$
and
$\lim _{n\rightarrow \infty }\lambda _{n}=0$
and
![]() $\sum _{n=0}^{\infty }\lambda _{n}=\infty $
.
$\sum _{n=0}^{\infty }\lambda _{n}=\infty $
.
Definition 3.18 (Waterman variation)
The function
![]() $f:[a,b]\rightarrow {\mathbb R}$
has bounded Waterman variation with sequence
$f:[a,b]\rightarrow {\mathbb R}$
has bounded Waterman variation with sequence
![]() $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
on
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
on
![]() $[a,b]$
if there is
$[a,b]$
if there is
![]() $k_{0}\in {\mathbb N}$
such that
$k_{0}\in {\mathbb N}$
such that
![]() $k_{0}\geq \sum _{i=0}^{n} \lambda _{i} |f(x_{i})-f(x_{i+1})|$
for any finite collection of pairwise non-overlapping intervals
$k_{0}\geq \sum _{i=0}^{n} \lambda _{i} |f(x_{i})-f(x_{i+1})|$
for any finite collection of pairwise non-overlapping intervals
![]() $(x_{i}, x_{i+1})\subset [a,b]$
.
$(x_{i}, x_{i+1})\subset [a,b]$
.
Note that Definition 3.18 is equivalent to the ‘official’ definition of Waterman variation by [Reference Appell, Bana’s and Merentes1, Proposition 2.18]. In case
![]() $f:[0,1]\rightarrow {\mathbb R}$
has bounded Waterman variation (with sequence
$f:[0,1]\rightarrow {\mathbb R}$
has bounded Waterman variation (with sequence
![]() $\Lambda $
as in Definition 3.17), we write ‘
$\Lambda $
as in Definition 3.17), we write ‘
![]() $f\in \Lambda BV$
’.
$f\in \Lambda BV$
’.
Thirdly, we make use of the usual definitions of Fourier coefficients and series.
Definition 3.19. The Fourier series
![]() $S(f)(x)$
of
$S(f)(x)$
of
![]() $f:[-\pi , \pi ]\rightarrow {\mathbb R}$
at
$f:[-\pi , \pi ]\rightarrow {\mathbb R}$
at
![]() $x\in [-\pi , \pi ]$
is
$x\in [-\pi , \pi ]$
is
with Fourier coefficients
![]() $ a_{n} := \frac {1}{\pi }\int _{-\pi }^{\pi } f (t)\cos (nt)dt$
and
$ a_{n} := \frac {1}{\pi }\int _{-\pi }^{\pi } f (t)\cos (nt)dt$
and
![]() $ b_{n} :=\frac 1\pi \int _{-\pi }^{\pi } f (t) \sin (nt)dt$
.
$ b_{n} :=\frac 1\pi \int _{-\pi }^{\pi } f (t) \sin (nt)dt$
.
By the proof of Theorem 3.22, the Fourier coefficients and series of
![]() $k:[0,1]\rightarrow {\mathbb R}$
as in (2.2) all exist. Our study of the Fourier series
$k:[0,1]\rightarrow {\mathbb R}$
as in (2.2) all exist. Our study of the Fourier series
![]() $S(f)$
as in (3.3) will always assume (at least) that the Fourier coefficients of f exist. Functions of bounded variation are of course Riemann integrable and similarly have Fourier coefficients, but only in sufficiently strong systems that seem to dwarf
$S(f)$
as in (3.3) will always assume (at least) that the Fourier coefficients of f exist. Functions of bounded variation are of course Riemann integrable and similarly have Fourier coefficients, but only in sufficiently strong systems that seem to dwarf
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
.
3.4.3 Basic equivalences
We establish a number of equivalences for
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
and basic properties of
$\mathsf {NIN}_{\mathsf {alt}}'$
and basic properties of
![]() $BV$
-functions, some similar to those in Theorems 3.6 and 3.7, some new. We note that
$BV$
-functions, some similar to those in Theorems 3.6 and 3.7, some new. We note that
![]() $\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
is no longer needed in the base theory.
$\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
is no longer needed in the base theory.
Theorem 3.20 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
The following are equivalent.
-
(i) The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
. -
(ii) For a positive unordered sum
 $\sum _{x\in [0,1]}a_{x}$
with upper bound (or Cauchy modulus; Definition 3.16), there is
$\sum _{x\in [0,1]}a_{x}$
with upper bound (or Cauchy modulus; Definition 3.16), there is
 $y\in [0,1]$
such that
$y\in [0,1]$
such that
 $a_{y}=0$
.
$a_{y}=0$
. -
(iii) For a positive unordered sum
 $\sum _{x\in [0,1]}a_{x}$
with upper bound (or Cauchy modulus; Definition 3.16), the set
$\sum _{x\in [0,1]}a_{x}$
with upper bound (or Cauchy modulus; Definition 3.16), the set
 $\{ x\in [0,1]: a_{x}=0\}$
is dense (or: not height countable, or: not countable, or: not strongly countable).
$\{ x\in [0,1]: a_{x}=0\}$
is dense (or: not height countable, or: not countable, or: not strongly countable). -
(iv)-(xix) Any of items (b)–(d) from Theorem 3.6 or items (ii)–(xiv) from Theorem 3.7 restricted to
 $BV$
-functions.
$BV$
-functions. -
(xx) For a Waterman sequence
 $\Lambda $
and
$\Lambda $
and
 $f:[0,1]\rightarrow {\mathbb R}$
in
$f:[0,1]\rightarrow {\mathbb R}$
in
 $\Lambda BV$
, there is
$\Lambda BV$
, there is
 $y\in [0,1]$
where f is continuous.
$y\in [0,1]$
where f is continuous. -
(xxi)-(xxxvi) Any of items (b)–(d) from Theorem 3.6 or items (ii)–(xiv) from Theorem 3.7 restricted to
 $\Lambda BV$
for any fixed Waterman sequence
$\Lambda BV$
for any fixed Waterman sequence
 $\Lambda $
.
$\Lambda $
.
Proof The equivalences involving the restrictions from regulated to
![]() $BV$
functions follow from the proofs of Theorems 3.6 and 3.7. Indeed, for
$BV$
functions follow from the proofs of Theorems 3.6 and 3.7. Indeed, for
![]() $f\in BV$
with variation bounded by
$f\in BV$
with variation bounded by
![]() $1$
, the set
$1$
, the set
![]() $X_{n}$
from (3.2) has size bounded by
$X_{n}$
from (3.2) has size bounded by
![]() $2^{n}$
since each element of
$2^{n}$
since each element of
![]() $X_{n}$
contributes at least
$X_{n}$
contributes at least
![]() $1/2^{n}$
to the variation. Moreover, rather than
$1/2^{n}$
to the variation. Moreover, rather than
![]() $h:[0,1]\rightarrow {\mathbb R}$
as in (2.2), we use
$h:[0,1]\rightarrow {\mathbb R}$
as in (2.2), we use
![]() $k:[0,1]\rightarrow {\mathbb R}$
as in (2.4) which has variation bounded by
$k:[0,1]\rightarrow {\mathbb R}$
as in (2.4) which has variation bounded by
![]() $1$
if
$1$
if
![]() $(Y_{n})_{n\in {\mathbb N}}$
is a sequence of sets with width function
$(Y_{n})_{n\in {\mathbb N}}$
is a sequence of sets with width function
![]() $g\in {\mathbb N}^{{\mathbb N}}$
. The properties of
$g\in {\mathbb N}^{{\mathbb N}}$
. The properties of
![]() $k:[0,1]\rightarrow {\mathbb R}$
are readily proved using
$k:[0,1]\rightarrow {\mathbb R}$
are readily proved using
![]() $\mathsf {FIN}$
; in particular, the width function g obviates the use of
$\mathsf {FIN}$
; in particular, the width function g obviates the use of
![]() $\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
as in the proofs of Theorems 3.6 and 3.7. Hence, the equivalence between
$\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
as in the proofs of Theorems 3.6 and 3.7. Hence, the equivalence between
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
and items (iv)–(xix) has been established.
$\mathsf {NIN}_{\mathsf {alt}}'$
and items (iv)–(xix) has been established.
The equivalence between items (i) and (ii) is as follows: assume the latter and let
![]() $(X_{n})_{n\in {\mathbb N}}$
and
$(X_{n})_{n\in {\mathbb N}}$
and
![]() $g:{\mathbb N}\rightarrow {\mathbb N}$
be as in item (i). Define
$g:{\mathbb N}\rightarrow {\mathbb N}$
be as in item (i). Define
![]() $(a_{x})_{x\in [0,1]}$
as follows:
$(a_{x})_{x\in [0,1]}$
as follows:
 $$\begin{align*}a_{x}:= \begin{cases} 0, & x\not \in \cup_{n\in {\mathbb N}} X_{n}, \\ \frac{1}{2^{n}}\frac{1}{g(n)+1}, & x\in X_{n} \text{ and } n \text{ is the least such natural.} \end{cases} \end{align*}$$
$$\begin{align*}a_{x}:= \begin{cases} 0, & x\not \in \cup_{n\in {\mathbb N}} X_{n}, \\ \frac{1}{2^{n}}\frac{1}{g(n)+1}, & x\in X_{n} \text{ and } n \text{ is the least such natural.} \end{cases} \end{align*}$$
Clearly, this unordered sum is Cauchy and has upper bound
![]() $1$
; if
$1$
; if
![]() $y\in [0,1]$
is such that
$y\in [0,1]$
is such that
![]() $a_{y}=0$
, then
$a_{y}=0$
, then
![]() $y\not \in \cup _{n\in {\mathbb N}}X_{n}$
, as required for item (i). Now assume the latter and let
$y\not \in \cup _{n\in {\mathbb N}}X_{n}$
, as required for item (i). Now assume the latter and let
![]() $(a_{x})_{x\in [0,1]}$
be an unordered sum that is Cauchy. Now consider the following set:
$(a_{x})_{x\in [0,1]}$
be an unordered sum that is Cauchy. Now consider the following set:
Apply the Cauchy property of
![]() $(a_{x})_{x\in [0,1]}$
for
$(a_{x})_{x\in [0,1]}$
for
![]() $\varepsilon =1$
, yielding an upper bound
$\varepsilon =1$
, yielding an upper bound
![]() $N_{0}\in {\mathbb N}$
for
$N_{0}\in {\mathbb N}$
for
![]() $\sum _{x\in K}a_{x}$
for any
$\sum _{x\in K}a_{x}$
for any
![]() $K\in \mathsf {fin}{({\mathbb R})}$
. Hence, the finite set
$K\in \mathsf {fin}{({\mathbb R})}$
. Hence, the finite set
![]() $X_{n}$
in (3.4) has size at most
$X_{n}$
in (3.4) has size at most
![]() $2^{n}N_{0}$
. In this way, we have a width function for
$2^{n}N_{0}$
. In this way, we have a width function for
![]() $(X_{n})_{n\in {\mathbb N}}$
; any
$(X_{n})_{n\in {\mathbb N}}$
; any
![]() $y\in [0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
is such that
$y\in [0,1]\setminus \cup _{n\in {\mathbb N}}X_{n}$
is such that
![]() $a_{y}=0$
, as required for item (ii). Item (iii) now follows in the same way as for item (e) in the proof of Theorem 3.6.
$a_{y}=0$
, as required for item (ii). Item (iii) now follows in the same way as for item (e) in the proof of Theorem 3.6.
For item (xx), assume the latter and note that (3.5) establishes that
![]() $f\in BV$
implies
$f\in BV$
implies
![]() $f\in \Lambda BV$
for any Waterman sequence
$f\in \Lambda BV$
for any Waterman sequence
![]() $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
:
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
:
as Waterman sequences are decreasing by definition. Hence, item (i) readily follows from item (xx). Now assume item (i) and recall that for
![]() $BV$
-functions with variation bounded by
$BV$
-functions with variation bounded by
![]() $1$
, the set
$1$
, the set
![]() $X_{n}$
from (3.2) can have at most
$X_{n}$
from (3.2) can have at most
![]() $2^{n}$
elements, as each element of
$2^{n}$
elements, as each element of
![]() $X_{n}$
contributes at least
$X_{n}$
contributes at least
![]() $\frac {1}{2^{n}}$
to the total variation. Functions with Waterman variation bounded by
$\frac {1}{2^{n}}$
to the total variation. Functions with Waterman variation bounded by
![]() $1$
similarly come with explicit upper bounds on the set
$1$
similarly come with explicit upper bounds on the set
![]() $X_{n}$
, namely
$X_{n}$
, namely
![]() $|X_{n}|\leq K_{n}$
where
$|X_{n}|\leq K_{n}$
where
![]() $K_{n}$
is the least
$K_{n}$
is the least
![]() $k\in {\mathbb N}$
such that
$k\in {\mathbb N}$
such that
![]() $2^{n}<\sum _{m=0}^{k}\lambda _{m}$
. Hence, item (xx) follows and we are done.
$2^{n}<\sum _{m=0}^{k}\lambda _{m}$
. Hence, item (xx) follows and we are done.
Following the proof of Theorem 3.8, we observe that we may restrict to
![]() $BV$
-function that are Baire 2 or Baire 1
$BV$
-function that are Baire 2 or Baire 1
![]() $^{*}$
in the previous theorem. With the gift of hindsight we can even obtain the following corollary. We recall that the space of regulated functions is the union over all Waterman sequences
$^{*}$
in the previous theorem. With the gift of hindsight we can even obtain the following corollary. We recall that the space of regulated functions is the union over all Waterman sequences
![]() $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
of the spaces
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
of the spaces
![]() $\Lambda BV$
, as established in [Reference Appell, Bana’s and Merentes1, Proposition 2.24].
$\Lambda BV$
, as established in [Reference Appell, Bana’s and Merentes1, Proposition 2.24].
Corollary 3.21 (Some generalisations)
-
• We may replace ‘
 $f\in \Lambda BV$
’ in items (xx)–(xxxvi) of the theorem by ‘regulated function
$f\in \Lambda BV$
’ in items (xx)–(xxxvi) of the theorem by ‘regulated function
 $f:[0,1]\rightarrow {\mathbb R}$
with
$f:[0,1]\rightarrow {\mathbb R}$
with
 $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
such that
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
such that
 $f\in \Lambda BV$
’.
$f\in \Lambda BV$
’. -
• Assuming
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
, the higher item implies the lower one.
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
, the higher item implies the lower one.-
– For any regulated
 $f:[0,1]\rightarrow {\mathbb R}$
, there is a Waterman sequence
$f:[0,1]\rightarrow {\mathbb R}$
, there is a Waterman sequence
 $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
such that
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
such that
 $f\in \Lambda BV$
.
$f\in \Lambda BV$
. -
– The uniform finite union theorem.
-
Proof For the first item, the last part of the proof of the theorem provides the required upper bound function for applying
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
.
For the second item, let
![]() $(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets in
$(X_{n})_{n\in {\mathbb N}}$
be a sequence of finite sets in
![]() $[0,1]$
and consider the regulated function
$[0,1]$
and consider the regulated function
![]() $h:[0,1]\rightarrow {\mathbb R}$
as in (2.2). Suppose h is in
$h:[0,1]\rightarrow {\mathbb R}$
as in (2.2). Suppose h is in
![]() $\Lambda BV$
with
$\Lambda BV$
with
![]() $\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
and with upper bound
$\Lambda =(\lambda _{n})_{n\in {\mathbb N}}$
and with upper bound
![]() $N_{0}\in {\mathbb N}$
on the Waterman variation. Then
$N_{0}\in {\mathbb N}$
on the Waterman variation. Then
![]() $A_{n+2}$
as in (2.1) is
$A_{n+2}$
as in (2.1) is
![]() $\cup _{i\leq n}X_{i}$
for all
$\cup _{i\leq n}X_{i}$
for all
![]() $n\in {\mathbb N}$
and
$n\in {\mathbb N}$
and
![]() $|A_n| \leq K_{n}$
where
$|A_n| \leq K_{n}$
where
![]() $K_{n}$
is the least
$K_{n}$
is the least
![]() $k\in {\mathbb N}$
such that
$k\in {\mathbb N}$
such that
![]() $2^{n}N_{0}<\sum _{m=0}^{k}\lambda _{m}$
. The uniform finite union theorem thus follows.
$2^{n}N_{0}<\sum _{m=0}^{k}\lambda _{m}$
. The uniform finite union theorem thus follows.
An equivalence is possible in the second item, but the technical details are considerable. Our above results suggest that the principles equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
also have a certain robustness since we can replace ‘one point’ properties like item (ii) in Theorem 3.20, by, e.g., ‘density’ versions like item (iii) in Theorem 3.20. Nonetheless, we believe we cannot replace ‘density’ by ‘full measure’. In particular, we conjecture that ‘measure theoretic’ statements like
$\mathsf {NIN}_{\mathsf {alt}}'$
also have a certain robustness since we can replace ‘one point’ properties like item (ii) in Theorem 3.20, by, e.g., ‘density’ versions like item (iii) in Theorem 3.20. Nonetheless, we believe we cannot replace ‘density’ by ‘full measure’. In particular, we conjecture that ‘measure theoretic’ statements like
-
• a
 $BV$
-function is continuous (or differentiable) almost
$BV$
-function is continuous (or differentiable) almost
 $^{14}$
everywhere,
$^{14}$
everywhere, -
• a height–width countable set
 $A\subset [0,1]$
has measureFootnote
14
zero
$A\subset [0,1]$
has measureFootnote
14
zero
are strictly stronger than
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
. We do not have a proof of this claim.
$\mathsf {NIN}_{\mathsf {alt}}'$
. We do not have a proof of this claim.
Finally, the variation function
![]() $\lambda x. V_{a}^{x}(f)$
is defined in the obvious way, namely based on (1.2). This function shares pointwise properties like continuity and differentiability with
$\lambda x. V_{a}^{x}(f)$
is defined in the obvious way, namely based on (1.2). This function shares pointwise properties like continuity and differentiability with
![]() $f:[a,b]\rightarrow {\mathbb R}$
. For instance, the following equivalence for any
$f:[a,b]\rightarrow {\mathbb R}$
. For instance, the following equivalence for any
![]() $x\in [0,1)$
is obtained in [Reference Huggins37, Corollary 1.1]:
$x\in [0,1)$
is obtained in [Reference Huggins37, Corollary 1.1]:
Here, ‘right-continuous at
![]() $y\in [0,1)$
’ means
$y\in [0,1)$
’ means
![]() $g(y)=g(y+)$
. Now, although the variation function may not exist for
$g(y)=g(y+)$
. Now, although the variation function may not exist for
![]() $BV$
-functions, say in
$BV$
-functions, say in
![]() $\mathsf {RCA}_{0}^{\omega }$
, the right-hand side of (3.6) makes sense using the well-known ‘virtual’ or ‘comparative’ meaning of suprema from second-order RM (see [Reference Simpson89, X.1]). Perhaps surprisingly, working over
$\mathsf {RCA}_{0}^{\omega }$
, the right-hand side of (3.6) makes sense using the well-known ‘virtual’ or ‘comparative’ meaning of suprema from second-order RM (see [Reference Simpson89, X.1]). Perhaps surprisingly, working over
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}+\neg \mathsf {NIN}_{\mathsf {alt}}'$
, the function
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}+\neg \mathsf {NIN}_{\mathsf {alt}}'$
, the function
![]() $k:[0,1]\rightarrow {\mathbb R}$
from (2.4) satisfies
$k:[0,1]\rightarrow {\mathbb R}$
from (2.4) satisfies
which is to be interpreted in the aforementioned virtual sense. Thus, one readily proves that the following are equivalent, where
![]() $E(f, x)$
is (3.6).
$E(f, x)$
is (3.6).
-
• The uncountability of
 ${\mathbb R}$
as in
${\mathbb R}$
as in
 $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
. -
• For
 $f:[0,1]\rightarrow {\mathbb R}$
in
$f:[0,1]\rightarrow {\mathbb R}$
in
 $BV$
, there is
$BV$
, there is
 $y\in [0,1]$
where f is continuous.
$y\in [0,1]$
where f is continuous. -
• For
 $f:[0,1]\rightarrow {\mathbb R}$
in
$f:[0,1]\rightarrow {\mathbb R}$
in
 $BV$
, there is
$BV$
, there is
 $y\in [0,1]$
where
$y\in [0,1]$
where
 $E(f, y)$
holds.
$E(f, y)$
holds. -
• For
 $f:[0,1]\rightarrow {\mathbb R}$
in
$f:[0,1]\rightarrow {\mathbb R}$
in
 $BV$
such that
$BV$
such that
 $\lambda x.V_{0}^{x}(f)$
is right-continuous on
$\lambda x.V_{0}^{x}(f)$
is right-continuous on
 $[0,1)$
, there is
$[0,1)$
, there is
 $y\in [0,1]$
where f is right-continuous.
$y\in [0,1]$
where f is right-continuous.
To be absolutely clear, we think this topic should not be pursued further: mistakes are (too) easily made when dealing with ‘virtual’ objects like
![]() $\lambda x.V_{0}^{x}(f)$
.
$\lambda x.V_{0}^{x}(f)$
.
3.4.4 Advanced equivalences: Fourier series
We obtain an equivalence for
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
and properties of the Fourier series of
$\mathsf {NIN}_{\mathsf {alt}}'$
and properties of the Fourier series of
![]() $BV$
-functions. Since the forward direction is rather involved, we have reserved a separate section for this result. Moreover, Theorem 3.22 is not at all straightforward: Jordan proves the convergence of Fourier series for
$BV$
-functions. Since the forward direction is rather involved, we have reserved a separate section for this result. Moreover, Theorem 3.22 is not at all straightforward: Jordan proves the convergence of Fourier series for
![]() $BV$
-functions using the Jordan decomposition theorem, and the same for, e.g., [Reference Zygmund106, pp. 57–58]. However, the latter theorem seems much strongerFootnote
15
than
$BV$
-functions using the Jordan decomposition theorem, and the same for, e.g., [Reference Zygmund106, pp. 57–58]. However, the latter theorem seems much strongerFootnote
15
than
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
.
Theorem 3.22 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
The following are equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
.
$\mathsf {NIN}_{\mathsf {alt}}'$
.
-
• For
 $f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
$f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
 $BV$
such that the Fourier coefficients exist, there is
$BV$
such that the Fourier coefficients exist, there is
 $x_{0}\in (-\pi ,\pi )$
where the Fourier series
$x_{0}\in (-\pi ,\pi )$
where the Fourier series
 $S(f)(x_{0})$
equals
$S(f)(x_{0})$
equals
 $f(x_{0})$
.
$f(x_{0})$
. -
• For
 $f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
$f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
 $BV$
such that the Fourier coefficients exist, the set of
$BV$
such that the Fourier coefficients exist, the set of
 $x\in (-\pi , \pi )$
where the Fourier series
$x\in (-\pi , \pi )$
where the Fourier series
 $S(f)(x)$
equals
$S(f)(x)$
equals
 $f(x)$
, is dense (or: not height countable, or: not countable, or: not strongly countable).
$f(x)$
, is dense (or: not height countable, or: not countable, or: not strongly countable).
Proof Assume the first item and consider
![]() $k:[0,1]\rightarrow {\mathbb R}$
from (2.4). This function is Riemann integrable with
$k:[0,1]\rightarrow {\mathbb R}$
from (2.4). This function is Riemann integrable with
![]() $\int _{0}^{1}k(x)dx=0$
, which one proves in the same way as for
$\int _{0}^{1}k(x)dx=0$
, which one proves in the same way as for
![]() $h:[0,1]\rightarrow {\mathbb R}$
from (2.2) in the proof of Theorem 3.7. Similarly (and with a suitable rescaling), the Fourier coefficients of the Fourier series of k are zero. Hence, any
$h:[0,1]\rightarrow {\mathbb R}$
from (2.2) in the proof of Theorem 3.7. Similarly (and with a suitable rescaling), the Fourier coefficients of the Fourier series of k are zero. Hence, any
![]() $x_{0}$
where the Fourier series of k converges to
$x_{0}$
where the Fourier series of k converges to
![]() $k(x_{0})$
must be such that
$k(x_{0})$
must be such that
![]() $k(x_{0})=0$
, as required for
$k(x_{0})=0$
, as required for
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
since then
$\mathsf {NIN}_{\mathsf {alt}}'$
since then
![]() $x_{0}\not \in \cup _{n\in {\mathbb N}}X_{n}$
.
$x_{0}\not \in \cup _{n\in {\mathbb N}}X_{n}$
.
Secondly, by items (iv)–(xix) in Theorem 3.20,
![]() $\mathsf {NIN}_{\mathsf {alt}}'$
implies that for a
$\mathsf {NIN}_{\mathsf {alt}}'$
implies that for a
![]() $BV$
-function, the set
$BV$
-function, the set
![]() $C_{f}$
is dense (or: not height countable, or: not countable, or: not strongly countable). Hence, the second item from Theorem 3.22 is immediate if we can show that
$C_{f}$
is dense (or: not height countable, or: not countable, or: not strongly countable). Hence, the second item from Theorem 3.22 is immediate if we can show that
![]() $S(f)(x)$
from Definition 3.19 equals
$S(f)(x)$
from Definition 3.19 equals
![]() $\frac {f(x+)-f(x-)}{2}$
for f in
$\frac {f(x+)-f(x-)}{2}$
for f in
![]() $BV$
and any x in the domain. Waterman provides an elementary and almost self-contained proof of this convergence fact in [Reference Waterman101], avoiding the Jordan decomposition theorem and only citing [Reference Zygmund106, Vol. I, p. 55, (7.1)]. The proof of the latter is straightforward trigonometry and Waterman’s argument is readily formalised in
$BV$
and any x in the domain. Waterman provides an elementary and almost self-contained proof of this convergence fact in [Reference Waterman101], avoiding the Jordan decomposition theorem and only citing [Reference Zygmund106, Vol. I, p. 55, (7.1)]. The proof of the latter is straightforward trigonometry and Waterman’s argument is readily formalised in
![]() $\mathsf {ACA}_{0}^{\omega }$
. Similarly, there are ‘textbook’ proofs that
$\mathsf {ACA}_{0}^{\omega }$
. Similarly, there are ‘textbook’ proofs that
![]() $S(f)(x)$
equals
$S(f)(x)$
equals
![]() $\frac {f(x+)-f(x-)}{2}$
for f in
$\frac {f(x+)-f(x-)}{2}$
for f in
![]() $BV$
and x in the domain that avoid the Jordan decomposition theorem. Such proofs generally seem to proceed as follows (see, e.g., [Reference Körner51, Reference Whittaker and Watson102, Reference Zygmund106]).
$BV$
and x in the domain that avoid the Jordan decomposition theorem. Such proofs generally seem to proceed as follows (see, e.g., [Reference Körner51, Reference Whittaker and Watson102, Reference Zygmund106]).
-
• By Fejér’s theorem ([Reference Zygmund106, p. 89] or [Reference Whittaker and Watson102, p. 170]), Fourier series of
 $BV$
-functions are convergent in the Césaro mean to
$BV$
-functions are convergent in the Césaro mean to
 $\frac {f(x+)-f(x-)}{2}$
.
$\frac {f(x+)-f(x-)}{2}$
. -
• For
 $BV$
-functions, Fourier coefficients are
$BV$
-functions, Fourier coefficients are
 $O(\frac {1}{n})$
([Reference Whittaker and Watson102, p. 172], [Reference Zygmund106, p. 48]).
$O(\frac {1}{n})$
([Reference Whittaker and Watson102, p. 172], [Reference Zygmund106, p. 48]). -
• By Hardy’s theorem ([Reference Zygmund106, p. 78] or [Reference Whittaker and Watson102, p. 156]), if a series converges in the Césaro mean, it also converges in case the terms are
 $O(\frac {1}{n})$
.
$O(\frac {1}{n})$
. -
• By Césaro’s method of summation (see [Reference Whittaker and Watson102, p. 155]), if a series converges in the Césaro mean to a limit s and the series also converges, then the series converges to the limit s.
Each of these results has an elementary (sometimes tedious and lengthy) proof that readily formalises in
![]() $\mathsf {ACA}_{0}^{\omega }$
. As an example, the proofs of the second item in [Reference Whittaker and Watson102, p. 172] and [Reference Appell, Bana’s and Merentes1, p. 288] make use of the Jordan decomposition theorem, while the proofs in [Reference Taibleson92] and [Reference Zygmund106, p. 48] do not and are completely elementary. Finally, the perhaps ‘most elementary’ proof based on the above items is found in [Reference Körner51].
$\mathsf {ACA}_{0}^{\omega }$
. As an example, the proofs of the second item in [Reference Whittaker and Watson102, p. 172] and [Reference Appell, Bana’s and Merentes1, p. 288] make use of the Jordan decomposition theorem, while the proofs in [Reference Taibleson92] and [Reference Zygmund106, p. 48] do not and are completely elementary. Finally, the perhaps ‘most elementary’ proof based on the above items is found in [Reference Körner51].
Regarding the conditional nature of the items in Theorem 3.22, the Fourier coefficients of
![]() $BV$
-functions of course exist by the Lebesgue criterion for the Riemann integral. However, that
$BV$
-functions of course exist by the Lebesgue criterion for the Riemann integral. However, that
![]() $BV$
-functions have a point of continuity is already non-trivial by items (iv)–(xix) in Theorem 3.20. Moreover, the Darboux formulation of the Riemann integral involves suprema of
$BV$
-functions have a point of continuity is already non-trivial by items (iv)–(xix) in Theorem 3.20. Moreover, the Darboux formulation of the Riemann integral involves suprema of
![]() $BV$
-functions, which are hard to compute by the second cluster theorem in [Reference Normann and Sanders71].
$BV$
-functions, which are hard to compute by the second cluster theorem in [Reference Normann and Sanders71].
Finally, we could generalise the items from Theorems 3.20 and 3.22 to other notions of ‘generalised’ bounded variation. The latter notions yield (many) intermediate spaces between
![]() $BV$
and regulated as follows.
$BV$
and regulated as follows.
Remark 3.23 (Between bounded variation and regulated)
The following spaces are intermediate between
![]() $BV$
and regulated; all details may be found in [Reference Appell, Bana’s and Merentes1].
$BV$
and regulated; all details may be found in [Reference Appell, Bana’s and Merentes1].
Wiener spaces from mathematical physics [Reference Wiener103] are based on p-variation, which amounts to replacing ‘
![]() $ |f(x_{i})-f(x_{i+1})|$
’ by ‘
$ |f(x_{i})-f(x_{i+1})|$
’ by ‘
![]() $ |f(x_{i})-f(x_{i+1})|^{p}$
’ in the definition of variation (1.2). Young [Reference Young104] generalises this to
$ |f(x_{i})-f(x_{i+1})|^{p}$
’ in the definition of variation (1.2). Young [Reference Young104] generalises this to
![]() $\phi $
-variation which instead involves
$\phi $
-variation which instead involves
![]() $\phi ( |f(x_{i})-f(x_{i+1})|)$
for so-called Young functions
$\phi ( |f(x_{i})-f(x_{i+1})|)$
for so-called Young functions
![]() $\phi $
, yielding the Wiener–Young spaces. Perhaps a simpler construct is the Waterman variation [Reference Waterman100], which involves
$\phi $
, yielding the Wiener–Young spaces. Perhaps a simpler construct is the Waterman variation [Reference Waterman100], which involves
![]() $ \lambda _{i}|f(x_{i})-f(x_{i+1})|$
and where
$ \lambda _{i}|f(x_{i})-f(x_{i+1})|$
and where
![]() $(\lambda _{n})_{n\in {\mathbb N}}$
is a sequence of reals with nice properties; in contrast to
$(\lambda _{n})_{n\in {\mathbb N}}$
is a sequence of reals with nice properties; in contrast to
![]() $BV$
, any continuous function is included in the Waterman space [Reference Appell, Bana’s and Merentes1, Proposition 2.23]. Combining ideas from the above, the Schramm variation involves
$BV$
, any continuous function is included in the Waterman space [Reference Appell, Bana’s and Merentes1, Proposition 2.23]. Combining ideas from the above, the Schramm variation involves
![]() $\phi _{i}( |f(x_{i})-f(x_{i+1})|)$
for a sequence
$\phi _{i}( |f(x_{i})-f(x_{i+1})|)$
for a sequence
![]() $(\phi _{n})_{n\in {\mathbb N}}$
of well-behaved ‘gauge’ functions [Reference Schramm85]. As to generality, the union (resp. intersection) of all Schramm spaces yields the space of regulated (resp.
$(\phi _{n})_{n\in {\mathbb N}}$
of well-behaved ‘gauge’ functions [Reference Schramm85]. As to generality, the union (resp. intersection) of all Schramm spaces yields the space of regulated (resp.
![]() $BV$
) functions, while all other aforementioned spaces are Schramm spaces [Reference Appell, Bana’s and Merentes1, Propositions 2.43 and 2.46]. In contrast to
$BV$
) functions, while all other aforementioned spaces are Schramm spaces [Reference Appell, Bana’s and Merentes1, Propositions 2.43 and 2.46]. In contrast to
![]() $BV$
and the Jordan decomposition theorem, these generalised notions of variation have no known ‘nice’ decomposition theorem. The notion of Korenblum variation [Reference Korenblum50] does have such a theorem (see [Reference Appell, Bana’s and Merentes1, Proposition 2.68]) and involves a distortion function acting on the partition, not on the function values (see [Reference Appell, Bana’s and Merentes1, Definition 2.60]).
$BV$
and the Jordan decomposition theorem, these generalised notions of variation have no known ‘nice’ decomposition theorem. The notion of Korenblum variation [Reference Korenblum50] does have such a theorem (see [Reference Appell, Bana’s and Merentes1, Proposition 2.68]) and involves a distortion function acting on the partition, not on the function values (see [Reference Appell, Bana’s and Merentes1, Definition 2.60]).
It is no exaggeration to say that there are many natural spaces between the regulated and
![]() $BV$
-functions, all of which yield equivalences in Theorem 3.20.
$BV$
-functions, all of which yield equivalences in Theorem 3.20.
3.5 When more is less in Reverse Mathematics
An important-if not central-aspect of analysis is the relationship between its many function classes. It goes without saying that these relationships need not hold in weak logical systems. For instance, the well-known inclusion regulated implies Baire 1 is not provable in
![]() $\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
by [Reference Normann and Sanders72, Theorem 2.34].
$\mathsf {Z}_{2}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
by [Reference Normann and Sanders72, Theorem 2.34].
In this section, we establish a kind of dual to the previous negative result: we show that most of the above statements that are equivalent to
![]() $\mathsf {NIN}_{\mathsf {alt}}$
or
$\mathsf {NIN}_{\mathsf {alt}}$
or
![]() $\mathsf {NIN}_{\mathsf {alt}'}$
, become provable in the much weaker system
$\mathsf {NIN}_{\mathsf {alt}'}$
, become provable in the much weaker system
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
if we additionally require the functions to be Baire 1. To this end, we need the following theorem, where a jump continuity is any
$\mathsf {ACA}_{0}^{\omega }+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}+\mathsf {FIN}$
if we additionally require the functions to be Baire 1. To this end, we need the following theorem, where a jump continuity is any
![]() $x\in (0,1)$
such that
$x\in (0,1)$
such that
![]() $f(x+)\ne f(x-)$
.
$f(x+)\ne f(x-)$
.
Theorem 3.24 (
 $\mathsf {ACA}_{0}^{\omega }$
)
$\mathsf {ACA}_{0}^{\omega }$
)
If
![]() $f:[0,1]\rightarrow {\mathbb R}$
is regulated, there is a sequence of reals containing all jump discontinuities of f.
$f:[0,1]\rightarrow {\mathbb R}$
is regulated, there is a sequence of reals containing all jump discontinuities of f.
Proof This is immediate from [Reference Normann and Sanders72, Theorem 2.16].
The following theorem should be contrasted with Theorems 3.8 and 3.20.
Theorem 3.25 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
For a Baire 1 function
![]() $f:[0,1]\rightarrow {\mathbb R}$
in
$f:[0,1]\rightarrow {\mathbb R}$
in
![]() $BV$
, the points of continuity of f are dense.
$BV$
, the points of continuity of f are dense.
Proof Let
![]() $f:[0,1]\rightarrow {\mathbb R}$
be Baire
$f:[0,1]\rightarrow {\mathbb R}$
be Baire
![]() $1$
and in
$1$
and in
![]() $BV$
with variation bounded by
$BV$
with variation bounded by
![]() $1$
. This function is regulated by Theorem 3.3. Use Theorem 3.24 to enumerate the jump discontinuities of f as
$1$
. This function is regulated by Theorem 3.3. Use Theorem 3.24 to enumerate the jump discontinuities of f as
![]() $(y_{n})_{n\in {\mathbb N}}$
. Let
$(y_{n})_{n\in {\mathbb N}}$
. Let
![]() $(f_{n})_{n\in {\mathbb N}}$
be a sequence of continuous functions with pointwise limit f on
$(f_{n})_{n\in {\mathbb N}}$
be a sequence of continuous functions with pointwise limit f on
![]() $[0,1]$
and consider the following formula:
$[0,1]$
and consider the following formula:
For fixed
![]() $k\in {\mathbb N}$
,
$k\in {\mathbb N}$
,
![]() $\varphi (n_{0}, k, x)$
holds for large enough
$\varphi (n_{0}, k, x)$
holds for large enough
![]() $n_{0}\in {\mathbb N}$
in case f has a removable discontinuity at
$n_{0}\in {\mathbb N}$
in case f has a removable discontinuity at
![]() $x\in (0,1)$
such that
$x\in (0,1)$
such that
![]() $|f(x)-f(x+)|>\frac 1{2^{k}}$
. For fixed
$|f(x)-f(x+)|>\frac 1{2^{k}}$
. For fixed
![]() $n_{0},k\in {\mathbb N}$
, there can only be
$n_{0},k\in {\mathbb N}$
, there can only be
![]() $2^{k}$
many pairwise distinct
$2^{k}$
many pairwise distinct
![]() $x\in [0,1]$
such that
$x\in [0,1]$
such that
![]() $\varphi (n_{0}, k, x)$
, as each such real contributes at least
$\varphi (n_{0}, k, x)$
, as each such real contributes at least
![]() $\frac 1{2^{k}}$
to the total variation of f.
$\frac 1{2^{k}}$
to the total variation of f.
Next, the formula
![]() $\varphi (n_{0}, k, x)$
is equivalent to (second-order)
$\varphi (n_{0}, k, x)$
is equivalent to (second-order)
![]() $\Pi _{1}^{0}$
as f only occurs with rational input and
$\Pi _{1}^{0}$
as f only occurs with rational input and
![]() $f_{n}$
can be replaced uniformly by a sequence of codes
$f_{n}$
can be replaced uniformly by a sequence of codes
![]() $\Phi _{n}$
. Moreover, in case
$\Phi _{n}$
. Moreover, in case
![]() $x=_{{\mathbb R}} y$
, then trivially
$x=_{{\mathbb R}} y$
, then trivially
![]() $\varphi (n_{0}, k, x)\leftrightarrow \varphi (n_{0}, k, y)$
, i.e., we have the extensionality property required for [Reference Simpson89, II.5.7]. By the latter there is an RM-code of a closed set
$\varphi (n_{0}, k, x)\leftrightarrow \varphi (n_{0}, k, y)$
, i.e., we have the extensionality property required for [Reference Simpson89, II.5.7]. By the latter there is an RM-code of a closed set
![]() $C_{n_{0},k}$
such that
$C_{n_{0},k}$
such that
![]() $x\in C_{n_{0},k}\leftrightarrow \varphi (n_{0}, k, x)$
for all
$x\in C_{n_{0},k}\leftrightarrow \varphi (n_{0}, k, x)$
for all
![]() $x\in {\mathbb R}$
and
$x\in {\mathbb R}$
and
![]() $n_{0}, k\in {\mathbb N}$
. Since
$n_{0}, k\in {\mathbb N}$
. Since
![]() $C_{n_{0}, k}$
is finite,
$C_{n_{0}, k}$
is finite,
![]() $O_{n_{0}, k}:= [0,1]\setminus \big (C_{n_{0}, k}\cup \{y_{0}, \dots , y_{k}\} \big )$
is open and dense. By the Baire category theorem for RM-codes [Reference Simpson89, II.5.8], the intersection
$O_{n_{0}, k}:= [0,1]\setminus \big (C_{n_{0}, k}\cup \{y_{0}, \dots , y_{k}\} \big )$
is open and dense. By the Baire category theorem for RM-codes [Reference Simpson89, II.5.8], the intersection
![]() $\cap _{n_{0}, k\in {\mathbb N}} O_{n_{0}, k} $
is dense in
$\cap _{n_{0}, k\in {\mathbb N}} O_{n_{0}, k} $
is dense in
![]() $[0,1]$
. By definition, this intersection does not contain any points of discontinuity of f, and we are done.
$[0,1]$
. By definition, this intersection does not contain any points of discontinuity of f, and we are done.
The following corollary should be contrasted with Theorem 3.22.
Corollary 3.26 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}$
)
-
• For a positive unordered sum
 $\sum _{x\in [0,1]}a_{x}$
with upper bound and where
$\sum _{x\in [0,1]}a_{x}$
with upper bound and where
 $(a_{x})_{x\in [0,1]}$
is Baire 1, the set
$(a_{x})_{x\in [0,1]}$
is Baire 1, the set
 $\{y\in [0,1]:a_{y}=0\}$
is dense.
$\{y\in [0,1]:a_{y}=0\}$
is dense. -
• For Baire 1 function
 $f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
$f:[-\pi ,\pi ]\rightarrow {\mathbb R}$
in
 $BV$
such that the Fourier coefficients exist, the set
$BV$
such that the Fourier coefficients exist, the set
 $\{x\in (-\pi , \pi ): S(f)(x)=f(x)\}$
, is dense.
$\{x\in (-\pi , \pi ): S(f)(x)=f(x)\}$
, is dense.
Proof Let
![]() $(a_{x})_{x\in [0,1]}$
be an unordered sum with upper bound
$(a_{x})_{x\in [0,1]}$
be an unordered sum with upper bound
![]() $1$
. Now consider
$1$
. Now consider
which can have at most
![]() $2^{n}$
elements. As in the proof of the theorem, the Baire 1 approximation of
$2^{n}$
elements. As in the proof of the theorem, the Baire 1 approximation of
![]() $(a_{x})_{x\in [0,1]}$
allows us to show that
$(a_{x})_{x\in [0,1]}$
allows us to show that
![]() $a_{x}>1/2^{n}$
is (implied by) a suitable (second-order)
$a_{x}>1/2^{n}$
is (implied by) a suitable (second-order)
![]() $\Sigma _{1}^{0}$
-formula. One then uses the (second-order) Baire category theorem to show that
$\Sigma _{1}^{0}$
-formula. One then uses the (second-order) Baire category theorem to show that
![]() $y\in [0,1]$
such that
$y\in [0,1]$
such that
![]() $a_{y}=0$
are dense.
$a_{y}=0$
are dense.
For the second item, following the proof of Theorem 3.22,
![]() $f(x)=S(f)(x)$
holds in case f is continuous at
$f(x)=S(f)(x)$
holds in case f is continuous at
![]() $x\in [0,1]$
, where the latter is provided by the theorem.
$x\in [0,1]$
, where the latter is provided by the theorem.
Next, the first item in Theorem 3.27 follows from [Reference Normann and Sanders72, Theorem 2.26], but the latter is proved using
![]() $\mathsf {ACA}_{0}^{\omega }+\mathsf {ATR}_{0}$
.
$\mathsf {ACA}_{0}^{\omega }+\mathsf {ATR}_{0}$
.
Theorem 3.27 (
 $\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
)
$\mathsf {ACA}_{0}^{\omega }+\mathsf {FIN}+\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
)
-
• For regulated Baire 1
 $f:[0,1]\rightarrow {\mathbb R}$
, the points of continuity of f are dense.
$f:[0,1]\rightarrow {\mathbb R}$
, the points of continuity of f are dense. -
• Volterra’s corollary: there is no regulated and Baire 1 function that is continuous on
 ${\mathbb Q}\cap [0,1]$
and discontinuous on
${\mathbb Q}\cap [0,1]$
and discontinuous on
 $[0,1]\setminus {\mathbb Q}$
.
$[0,1]\setminus {\mathbb Q}$
. -
• For a Riemann integrable and regulated
 $f:[0,1]\rightarrow [0,1]$
in Baire 1 with
$f:[0,1]\rightarrow [0,1]$
in Baire 1 with
 $\int _{0}^{1}f(x)dx=0$
, the set
$\int _{0}^{1}f(x)dx=0$
, the set
 $\{x\in [0,1]:f(x)=0\}$
is dense.
$\{x\in [0,1]:f(x)=0\}$
is dense. -
• Blumberg’s theorem [Reference Blumberg8] restricted to regulated Baire 1 functions on
 $[0,1]$
.
$[0,1]$
.
Proof For the first item, the proof of Theorem 3.25 can be modified follows: for regulated f and fixed
![]() $k\in {\mathbb N}$
, there can be at most finitely many
$k\in {\mathbb N}$
, there can be at most finitely many
![]() $x\in [0,1]$
such that
$x\in [0,1]$
such that
![]() $|f(x)-f(x+)|>\frac 1{2^{k}}$
. One proves this fact by contradiction (as in the proof of Theorem 3.6), where
$|f(x)-f(x+)|>\frac 1{2^{k}}$
. One proves this fact by contradiction (as in the proof of Theorem 3.6), where
![]() $\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
provides a sequence
$\mathsf {QF}\text{-}\mathsf {AC}^{0,1}$
provides a sequence
![]() $(x_{n})_{n\in {\mathbb N}}$
of reals in
$(x_{n})_{n\in {\mathbb N}}$
of reals in
![]() $ [0,1]$
such that
$ [0,1]$
such that
![]() $|f(x_{n})-f(x_{n}+)|>\frac 1{2^{k}}$
for all
$|f(x_{n})-f(x_{n}+)|>\frac 1{2^{k}}$
for all
![]() $n\in {\mathbb N}$
. This sequence has a convergent sub-sequence, say with limit
$n\in {\mathbb N}$
. This sequence has a convergent sub-sequence, say with limit
![]() $y\in [0,1]$
; one readily verifies that
$y\in [0,1]$
; one readily verifies that
![]() $f(y+)$
or
$f(y+)$
or
![]() $f(y-)$
does not exist. The rest of the proof is now identical to that of Theorem 3.25.
$f(y-)$
does not exist. The rest of the proof is now identical to that of Theorem 3.25.
For the second item, the proof of Theorem 3.25 is readily adapted as follows: let
![]() $(q_{n})_{n\in {\mathbb N}}$
be an enumeration of the rationals in
$(q_{n})_{n\in {\mathbb N}}$
be an enumeration of the rationals in
![]() $[0,1]$
and define
$[0,1]$
and define
![]() $O_{n_{0}, k}'$
as
$O_{n_{0}, k}'$
as
![]() $O_{n_{0},k}\setminus \{q_{0}, \dots , q_{k}\}$
. Then Theorem 3.25 must yield an irrational point of continuity, a contradiction.
$O_{n_{0},k}\setminus \{q_{0}, \dots , q_{k}\}$
. Then Theorem 3.25 must yield an irrational point of continuity, a contradiction.
For the third item, note that
![]() $f(x)=0$
must hold in case f is continuous at
$f(x)=0$
must hold in case f is continuous at
![]() $x\in [0,1]$
, where the (dense set of the) latter is provided by the first item.
$x\in [0,1]$
, where the (dense set of the) latter is provided by the first item.
For the fourth item, this immediately follows from the first item.
We could obtain similar results for most items of Theorem 3.7 and related theorems. We could also replace ‘Baire 1’ by ‘effectively Baire 2’ in Theorem 3.8 where the latter means that the function is given as the pointwise iterated limit of a double sequence of continuous functions; however, this would require us to go up to at least
![]() $\mathsf {ATR}_{0}$
, as suggested by the results in [Reference Normann and Sanders72, Section 2.6].
$\mathsf {ATR}_{0}$
, as suggested by the results in [Reference Normann and Sanders72, Section 2.6].
Acknowledgements
We thank Anil Nerode and Chris Impens for their valuable advice. We also thank the anonymous referee for the helpful suggestions.
Funding
Our research was supported by the Deutsche Forschungsgemeinschaft via the DFG grant SA3418/1-1 and the Klaus Tschira Boost Fund via the grant Projekt KT43. Some results were obtained during the stimulating Workshop on Reverse Mathematics and Philosophy in June 2022 in Paris, sponsored by the NSF FRG grant Collaborative Research: Computability Theoretic Aspects of Combinatorics. We express our gratitude towards the aforementioned institutions.