Hostname: page-component-848d4c4894-wzw2p Total loading time: 0 Render date: 2024-05-04T14:26:06.245Z Has data issue: false hasContentIssue false
  • English
  • Français

MINIMALITY AND MUTATION-EQUIVALENCE OF POLYGONS

Published online by Cambridge University Press:  15 August 2017

ALEXANDER KASPRZYK ,
BENJAMIN NILL  and
THOMAS PRINCE
ALEXANDER KASPRZYK
Affiliation:
School of Mathematical Sciences, University of Nottingham, University Park, Nottingham NG7 2RD, UK; a.m.kasprzyk@nottingham.ac.uk
BENJAMIN NILL
Affiliation:
Fakultät für Mathematik, Otto-von-Guericke-Universität Magdeburg, Postschließfach 4120, 39016 Magdeburg, Germany; benjamin.nill@ovgu.de
THOMAS PRINCE
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen’s Gate, London SW7 2AZ, UK; t.prince12@imperial.ac.uk

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We introduce a concept of minimality for Fano polygons. We show that, up to mutation, there are only finitely many Fano polygons with given singularity content, and give an algorithm to determine representatives for all mutation-equivalence classes of such polygons. This is a key step in a program to classify orbifold del Pezzo surfaces using mirror symmetry. As an application, we classify all Fano polygons such that the corresponding toric surface is qG-deformation-equivalent to either (i) a smooth surface; or (ii) a surface with only singularities of type 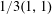$1/3(1,1)$.

Type
Research Article
Information
Forum of Mathematics, Sigma , Volume 5 , 2017 , e18
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2017

References

Akhtar, M., Coates, T., Corti, A., Heuberger, L., Kasprzyk, A. M., Oneto, A., Petracci, A., Prince, T. and Tveiten, K., ‘Mirror symmetry and the classification of orbifold del Pezzo surfaces’, Proc. Amer. Math. Soc. 144(2) (2016), 513–527.Google Scholar
Akhtar, M., Coates, T., Galkin, S. and Kasprzyk, A. M., ‘Minkowski polynomials and mutations’, SIGMA Symmetry Integrability Geom. Methods Appl. 8 (2012), Paper 094, 17.Google Scholar
Akhtar, M. and Kasprzyk, A. M., ‘Singularity content’, Preprint (2014), arXiv:1401.5458 [math.AG].Google Scholar
Akhtar, M. and Kasprzyk, A. M., ‘Mutations of fake weighted projective planes’, Proc. Edinb. Math. Soc. (2) 59(2) (2016), 271–285.CrossRefGoogle Scholar
Auroux, D., ‘Mirror symmetry and T-duality in the complement of an anticanonical divisor’, J. Gökova Geom. Topol. GGT 1 (2007), 51–91.Google Scholar
Averkov, G., Krümpelmann, J. and Nill, B., ‘Largest integral simplices with one interior integral point: solution of Hensley’s conjecture and related results’, Adv. Math. 274 (2015), 118–166.CrossRefGoogle Scholar
Batyrev, V. V., ‘Higher dimensional toric varieties with ample anticanonical class’, PhD Thesis, Moscow State University, 1985, Text in Russian.Google Scholar
Batyrev, V. V., ‘Toric degenerations of Fano varieties and constructing mirror manifolds’, inThe Fano Conference (Univ. Torino, Turin, 2004), 109–122.Google Scholar
Bernšteĭn, I. N., Gel’fand, I. M. and Ponomarev, V. A.,‘Coxeter functors, and Gabriel’s theorem’, Uspekhi Mat. Nauk 28(2(170)) (1973), 19–33.Google Scholar
Brown, G. and Kasprzyk, A. M., The graded ring database, online database, http://www.grdb.co.uk/.Google Scholar
Coates, T., Corti, A., Galkin, S., Golyshev, V. and Kasprzyk, A. M., ‘Mirror symmetry and Fano manifolds’, inEuropean Congress of Mathematics Kraków, 2–7 July, 2012 (Eur. Math. Soc., Zürich, 2014), 285–300.Google Scholar
Coates, T., Corti, A., Galkin, S. and Kasprzyk, A., ‘Quantum periods for 3-dimensional Fano manifolds’, Geom. Topol. 20(1) (2016), 103–256.Google Scholar
Coates, T., Kasprzyk, A. M. and Prince, T., ‘Laurent inversion’, Preprint (2017), arXiv:1707.05842 [math.AG].Google Scholar
Corti, A. and Heuberger, L., ‘Del Pezzo surfaces with [[()[]mml:mfrac[]()]][[()[]mml:mrow []()]]1[[()[]/mml:mrow[]()]] [[()[]mml:mrow []()]]3[[()[]/mml:mrow[]()]][[()[]/mml:mfrac[]()]](1, 1) points’, Manuscripta Math. 153(1–2) (2017), 71–118.Google Scholar
Dais, D. I., ‘Classification of toric log del Pezzo surfaces having Picard number 1 and index ⩽3’, Results Math. 54(3–4) (2009), 219–252.CrossRefGoogle Scholar
Fock, V. V. and Goncharov, A. B., ‘Cluster ensembles, quantization and the dilogarithm. II. The intertwiner’, inAlgebra, Arithmetic, and Geometry: in Honor of Yu. I. Manin., Vol. I, Progress in Mathematics, 269 (Birkhäuser Boston Inc., Boston, MA, 2009), 655–673.CrossRefGoogle Scholar
Fomin, S. and Zelevinsky, A., ‘Cluster algebras. I. Foundations’, J. Amer. Math. Soc. 15(2) (2002), 497–529. (electronic).CrossRefGoogle Scholar
Fulton, W., Introduction to Toric Varieties, Annals of Mathematics Studies, 131 (Princeton University Press, Princeton, NJ, 1993), The William H. Roever Lectures in Geometry.Google Scholar
Galkin, S. and Usnich, A., ‘Laurent phenomenon for Ginzburg-Landau potential’, IPMU Preprint 10-0100, 2010.Google Scholar
Gross, M., Hacking, P. and Keel, S., ‘Birational geometry of cluster algebras’, Preprint (2013), arXiv:1309.2573 [math.AG].Google Scholar
Gross, M., Hacking, P. and Keel, S., ‘Mirror symmetry for log Calabi–Yau surfaces I’, Publ. Math. Inst. Hautes Études Sci. 122 (2015), 65–168.Google Scholar
Gross, M. and Siebert, B., ‘From real affine geometry to complex geometry’, Ann. of Math. (2) 174(3) (2011), 1301–1428.CrossRefGoogle Scholar
Gross, M. and Siebert, B., ‘An invitation to toric degenerations’, inSurveys in Differential Geometry, Vol. XVI, Geometry of Special Holonomy and Related Topics, Surveys in Differential Geometry, 16 (Int. Press, Somerville, MA, 2011), 43–78.Google Scholar
Haase, C. and Schicho, J., ‘Lattice polygons and the number 2i + 7’, Amer. Math. Monthly 116(2) (2009), 151–165.Google Scholar
Hacking, P. and Prokhorov, Y., ‘Smoothable del Pezzo surfaces with quotient singularities’, Compos. Math. 146(1) (2010), 169–192.Google Scholar
Ilten, N. O., ‘Mutations of Laurent polynomials and flat families with toric fibers’, SIGMA Symmetry Integrability Geom. Methods Appl. 8 (2012), 47–53.Google Scholar
Kasprzyk, A. M., ‘Bounds on fake weighted projective space’, Kodai Math. J. 32 (2009), 197–208.Google Scholar
Kasprzyk, A. M., Kreuzer, M. and Nill, B., ‘On the combinatorial classification of toric log del Pezzo surfaces’, LMS J. Comput. Math. 13 (2010), 33–46.Google Scholar
Kollár, J. and Shepherd–Barron, N. I., ‘Threefolds and deformations of surface singularities’, Invent. Math. 91(2) (1988), 299–338.Google Scholar
Kontsevich, M. and Soibelman, Y., ‘Affine structures and non-Archimedean analytic spaces’, inThe Unity of Mathematics, Progress in Mathematics, 244 (Birkhäuser Boston, Boston, MA, 2006), 321–385.Google Scholar
Lagarias, J. C. and Ziegler, G. M., ‘Bounds for lattice polytopes containing a fixed number of interior points in a sublattice’, Canad. J. Math. 43(5) (1991), 1022–1035.CrossRefGoogle Scholar
Mahler, K., ‘Ein Übertragungsprinzip für konvexe Körper’, Časopis Pěst. Mat. Fys. 68 (1939), 93–102.Google Scholar
Mandel, T., ‘Classification of rank 2 cluster varieties’, Preprint (2014), arXiv:1407.6241 [math.AG].Google Scholar
Perling, M., ‘Combinatorial aspects of exceptional sequences on (rational) surfaces’, Preprint (2013), arXiv:1311.7349 [math.AG].Google Scholar
Pick, G. A., ‘Geometrisches zur zahlenlehre’, Sitzungber Lotos 19 (1899), 311–319.Google Scholar
Prince, T., ‘Smoothing toric Fano surfaces using the Gross–Siebert algorithm’, Preprint (2015), arXiv:1504.05969 [math.AG].Google Scholar
Rabinowitz, S., ‘A census of convex lattice polygons with at most one interior lattice point’, Ars Combin. 28 (1989), 83–96.Google Scholar
Reid, M., ‘Young person’s guide to canonical singularities’, inAlgebraic Geometry, Bowdoin, 1985 (Brunswick, Maine, 1985), Proceedings of Symposia in Pure Mathematics, 46 (American Mathematical Society, Providence, RI, 1987), 345–414.Google Scholar
Scott, P. R., ‘On convex lattice polygons’, Bull. Aust. Math. Soc. 15(3) (1976), 395–399.Google Scholar
Symington, M., ‘Four dimensions from two in symplectic topology’, inTopology and Geometry of Manifolds (Athens, GA, 2001), Proceedings of Symposia in Pure Mathematics, 71 (American Mathematical Society, Providence, RI, 2003), 153–208.Google Scholar
Wahl, J. M., ‘Elliptic deformations of minimally elliptic singularities’, Math. Ann. 253(3) (1980), 241–262.CrossRefGoogle Scholar