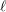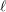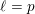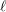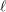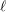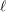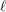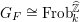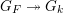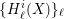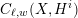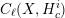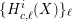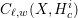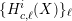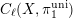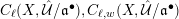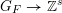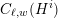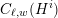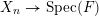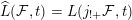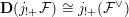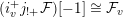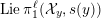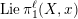1 Introduction
One intriguing aspect of the proof of the Weil conjectures by Grothendieck and his school, via the theory of étale cohomology, is in the need to choose an auxiliary prime
![]() $\ell$
; the field
$\ell$
; the field
![]() $\mathbb{Q}_{\ell }$
of
$\mathbb{Q}_{\ell }$
of
![]() $\ell$
-adic numbers then providing the coefficient field for a Weil cohomology theory. (To fix terms, let
$\ell$
-adic numbers then providing the coefficient field for a Weil cohomology theory. (To fix terms, let
![]() $F$
be a field,
$F$
be a field,
![]() $X/F$
an algebraic variety,
$X/F$
an algebraic variety,
![]() $F^{\text{sep}}$
a separable closure and
$F^{\text{sep}}$
a separable closure and
![]() $G_{F}$
the corresponding absolute Galois group.) While a priori it is not remotely clear how the cohomology groups
$G_{F}$
the corresponding absolute Galois group.) While a priori it is not remotely clear how the cohomology groups
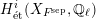 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
(considered as representations of
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
(considered as representations of
![]() $G_{F}$
) are related for different values of
$G_{F}$
) are related for different values of
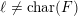 $\ell \neq \operatorname{char}(F)$
, the Riemann hypothesis [Reference DeligneDel74] shows that, at least over finite fields, they essentially contain the same information. Indeed, for curves
$\ell \neq \operatorname{char}(F)$
, the Riemann hypothesis [Reference DeligneDel74] shows that, at least over finite fields, they essentially contain the same information. Indeed, for curves
![]() $C$
(and again
$C$
(and again
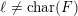 $\ell \neq \operatorname{char}(F)$
) one essentially has
$\ell \neq \operatorname{char}(F)$
) one essentially has
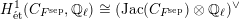 $H_{\acute{\text{e}}\text{t}}^{1}(C_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong (\operatorname{Jac}(C_{F^{\text{sep}}})\otimes \mathbb{Q}_{\ell })^{\vee }$
(again as Galois representations),
$H_{\acute{\text{e}}\text{t}}^{1}(C_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong (\operatorname{Jac}(C_{F^{\text{sep}}})\otimes \mathbb{Q}_{\ell })^{\vee }$
(again as Galois representations),
![]() $H^{1}$
being the only group of real interest in this case.
$H^{1}$
being the only group of real interest in this case.
To account for this plethora of different but subtly related cohomology groups, Grothendieck proposed the theory of pure motives, which for smooth and proper varieties at least should provide some form of algebro-geometric object
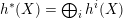 $h^{\ast }(X)=\bigoplus _{i}h^{i}(X)$
such that
$h^{\ast }(X)=\bigoplus _{i}h^{i}(X)$
such that
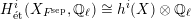 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong h^{i}(X)\otimes \mathbb{Q}_{\ell }$
(again, suitably interpreted). Thus the groups
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong h^{i}(X)\otimes \mathbb{Q}_{\ell }$
(again, suitably interpreted). Thus the groups
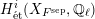 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
should be ‘independent of
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
should be ‘independent of
![]() $\ell$
’, since all the information they contain can be deduced from the ‘absolute’ cohomology groups
$\ell$
’, since all the information they contain can be deduced from the ‘absolute’ cohomology groups
![]() $h^{i}(X)$
. In particular for curves, we essentially have
$h^{i}(X)$
. In particular for curves, we essentially have
 $h^{1}(C)=\operatorname{Jac}(C_{F^{\text{sep}}})^{\vee }$
.
$h^{1}(C)=\operatorname{Jac}(C_{F^{\text{sep}}})^{\vee }$
.
While the theory of motives, despite significant recent advances, is still incomplete, its prediction that various cohomology groups should be ‘independent of
![]() $\ell$
’ can, over certain ground fields, be precisely formulated in ways which do not depend on such a theory. For example, one such statement is that for smooth and proper varieties
$\ell$
’ can, over certain ground fields, be precisely formulated in ways which do not depend on such a theory. For example, one such statement is that for smooth and proper varieties
![]() $X$
over finite fields of characteristic
$X$
over finite fields of characteristic
![]() $p$
, the trace of Frobenius on
$p$
, the trace of Frobenius on
 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
(for
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
(for
![]() $\ell \neq p$
) has values in
$\ell \neq p$
) has values in
![]() $\mathbb{Z}$
and is independent of
$\mathbb{Z}$
and is independent of
![]() $\ell$
. Since the absolute Galois group of finite fields is generated by Frobenius, this tells us that, up to semisimplification, the various Galois representations
$\ell$
. Since the absolute Galois group of finite fields is generated by Frobenius, this tells us that, up to semisimplification, the various Galois representations
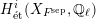 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
are, once restricted to
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
are, once restricted to
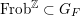 $\operatorname{Frob}^{\mathbb{Z}}\subset G_{F}$
, pairwise isomorphic (at least after base changing to any suitably large field
$\operatorname{Frob}^{\mathbb{Z}}\subset G_{F}$
, pairwise isomorphic (at least after base changing to any suitably large field
![]() $\unicode[STIX]{x1D6FA}$
).
$\unicode[STIX]{x1D6FA}$
).
Over more general fields, formulating similarly precise statements is somewhat tricky, since the Galois representation
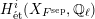 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
depends on the interaction between the topology of
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
depends on the interaction between the topology of
![]() $\mathbb{Q}_{\ell }$
and the profinite topology on
$\mathbb{Q}_{\ell }$
and the profinite topology on
![]() $G_{F}$
. For local fields (i.e. complete discrete valuation fields with finite residue fields) one can, thanks to the theory of Weil–Deligne representations and Grothendieck’s
$G_{F}$
. For local fields (i.e. complete discrete valuation fields with finite residue fields) one can, thanks to the theory of Weil–Deligne representations and Grothendieck’s
![]() $\ell$
-adic monodromy theorem (and at least for
$\ell$
-adic monodromy theorem (and at least for
![]() $\ell$
different from the residue characteristic), package up the information contained in
$\ell$
different from the residue characteristic), package up the information contained in
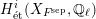 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
in a way that only depends on
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
in a way that only depends on
![]() $\mathbb{Q}_{\ell }$
as an abstract field; it therefore makes sense to conjecture that these groups become ‘pairwise isomorphic’ over suitably large fields.
$\mathbb{Q}_{\ell }$
as an abstract field; it therefore makes sense to conjecture that these groups become ‘pairwise isomorphic’ over suitably large fields.
For
![]() $\ell =p$
one needs a slightly different approach. Over finite fields, one needs to replace étale cohomology by crystalline cohomology
$\ell =p$
one needs a slightly different approach. Over finite fields, one needs to replace étale cohomology by crystalline cohomology
 $H_{\text{crys}}^{i}(X/K)$
, and over mixed characteristic local fields one needs to replace the
$H_{\text{crys}}^{i}(X/K)$
, and over mixed characteristic local fields one needs to replace the
![]() $\ell$
-adic monodromy theorem by its
$\ell$
-adic monodromy theorem by its
![]() $p$
-adic analogue, stating that the étale cohomology of varieties over mixed characteristic local fields is potentially semistable. Over finite fields, the formalism of crystalline cohomology (including weights) shows that the ‘independence of
$p$
-adic analogue, stating that the étale cohomology of varieties over mixed characteristic local fields is potentially semistable. Over finite fields, the formalism of crystalline cohomology (including weights) shows that the ‘independence of
![]() $\ell$
’ results can be extended to include the case
$\ell$
’ results can be extended to include the case
![]() $\ell =p$
, and over mixed characteristic local fields it was shown in [Reference FontaineFon94] how to use
$\ell =p$
, and over mixed characteristic local fields it was shown in [Reference FontaineFon94] how to use
![]() $p$
-adic Hodge theory to attach Weil–Deligne representations to de Rham Galois representations, thus again making sense of the
$p$
-adic Hodge theory to attach Weil–Deligne representations to de Rham Galois representations, thus again making sense of the
![]() $\ell$
-independence conjectures ‘at
$\ell$
-independence conjectures ‘at
![]() $p$
’. When
$p$
’. When
![]() $F$
is an equicharacteristic local field, then a more subtle use of crystalline cohomology allows one to view the cohomology of smooth and proper varieties over
$F$
is an equicharacteristic local field, then a more subtle use of crystalline cohomology allows one to view the cohomology of smooth and proper varieties over
![]() $F$
as
$F$
as
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring, and again the appropriate local monodromy theorem allows one to construct associated Weil–Deligne representations (see, for example, [Reference MarmoraMar08, § 3]). Thus, following Fontaine [Reference FontaineFon94], one can again formulate
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring, and again the appropriate local monodromy theorem allows one to construct associated Weil–Deligne representations (see, for example, [Reference MarmoraMar08, § 3]). Thus, following Fontaine [Reference FontaineFon94], one can again formulate
![]() $\ell$
-independence conjectures in this case, including at
$\ell$
-independence conjectures in this case, including at
![]() $\ell =p$
.
$\ell =p$
.
One can also ask about other expected ‘motivic’ invariants, the most basic being the unipotent fundamental group. When
![]() $\ell \neq p$
this can be defined as the
$\ell \neq p$
this can be defined as the
![]() $\mathbb{Q}_{\ell }$
-pro-unipotent completion of the geometric étale fundamental group. When
$\mathbb{Q}_{\ell }$
-pro-unipotent completion of the geometric étale fundamental group. When
![]() $F$
is finite and
$F$
is finite and
![]() $\ell \neq p$
it is therefore acted on by Frobenius, and again we can ask whether or not this ‘non-abelian’ representation of
$\ell \neq p$
it is therefore acted on by Frobenius, and again we can ask whether or not this ‘non-abelian’ representation of
![]() $\operatorname{Frob}^{\mathbb{Z}}$
is independent of
$\operatorname{Frob}^{\mathbb{Z}}$
is independent of
![]() $\ell$
. When
$\ell$
. When
![]() $F$
is local (and again when
$F$
is local (and again when
![]() $\ell \neq p$
) it is acted on ‘continuously’ by
$\ell \neq p$
) it is acted on ‘continuously’ by
![]() $G_{F}$
, so we can apply Grothendieck’s monodromy theorem and produce a ‘non-abelian’ Weil–Deligne representation. For
$G_{F}$
, so we can apply Grothendieck’s monodromy theorem and produce a ‘non-abelian’ Weil–Deligne representation. For
![]() $\ell =p$
and
$\ell =p$
and
![]() $F$
finite one again needs to take a different approach to define the pro-unipotent fundamental group, using Tannakian duality and the theory of isocrystals to produce a pro-unipotent group scheme on which Frobenius acts. When
$F$
finite one again needs to take a different approach to define the pro-unipotent fundamental group, using Tannakian duality and the theory of isocrystals to produce a pro-unipotent group scheme on which Frobenius acts. When
![]() $F$
is equicharacteristic local, one needs to appeal to the categories of overconvergent isocrystals introduced in [Reference Lazda and PálLP16] and studied in [Reference LazdaLaz16] in order to define the pro-unipotent fundamental group as a ‘non-abelian
$F$
is equicharacteristic local, one needs to appeal to the categories of overconvergent isocrystals introduced in [Reference Lazda and PálLP16] and studied in [Reference LazdaLaz16] in order to define the pro-unipotent fundamental group as a ‘non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module’ over the bounded Robba ring
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module’ over the bounded Robba ring
![]() ${\mathcal{E}}^{\dagger }$
. Again, appealing to the
${\mathcal{E}}^{\dagger }$
. Again, appealing to the
![]() $p$
-adic local monodromy theorem allows us to produce a non-abelian
$p$
-adic local monodromy theorem allows us to produce a non-abelian
![]() $p$
-adic Weil–Deligne representations in this case. Hence in both these cases (i.e.
$p$
-adic Weil–Deligne representations in this case. Hence in both these cases (i.e.
![]() $F$
finite or equicharacteristic local) one can formulate precise
$F$
finite or equicharacteristic local) one can formulate precise
![]() $\ell$
-independence conjectures for the unipotent fundamental group, including the case
$\ell$
-independence conjectures for the unipotent fundamental group, including the case
![]() $\ell =p$
. When
$\ell =p$
. When
![]() $F$
is mixed characteristic local and
$F$
is mixed characteristic local and
![]() $\ell =p$
things are more tricky, since in order to apply Fontaine’s methods one first needs to know that the
$\ell =p$
things are more tricky, since in order to apply Fontaine’s methods one first needs to know that the
![]() $p$
-adic unipotent
$p$
-adic unipotent
![]() $\unicode[STIX]{x1D70B}_{1}$
is de Rham as a
$\unicode[STIX]{x1D70B}_{1}$
is de Rham as a
![]() $G_{F}$
-representation. For semistable curves this follows from the results of [Reference Andreatta, Iovita and KimAIK15] and in general from results of Déglise and Nizioł [Reference Déglise and NiziołDN15].
$G_{F}$
-representation. For semistable curves this follows from the results of [Reference Andreatta, Iovita and KimAIK15] and in general from results of Déglise and Nizioł [Reference Déglise and NiziołDN15].
The purpose of this paper, then, is to prove various cases of these
![]() $\ell$
-independence conjectures, all including the case
$\ell$
-independence conjectures, all including the case
![]() $\ell =p$
. After recalling the various facts we need concerning cohomology and fundamental groups in § 2, the precise formulation of the conjectures is given in § 3. In the rest of the article we will then prove certain versions of them in the following four cases:
$\ell =p$
. After recalling the various facts we need concerning cohomology and fundamental groups in § 2, the precise formulation of the conjectures is given in § 3. In the rest of the article we will then prove certain versions of them in the following four cases:
-
(i) unipotent fundamental groups of smooth projective varieties over finite fields;
-
(ii) cohomology groups of smooth, proper varieties over equicharacteristic local fields;
-
(iii) unipotent fundamental groups of smooth, proper varieties with semistable reduction over equicharacteristic local fields;
-
(iv) unipotent fundamental groups of smooth, proper curves with semistable reduction over mixed characteristic local fields.
In the first case, the subject of § 4, we prove the strongest possible form of
![]() $\ell$
-independence, stating that the various unipotent
$\ell$
-independence, stating that the various unipotent
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{uni}}$
become isomorphic as ‘non-abelian’ Weil–Deligne representations over suitably large fields. Here the basic idea behind the proof is formality, that is, that the rational homotopy type is a formal consequence of the cohomology ring.
$\unicode[STIX]{x1D70B}_{1}^{\text{uni}}$
become isomorphic as ‘non-abelian’ Weil–Deligne representations over suitably large fields. Here the basic idea behind the proof is formality, that is, that the rational homotopy type is a formal consequence of the cohomology ring.
In the second case, treated in §§ 5 and 6, we show a weaker form of
![]() $\ell$
-independence, stating that the Frobenius semisimplifications of the various cohomology groups become isomorphic over suitably large fields. The proof proceeds by first considering the case when the variety in question has semistable reduction (Theorem 5.1). In this situation we use a ‘spreading out’ result to reduce to the case of a global semistable family, and then apply a result of Deligne in [Reference DeligneDel80] stating that ‘
$\ell$
-independence, stating that the Frobenius semisimplifications of the various cohomology groups become isomorphic over suitably large fields. The proof proceeds by first considering the case when the variety in question has semistable reduction (Theorem 5.1). In this situation we use a ‘spreading out’ result to reduce to the case of a global semistable family, and then apply a result of Deligne in [Reference DeligneDel80] stating that ‘
![]() $\ell$
-independence’ of a suitable local system on an open curve implies
$\ell$
-independence’ of a suitable local system on an open curve implies
![]() $\ell$
-independence at the missing points. In fact Deligne’s result only applies for
$\ell$
-independence at the missing points. In fact Deligne’s result only applies for
![]() $\ell \neq p$
, but it is not difficult to follow through his proof ‘at
$\ell \neq p$
, but it is not difficult to follow through his proof ‘at
![]() $p$
’, as we do in § 7. The general case is then deduced by using alterations and an argument involving weights (Theorem 6.1).
$p$
’, as we do in § 7. The general case is then deduced by using alterations and an argument involving weights (Theorem 6.1).
In the third case, the topic of § 8, we prove a fairly weak form of
![]() $\ell$
-independence, stating that the Frobenius semisimplifications of the graded pieces of the universal enveloping algebra of the Lie algebra of
$\ell$
-independence, stating that the Frobenius semisimplifications of the graded pieces of the universal enveloping algebra of the Lie algebra of
![]() $\unicode[STIX]{x1D70B}_{1}^{\text{uni}}$
(for the filtration coming from the augmentation ideal) become isomorphic over suitably large fields. The proof here follows that of the second case, again reducing to the case of a global semistable family and applying the same independence result of Deligne. Finally, in § 9, we consider the last case, proving the same result for curves in mixed characteristic by reducing to the equicharacteristic situation.
$\unicode[STIX]{x1D70B}_{1}^{\text{uni}}$
(for the filtration coming from the augmentation ideal) become isomorphic over suitably large fields. The proof here follows that of the second case, again reducing to the case of a global semistable family and applying the same independence result of Deligne. Finally, in § 9, we consider the last case, proving the same result for curves in mixed characteristic by reducing to the equicharacteristic situation.
Notation and conventions
We will let
![]() $F$
denote either a finite field
$F$
denote either a finite field
![]() $k$
or a local field with residue field
$k$
or a local field with residue field
![]() $k$
(usually equicharacteristic). As always,
$k$
(usually equicharacteristic). As always,
![]() $p$
will be the characteristic of
$p$
will be the characteristic of
![]() $k$
, and we will let
$k$
, and we will let
![]() $q$
denote its cardinality. The term ‘Frobenius’ without further qualification will always be understood to mean geometric Frobenius, and Frobenius structures on isocrystals will, unless specified otherwise, mean
$q$
denote its cardinality. The term ‘Frobenius’ without further qualification will always be understood to mean geometric Frobenius, and Frobenius structures on isocrystals will, unless specified otherwise, mean
![]() $q$
-power Frobenius structures. We will let
$q$
-power Frobenius structures. We will let
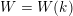 $W=W(k)$
denote the ring of Witt vectors of
$W=W(k)$
denote the ring of Witt vectors of
![]() $k$
,
$k$
,
![]() $K$
its fraction field and
$K$
its fraction field and
![]() $K^{\text{un}}$
the maximal unramified extension of
$K^{\text{un}}$
the maximal unramified extension of
![]() $K$
. By ‘variety’ we will mean ‘separated scheme of finite type’. We will denote a separable closure of
$K$
. By ‘variety’ we will mean ‘separated scheme of finite type’. We will denote a separable closure of
![]() $F$
by
$F$
by
![]() $F^{\text{sep}}$
, and the corresponding absolute Galois group by
$F^{\text{sep}}$
, and the corresponding absolute Galois group by
![]() $G_{F}$
. We will denote by
$G_{F}$
. We will denote by
![]() ${\mathcal{R}}$
the Robba ring over
${\mathcal{R}}$
the Robba ring over
![]() $K$
, and by
$K$
, and by
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}}^{\unicode[STIX]{x1D6FB}}$
the category of
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}}^{\unicode[STIX]{x1D6FB}}$
the category of
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{R}}$
. We will denote by
${\mathcal{R}}$
. We will denote by
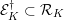 ${\mathcal{E}}_{K}^{\dagger }\subset {\mathcal{R}}_{K}$
the bounded Robba ring.
${\mathcal{E}}_{K}^{\dagger }\subset {\mathcal{R}}_{K}$
the bounded Robba ring.
2 Preliminaries on fundamental groups and
 $p$
-adic cohomology
$p$
-adic cohomology
The purpose of this section is to recall some general facts about unipotent fundamental groups, as well as some of the material from [Reference Lazda and PálLP16] concerning
![]() $p$
-adic cohomology over equicharacteristic local fields. So let
$p$
-adic cohomology over equicharacteristic local fields. So let
![]() $X/F$
be a smooth, geometrically connected, quasi-projective variety and
$X/F$
be a smooth, geometrically connected, quasi-projective variety and
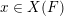 $x\in X(F)$
. Then, for any
$x\in X(F)$
. Then, for any
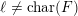 $\ell \neq \operatorname{char}(F)$
, the
$\ell \neq \operatorname{char}(F)$
, the
![]() $\ell$
-adic version of the unipotent fundamental group has two different but equivalent interpretations. First of all, we may consider the étale fundamental group
$\ell$
-adic version of the unipotent fundamental group has two different but equivalent interpretations. First of all, we may consider the étale fundamental group
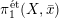 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X,\bar{x})$
based at a geometric point above
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X,\bar{x})$
based at a geometric point above
![]() $x$
, together with the homotopy exact sequence
$x$
, together with the homotopy exact sequence
 $$\begin{eqnarray}1\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X,\bar{x})\rightarrow G_{F}\rightarrow 1.\end{eqnarray}$$
$$\begin{eqnarray}1\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X,\bar{x})\rightarrow G_{F}\rightarrow 1.\end{eqnarray}$$
The point
![]() $x$
induces a splitting of this exact sequence, and this gives rise to an action of
$x$
induces a splitting of this exact sequence, and this gives rise to an action of
![]() $G_{F}$
on
$G_{F}$
on
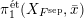 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})$
. Applying the Malcev completion functor over
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})$
. Applying the Malcev completion functor over
![]() $\mathbb{Q}_{\ell }$
then gives a pro-unipotent group scheme
$\mathbb{Q}_{\ell }$
then gives a pro-unipotent group scheme
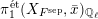 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
over
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
over
![]() $\mathbb{Q}_{\ell }$
which by functoriality is acted on by
$\mathbb{Q}_{\ell }$
which by functoriality is acted on by
![]() $G_{F}$
.
$G_{F}$
.
Alternatively, we can use a Tannakian approach. That is, we consider the Tannakian category
 $\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})$
of unipotent lisse
$\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})$
of unipotent lisse
![]() $\mathbb{Q}_{\ell }$
-sheaves on
$\mathbb{Q}_{\ell }$
-sheaves on
![]() $X$
. The point
$X$
. The point
![]() $x$
gives rise to a fibre functor
$x$
gives rise to a fibre functor
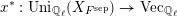 $x^{\ast }:\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})\rightarrow \operatorname{Vec}_{\mathbb{Q}_{\ell }}$
and hence we may define
$x^{\ast }:\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})\rightarrow \operatorname{Vec}_{\mathbb{Q}_{\ell }}$
and hence we may define
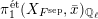 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
to be the (pro-unipotent) affine group scheme representing automorphisms of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
to be the (pro-unipotent) affine group scheme representing automorphisms of
![]() $x^{\ast }$
. By functoriality
$x^{\ast }$
. By functoriality
![]() $G_{F}$
acts on
$G_{F}$
acts on
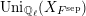 $\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})$
, preserving
$\operatorname{Uni}_{\mathbb{Q}_{\ell }}(X_{F^{\text{sep}}})$
, preserving
![]() $x^{\ast }$
, and hence by Tannaka duality on
$x^{\ast }$
, and hence by Tannaka duality on
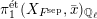 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
. As the notation suggests, this coincides with the previous construction by [Reference IllusieSGA5, Exposé VI, § 1.4.2].
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
. As the notation suggests, this coincides with the previous construction by [Reference IllusieSGA5, Exposé VI, § 1.4.2].
While the first definition does not behave well when
 $\ell =\operatorname{char}(F)$
, the second has a clear analogue, at least when
$\ell =\operatorname{char}(F)$
, the second has a clear analogue, at least when
![]() $F=k$
is finite, replacing lisse
$F=k$
is finite, replacing lisse
![]() $\mathbb{Q}_{\ell }$
-sheaves on
$\mathbb{Q}_{\ell }$
-sheaves on
![]() $X_{F^{\text{sep}}}$
by overconvergent isocrystals on
$X_{F^{\text{sep}}}$
by overconvergent isocrystals on
![]() $X/K$
, the category of which we will denote by
$X/K$
, the category of which we will denote by
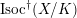 $\operatorname{Isoc}^{\dagger }(X/K)$
. Let
$\operatorname{Isoc}^{\dagger }(X/K)$
. Let
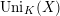 $\operatorname{Uni}_{K}(X)$
denote its full subcategory of unipotent objects. Again, the point
$\operatorname{Uni}_{K}(X)$
denote its full subcategory of unipotent objects. Again, the point
![]() $x$
induces a fibre functor
$x$
induces a fibre functor
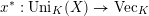 $x^{\ast }:\operatorname{Uni}_{K}(X)\rightarrow \operatorname{Vec}_{K}$
and by definition
$x^{\ast }:\operatorname{Uni}_{K}(X)\rightarrow \operatorname{Vec}_{K}$
and by definition
 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
is the (pro-unipotent) affine group scheme representating automorphisms of
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
is the (pro-unipotent) affine group scheme representating automorphisms of
![]() $x^{\ast }$
. The Frobenius pullback functor
$x^{\ast }$
. The Frobenius pullback functor
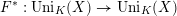 $F^{\ast }:\operatorname{Uni}_{K}(X)\rightarrow \operatorname{Uni}_{K}(X)$
is an autoequivalence, and induces an isomorphism
$F^{\ast }:\operatorname{Uni}_{K}(X)\rightarrow \operatorname{Uni}_{K}(X)$
is an autoequivalence, and induces an isomorphism
 $F_{\ast }:\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
.
$F_{\ast }:\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
.
When
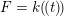 $F=k(\!(t)\!)$
is equicharacteristic local, we need to use the machinery of [Reference Lazda and PálLP16] to define the
$F=k(\!(t)\!)$
is equicharacteristic local, we need to use the machinery of [Reference Lazda and PálLP16] to define the
![]() $p$
-adic unipotent fundamental groups. Since we will also need the results from [Reference Lazda and PálLP16] concerning
$p$
-adic unipotent fundamental groups. Since we will also need the results from [Reference Lazda and PálLP16] concerning
![]() $p$
-adic cohomology for varieties over such
$p$
-adic cohomology for varieties over such
![]() $F$
, we will take the opportunity now to quickly recap some of the main points from [Reference Lazda and PálLP16]. There we constructed, for any variety
$F$
, we will take the opportunity now to quickly recap some of the main points from [Reference Lazda and PálLP16]. There we constructed, for any variety
![]() $X/F$
, cohomology groups
$X/F$
, cohomology groups
 $H_{\text{rig}}^{i}(X/{\mathcal{E}}_{K}^{\dagger })$
as
$H_{\text{rig}}^{i}(X/{\mathcal{E}}_{K}^{\dagger })$
as
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the bounded Robba ring
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the bounded Robba ring
![]() ${\mathcal{E}}_{K}^{\dagger }$
, satisfying all the expected properties of an ‘extended’ Weil cohomology theory. In other words, we have finite dimensionality, vanishing in the expected degrees, versions with compact support or support in a closed subscheme, Poincaré duality, Künneth formula, etc. We also constructed a category
${\mathcal{E}}_{K}^{\dagger }$
, satisfying all the expected properties of an ‘extended’ Weil cohomology theory. In other words, we have finite dimensionality, vanishing in the expected degrees, versions with compact support or support in a closed subscheme, Poincaré duality, Künneth formula, etc. We also constructed a category
 $\operatorname{Isoc}^{\dagger }(X/{\mathcal{E}}_{K}^{\dagger })$
of overconvergent isocrystals on
$\operatorname{Isoc}^{\dagger }(X/{\mathcal{E}}_{K}^{\dagger })$
of overconvergent isocrystals on
![]() $X$
relative to
$X$
relative to
![]() ${\mathcal{E}}_{K}^{\dagger }$
, and in [Reference LazdaLaz16, Corollary 2.2] it was proved that this category is Tannakian. The point
${\mathcal{E}}_{K}^{\dagger }$
, and in [Reference LazdaLaz16, Corollary 2.2] it was proved that this category is Tannakian. The point
![]() $x$
provides a fibre functor
$x$
provides a fibre functor
 $$\begin{eqnarray}x^{\ast }:\operatorname{Uni}_{{\mathcal{E}}_{K}^{\dagger }}(X)\rightarrow \operatorname{Vec}_{{\mathcal{E}}_{K}^{\dagger }}\end{eqnarray}$$
$$\begin{eqnarray}x^{\ast }:\operatorname{Uni}_{{\mathcal{E}}_{K}^{\dagger }}(X)\rightarrow \operatorname{Vec}_{{\mathcal{E}}_{K}^{\dagger }}\end{eqnarray}$$
from the subcategory of unipotent isocrystals, and again we may define
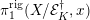 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
to be the corresponding pro-unipotent group scheme over
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
to be the corresponding pro-unipotent group scheme over
![]() ${\mathcal{E}}_{K}^{\dagger }$
. It was also shown in [Reference LazdaLaz16, § 5] how to put a canonical ‘
${\mathcal{E}}_{K}^{\dagger }$
. It was also shown in [Reference LazdaLaz16, § 5] how to put a canonical ‘
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module structure’ on
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module structure’ on
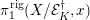 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
, that is, a
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
, that is, a
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module structure on its Hopf algebra, which simply as a
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module structure on its Hopf algebra, which simply as a
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module (i.e. forgetting the Hopf algebra structure) is a direct limit of its finite-dimensional sub-
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module (i.e. forgetting the Hopf algebra structure) is a direct limit of its finite-dimensional sub-
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules.
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules.
Base changing the whole situation to
![]() ${\mathcal{R}}_{K}$
, we therefore obtain a non-abelian
${\mathcal{R}}_{K}$
, we therefore obtain a non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x):=\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x):=\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}\end{eqnarray}$$
over
![]() ${\mathcal{R}}_{K}$
, as well as abelian ones,
${\mathcal{R}}_{K}$
, as well as abelian ones,
 $$\begin{eqnarray}H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K}):=H_{\text{rig}}^{i}(X/{\mathcal{E}}_{K}^{\dagger })\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}.\end{eqnarray}$$
$$\begin{eqnarray}H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K}):=H_{\text{rig}}^{i}(X/{\mathcal{E}}_{K}^{\dagger })\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}.\end{eqnarray}$$
These should be considered
![]() $p$
-adic analogues of the
$p$
-adic analogues of the
![]() $\ell$
-adic Galois representations
$\ell$
-adic Galois representations
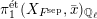 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
and
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
and
 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
, respectively.
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
, respectively.
3 The formalism of
 $\ell$
-independence and statement of conjectures
$\ell$
-independence and statement of conjectures
In this section we will review some of the formalism behind the notion of
![]() $\ell$
-independence over finite and equicharacteristic local fields, following [Reference DeligneDel80, Reference FontaineFon94]. For this section,
$\ell$
-independence over finite and equicharacteristic local fields, following [Reference DeligneDel80, Reference FontaineFon94]. For this section,
![]() $F$
will stand for either a finite field
$F$
will stand for either a finite field
![]() $k$
or a local field with residue field
$k$
or a local field with residue field
![]() $k$
.
$k$
.
Definition 3.1. We define the Weil group
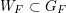 $W_{F}\subset G_{F}$
as follows.
$W_{F}\subset G_{F}$
as follows.
-
– If
 $F=k$
, then we will let
$F=k$
, then we will let
 $W_{F}$
denote the subgroup of
$W_{F}$
denote the subgroup of
 $G_{F}\cong \operatorname{Frob}_{k}^{\widehat{\mathbb{Z}}}$
consisting of integer powers of Frobenius.
$G_{F}\cong \operatorname{Frob}_{k}^{\widehat{\mathbb{Z}}}$
consisting of integer powers of Frobenius. -
– If
 $F$
is local, then we will let
$F$
is local, then we will let
 $W_{F}$
denote the inverse image of
$W_{F}$
denote the inverse image of
 $W_{k}$
under the surjection
$W_{k}$
under the surjection
 $G_{F}{\twoheadrightarrow}G_{k}$
.
$G_{F}{\twoheadrightarrow}G_{k}$
.
It is topologised as follows: if
![]() $F$
is finite then
$F$
is finite then
![]() $W_{F}$
is given the discrete topology, while if
$W_{F}$
is given the discrete topology, while if
![]() $F$
is local then
$F$
is local then
![]() $W_{F}$
is given the unique topology such that the inertia group
$W_{F}$
is given the unique topology such that the inertia group
![]() $I_{F}$
is open.
$I_{F}$
is open.
Since
![]() $W_{F}$
is pro-discrete, we may form the associated group scheme
$W_{F}$
is pro-discrete, we may form the associated group scheme
![]() $W_{F}^{\text{alg}}$
over
$W_{F}^{\text{alg}}$
over
![]() $\mathbb{Q}$
by writing
$\mathbb{Q}$
by writing
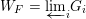 $W_{F}=\mathop{\varprojlim }\nolimits_{i}G_{i}$
in the category of groups, viewing each discrete group
$W_{F}=\mathop{\varprojlim }\nolimits_{i}G_{i}$
in the category of groups, viewing each discrete group
![]() $G_{i}$
as a group scheme over
$G_{i}$
as a group scheme over
![]() $\mathbb{Q}$
and then setting
$\mathbb{Q}$
and then setting
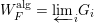 $W_{F}^{\text{alg}}=\mathop{\varprojlim }\nolimits_{i}G_{i}$
in the category of group schemes.
$W_{F}^{\text{alg}}=\mathop{\varprojlim }\nolimits_{i}G_{i}$
in the category of group schemes.
Lemma 3.2. For any field
![]() $E$
of characteristic
$E$
of characteristic
![]() $0$
there is an equivalence of categories between continuous representations
$0$
there is an equivalence of categories between continuous representations
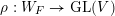 $\unicode[STIX]{x1D70C}:W_{F}\rightarrow \operatorname{GL}(V)$
in finite-dimensional
$\unicode[STIX]{x1D70C}:W_{F}\rightarrow \operatorname{GL}(V)$
in finite-dimensional
![]() $E$
-vector spaces and finite-dimensional algebraic representations
$E$
-vector spaces and finite-dimensional algebraic representations
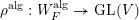 $\unicode[STIX]{x1D70C}^{\text{alg}}:W_{F}^{\text{alg}}\rightarrow \operatorname{GL}(V)$
of the group scheme
$\unicode[STIX]{x1D70C}^{\text{alg}}:W_{F}^{\text{alg}}\rightarrow \operatorname{GL}(V)$
of the group scheme
![]() $W_{F}^{\text{alg}}$
over
$W_{F}^{\text{alg}}$
over
![]() $E$
.
$E$
.
If
![]() $F$
is local then there is a natural action of
$F$
is local then there is a natural action of
![]() $W_{F}^{\text{alg}}$
on the additive group
$W_{F}^{\text{alg}}$
on the additive group
![]() $\mathbb{G}_{a}$
by setting
$\mathbb{G}_{a}$
by setting
 $$\begin{eqnarray}gxg^{-1}=q^{-v(g)}x,\end{eqnarray}$$
$$\begin{eqnarray}gxg^{-1}=q^{-v(g)}x,\end{eqnarray}$$
where
![]() $v(g)$
is such that
$v(g)$
is such that
![]() $g$
maps to
$g$
maps to
 $\operatorname{Frob}_{k}^{v(g)}$
in
$\operatorname{Frob}_{k}^{v(g)}$
in
![]() $W_{k}$
.
$W_{k}$
.
Definition 3.3. We define the Weil–Deligne group
![]() $W_{F}^{\prime }$
to be
$W_{F}^{\prime }$
to be
![]() $W_{F}^{\text{alg}}$
if
$W_{F}^{\text{alg}}$
if
![]() $F=k$
is finite or
$F=k$
is finite or
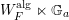 $W_{F}^{\text{alg}}\ltimes \mathbb{G}_{a}$
if
$W_{F}^{\text{alg}}\ltimes \mathbb{G}_{a}$
if
![]() $F$
is local.
$F$
is local.
Note that the usage of the term ‘Weil–Deligne’ group to refer to
![]() $W_{F}^{\text{alg}}$
over finite fields is not at all standard, and we employ it here only so as to be able to make uniform statements for finite and local fields.
$W_{F}^{\text{alg}}$
over finite fields is not at all standard, and we employ it here only so as to be able to make uniform statements for finite and local fields.
We will now let
![]() $E$
be a field of characteristic
$E$
be a field of characteristic
![]() $0$
, and let
$0$
, and let
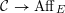 ${\mathcal{C}}\rightarrow \operatorname{Aff}_{E}$
be a category fibred over the category of affine
${\mathcal{C}}\rightarrow \operatorname{Aff}_{E}$
be a category fibred over the category of affine
![]() $E$
-schemes. In other words, for every
$E$
-schemes. In other words, for every
![]() $E$
-algebra
$E$
-algebra
![]() $R$
we have a category
$R$
we have a category
![]() ${\mathcal{C}}_{R}$
and for every morphism
${\mathcal{C}}_{R}$
and for every morphism
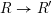 $R\rightarrow R^{\prime }$
we have a pullback (or ‘base extension’) functor
$R\rightarrow R^{\prime }$
we have a pullback (or ‘base extension’) functor
 ${\mathcal{C}}_{R}\rightarrow {\mathcal{C}}_{R^{\prime }}$
. The reader should keep in mind the following examples:
${\mathcal{C}}_{R}\rightarrow {\mathcal{C}}_{R^{\prime }}$
. The reader should keep in mind the following examples:
-
– the category of (quasi-)coherent
 ${\mathcal{O}}$
-modules;
${\mathcal{O}}$
-modules; -
– the category of pro-nilpotent Lie
 ${\mathcal{O}}$
-algebras;
${\mathcal{O}}$
-algebras; -
– the category of (quasi-coherent) Hopf
 ${\mathcal{O}}$
-algebras;
${\mathcal{O}}$
-algebras; -
– the category of pro-unipotent group schemes.
In particular, given an object
 $X\in {\mathcal{C}}_{E}$
, it makes sense to speak of the functor on
$X\in {\mathcal{C}}_{E}$
, it makes sense to speak of the functor on
![]() $E$
-algebras,
$E$
-algebras,
 $$\begin{eqnarray}\text{}\underline{\operatorname{Aut}}_{E}(X)(R)=\operatorname{Aut}_{{\mathcal{C}}_{R}}(X\otimes R).\end{eqnarray}$$
$$\begin{eqnarray}\text{}\underline{\operatorname{Aut}}_{E}(X)(R)=\operatorname{Aut}_{{\mathcal{C}}_{R}}(X\otimes R).\end{eqnarray}$$
Definition 3.4. A
![]() ${\mathcal{C}}_{E}$
-representation of
${\mathcal{C}}_{E}$
-representation of
![]() $W_{F}^{\prime }$
is an object
$W_{F}^{\prime }$
is an object
 $X\in {\mathcal{C}}_{E}$
together with a morphism of functors
$X\in {\mathcal{C}}_{E}$
together with a morphism of functors
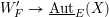 $W_{F}^{\prime }\rightarrow \text{}\underline{\operatorname{Aut}}_{E}(X)$
. The category of such objects is denoted by
$W_{F}^{\prime }\rightarrow \text{}\underline{\operatorname{Aut}}_{E}(X)$
. The category of such objects is denoted by
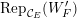 $\operatorname{Rep}_{{\mathcal{C}}_{E}}(W_{F}^{\prime })$
.
$\operatorname{Rep}_{{\mathcal{C}}_{E}}(W_{F}^{\prime })$
.
Note that when
![]() ${\mathcal{C}}$
is the category of coherent
${\mathcal{C}}$
is the category of coherent
![]() ${\mathcal{O}}$
-modules, one recovers the usual notion of an
${\mathcal{O}}$
-modules, one recovers the usual notion of an
![]() $E$
-valued Weil(–Deligne) representation; in general the point of this slightly fiddly approach is in order to have a well-defined notion for non-abelian objects such as Hopf algebras or group schemes. We can now finally make precise the concept of
$E$
-valued Weil(–Deligne) representation; in general the point of this slightly fiddly approach is in order to have a well-defined notion for non-abelian objects such as Hopf algebras or group schemes. We can now finally make precise the concept of
![]() $\ell$
-independence for (not necessarily abelian) Weil–Deligne representations.
$\ell$
-independence for (not necessarily abelian) Weil–Deligne representations.
Definition 3.5 [Reference DeligneDel73, § 8].
(i) Let
![]() $E^{\prime }/E$
be a field extension, and
$E^{\prime }/E$
be a field extension, and
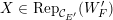 $X\in \operatorname{Rep}_{{\mathcal{C}}_{E^{\prime }}}(W_{F}^{\prime })$
a
$X\in \operatorname{Rep}_{{\mathcal{C}}_{E^{\prime }}}(W_{F}^{\prime })$
a
![]() ${\mathcal{C}}_{E^{\prime }}$
-valued Weil–Deligne representation. Then we say that
${\mathcal{C}}_{E^{\prime }}$
-valued Weil–Deligne representation. Then we say that
![]() $X$
is defined over
$X$
is defined over
![]() $E$
if for any algebraically closed field
$E$
if for any algebraically closed field
![]() $\unicode[STIX]{x1D6FA}$
containing
$\unicode[STIX]{x1D6FA}$
containing
![]() $E^{\prime }$
,
$E^{\prime }$
,
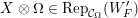 $X\otimes \unicode[STIX]{x1D6FA}\in \operatorname{Rep}_{{\mathcal{C}}_{\unicode[STIX]{x1D6FA}}}(W_{F}^{\prime })$
is isomorphic to all of its
$X\otimes \unicode[STIX]{x1D6FA}\in \operatorname{Rep}_{{\mathcal{C}}_{\unicode[STIX]{x1D6FA}}}(W_{F}^{\prime })$
is isomorphic to all of its
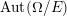 $\operatorname{Aut}(\unicode[STIX]{x1D6FA}/E)$
-conjugates.
$\operatorname{Aut}(\unicode[STIX]{x1D6FA}/E)$
-conjugates.
(ii) Let
![]() $\{E_{i}\}_{i\in I}$
be a family of field extensions of
$\{E_{i}\}_{i\in I}$
be a family of field extensions of
![]() $E$
, and
$E$
, and
 $\{X_{i}\}_{i\in I}$
a family of
$\{X_{i}\}_{i\in I}$
a family of
![]() ${\mathcal{C}}_{E_{i}}$
-valued Weil–Deligne representations. Then we say that
${\mathcal{C}}_{E_{i}}$
-valued Weil–Deligne representations. Then we say that
 $\{X_{i}\}_{i\in I}$
is
$\{X_{i}\}_{i\in I}$
is
![]() $E$
-compatible if each
$E$
-compatible if each
![]() $X_{i}$
is defined over
$X_{i}$
is defined over
![]() $E$
, and for any
$E$
, and for any
![]() $i,j$
and any algebraically closed field
$i,j$
and any algebraically closed field
![]() $\unicode[STIX]{x1D6FA}$
containing
$\unicode[STIX]{x1D6FA}$
containing
![]() $E_{i}$
and
$E_{i}$
and
![]() $E_{j}$
, the objects
$E_{j}$
, the objects
![]() $X_{i}\otimes \unicode[STIX]{x1D6FA}$
and
$X_{i}\otimes \unicode[STIX]{x1D6FA}$
and
![]() $X_{j}\otimes \unicode[STIX]{x1D6FA}$
in
$X_{j}\otimes \unicode[STIX]{x1D6FA}$
in
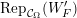 $\operatorname{Rep}_{{\mathcal{C}}_{\unicode[STIX]{x1D6FA}}}(W_{F}^{\prime })$
are isomorphic.
$\operatorname{Rep}_{{\mathcal{C}}_{\unicode[STIX]{x1D6FA}}}(W_{F}^{\prime })$
are isomorphic.
When
![]() ${\mathcal{C}}$
is the category of coherent
${\mathcal{C}}$
is the category of coherent
![]() ${\mathcal{O}}$
-modules, we also have a weaker notion of compatibility described as follows. If
${\mathcal{O}}$
-modules, we also have a weaker notion of compatibility described as follows. If
![]() $F$
is finite then we define the ‘monodromy’ filtration
$F$
is finite then we define the ‘monodromy’ filtration
![]() $M_{\bullet }$
on a representation
$M_{\bullet }$
on a representation
![]() $V$
of
$V$
of
![]() $W_{F}^{\prime }$
to be the trivial one, namely,
$W_{F}^{\prime }$
to be the trivial one, namely,
![]() $0$
in negative degrees and
$0$
in negative degrees and
![]() $V$
otherwise. If
$V$
otherwise. If
![]() $F$
is local then we will let
$F$
is local then we will let
![]() $M_{\bullet }$
be the usual monodromy filtration coming from viewing the
$M_{\bullet }$
be the usual monodromy filtration coming from viewing the
![]() $\mathbb{G}_{a}$
action as a nilpotent endomorphism
$\mathbb{G}_{a}$
action as a nilpotent endomorphism
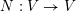 $N:V\rightarrow V$
.
$N:V\rightarrow V$
.
Definition 3.6. Let
![]() $\{V_{i}\}_{i\in I}$
be a family of finite-dimensional
$\{V_{i}\}_{i\in I}$
be a family of finite-dimensional
![]() $E_{i}$
-valued Weil–Deligne representations as above. We say that
$E_{i}$
-valued Weil–Deligne representations as above. We say that
![]() $\{V_{i}\}_{i\in I}$
is weakly
$\{V_{i}\}_{i\in I}$
is weakly
![]() $E$
-compatible if for all
$E$
-compatible if for all
![]() $k$
the character
$k$
the character
 $$\begin{eqnarray}\operatorname{Tr}(-|\operatorname{Gr}_{k}^{M}V_{i}):W_{F}\rightarrow E_{i}\end{eqnarray}$$
$$\begin{eqnarray}\operatorname{Tr}(-|\operatorname{Gr}_{k}^{M}V_{i}):W_{F}\rightarrow E_{i}\end{eqnarray}$$
of the
![]() $k$
th graded piece of the monodromy filtration has values in
$k$
th graded piece of the monodromy filtration has values in
![]() $E$
and is independent of
$E$
and is independent of
![]() $i$
.
$i$
.
Note that the difference between weak compatibility and compatibility is essentially that of Frobenius semisimplicity. For a representation
![]() $V$
of
$V$
of
![]() $W_{F}^{\prime }$
, we will let
$W_{F}^{\prime }$
, we will let
![]() $V^{F\text{-}\text{ss}}$
denote its Frobenius semisimplification. Thus when
$V^{F\text{-}\text{ss}}$
denote its Frobenius semisimplification. Thus when
![]() $F=k$
is finite this is just the semisimplification, and when
$F=k$
is finite this is just the semisimplification, and when
![]() $F$
is local we semisimplify the underlying
$F$
is local we semisimplify the underlying
![]() $W_{F}$
-representation.
$W_{F}$
-representation.
Lemma 3.7. Let
![]() $\{V_{i}\}$
be a family of
$\{V_{i}\}$
be a family of
![]() $W_{F}^{\prime }$
-representations. Then
$W_{F}^{\prime }$
-representations. Then
![]() $\{V_{i}\}$
is weakly
$\{V_{i}\}$
is weakly
![]() $E$
-compatible if and only if
$E$
-compatible if and only if
![]() $\{V_{i}^{F\text{-}\text{ss}}\}$
is
$\{V_{i}^{F\text{-}\text{ss}}\}$
is
![]() $E$
-compatible.
$E$
-compatible.
Proof. When
![]() $F$
is finite this is clear; when
$F$
is finite this is clear; when
![]() $F$
is local this is [Reference DeligneDel73, Proposition 8.9].◻
$F$
is local this is [Reference DeligneDel73, Proposition 8.9].◻
Now, if
![]() $X/F$
is a variety and
$X/F$
is a variety and
![]() $\ell \neq p$
, then one can view the
$\ell \neq p$
, then one can view the
![]() $i$
th étale cohomology group
$i$
th étale cohomology group
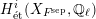 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
as a continuous representation of
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })$
as a continuous representation of
![]() $G_{F}$
, and hence as an
$G_{F}$
, and hence as an
![]() $\mathbb{Q}_{\ell }$
-valued algebraic representation of
$\mathbb{Q}_{\ell }$
-valued algebraic representation of
![]() $W_{F}^{\prime }$
. When we consider it as the latter we will generally write
$W_{F}^{\prime }$
. When we consider it as the latter we will generally write
![]() $H_{\ell }^{i}(X)$
. For
$H_{\ell }^{i}(X)$
. For
![]() $\ell =p$
and
$\ell =p$
and
![]() $F=k$
finite, the natural Frobenius action on the rigid cohomology
$F=k$
finite, the natural Frobenius action on the rigid cohomology
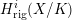 $H_{\text{rig}}^{i}(X/K)$
allows us to consider it as a
$H_{\text{rig}}^{i}(X/K)$
allows us to consider it as a
![]() $K$
-valued algebraic representation
$K$
-valued algebraic representation
![]() $H_{p}^{i}(X)$
of
$H_{p}^{i}(X)$
of
![]() $W_{F}^{\prime }$
. For
$W_{F}^{\prime }$
. For
![]() $\ell =p$
and
$\ell =p$
and
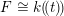 $F\cong k(\!(t)\!)$
equicharacteristic local, we may consider the rigid cohomology groups
$F\cong k(\!(t)\!)$
equicharacteristic local, we may consider the rigid cohomology groups
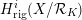 $H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})$
of
$H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})$
of
![]() $X$
as
$X$
as
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring
![]() ${\mathcal{R}}_{K}$
. Using Marmora’s functor [Reference MarmoraMar08] from
${\mathcal{R}}_{K}$
. Using Marmora’s functor [Reference MarmoraMar08] from
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{R}}_{K}$
to
${\mathcal{R}}_{K}$
to
![]() $K^{\text{un}}$
-valued Weil–Deligne representations, we may therefore consider the Weil–Deligne representation
$K^{\text{un}}$
-valued Weil–Deligne representations, we may therefore consider the Weil–Deligne representation
![]() $H_{p}^{i}(X)$
associated to
$H_{p}^{i}(X)$
associated to
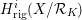 $H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})$
. When
$H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})$
. When
![]() $\ell =p$
and
$\ell =p$
and
![]() $F$
is mixed characteristic local, the étale cohomology
$F$
is mixed characteristic local, the étale cohomology
 $H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{p})$
is de Rham as a representation of
$H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{p})$
is de Rham as a representation of
![]() $G_{F}$
, hence we may follow Fontaine’s construction [Reference FontaineFon94] to produce a
$G_{F}$
, hence we may follow Fontaine’s construction [Reference FontaineFon94] to produce a
![]() $K^{\text{un}}$
-valued Weil–Deligne representation, which we will again denote by
$K^{\text{un}}$
-valued Weil–Deligne representation, which we will again denote by
![]() $H_{p}^{i}(X)$
. Of course, we may also consider versions
$H_{p}^{i}(X)$
. Of course, we may also consider versions
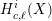 $H_{c,\ell }^{i}(X)$
with compact support. For cohomology, then, the
$H_{c,\ell }^{i}(X)$
with compact support. For cohomology, then, the
![]() $\ell$
-independence conjectures (first formulated for
$\ell$
-independence conjectures (first formulated for
![]() $F$
local in mixed characteristic by Fontaine in [Reference FontaineFon94]) are as follows.
$F$
local in mixed characteristic by Fontaine in [Reference FontaineFon94]) are as follows.
Conjecture 3.8.
-
–
 $C_{\ell }(X,H^{i})$
: the system
$C_{\ell }(X,H^{i})$
: the system
 $\{H_{\ell }^{i}(X)\}_{\ell }$
is
$\{H_{\ell }^{i}(X)\}_{\ell }$
is
 $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible. -
–
 $C_{\ell ,w}(X,H^{i})$
: the system
$C_{\ell ,w}(X,H^{i})$
: the system
 $\{H_{\ell }^{i}(X)\}_{\ell }$
is weakly
$\{H_{\ell }^{i}(X)\}_{\ell }$
is weakly
 $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible. -
–
 $C_{\ell }(X,H_{c}^{i})$
: the system
$C_{\ell }(X,H_{c}^{i})$
: the system
 $\{H_{c,\ell }^{i}(X)\}_{\ell }$
is
$\{H_{c,\ell }^{i}(X)\}_{\ell }$
is
 $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible. -
–
 $C_{\ell ,w}(X,H_{c}^{i})$
: the system
$C_{\ell ,w}(X,H_{c}^{i})$
: the system
 $\{H_{c,\ell }^{i}(X)\}_{\ell }$
is weakly
$\{H_{c,\ell }^{i}(X)\}_{\ell }$
is weakly
 $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible.
When
![]() $F$
is finite,
$F$
is finite,
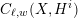 $C_{\ell ,w}(X,H^{i})$
is known whenever
$C_{\ell ,w}(X,H^{i})$
is known whenever
![]() $X$
is smooth and proper. (For
$X$
is smooth and proper. (For
![]() $\ell \neq p$
this follows from Deligne’s proof of the Riemann hypothesis [Reference DeligneDel74]. The fact that the same is true at
$\ell \neq p$
this follows from Deligne’s proof of the Riemann hypothesis [Reference DeligneDel74]. The fact that the same is true at
![]() $\ell =p$
then follows from [Reference Katz and MessingKM74, Corollary 1].) Even in this case, however,
$\ell =p$
then follows from [Reference Katz and MessingKM74, Corollary 1].) Even in this case, however,
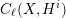 $C_{\ell }(X,H^{i})$
is still wide open in general; by Lemma 3.7 it would follow from the Frobenius semisimplicity conjecture. Hence for
$C_{\ell }(X,H^{i})$
is still wide open in general; by Lemma 3.7 it would follow from the Frobenius semisimplicity conjecture. Hence for
![]() $X$
proper and smooth of dimension
$X$
proper and smooth of dimension
![]() $d$
we know
$d$
we know
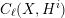 $C_{\ell }(X,H^{i})$
for
$C_{\ell }(X,H^{i})$
for
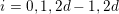 $i=0,1,2d-1,2d$
, as well as for abelian varieties in all degrees. Let us also remark that in [Reference Mannisto and OlssonMO] the authors prove
$i=0,1,2d-1,2d$
, as well as for abelian varieties in all degrees. Let us also remark that in [Reference Mannisto and OlssonMO] the authors prove
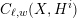 $C_{\ell ,w}(X,H^{i})$
and
$C_{\ell ,w}(X,H^{i})$
and
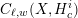 $C_{\ell ,w}(X,H_{c}^{i})$
whenever
$C_{\ell ,w}(X,H_{c}^{i})$
whenever
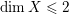 $\dim X\leqslant 2$
.
$\dim X\leqslant 2$
.
When
![]() $F$
is local, a much weaker version of
$F$
is local, a much weaker version of
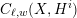 $C_{\ell ,w}(X,H^{i})$
and
$C_{\ell ,w}(X,H^{i})$
and
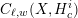 $C_{\ell ,w}(X,H_{c}^{i})$
forms part of the main result of [Reference ZhengZhe09]. However, even for smooth and projective varieties the fact that ‘purity’ for
$C_{\ell ,w}(X,H_{c}^{i})$
forms part of the main result of [Reference ZhengZhe09]. However, even for smooth and projective varieties the fact that ‘purity’ for
![]() $H^{i}$
is more complicated for local fields means that there is no straightforward deduction of
$H^{i}$
is more complicated for local fields means that there is no straightforward deduction of
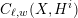 $C_{\ell ,w}(X,H^{i})$
from Zheng’s results. In [Reference Lazda and PálLP16, Theorem 5.85] it was proved that when
$C_{\ell ,w}(X,H^{i})$
from Zheng’s results. In [Reference Lazda and PálLP16, Theorem 5.85] it was proved that when
 $F\cong k(\!(t)\!)$
is equicharacteristic local,
$F\cong k(\!(t)\!)$
is equicharacteristic local,
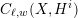 $C_{\ell ,w}(X,H^{i})$
holds for smooth (possibly open) curves and abelian varieties, or for smooth and proper varieties with good reduction. In fact, it was claimed there that
$C_{\ell ,w}(X,H^{i})$
holds for smooth (possibly open) curves and abelian varieties, or for smooth and proper varieties with good reduction. In fact, it was claimed there that
 $C_{\ell }(X,H^{i})$
holds for smooth curves and abelian varieties; however, there is a gap in the proof which we shall correct in Lemma 3.12 below.
$C_{\ell }(X,H^{i})$
holds for smooth curves and abelian varieties; however, there is a gap in the proof which we shall correct in Lemma 3.12 below.
Next let us turn to the unipotent fundamental group
![]() $\unicode[STIX]{x1D70B}_{1}$
, whose definition we recalled in the previous section. When
$\unicode[STIX]{x1D70B}_{1}$
, whose definition we recalled in the previous section. When
![]() $X/F$
is a geometrically connected variety with base point
$X/F$
is a geometrically connected variety with base point
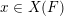 $x\in X(F)$
, and
$x\in X(F)$
, and
![]() $\ell \neq p$
, we may again consider the
$\ell \neq p$
, we may again consider the
![]() $\ell$
-adic unipotent fundamental group
$\ell$
-adic unipotent fundamental group
 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
as a
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}$
as a
![]() $G_{F}$
-representation, and we will write
$G_{F}$
-representation, and we will write
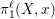 $\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
for the associated
$\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
for the associated
![]() $W_{F}^{\prime }$
-representation (with values in the fibred category of pro-unipotent group schemes). When
$W_{F}^{\prime }$
-representation (with values in the fibred category of pro-unipotent group schemes). When
 $\operatorname{char}(F)=\ell =p$
we use the rigid fundamental group (
$\operatorname{char}(F)=\ell =p$
we use the rigid fundamental group (
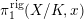 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
when
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/K,x)$
when
![]() $F=k$
is finite,
$F=k$
is finite,
 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)$
when
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)$
when
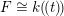 $F\cong k(\!(t)\!)$
is local) to produce a
$F\cong k(\!(t)\!)$
is local) to produce a
![]() $p$
-adic representation of
$p$
-adic representation of
![]() $W_{F}^{\prime }$
, with values in the fibred category of pro-unipotent group schemes, which we will denote by
$W_{F}^{\prime }$
, with values in the fibred category of pro-unipotent group schemes, which we will denote by
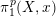 $\unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
(again using Marmora’s functor from
$\unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
(again using Marmora’s functor from
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules to Weil–Deligne representations when
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules to Weil–Deligne representations when
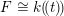 $F\cong k(\!(t)\!)$
). When
$F\cong k(\!(t)\!)$
). When
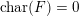 $\operatorname{char}(F)=0$
,
$\operatorname{char}(F)=0$
,
![]() $\ell =p$
and
$\ell =p$
and
![]() $X$
is a semistable curve we note that by [Reference Andreatta, Iovita and KimAIK15, Theorem 1.8] the
$X$
is a semistable curve we note that by [Reference Andreatta, Iovita and KimAIK15, Theorem 1.8] the
![]() $p$
-adic étale pro-unipotent fundamental group
$p$
-adic étale pro-unipotent fundamental group
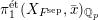 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{p}}$
is de Rham as a non-abelian representation of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{p}}$
is de Rham as a non-abelian representation of
![]() $G_{F}$
, hence we may again associate to it a
$G_{F}$
, hence we may again associate to it a
![]() $K^{\text{un}}$
-valued (non-abelian) Weil–Deligne representation
$K^{\text{un}}$
-valued (non-abelian) Weil–Deligne representation
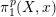 $\unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
. For more general
$\unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
. For more general
![]() $X$
, there are results of Déglise and Nizioł in [Reference Déglise and NiziołDN15] which again state that
$X$
, there are results of Déglise and Nizioł in [Reference Déglise and NiziołDN15] which again state that
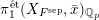 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{p}}$
is de Rham.
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{p}}$
is de Rham.
We will write
![]() $A_{\ell ,X,x}$
for the Hopf algebra of
$A_{\ell ,X,x}$
for the Hopf algebra of
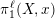 $\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
,
$\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
,
![]() $L_{\ell ,X,x}$
for its Lie algebra,
$L_{\ell ,X,x}$
for its Lie algebra,
![]() $\hat{{\mathcal{U}}}_{\ell ,X,x}$
for the completed universal enveloping algebra of
$\hat{{\mathcal{U}}}_{\ell ,X,x}$
for the completed universal enveloping algebra of
![]() $L_{\ell ,X,x}$
and
$L_{\ell ,X,x}$
and
![]() $\mathfrak{a}_{\ell ,X,x}$
for the augmentation ideal of
$\mathfrak{a}_{\ell ,X,x}$
for the augmentation ideal of
![]() $\hat{{\mathcal{U}}}_{\ell ,X,x}$
(these are representations in Hopf algebras, pro-nilpotent Lie algebras and associative algebras respectively, and for each
$\hat{{\mathcal{U}}}_{\ell ,X,x}$
(these are representations in Hopf algebras, pro-nilpotent Lie algebras and associative algebras respectively, and for each
![]() $k\geqslant 1$
we may consider
$k\geqslant 1$
we may consider
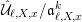 $\hat{{\mathcal{U}}}_{\ell ,X,x}/\mathfrak{a}_{\ell ,X,x}^{k}$
as a Weil–Deligne representation in vector spaces). The strongest form of
$\hat{{\mathcal{U}}}_{\ell ,X,x}/\mathfrak{a}_{\ell ,X,x}^{k}$
as a Weil–Deligne representation in vector spaces). The strongest form of
![]() $\ell$
-independence one can state for fundamental groups is the following.
$\ell$
-independence one can state for fundamental groups is the following.
Conjecture 3.9 (
 $C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})$
).
$C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})$
).
The collection
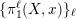 $\{\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)\}_{\ell }$
of pro-unipotent groups with an action of
$\{\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)\}_{\ell }$
of pro-unipotent groups with an action of
![]() $W_{F}^{\prime }$
is
$W_{F}^{\prime }$
is
![]() $\mathbb{Q}$
-compatible. Equivalently the collection
$\mathbb{Q}$
-compatible. Equivalently the collection
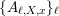 $\{A_{\ell ,X,x}\}_{\ell }$
(respectively,
$\{A_{\ell ,X,x}\}_{\ell }$
(respectively,
 $\{L_{\ell ,X,x}\}_{\ell }$
,
$\{L_{\ell ,X,x}\}_{\ell }$
,
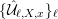 $\{\hat{{\mathcal{U}}}_{\ell ,X,x}\}_{\ell }$
) of Hopf algebras (respectively, Lie algebras, associative algebras) is
$\{\hat{{\mathcal{U}}}_{\ell ,X,x}\}_{\ell }$
) of Hopf algebras (respectively, Lie algebras, associative algebras) is
![]() $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible.
We will also be interested in the following weakening of the above conjecture.
Conjecture 3.10 (
 $C_{\ell }(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet }),C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
).
$C_{\ell }(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet }),C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
).
Fix
![]() $k\geqslant 1$
. Then the system
$k\geqslant 1$
. Then the system
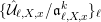 $\{\hat{{\mathcal{U}}}_{\ell ,X,x}/\mathfrak{a}_{\ell ,X,x}^{k}\}_{\ell }$
of
$\{\hat{{\mathcal{U}}}_{\ell ,X,x}/\mathfrak{a}_{\ell ,X,x}^{k}\}_{\ell }$
of
![]() $W_{F}^{\prime }$
-representations is (weakly)
$W_{F}^{\prime }$
-representations is (weakly)
![]() $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible.
Remark 3.11. Note that in this conjecture we only require compatibility as
![]() $W_{F}^{\prime }$
-representations, that is, we are saying nothing about the algebra structure. We clearly have the implications
$W_{F}^{\prime }$
-representations, that is, we are saying nothing about the algebra structure. We clearly have the implications
 $C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})\Rightarrow C_{\ell }(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })\Rightarrow C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
.
$C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})\Rightarrow C_{\ell }(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })\Rightarrow C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
.
We would like to finish this section by fixing a hole in the proof of [Reference Lazda and PálLP16, Theorem 5.85], stating that if
 $F\cong k(\!(t)\!)$
is equicharacteristic local and
$F\cong k(\!(t)\!)$
is equicharacteristic local and
![]() $X/F$
is a smooth curve or an abelian variety, then
$X/F$
is a smooth curve or an abelian variety, then
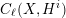 $C_{\ell }(X,H^{i})$
holds; in fact we only showed there that
$C_{\ell }(X,H^{i})$
holds; in fact we only showed there that
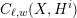 $C_{\ell ,w}(X,H^{i})$
holds, that is, weak
$C_{\ell ,w}(X,H^{i})$
holds, that is, weak
![]() $\ell$
-independence. Given Lemma 3.7, the next result completes the proof of ‘strong’
$\ell$
-independence. Given Lemma 3.7, the next result completes the proof of ‘strong’
![]() $\ell$
-independence in these cases.
$\ell$
-independence in these cases.
Lemma 3.12. Let
 $F\cong k(\!(t)\!)$
and
$F\cong k(\!(t)\!)$
and
![]() $X/F$
be either a smooth curve or an abelian variety. Then
$X/F$
be either a smooth curve or an abelian variety. Then
![]() $H_{\ell }^{1}(X)$
is Frobenius semisimple for all
$H_{\ell }^{1}(X)$
is Frobenius semisimple for all
![]() $\ell$
.
$\ell$
.
Proof. First suppose
![]() $X=A$
is an abelian variety. The proof of [Reference Lazda and PálLP16, Theorem 5.88] shows that the graded pieces of the monodromy filtration on
$X=A$
is an abelian variety. The proof of [Reference Lazda and PálLP16, Theorem 5.88] shows that the graded pieces of the monodromy filtration on
![]() $H_{\ell }^{1}(A)$
(for any
$H_{\ell }^{1}(A)$
(for any
![]() $\ell$
, including
$\ell$
, including
![]() $\ell =p$
) are given by the following:
$\ell =p$
) are given by the following:
-
– the cohomology
 $H_{\ell }^{1}(T)$
of a torus over
$H_{\ell }^{1}(T)$
of a torus over
 $F$
;
$F$
; -
– the cohomology
 $H_{\ell }^{1}(B)$
of an abelian variety with potentially good reduction;
$H_{\ell }^{1}(B)$
of an abelian variety with potentially good reduction; -
– the Weil–Deligne representation coming from a continuous representation
 $G_{F}\rightarrow \mathbb{Z}^{s}$
for some
$G_{F}\rightarrow \mathbb{Z}^{s}$
for some
 $s\geqslant 0$
.
$s\geqslant 0$
.
Frobenius semisimplicity of the first and third are clear (since they both factor through a finite quotient of
![]() $G_{F}$
), while the second follows from Frobenius semisimplicity for abelian varieties over finite fields. Since the three graded pieces are of different weights, Frobenius semisimplicity of
$G_{F}$
), while the second follows from Frobenius semisimplicity for abelian varieties over finite fields. Since the three graded pieces are of different weights, Frobenius semisimplicity of
![]() $H_{\ell }^{1}(A)$
follows. The deduction for smooth (possibly open) curves now follows exactly as in [Reference Lazda and PálLP16, § 5.4].◻
$H_{\ell }^{1}(A)$
follows. The deduction for smooth (possibly open) curves now follows exactly as in [Reference Lazda and PálLP16, § 5.4].◻
4 Unipotent fundamental groups over finite fields
In this section, we will prove the following result.
Theorem 4.1. Let
![]() $F=k$
be a finite field, and
$F=k$
be a finite field, and
![]() $X/F$
a smooth, projective variety. Then
$X/F$
a smooth, projective variety. Then
 $C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})$
holds.
$C_{\ell }(X,\unicode[STIX]{x1D70B}_{1}^{\text{uni}})$
holds.
Let us first note the following consequence of the weak Lefschetz theorem.
Proposition 4.2. To prove Theorem 4.1 it suffices to consider
![]() $X$
of dimension at most
$X$
of dimension at most
![]() $2$
.
$2$
.
Proof. If
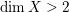 $\dim X>2$
then choose an iterated hyperplane section
$\dim X>2$
then choose an iterated hyperplane section
 $Y{\hookrightarrow}X$
passing through
$Y{\hookrightarrow}X$
passing through
![]() $x$
with
$x$
with
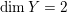 $\dim Y=2$
. The weak Lefschetz theorem [Reference GrothendieckSGA2, Exposé XII, Corollaire 3.5] says that the map
$\dim Y=2$
. The weak Lefschetz theorem [Reference GrothendieckSGA2, Exposé XII, Corollaire 3.5] says that the map
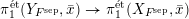 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(Y_{F^{\text{sep}}},\bar{x})\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})$
is an isomorphism, hence the same is true of their
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(Y_{F^{\text{sep}}},\bar{x})\rightarrow \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})$
is an isomorphism, hence the same is true of their
![]() $\mathbb{Q}_{\ell }$
-unipotent completions; in other words,
$\mathbb{Q}_{\ell }$
-unipotent completions; in other words,
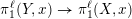 $\unicode[STIX]{x1D70B}_{1}^{\ell }(Y,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
is an isomorphism for
$\unicode[STIX]{x1D70B}_{1}^{\ell }(Y,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
is an isomorphism for
![]() $\ell \neq p$
.
$\ell \neq p$
.
When
![]() $\ell =p$
we use the weak Lefschetz theorem slightly differently. We claim that for any unipotent isocrystal
$\ell =p$
we use the weak Lefschetz theorem slightly differently. We claim that for any unipotent isocrystal
![]() $E$
on
$E$
on
![]() $X/K$
, the induced map
$X/K$
, the induced map
 $$\begin{eqnarray}H_{\text{rig}}^{i}(X/K,E)\rightarrow H_{\text{rig}}^{i}(Y/K,E|_{Y})\end{eqnarray}$$
$$\begin{eqnarray}H_{\text{rig}}^{i}(X/K,E)\rightarrow H_{\text{rig}}^{i}(Y/K,E|_{Y})\end{eqnarray}$$
is an isomorphism for
![]() $i=0,1$
and injective for
$i=0,1$
and injective for
![]() $i=2$
; this suffices to prove
$i=2$
; this suffices to prove
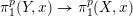 $\unicode[STIX]{x1D70B}_{1}^{p}(Y,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
is an isomorphism by combining [Reference Chiarellotto and Le StumCL99, Propositions 1.2.2 and 1.3.1] with Tannakian duality. If
$\unicode[STIX]{x1D70B}_{1}^{p}(Y,x)\rightarrow \unicode[STIX]{x1D70B}_{1}^{p}(X,x)$
is an isomorphism by combining [Reference Chiarellotto and Le StumCL99, Propositions 1.2.2 and 1.3.1] with Tannakian duality. If
![]() $E$
is constant then this is simply the usual weak Lefschetz. In general one inducts on the degree of unipotence and uses the five lemma.◻
$E$
is constant then this is simply the usual weak Lefschetz. In general one inducts on the degree of unipotence and uses the five lemma.◻
The key ingredient in the proof of Theorem 4.1 is the following concrete description of the Lie algebra
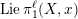 $\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
, due to Pridham [Reference PridhamPri09].
$\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
, due to Pridham [Reference PridhamPri09].
Proposition 4.3. Let
 $L(H_{\ell }^{1}(X)^{\vee })$
denote the free Lie algebra on the dual of
$L(H_{\ell }^{1}(X)^{\vee })$
denote the free Lie algebra on the dual of
![]() $H_{\ell }^{1}(X)$
, and
$H_{\ell }^{1}(X)$
, and
![]() $I_{\ell }$
the ideal generated by the image of the dual of the cup product
$I_{\ell }$
the ideal generated by the image of the dual of the cup product
 $$\begin{eqnarray}H_{\ell }^{2}(X)^{\vee }\overset{\cup _{\ell }^{\vee }}{\rightarrow }H_{\ell }^{1}(X)^{\vee }\otimes H_{\ell }^{1}(X)^{\vee }.\end{eqnarray}$$
$$\begin{eqnarray}H_{\ell }^{2}(X)^{\vee }\overset{\cup _{\ell }^{\vee }}{\rightarrow }H_{\ell }^{1}(X)^{\vee }\otimes H_{\ell }^{1}(X)^{\vee }.\end{eqnarray}$$
Then there is a
![]() $W_{F}$
-equivariant isomorphism
$W_{F}$
-equivariant isomorphism
 $$\begin{eqnarray}L_{\ell ,X,x}=\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)\cong \frac{L(H_{\ell }^{1}(X)^{\vee })}{I_{\ell }}.\end{eqnarray}$$
$$\begin{eqnarray}L_{\ell ,X,x}=\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)\cong \frac{L(H_{\ell }^{1}(X)^{\vee })}{I_{\ell }}.\end{eqnarray}$$
Proof. For
![]() $\ell \neq p$
this is [Reference PridhamPri09, Corollary 2.15]. When
$\ell \neq p$
this is [Reference PridhamPri09, Corollary 2.15]. When
![]() $\ell =p$
exactly the same proof works, using the
$\ell =p$
exactly the same proof works, using the
![]() $p$
-adic formalism of weights (see, for example, [Reference KedlayaKed06]).◻
$p$
-adic formalism of weights (see, for example, [Reference KedlayaKed06]).◻
Since Frobenius semisimplicity is known for
![]() $H_{\ell }^{1}(X)$
for
$H_{\ell }^{1}(X)$
for
![]() $X$
smooth and projective of any dimension, and any
$X$
smooth and projective of any dimension, and any
![]() $\ell$
, we deduce the following corollary.
$\ell$
, we deduce the following corollary.
Corollary 4.4. To prove Theorem 4.1, it suffices to show that the image of the cup product
 $$\begin{eqnarray}H_{\ell }^{1}(X)\otimes H_{\ell }^{1}(X)\overset{\cup _{\ell }}{\rightarrow }H_{\ell }^{2}(X)\end{eqnarray}$$
$$\begin{eqnarray}H_{\ell }^{1}(X)\otimes H_{\ell }^{1}(X)\overset{\cup _{\ell }}{\rightarrow }H_{\ell }^{2}(X)\end{eqnarray}$$
satisfies weak
![]() $\ell$
-independence, that is, the family of representations
$\ell$
-independence, that is, the family of representations
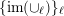 $\{\operatorname{im}(\cup _{\ell })\}_{\ell }$
is weakly
$\{\operatorname{im}(\cup _{\ell })\}_{\ell }$
is weakly
![]() $\mathbb{Q}$
-compatible.
$\mathbb{Q}$
-compatible.
Clearly this holds when
![]() $X$
is a smooth, projective curve, since then the cup product map is surjective. When
$X$
is a smooth, projective curve, since then the cup product map is surjective. When
![]() $X$
is a surface, we use the existence of Künneth projectors on
$X$
is a surface, we use the existence of Künneth projectors on
![]() $X$
.
$X$
.
Proposition 4.5 [Reference KleimanKle68, Corollary 2A10 and Lemma 2.4].
Let
![]() $X/F$
be a smooth projective surface. Then there exist correspondences
$X/F$
be a smooth projective surface. Then there exist correspondences
 $\unicode[STIX]{x1D71B}_{i}\in \operatorname{CH}_{2}(X\times X)$
such that for all
$\unicode[STIX]{x1D71B}_{i}\in \operatorname{CH}_{2}(X\times X)$
such that for all
![]() $\ell$
, the action of
$\ell$
, the action of
![]() $\unicode[STIX]{x1D71B}_{i}$
on cohomology
$\unicode[STIX]{x1D71B}_{i}$
on cohomology
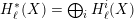 $H_{\ell }^{\ast }(X)=\bigoplus _{i}H_{\ell }^{i}(X)$
is to project to the summand
$H_{\ell }^{\ast }(X)=\bigoplus _{i}H_{\ell }^{i}(X)$
is to project to the summand
![]() $H_{\ell }^{i}(X)$
.
$H_{\ell }^{i}(X)$
.
We can now complete the proof of Theorem 4.1.
Proof of Theorem 4.1.
We may assume that
 $\dim X=2$
. Choose
$\dim X=2$
. Choose
 $\unicode[STIX]{x1D71B}_{1}\in \operatorname{CH}_{2}(X\times X)$
as above, and let
$\unicode[STIX]{x1D71B}_{1}\in \operatorname{CH}_{2}(X\times X)$
as above, and let
 $\unicode[STIX]{x1D71B}_{1\otimes 1}:=\unicode[STIX]{x1D71B}_{1}\times \unicode[STIX]{x1D71B}_{1}\in \operatorname{CH}_{4}(X^{4})$
. Then the effect of
$\unicode[STIX]{x1D71B}_{1\otimes 1}:=\unicode[STIX]{x1D71B}_{1}\times \unicode[STIX]{x1D71B}_{1}\in \operatorname{CH}_{4}(X^{4})$
. Then the effect of
![]() $\unicode[STIX]{x1D71B}_{1\otimes 1}$
on
$\unicode[STIX]{x1D71B}_{1\otimes 1}$
on
 $H_{\ell }^{\ast }(X\times X)$
is to project to the summand
$H_{\ell }^{\ast }(X\times X)$
is to project to the summand
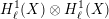 $H_{\ell }^{1}(X)\otimes H_{\ell }^{1}(X)$
.
$H_{\ell }^{1}(X)\otimes H_{\ell }^{1}(X)$
.
Now let
![]() $\unicode[STIX]{x1D71B}_{1\cup 1}$
be the pullback of
$\unicode[STIX]{x1D71B}_{1\cup 1}$
be the pullback of
![]() $\unicode[STIX]{x1D71B}_{1\otimes 1}$
to
$\unicode[STIX]{x1D71B}_{1\otimes 1}$
to
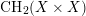 $\operatorname{CH}_{2}(X\times X)$
via
$\operatorname{CH}_{2}(X\times X)$
via
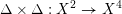 $\unicode[STIX]{x1D6E5}\times \unicode[STIX]{x1D6E5}:X^{2}\rightarrow X^{4}$
. The effect of
$\unicode[STIX]{x1D6E5}\times \unicode[STIX]{x1D6E5}:X^{2}\rightarrow X^{4}$
. The effect of
![]() $\unicode[STIX]{x1D71B}_{1\cup 1}$
on
$\unicode[STIX]{x1D71B}_{1\cup 1}$
on
![]() $H_{\ell }^{\ast }(X)$
is therefore to project onto the image of
$H_{\ell }^{\ast }(X)$
is therefore to project onto the image of
![]() $\cup _{\ell }$
inside
$\cup _{\ell }$
inside
![]() $H_{\ell }^{2}(X)$
. Using Corollary 4.4, the result now follows by composing
$H_{\ell }^{2}(X)$
. Using Corollary 4.4, the result now follows by composing
![]() $\unicode[STIX]{x1D71B}_{1\cup 1}$
with the graph of (some power of) Frobenius and applying the Lefschetz fixed point formula.◻
$\unicode[STIX]{x1D71B}_{1\cup 1}$
with the graph of (some power of) Frobenius and applying the Lefschetz fixed point formula.◻
5 Cohomology over equicharacteristic local fields I
The main result of this section is the following theorem.
Theorem 5.1. Suppose that
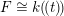 $F\cong k(\!(t)\!)$
is equicharacteristic local, and let
$F\cong k(\!(t)\!)$
is equicharacteristic local, and let
![]() $X/F$
be a smooth, proper variety with semistable reduction. Then
$X/F$
be a smooth, proper variety with semistable reduction. Then
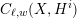 $C_{\ell ,w}(X,H^{i})$
holds.
$C_{\ell ,w}(X,H^{i})$
holds.
This is the main step in showing such
![]() $\ell$
-independence unconditionally for smooth and proper varieties over
$\ell$
-independence unconditionally for smooth and proper varieties over
![]() $F$
. The first key result we need in the proof of Theorem 5.1 essentially allows us to reduce to the ‘globally defined’ case, and in fact works in slightly larger generality than we will need. So for now let
$F$
. The first key result we need in the proof of Theorem 5.1 essentially allows us to reduce to the ‘globally defined’ case, and in fact works in slightly larger generality than we will need. So for now let
![]() $S$
denote a Dedekind scheme,
$S$
denote a Dedekind scheme,
![]() $s\in S$
a closed point and
$s\in S$
a closed point and
![]() $\widehat{{\mathcal{O}}}_{S,s}$
the completed local ring at
$\widehat{{\mathcal{O}}}_{S,s}$
the completed local ring at
![]() $s$
. By definition a semistable scheme over
$s$
. By definition a semistable scheme over
![]() $\widehat{{\mathcal{O}}}_{S,s}$
is one that is étale locally smooth over
$\widehat{{\mathcal{O}}}_{S,s}$
is one that is étale locally smooth over
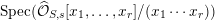 $\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s}[x_{1},\ldots ,x_{r}]/(x_{1}\cdots x_{r}))$
.
$\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s}[x_{1},\ldots ,x_{r}]/(x_{1}\cdots x_{r}))$
.
Proposition 5.2. Let
 ${\mathcal{X}}\rightarrow \operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s})$
be a semistable scheme of finite type and
${\mathcal{X}}\rightarrow \operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s})$
be a semistable scheme of finite type and
![]() $n\geqslant 2$
an integer. Then there exist an étale neighbourhood
$n\geqslant 2$
an integer. Then there exist an étale neighbourhood
 $(U,u)\rightarrow (S,s)$
of
$(U,u)\rightarrow (S,s)$
of
![]() $s$
and a flat scheme
$s$
and a flat scheme
![]() ${\mathcal{Y}}\rightarrow U$
, smooth away from
${\mathcal{Y}}\rightarrow U$
, smooth away from
![]() $u$
and semistable at
$u$
and semistable at
![]() $u$
, such that
$u$
, such that
 $$\begin{eqnarray}{\mathcal{Y}}\times _{U}\frac{{\mathcal{O}}_{U,u}}{\mathfrak{m}_{u}^{n}}\cong {\mathcal{X}}\times _{\widehat{{\mathcal{O}}}_{S,s}}\frac{\widehat{{\mathcal{O}}}_{S,s}}{\mathfrak{m}_{s}^{n}}\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{Y}}\times _{U}\frac{{\mathcal{O}}_{U,u}}{\mathfrak{m}_{u}^{n}}\cong {\mathcal{X}}\times _{\widehat{{\mathcal{O}}}_{S,s}}\frac{\widehat{{\mathcal{O}}}_{S,s}}{\mathfrak{m}_{s}^{n}}\end{eqnarray}$$
as schemes over
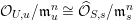 ${\mathcal{O}}_{U,u}/\mathfrak{m}_{u}^{n}\cong \widehat{{\mathcal{O}}}_{S,s}/\mathfrak{m}_{s}^{n}$
.
${\mathcal{O}}_{U,u}/\mathfrak{m}_{u}^{n}\cong \widehat{{\mathcal{O}}}_{S,s}/\mathfrak{m}_{s}^{n}$
.
Proof. Choose a local parameter
![]() $t$
at
$t$
at
![]() $s$
. After shrinking
$s$
. After shrinking
![]() $S$
we may assume that
$S$
we may assume that
 $S=\operatorname{Spec}(R)$
is affine and
$S=\operatorname{Spec}(R)$
is affine and
![]() $t\in R$
. Then there exists an étale cover
$t\in R$
. Then there exists an étale cover
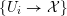 $\{U_{i}\rightarrow {\mathcal{X}}\}$
of
$\{U_{i}\rightarrow {\mathcal{X}}\}$
of
![]() ${\mathcal{X}}$
such that each
${\mathcal{X}}$
such that each
![]() $U_{i}$
is étale over some
$U_{i}$
is étale over some
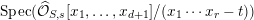 $\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s}[x_{1},\ldots ,x_{d+1}]/(x_{1}\cdots x_{r}-t))$
(with
$\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s}[x_{1},\ldots ,x_{d+1}]/(x_{1}\cdots x_{r}-t))$
(with
![]() $r$
and
$r$
and
![]() $d$
allowed to vary with
$d$
allowed to vary with
![]() $i$
.)
$i$
.)
Choose some finitely generated, normal
![]() $R$
-algebra
$R$
-algebra
![]() $A$
contained within
$A$
contained within
![]() $\widehat{{\mathcal{O}}}_{S,s}$
such that everything in sight is defined over
$\widehat{{\mathcal{O}}}_{S,s}$
such that everything in sight is defined over
![]() $A$
, namely,
$A$
, namely,
![]() ${\mathcal{X}}$
, the étale cover
${\mathcal{X}}$
, the étale cover
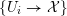 $\{U_{i}\rightarrow {\mathcal{X}}\}$
and the étale maps
$\{U_{i}\rightarrow {\mathcal{X}}\}$
and the étale maps
 $$\begin{eqnarray}U_{i}\rightarrow \operatorname{Spec}\biggl(\frac{A[x_{1},\ldots ,x_{d+1}]}{(x_{1}\cdots x_{r}-t)}\biggr).\end{eqnarray}$$
$$\begin{eqnarray}U_{i}\rightarrow \operatorname{Spec}\biggl(\frac{A[x_{1},\ldots ,x_{d+1}]}{(x_{1}\cdots x_{r}-t)}\biggr).\end{eqnarray}$$
Now by Artin’s approximation theorem, the point
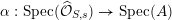 $\unicode[STIX]{x1D6FC}:\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s})\rightarrow \operatorname{Spec}(A)$
of the finite type
$\unicode[STIX]{x1D6FC}:\operatorname{Spec}(\widehat{{\mathcal{O}}}_{S,s})\rightarrow \operatorname{Spec}(A)$
of the finite type
![]() $R$
-scheme
$R$
-scheme
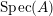 $\operatorname{Spec}(A)$
, coming from the inclusion
$\operatorname{Spec}(A)$
, coming from the inclusion
 $A\subset \widehat{{\mathcal{O}}}_{S,s}$
, can be approximated modulo
$A\subset \widehat{{\mathcal{O}}}_{S,s}$
, can be approximated modulo
![]() $t^{n}$
by a Henselian point; in other words, there exists a morphism
$t^{n}$
by a Henselian point; in other words, there exists a morphism
 $\unicode[STIX]{x1D6FC}^{h,n}:\operatorname{Spec}({\mathcal{O}}_{S,s}^{h})\rightarrow \operatorname{Spec}(A)$
which agrees with
$\unicode[STIX]{x1D6FC}^{h,n}:\operatorname{Spec}({\mathcal{O}}_{S,s}^{h})\rightarrow \operatorname{Spec}(A)$
which agrees with
![]() $\unicode[STIX]{x1D6FC}$
modulo
$\unicode[STIX]{x1D6FC}$
modulo
![]() $t^{n}$
. Then pulling back via
$t^{n}$
. Then pulling back via
![]() $\unicode[STIX]{x1D6FC}^{h,n}$
and using the fact that
$\unicode[STIX]{x1D6FC}^{h,n}$
and using the fact that
![]() $t$
is a local parameter at
$t$
is a local parameter at
![]() $s$
, we can see that there exists a semistable family
$s$
, we can see that there exists a semistable family
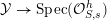 ${\mathcal{Y}}\rightarrow \operatorname{Spec}({\mathcal{O}}_{S,s}^{h})$
which agrees with
${\mathcal{Y}}\rightarrow \operatorname{Spec}({\mathcal{O}}_{S,s}^{h})$
which agrees with
![]() ${\mathcal{X}}$
modulo
${\mathcal{X}}$
modulo
![]() $t^{n}$
.
$t^{n}$
.
Now, finally, we note that there must be some finitely generated sub-algebra
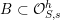 $B\subset {\mathcal{O}}_{S,s}^{h}$
, étale over
$B\subset {\mathcal{O}}_{S,s}^{h}$
, étale over
![]() $S$
such that
$S$
such that
![]() ${\mathcal{Y}}$
is defined over
${\mathcal{Y}}$
is defined over
![]() $B$
and smooth away from
$B$
and smooth away from
![]() $s$
. Setting
$s$
. Setting
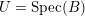 $U=\operatorname{Spec}(B)$
then completes the proof.◻
$U=\operatorname{Spec}(B)$
then completes the proof.◻
To apply this result, we will specialise, and let
 $R\cong k\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7}$
be the ring of integers inside
$R\cong k\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7}$
be the ring of integers inside
 $F\cong k(\!(t)\!)$
. Suppose that we are given a semistable scheme
$F\cong k(\!(t)\!)$
. Suppose that we are given a semistable scheme
![]() ${\mathcal{X}}/R$
. We will let
${\mathcal{X}}/R$
. We will let
![]() ${\mathcal{X}}^{\times }$
denote
${\mathcal{X}}^{\times }$
denote
![]() ${\mathcal{X}}$
endowed with the log structure given by the special fibre, and
${\mathcal{X}}$
endowed with the log structure given by the special fibre, and
![]() $X_{0}^{\times }$
the special fibre endowed with the inverse image log structure from
$X_{0}^{\times }$
the special fibre endowed with the inverse image log structure from
![]() ${\mathcal{X}}$
. Let
${\mathcal{X}}$
. Let
![]() $X$
denote the generic fibre of
$X$
denote the generic fibre of
![]() ${\mathcal{X}}$
. Then we may consider, as in [Reference NakayamaNak98], the log-étale cohomology
${\mathcal{X}}$
. Then we may consider, as in [Reference NakayamaNak98], the log-étale cohomology
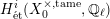 $H_{\acute{\text{e}}\text{t}}^{i}(X_{0}^{\times ,\text{tame}},\mathbb{Q}_{\ell })$
of the log scheme
$H_{\acute{\text{e}}\text{t}}^{i}(X_{0}^{\times ,\text{tame}},\mathbb{Q}_{\ell })$
of the log scheme
![]() $X_{0}^{\times }$
, as an
$X_{0}^{\times }$
, as an
![]() $\ell$
-adic representation of
$\ell$
-adic representation of
![]() $G_{F}$
(on which the wild inertia group
$G_{F}$
(on which the wild inertia group
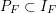 $P_{F}\subset I_{F}$
acts trivially). We have the following logarithmic analogue of the smooth and proper base change theorem.
$P_{F}\subset I_{F}$
acts trivially). We have the following logarithmic analogue of the smooth and proper base change theorem.
Proposition 5.3 [Reference NakayamaNak98, Proposition (4.2)].
There is an isomorphism
 $$\begin{eqnarray}H_{\acute{\text{e}}\text{t}}^{i}(X_{0}^{\times ,\text{tame}},\mathbb{Q}_{\ell })\cong H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\end{eqnarray}$$
$$\begin{eqnarray}H_{\acute{\text{e}}\text{t}}^{i}(X_{0}^{\times ,\text{tame}},\mathbb{Q}_{\ell })\cong H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\end{eqnarray}$$
of
![]() $G_{F}$
-representations.
$G_{F}$
-representations.
Of course, there is a similar result for
![]() $p$
-adic cohomology, which goes as follows. Let
$p$
-adic cohomology, which goes as follows. Let
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
denote the category of
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
denote the category of
![]() $(\unicode[STIX]{x1D711},N)$
-modules over
$(\unicode[STIX]{x1D711},N)$
-modules over
![]() $K$
, that is, finite-dimensional vector spaces together with a semilinear, bijective Frobenius
$K$
, that is, finite-dimensional vector spaces together with a semilinear, bijective Frobenius
![]() $\unicode[STIX]{x1D711}$
and a nilpotent endomorphism
$\unicode[STIX]{x1D711}$
and a nilpotent endomorphism
![]() $N$
, such that
$N$
, such that
 $N\unicode[STIX]{x1D711}=q\unicode[STIX]{x1D711}N$
. Given such a
$N\unicode[STIX]{x1D711}=q\unicode[STIX]{x1D711}N$
. Given such a
![]() $(\unicode[STIX]{x1D711},N)$
-module
$(\unicode[STIX]{x1D711},N)$
-module
![]() $V$
, we can put a connection on
$V$
, we can put a connection on
 $V\otimes _{K}{\mathcal{R}}_{K}$
by setting
$V\otimes _{K}{\mathcal{R}}_{K}$
by setting
 $\unicode[STIX]{x1D6FB}(v\otimes 1)=N(v)\otimes t^{-1}$
, and this induces a fully faithful functor
$\unicode[STIX]{x1D6FB}(v\otimes 1)=N(v)\otimes t^{-1}$
, and this induces a fully faithful functor
 $$\begin{eqnarray}-\otimes _{K}\,{\mathcal{R}}_{K}:\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{K}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
$$\begin{eqnarray}-\otimes _{K}\,{\mathcal{R}}_{K}:\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{K}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
from
![]() $(\unicode[STIX]{x1D711},N)$
-modules over
$(\unicode[STIX]{x1D711},N)$
-modules over
![]() $K$
to
$K$
to
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{R}}_{K}$
, whose essential image is exactly the objects for which the connection acts unipotently. For
${\mathcal{R}}_{K}$
, whose essential image is exactly the objects for which the connection acts unipotently. For
![]() ${\mathcal{X}}$
as above, we may consider the log-crystalline cohomology
${\mathcal{X}}$
as above, we may consider the log-crystalline cohomology
 $H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/K^{\times }):=H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/W^{\times })\otimes K$
of its special fibre as a
$H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/K^{\times }):=H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/W^{\times })\otimes K$
of its special fibre as a
![]() $(\unicode[STIX]{x1D711},N)$
-module over
$(\unicode[STIX]{x1D711},N)$
-module over
![]() $K$
, as in [Reference Hyodo and KatoHK94] (the notation
$K$
, as in [Reference Hyodo and KatoHK94] (the notation
![]() $W^{\times }$
,
$W^{\times }$
,
![]() $K^{\times }$
refers to the fact that we are endowing
$K^{\times }$
refers to the fact that we are endowing
![]() $W$
with the Teichmüller lift of the log structure of the punctured point).
$W$
with the Teichmüller lift of the log structure of the punctured point).
Proposition 5.4 [Reference Lazda and PálLP16, Theorem 5.46].
There is an isomorphism
 $$\begin{eqnarray}H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/K^{\times })\otimes _{K}{\mathcal{R}}\cong H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})\end{eqnarray}$$
$$\begin{eqnarray}H_{\log \text{-}\text{crys}}^{i}(X_{0}^{\times }/K^{\times })\otimes _{K}{\mathcal{R}}\cong H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})\end{eqnarray}$$
inside
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{K}}^{\unicode[STIX]{x1D6FB}}$
.
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{K}}^{\unicode[STIX]{x1D6FB}}$
.
We therefore obtain the following corollary.
Corollary 5.5. Let
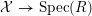 ${\mathcal{X}}\rightarrow \operatorname{Spec}(R)$
be a proper, semistable scheme with generic fibre
${\mathcal{X}}\rightarrow \operatorname{Spec}(R)$
be a proper, semistable scheme with generic fibre
![]() $X$
. Then there exist a smooth, geometrically connected curve
$X$
. Then there exist a smooth, geometrically connected curve
![]() $C/k$
, a
$C/k$
, a
![]() $k$
-rational point
$k$
-rational point
 $c\in C(k)$
, a proper, semistable scheme
$c\in C(k)$
, a proper, semistable scheme
![]() ${\mathcal{Y}}\rightarrow C$
, smooth away from
${\mathcal{Y}}\rightarrow C$
, smooth away from
![]() $c$
, and an isomorphism
$c$
, and an isomorphism
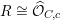 $R\cong \widehat{{\mathcal{O}}}_{C,c}$
(inducing
$R\cong \widehat{{\mathcal{O}}}_{C,c}$
(inducing
 $F\cong \widehat{k(C)}_{c}$
) such that
$F\cong \widehat{k(C)}_{c}$
) such that
 $$\begin{eqnarray}H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong H_{\acute{\text{e}}\text{t}}^{i}({\mathcal{Y}}_{F^{\text{sep}}},\mathbb{Q}_{\ell })\end{eqnarray}$$
$$\begin{eqnarray}H_{\acute{\text{e}}\text{t}}^{i}(X_{F^{\text{sep}}},\mathbb{Q}_{\ell })\cong H_{\acute{\text{e}}\text{t}}^{i}({\mathcal{Y}}_{F^{\text{sep}}},\mathbb{Q}_{\ell })\end{eqnarray}$$
as
![]() $G_{F}$
representations for all
$G_{F}$
representations for all
![]() $i,\ell \neq p$
, and
$i,\ell \neq p$
, and
 $$\begin{eqnarray}H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})\cong H_{\text{rig}}^{i}({\mathcal{Y}}_{F}/{\mathcal{R}}_{K})\end{eqnarray}$$
$$\begin{eqnarray}H_{\text{rig}}^{i}(X/{\mathcal{R}}_{K})\cong H_{\text{rig}}^{i}({\mathcal{Y}}_{F}/{\mathcal{R}}_{K})\end{eqnarray}$$
as
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules, for all
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules, for all
![]() $i$
. In particular,
$i$
. In particular,
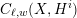 $C_{\ell ,w}(X,H^{i})$
is equivalent to
$C_{\ell ,w}(X,H^{i})$
is equivalent to
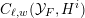 $C_{\ell ,w}({\mathcal{Y}}_{F},H^{i})$
.
$C_{\ell ,w}({\mathcal{Y}}_{F},H^{i})$
.
Proof. By Proposition 5.2 we know that there exists a globally defined semistable scheme which agrees with
![]() ${\mathcal{X}}$
up to order
${\mathcal{X}}$
up to order
![]() $2$
, hence the special fibres coincide as log schemes. Hence by Propositions 5.3 and 5.4 the cohomologies also coincide.◻
$2$
, hence the special fibres coincide as log schemes. Hence by Propositions 5.3 and 5.4 the cohomologies also coincide.◻
Our primary interest here is in
![]() $\ell$
-independence results; however, let us note in passing that Corollary 5.5 implies that the Clemens–Schmid exact sequence, obtained by the first author in collaboration with Tsuzuki in [Reference Chiarellotto and TsuzukiCT14], exists for
$\ell$
-independence results; however, let us note in passing that Corollary 5.5 implies that the Clemens–Schmid exact sequence, obtained by the first author in collaboration with Tsuzuki in [Reference Chiarellotto and TsuzukiCT14], exists for
![]() ${\mathcal{X}}$
.
${\mathcal{X}}$
.
Corollary 5.6. Let
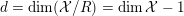 $d=\dim ({\mathcal{X}}/R)=\dim {\mathcal{X}}-1$
. There is a Clemens–Schmid long exact sequence
$d=\dim ({\mathcal{X}}/R)=\dim {\mathcal{X}}-1$
. There is a Clemens–Schmid long exact sequence
 $$\begin{eqnarray}\displaystyle \cdots \rightarrow H_{\text{rig}}^{m}(X_{0}/K)\rightarrow H_{\log \text{-}\text{crys}}^{m}(X_{0}^{\times }/K^{\times }) & \overset{N}{\rightarrow } & \displaystyle H_{\log \text{-}\text{crys}}^{m}(X_{0}^{\times }/K^{\times })(-1)\nonumber\\ \displaystyle & \rightarrow & \displaystyle H_{\text{rig}}^{2d-m}(X_{0}/K)^{\vee }(-d-1)\rightarrow \cdots \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \cdots \rightarrow H_{\text{rig}}^{m}(X_{0}/K)\rightarrow H_{\log \text{-}\text{crys}}^{m}(X_{0}^{\times }/K^{\times }) & \overset{N}{\rightarrow } & \displaystyle H_{\log \text{-}\text{crys}}^{m}(X_{0}^{\times }/K^{\times })(-1)\nonumber\\ \displaystyle & \rightarrow & \displaystyle H_{\text{rig}}^{2d-m}(X_{0}/K)^{\vee }(-d-1)\rightarrow \cdots \nonumber\end{eqnarray}$$
Proof. When
![]() ${\mathcal{X}}$
extends to a global semistable family, this is the main result of [Reference Chiarellotto and TsuzukiCT14]. Given Proposition 5.2, it therefore suffices to note that all terms in the exact sequence only depend on the log scheme
${\mathcal{X}}$
extends to a global semistable family, this is the main result of [Reference Chiarellotto and TsuzukiCT14]. Given Proposition 5.2, it therefore suffices to note that all terms in the exact sequence only depend on the log scheme
![]() $X_{0}^{\times }$
.◻
$X_{0}^{\times }$
.◻
To return to our main focus, then, to prove Theorem 5.1 it suffices to do so in the ‘globally defined’ case, that is, we may assume that we have a smooth geometrically connected curve
![]() $C/k$
,
$C/k$
,
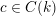 $c\in C(k)$
such that
$c\in C(k)$
such that
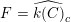 $F=\widehat{k(C)}_{c}$
, and a semistable scheme
$F=\widehat{k(C)}_{c}$
, and a semistable scheme
 ${\mathcal{X}}\rightarrow C$
, smooth away from
${\mathcal{X}}\rightarrow C$
, smooth away from
![]() $c$
, such that
$c$
, such that
 $X={\mathcal{X}}_{F}$
.
$X={\mathcal{X}}_{F}$
.
Let
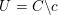 $U=C\setminus c$
, and consider a collection
$U=C\setminus c$
, and consider a collection
![]() $\{{\mathcal{F}}_{\ell }\}_{\ell }$
of local systems on
$\{{\mathcal{F}}_{\ell }\}_{\ell }$
of local systems on
![]() $U$
, where
$U$
, where
![]() ${\mathcal{F}}_{\ell }$
is a lisse
${\mathcal{F}}_{\ell }$
is a lisse
![]() $\mathbb{Q}_{\ell }$
sheaf on
$\mathbb{Q}_{\ell }$
sheaf on
![]() $U$
for
$U$
for
![]() $\ell \neq p$
and
$\ell \neq p$
and
![]() ${\mathcal{F}}_{p}$
is an overconvergent
${\mathcal{F}}_{p}$
is an overconvergent
![]() $F$
-isocrystal on
$F$
-isocrystal on
![]() $U/K$
(here
$U/K$
(here
![]() $F$
is the
$F$
is the
![]() $q=\#k$
-power Frobenius). Then for any closed point
$q=\#k$
-power Frobenius). Then for any closed point
![]() $x\in U$
the action of geometric Frobenius on
$x\in U$
the action of geometric Frobenius on
![]() ${\mathcal{F}}_{\ell ,\bar{x}}$
for
${\mathcal{F}}_{\ell ,\bar{x}}$
for
![]() $\ell \neq p$
, plus the action of
$\ell \neq p$
, plus the action of
![]() $F^{\deg (x)}$
on
$F^{\deg (x)}$
on
![]() ${\mathcal{F}}_{p,x}$
, defines a collection
${\mathcal{F}}_{p,x}$
, defines a collection
![]() $\{{\mathcal{F}}_{\ell ,x}\}_{\ell }$
of representations of the Weil group
$\{{\mathcal{F}}_{\ell ,x}\}_{\ell }$
of representations of the Weil group
![]() $W_{k(x)}$
.
$W_{k(x)}$
.
Definition 5.7. (i) We say that the system
![]() $\{{\mathcal{F}}_{\ell }\}$
is weakly
$\{{\mathcal{F}}_{\ell }\}$
is weakly
![]() $\mathbb{Q}$
-compatible if
$\mathbb{Q}$
-compatible if
![]() $\{{\mathcal{F}}_{\ell ,x}\}_{\ell }$
is so for all closed points
$\{{\mathcal{F}}_{\ell ,x}\}_{\ell }$
is so for all closed points
![]() $x\in U$
.
$x\in U$
.
(ii) We say that the system
![]() $\{{\mathcal{F}}_{\ell }\}$
is pure of weight
$\{{\mathcal{F}}_{\ell }\}$
is pure of weight
![]() $n$
if for each closed point
$n$
if for each closed point
![]() $x\in U$
the eigenvalues of
$x\in U$
the eigenvalues of
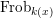 $\operatorname{Frob}_{k(x)}$
(respectively,
$\operatorname{Frob}_{k(x)}$
(respectively,
![]() $F^{\deg (x)}$
) on
$F^{\deg (x)}$
) on
![]() ${\mathcal{F}}_{\ell ,\bar{x}}$
(respectively,
${\mathcal{F}}_{\ell ,\bar{x}}$
(respectively,
![]() ${\mathcal{F}}_{p,x}$
) are Weil numbers of weight
${\mathcal{F}}_{p,x}$
) are Weil numbers of weight
![]() $n$
.
$n$
.
Given such a system, we may also restrict to the Weil–Deligne group at
![]() $c$
. When
$c$
. When
![]() $\ell \neq p$
, this is fairly standard, since we may consider the Galois group
$\ell \neq p$
, this is fairly standard, since we may consider the Galois group
![]() $G_{F}$
as a decomposition group at
$G_{F}$
as a decomposition group at
![]() $c$
, and hence restrict the
$c$
, and hence restrict the
![]() $\ell$
-adic representation
$\ell$
-adic representation
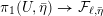 $\unicode[STIX]{x1D70B}_{1}(U,\bar{\unicode[STIX]{x1D702}})\rightarrow {\mathcal{F}}_{\ell ,\bar{\unicode[STIX]{x1D702}}}$
of the fundamental group of
$\unicode[STIX]{x1D70B}_{1}(U,\bar{\unicode[STIX]{x1D702}})\rightarrow {\mathcal{F}}_{\ell ,\bar{\unicode[STIX]{x1D702}}}$
of the fundamental group of
![]() $U$
(based at some geometric generic point) to get an
$U$
(based at some geometric generic point) to get an
![]() $\ell$
-adic representation of
$\ell$
-adic representation of
![]() $G_{F}$
, and hence a Weil–Deligne representation, which we shall call
$G_{F}$
, and hence a Weil–Deligne representation, which we shall call
![]() ${\mathcal{F}}_{\ell ,c}$
. When
${\mathcal{F}}_{\ell ,c}$
. When
![]() $\ell =p$
we use the construction of [Reference TsuzukiTsu98, § 6.1]. Pulling back to an appropriate tubular neighbourhood of
$\ell =p$
we use the construction of [Reference TsuzukiTsu98, § 6.1]. Pulling back to an appropriate tubular neighbourhood of
![]() $c$
gives a functor
$c$
gives a functor
 $$\begin{eqnarray}F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{c}}^{\unicode[STIX]{x1D6FB}},\end{eqnarray}$$
$$\begin{eqnarray}F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{c}}^{\unicode[STIX]{x1D6FB}},\end{eqnarray}$$
where
![]() ${\mathcal{R}}_{c}$
is a copy of the Robba ring at
${\mathcal{R}}_{c}$
is a copy of the Robba ring at
![]() $c$
. Then applying Marmora’s functor from
$c$
. Then applying Marmora’s functor from
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring to Weil–Deligne representations [Reference MarmoraMar08] gives us a
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over the Robba ring to Weil–Deligne representations [Reference MarmoraMar08] gives us a
![]() $K^{\text{un}}$
-valued representation of
$K^{\text{un}}$
-valued representation of
![]() $W_{F}^{\prime }$
which we shall call
$W_{F}^{\prime }$
which we shall call
![]() ${\mathcal{F}}_{p,c}$
. The key result is then the following, whose proof we will defer to § 7 below.
${\mathcal{F}}_{p,c}$
. The key result is then the following, whose proof we will defer to § 7 below.
Proposition 5.8. If
![]() $\{{\mathcal{F}}_{\ell }\}_{\ell }$
is a weakly
$\{{\mathcal{F}}_{\ell }\}_{\ell }$
is a weakly
![]() $\mathbb{Q}$
-compatible system on
$\mathbb{Q}$
-compatible system on
![]() $U$
, which is moreover pure of some weight
$U$
, which is moreover pure of some weight
![]() $n$
, then
$n$
, then
![]() $\{{\mathcal{F}}_{\ell ,c}\}_{\ell }$
is a weakly
$\{{\mathcal{F}}_{\ell ,c}\}_{\ell }$
is a weakly
![]() $\mathbb{Q}$
-compatible system of Weil–Deligne representations.
$\mathbb{Q}$
-compatible system of Weil–Deligne representations.
We can now complete the proof of Theorem 5.1
Proof of Theorem 5.1.
We may assume that
![]() $X$
is globally defined, that is, we have
$X$
is globally defined, that is, we have
 $C,c,U,{\mathcal{X}}$
as above such that
$C,c,U,{\mathcal{X}}$
as above such that
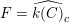 $F=\widehat{k(C)}_{c}$
and
$F=\widehat{k(C)}_{c}$
and
 ${\mathcal{X}}_{F}\cong X$
. We will apply Proposition 5.8, taking
${\mathcal{X}}_{F}\cong X$
. We will apply Proposition 5.8, taking
![]() ${\mathcal{F}}_{\ell }$
to be the
${\mathcal{F}}_{\ell }$
to be the
![]() $i$
th higher direct image of the constant sheaf under the map
$i$
th higher direct image of the constant sheaf under the map
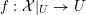 $f:{\mathcal{X}}|_{U}\rightarrow U$
. Hence for
$f:{\mathcal{X}}|_{U}\rightarrow U$
. Hence for
![]() $\ell \neq p$
we take
$\ell \neq p$
we take
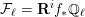 ${\mathcal{F}}_{\ell }=\mathbf{R}^{i}f_{\ast }\mathbb{Q}_{\ell }$
, and for
${\mathcal{F}}_{\ell }=\mathbf{R}^{i}f_{\ast }\mathbb{Q}_{\ell }$
, and for
![]() $\ell =p$
we take
$\ell =p$
we take
![]() ${\mathcal{F}}_{p}$
to be the overconvergent
${\mathcal{F}}_{p}$
to be the overconvergent
![]() $F$
-isocrystal
$F$
-isocrystal
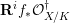 $\mathbf{R}^{i}f_{\ast }{\mathcal{O}}_{X/K}^{\dagger }$
on
$\mathbf{R}^{i}f_{\ast }{\mathcal{O}}_{X/K}^{\dagger }$
on
![]() $U/K$
constructed by Matsuda and Trihan in [Reference Matsuda and TrihanMT04]. By smooth and proper base change in étale cohomology we have, for any closed point
$U/K$
constructed by Matsuda and Trihan in [Reference Matsuda and TrihanMT04]. By smooth and proper base change in étale cohomology we have, for any closed point
![]() $x\in U$
,
$x\in U$
,
 $$\begin{eqnarray}{\mathcal{F}}_{\ell ,\overline{x}}=H_{\acute{\text{e}}\text{t}}^{i}({\mathcal{X}}_{\bar{x}},\mathbb{Q}_{\ell }),\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{F}}_{\ell ,\overline{x}}=H_{\acute{\text{e}}\text{t}}^{i}({\mathcal{X}}_{\bar{x}},\mathbb{Q}_{\ell }),\end{eqnarray}$$
and by the same result for Ogus’s convergent cohomology [Reference OgusOgu84, Proposition 3.5] we have
 $$\begin{eqnarray}{\mathcal{F}}_{p,x}=H_{\text{rig}}^{i}({\mathcal{X}}_{x}/K(x)),\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{F}}_{p,x}=H_{\text{rig}}^{i}({\mathcal{X}}_{x}/K(x)),\end{eqnarray}$$
where
![]() $K(x)$
is the fraction field of the Witt vectors of
$K(x)$
is the fraction field of the Witt vectors of
![]() $k(x)$
. Hence by
$k(x)$
. Hence by
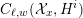 $C_{\ell ,w}({\mathcal{X}}_{x},H^{i})$
we know that
$C_{\ell ,w}({\mathcal{X}}_{x},H^{i})$
we know that
![]() $\{{\mathcal{F}}_{\ell }\}_{\ell }$
is a
$\{{\mathcal{F}}_{\ell }\}_{\ell }$
is a
![]() $\mathbb{Q}$
-compatible system on
$\mathbb{Q}$
-compatible system on
![]() $U$
, and by Proposition 5.8 it follows that
$U$
, and by Proposition 5.8 it follows that
![]() $\{{\mathcal{F}}_{\ell ,c}\}_{\ell }$
is a weakly
$\{{\mathcal{F}}_{\ell ,c}\}_{\ell }$
is a weakly
![]() $\mathbb{Q}$
-compatible system of Weil–Deligne representations. But again applying smooth and proper base change in étale cohomology tells us that
$\mathbb{Q}$
-compatible system of Weil–Deligne representations. But again applying smooth and proper base change in étale cohomology tells us that
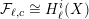 ${\mathcal{F}}_{\ell ,c}\cong H_{\ell }^{i}(X)$
for
${\mathcal{F}}_{\ell ,c}\cong H_{\ell }^{i}(X)$
for
![]() $\ell \neq p$
, and the proof of [Reference Lazda and PálLP16, Proposition 5.52] tells us that
$\ell \neq p$
, and the proof of [Reference Lazda and PálLP16, Proposition 5.52] tells us that
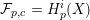 ${\mathcal{F}}_{p,c}=H_{p}^{i}(X)$
. The result follows.◻
${\mathcal{F}}_{p,c}=H_{p}^{i}(X)$
. The result follows.◻
6 Cohomology over equicharacteristic local fields II
In this section we generalise Theorem 5.1 to apply to all smooth and proper varieties over an equicharacteristic local field, not just those with semistable reduction.
Theorem 6.1. Suppose that
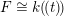 $F\cong k(\!(t)\!)$
is equicharacteristic local, and let
$F\cong k(\!(t)\!)$
is equicharacteristic local, and let
![]() $X/F$
be a smooth, proper variety. Then
$X/F$
be a smooth, proper variety. Then
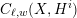 $C_{\ell ,w}(X,H^{i})$
holds.
$C_{\ell ,w}(X,H^{i})$
holds.
The proof will be via a rather delicate weight argument for Weil–Deligne representations. We will therefore let
![]() $\mathbb{Q}_{\ell }^{\prime }$
denote the field
$\mathbb{Q}_{\ell }^{\prime }$
denote the field
![]() $\mathbb{Q}_{\ell }$
if
$\mathbb{Q}_{\ell }$
if
![]() $\ell \neq p$
, and
$\ell \neq p$
, and
![]() $K^{\text{un}}$
if
$K^{\text{un}}$
if
![]() $\ell =p$
, and throughout consider
$\ell =p$
, and throughout consider
![]() $\mathbb{Q}_{\ell }^{\prime }$
-valued Weil–Deligne representations (i.e.
$\mathbb{Q}_{\ell }^{\prime }$
-valued Weil–Deligne representations (i.e.
![]() $\mathbb{Q}_{\ell }^{\prime }$
-valued representations of
$\mathbb{Q}_{\ell }^{\prime }$
-valued representations of
![]() $W_{F}^{\prime }$
). If
$W_{F}^{\prime }$
). If
 $$\begin{eqnarray}(V,N,\unicode[STIX]{x1D70C}:W_{F}\rightarrow \operatorname{GL}(V))\end{eqnarray}$$
$$\begin{eqnarray}(V,N,\unicode[STIX]{x1D70C}:W_{F}\rightarrow \operatorname{GL}(V))\end{eqnarray}$$
is such a representation then we have a canonical filtration
 $$\begin{eqnarray}M_{k}:=\mathop{\sum }_{k=i-j}\ker \;N^{i+1}\cap \operatorname{im}N^{j}\end{eqnarray}$$
$$\begin{eqnarray}M_{k}:=\mathop{\sum }_{k=i-j}\ker \;N^{i+1}\cap \operatorname{im}N^{j}\end{eqnarray}$$
on
![]() $V$
, called the monodromy filtration. This is functorial in
$V$
, called the monodromy filtration. This is functorial in
![]() $V$
in the sense that if
$V$
in the sense that if
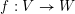 $f:V\rightarrow W$
is a morphism of Weil–Deligne representations, then
$f:V\rightarrow W$
is a morphism of Weil–Deligne representations, then
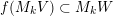 $f(M_{k}V)\subset M_{k}W$
for all
$f(M_{k}V)\subset M_{k}W$
for all
![]() $k$
. Moreover, each
$k$
. Moreover, each
![]() $M_{k}$
is preserved by the
$M_{k}$
is preserved by the
![]() $W_{F}$
-action, and hence there is an induced action of
$W_{F}$
-action, and hence there is an induced action of
![]() $W_{F}$
on
$W_{F}$
on
![]() $\operatorname{Gr}_{k}^{M}V$
.
$\operatorname{Gr}_{k}^{M}V$
.
Definition 6.2. We say that
![]() $V$
is quasi-pure of weight
$V$
is quasi-pure of weight
![]() $i$
if the eigenvalues of any lift
$i$
if the eigenvalues of any lift
 $\unicode[STIX]{x1D711}\in W_{F}$
of geometric Frobenius are
$\unicode[STIX]{x1D711}\in W_{F}$
of geometric Frobenius are
![]() $q$
-Weil numbers of weight
$q$
-Weil numbers of weight
![]() $i$
. By convention, the zero object is pure of all weights.
$i$
. By convention, the zero object is pure of all weights.
Since the inertia
![]() $I_{F}$
acts through a finite quotient, this does not depend on the choice of Frobenius lift
$I_{F}$
acts through a finite quotient, this does not depend on the choice of Frobenius lift
![]() $\unicode[STIX]{x1D711}$
. We will need the following results concerning purity and Weil–Deligne representations.
$\unicode[STIX]{x1D711}$
. We will need the following results concerning purity and Weil–Deligne representations.
Proposition 6.3.
![]() $\text{(i)}$
The subcategory
$\text{(i)}$
The subcategory
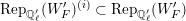 $\operatorname{Rep}_{\mathbb{Q}_{\ell }^{\prime }}(W_{F}^{\prime })^{(i)}\subset \operatorname{Rep}_{\mathbb{Q}_{\ell }^{\prime }}(W_{F}^{\prime })$
of Weil–Deligne representations which are pure of some fixed weight
$\operatorname{Rep}_{\mathbb{Q}_{\ell }^{\prime }}(W_{F}^{\prime })^{(i)}\subset \operatorname{Rep}_{\mathbb{Q}_{\ell }^{\prime }}(W_{F}^{\prime })$
of Weil–Deligne representations which are pure of some fixed weight
![]() $i$
is abelian, that is, stable under taking kernels and cokernels.
$i$
is abelian, that is, stable under taking kernels and cokernels.
![]() $\text{(ii)}$
Any morphism
$\text{(ii)}$
Any morphism
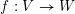 $f:V\rightarrow W$
between Weil–Deligne representations which are pure of weights
$f:V\rightarrow W$
between Weil–Deligne representations which are pure of weights
![]() $i>j$
respectively is zero.
$i>j$
respectively is zero.
![]() $\text{(iii)}$
Let
$\text{(iii)}$
Let
![]() $X/F$
be smooth and proper. Then the
$X/F$
be smooth and proper. Then the
![]() $\mathbb{Q}_{\ell }^{\prime }$
-valued Weil–Deligne representation
$\mathbb{Q}_{\ell }^{\prime }$
-valued Weil–Deligne representation
![]() $H_{\ell }^{i}(X)$
attached to the
$H_{\ell }^{i}(X)$
attached to the
![]() $\ell$
-adic cohomology of
$\ell$
-adic cohomology of
![]() $X$
is quasi-pure of weight
$X$
is quasi-pure of weight
![]() $i$
.
$i$
.
Proof. If
![]() $\ell =p$
then (i) is [Reference Lazda and PálLP16, Lemma 5.61], and (ii) is [Reference Lazda and PálLP16, Lemma 5.59]; exactly the same proof works for
$\ell =p$
then (i) is [Reference Lazda and PálLP16, Lemma 5.61], and (ii) is [Reference Lazda and PálLP16, Lemma 5.59]; exactly the same proof works for
![]() $\ell \neq p$
. If
$\ell \neq p$
. If
![]() $\ell \neq p$
then (iii) follows from [Reference ItoIto05, Theorem 1.1], and if
$\ell \neq p$
then (iii) follows from [Reference ItoIto05, Theorem 1.1], and if
![]() $\ell =p$
from [Reference Lazda and PálLP16, Theorem 5.33].◻
$\ell =p$
from [Reference Lazda and PálLP16, Theorem 5.33].◻
We will also need the following result.
Lemma 6.4. Let
![]() $F^{\prime }/F$
be a finite extension (not necessarily Galois or even separable), and let
$F^{\prime }/F$
be a finite extension (not necessarily Galois or even separable), and let
![]() $X^{\prime }/F^{\prime }$
be smooth and proper. Let
$X^{\prime }/F^{\prime }$
be smooth and proper. Let
![]() $X$
denote
$X$
denote
![]() $X^{\prime }$
considered as a variety over
$X^{\prime }$
considered as a variety over
![]() $F$
, and fix a prime
$F$
, and fix a prime
![]() $\ell$
.
$\ell$
.
-
(i) If the
 $\ell$
-adic cohomology
$\ell$
-adic cohomology
 $H_{\ell }^{i}(X^{\prime })$
is quasi-pure of weight
$H_{\ell }^{i}(X^{\prime })$
is quasi-pure of weight
 $i$
as a
$i$
as a
 $W_{F^{\prime }}^{\prime }$
-representation, then
$W_{F^{\prime }}^{\prime }$
-representation, then
 $H_{\ell }^{i}(X)$
is quasi-pure of weight
$H_{\ell }^{i}(X)$
is quasi-pure of weight
 $i$
as a
$i$
as a
 $W_{F}^{\prime }$
-representation.
$W_{F}^{\prime }$
-representation. -
(ii) If
 $C_{\ell ,w}(H^{i})$
holds for
$C_{\ell ,w}(H^{i})$
holds for
 $X^{\prime }/F^{\prime }$
, then
$X^{\prime }/F^{\prime }$
, then
 $C_{\ell ,w}(H^{i})$
holds for
$C_{\ell ,w}(H^{i})$
holds for
 $X/F$
.
$X/F$
.
Proof. It suffices to treat the cases where
![]() $F^{\prime }/F$
is separable, and where
$F^{\prime }/F$
is separable, and where
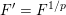 $F^{\prime }=F^{1/p}$
. If
$F^{\prime }=F^{1/p}$
. If
![]() $F^{\prime }/F$
is separable, then we may view
$F^{\prime }/F$
is separable, then we may view
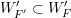 $W_{F^{\prime }}^{\prime }\subset W_{F}^{\prime }$
as a finite-index subgroup. Then we have that
$W_{F^{\prime }}^{\prime }\subset W_{F}^{\prime }$
as a finite-index subgroup. Then we have that
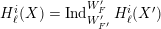 $H_{\ell }^{i}(X)=\operatorname{Ind}_{W_{F^{\prime }}^{\prime }}^{W_{F}^{\prime }}H_{\ell }^{i}(X^{\prime })$
is simply the induced representation, which easily implies both (i) and (ii).
$H_{\ell }^{i}(X)=\operatorname{Ind}_{W_{F^{\prime }}^{\prime }}^{W_{F}^{\prime }}H_{\ell }^{i}(X^{\prime })$
is simply the induced representation, which easily implies both (i) and (ii).
Next suppose that
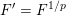 $F^{\prime }=F^{1/p}$
, or, in other words, that we are looking at the finite extension
$F^{\prime }=F^{1/p}$
, or, in other words, that we are looking at the finite extension
 $\operatorname{Frob}:F\rightarrow F$
. Let
$\operatorname{Frob}:F\rightarrow F$
. Let
![]() $X_{1}$
be a given smooth and proper variety over
$X_{1}$
be a given smooth and proper variety over
![]() $F$
, and
$F$
, and
![]() $X_{0}$
the
$X_{0}$
the
![]() $F$
-variety by considering
$F$
-variety by considering
![]() $X_{1}$
with the composite structure morphism
$X_{1}$
with the composite structure morphism
 $X_{1}\rightarrow \operatorname{Spec}(F)\overset{\operatorname{Spec}(\operatorname{Frob})}{\rightarrow }\operatorname{Spec}(F)$
. Since the map
$X_{1}\rightarrow \operatorname{Spec}(F)\overset{\operatorname{Spec}(\operatorname{Frob})}{\rightarrow }\operatorname{Spec}(F)$
. Since the map
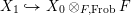 $X_{1}{\hookrightarrow}X_{0}\otimes _{F,\text{Frob}}F$
is a nilpotent thickening, we have
$X_{1}{\hookrightarrow}X_{0}\otimes _{F,\text{Frob}}F$
is a nilpotent thickening, we have
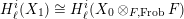 $H_{\ell }^{i}(X_{1})\cong H_{\ell }^{i}(X_{0}\otimes _{F,\text{Frob}}F)$
as
$H_{\ell }^{i}(X_{1})\cong H_{\ell }^{i}(X_{0}\otimes _{F,\text{Frob}}F)$
as
![]() $W_{F}^{\prime }$
-representations, and since the Frobenius map
$W_{F}^{\prime }$
-representations, and since the Frobenius map
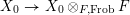 $X_{0}\rightarrow X_{0}\otimes _{F,\text{Frob}}F$
is a universal homeomorphism, it follows that
$X_{0}\rightarrow X_{0}\otimes _{F,\text{Frob}}F$
is a universal homeomorphism, it follows that
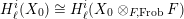 $H_{\ell }^{i}(X_{0})\cong H_{\ell }^{i}(X_{0}\otimes _{F,\text{Frob}}F)$
as
$H_{\ell }^{i}(X_{0})\cong H_{\ell }^{i}(X_{0}\otimes _{F,\text{Frob}}F)$
as
![]() $W_{F}^{\prime }$
-representations. Hence we can deduce that
$W_{F}^{\prime }$
-representations. Hence we can deduce that
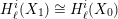 $H_{\ell }^{i}(X_{1})\cong H_{\ell }^{i}(X_{0})$
as
$H_{\ell }^{i}(X_{1})\cong H_{\ell }^{i}(X_{0})$
as
![]() $W_{F}^{\prime }$
-representations, which allows us to conclude.◻
$W_{F}^{\prime }$
-representations, which allows us to conclude.◻
With these preliminaries in place, we can now prove Theorem 6.1.
Proof of Theorem 6.1.
Let
![]() $X/F$
be smooth and proper. Then by de Jong’s theorem on alterations [Reference de JongdeJ96, Theorem 8.2], we may choose a proper hypercover
$X/F$
be smooth and proper. Then by de Jong’s theorem on alterations [Reference de JongdeJ96, Theorem 8.2], we may choose a proper hypercover
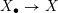 $X_{\bullet }\rightarrow X$
and finite extensions
$X_{\bullet }\rightarrow X$
and finite extensions
![]() $F_{n}/F$
such that:
$F_{n}/F$
such that:
-
– the structure morphism
 $X_{n}\rightarrow \operatorname{Spec}(F)$
factors through
$X_{n}\rightarrow \operatorname{Spec}(F)$
factors through
 $\operatorname{Spec}(F_{n})$
;
$\operatorname{Spec}(F_{n})$
; -
–
 $X_{n}/F_{n}$
has strictly semistable reduction.
$X_{n}/F_{n}$
has strictly semistable reduction.
In particular, by applying Theorem 5.1, Proposition 6.3(iii) and Lemma 6.4, we can see that the cohomology groups
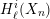 $H_{\ell }^{i}(X_{n})$
, where
$H_{\ell }^{i}(X_{n})$
, where
![]() $X_{n}$
is viewed as an
$X_{n}$
is viewed as an
![]() $F$
-variety, are quasi-pure of weight
$F$
-variety, are quasi-pure of weight
![]() $i$
, and moreover that they satisfy weak
$i$
, and moreover that they satisfy weak
![]() $\ell$
-independence. We now consider the spectral sequence
$\ell$
-independence. We now consider the spectral sequence
 $$\begin{eqnarray}E_{1}^{n,i}=H_{\ell }^{i}(X_{n})\Rightarrow H_{\ell }^{i+n}(X).\end{eqnarray}$$
$$\begin{eqnarray}E_{1}^{n,i}=H_{\ell }^{i}(X_{n})\Rightarrow H_{\ell }^{i+n}(X).\end{eqnarray}$$
Since
![]() $E_{1}^{n,i}$
is quasi-pure of weight
$E_{1}^{n,i}$
is quasi-pure of weight
![]() $i$
, it follows from Proposition 6.3(i) that
$i$
, it follows from Proposition 6.3(i) that
![]() $E_{2}^{i,n}$
is also quasi-pure of weight
$E_{2}^{i,n}$
is also quasi-pure of weight
![]() $n$
, and hence from Proposition 6.3(ii) that the spectral sequence degenerates at
$n$
, and hence from Proposition 6.3(ii) that the spectral sequence degenerates at
![]() $E_{2}$
.
$E_{2}$
.
We will now consider the induced filtration
![]() $P_{\bullet }$
on
$P_{\bullet }$
on
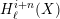 $H_{\ell }^{i+n}(X)$
, whose
$H_{\ell }^{i+n}(X)$
, whose
![]() $i$
th grade piece
$i$
th grade piece
 $$\begin{eqnarray}\operatorname{Gr}_{i}^{P}H_{\ell }^{i+n}(X)=E_{2}^{n,i}\end{eqnarray}$$
$$\begin{eqnarray}\operatorname{Gr}_{i}^{P}H_{\ell }^{i+n}(X)=E_{2}^{n,i}\end{eqnarray}$$
is therefore quasi-pure of weight
![]() $i$
. If we look at the quotient map
$i$
. If we look at the quotient map
 $$\begin{eqnarray}H_{\ell }^{i+n}(X)\rightarrow \operatorname{Gr}_{i+n}^{P}=E_{2}^{0,i+n}\end{eqnarray}$$
$$\begin{eqnarray}H_{\ell }^{i+n}(X)\rightarrow \operatorname{Gr}_{i+n}^{P}=E_{2}^{0,i+n}\end{eqnarray}$$
then it follows from Proposition 6.3(i) that the kernel
 $P_{i+n-1}H_{\ell }^{i+n}(X)$
is quasi-pure of weight
$P_{i+n-1}H_{\ell }^{i+n}(X)$
is quasi-pure of weight
![]() $i+n$
, and hence that the quotient map
$i+n$
, and hence that the quotient map
 $$\begin{eqnarray}P_{i+n-1}H_{\ell }^{i+n}(X){\twoheadrightarrow}\operatorname{Gr}_{i+n-1}^{P}=E_{2}^{1,i+n-1}\end{eqnarray}$$
$$\begin{eqnarray}P_{i+n-1}H_{\ell }^{i+n}(X){\twoheadrightarrow}\operatorname{Gr}_{i+n-1}^{P}=E_{2}^{1,i+n-1}\end{eqnarray}$$
must be zero by Proposition 6.3(ii). In particular, we have
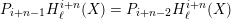 $P_{i+n-1}H_{\ell }^{i+n}(X)=P_{i+n-2}H_{\ell }^{i+n}(X)$
, and, again applying Proposition 6.3(ii), we can see that the quotient
$P_{i+n-1}H_{\ell }^{i+n}(X)=P_{i+n-2}H_{\ell }^{i+n}(X)$
, and, again applying Proposition 6.3(ii), we can see that the quotient
 $$\begin{eqnarray}P_{i+n-2}H_{\ell }^{i+n}(X){\twoheadrightarrow}\operatorname{Gr}_{i+n-2}^{P}=E_{2}^{2,i+n-2}\end{eqnarray}$$
$$\begin{eqnarray}P_{i+n-2}H_{\ell }^{i+n}(X){\twoheadrightarrow}\operatorname{Gr}_{i+n-2}^{P}=E_{2}^{2,i+n-2}\end{eqnarray}$$
must be zero. Continuing thus, we find that
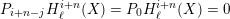 $P_{i+n-j}H_{\ell }^{i+n}(X)=P_{0}H_{\ell }^{i+n}(X)=0$
for all
$P_{i+n-j}H_{\ell }^{i+n}(X)=P_{0}H_{\ell }^{i+n}(X)=0$
for all
![]() $j>0$
, from which we deduce that the
$j>0$
, from which we deduce that the
![]() $E_{2}$
-page of the spectral sequence only has non-zero terms in the first column. Put differently, we have a long exact sequence
$E_{2}$
-page of the spectral sequence only has non-zero terms in the first column. Put differently, we have a long exact sequence
 $$\begin{eqnarray}0\rightarrow H_{\ell }^{i+n}(X)\rightarrow H_{\ell }^{i+n}(X_{0})\rightarrow H_{\ell }^{i+n}(X_{1})\rightarrow H_{\ell }^{i+n}(X_{2})\rightarrow \cdots\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow H_{\ell }^{i+n}(X)\rightarrow H_{\ell }^{i+n}(X_{0})\rightarrow H_{\ell }^{i+n}(X_{1})\rightarrow H_{\ell }^{i+n}(X_{2})\rightarrow \cdots\end{eqnarray}$$
for all
![]() $i,n,\ell$
. Since we know weak
$i,n,\ell$
. Since we know weak
![]() $\ell$
-independence for all the terms
$\ell$
-independence for all the terms
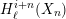 $H_{\ell }^{i+n}(X_{n})$
, we can therefore deduce weak
$H_{\ell }^{i+n}(X_{n})$
, we can therefore deduce weak
![]() $\ell$
-independence for
$\ell$
-independence for
 $H_{\ell }^{i+n}(X)$
.◻
$H_{\ell }^{i+n}(X)$
.◻
7
 $L$
-functions and the proof of Proposition 5.8
$L$
-functions and the proof of Proposition 5.8
The purpose of this section is to prove Proposition 5.8, as well as to give an application of Theorem 6.1 showing that the Hasse–Weil
![]() $L$
-functions of smooth and proper varieties over global function fields (including those defined using
$L$
-functions of smooth and proper varieties over global function fields (including those defined using
![]() $p$
-adic cohomology) are independent of
$p$
-adic cohomology) are independent of
![]() $\ell$
. The basic idea is that away from
$\ell$
. The basic idea is that away from
![]() $\ell =p$
Proposition 5.8 follows from [Reference DeligneDel73, Théorème 9.8], and in fact Deligne’s proof also works at
$\ell =p$
Proposition 5.8 follows from [Reference DeligneDel73, Théorème 9.8], and in fact Deligne’s proof also works at
![]() $\ell =p$
once certain facts about
$\ell =p$
once certain facts about
![]() $p$
-adic cohomology of smooth curves are in place. The deduction of
$p$
-adic cohomology of smooth curves are in place. The deduction of
![]() $\ell$
-independence for the Hasse–Weil
$\ell$
-independence for the Hasse–Weil
![]() $L$
-function is then entirely straightforward. In fact, it is not entirely accurate to describe this as an application of Theorem 6.1, since it is in fact a version of
$L$
-function is then entirely straightforward. In fact, it is not entirely accurate to describe this as an application of Theorem 6.1, since it is in fact a version of
![]() $\ell$
-independence for local
$\ell$
-independence for local
![]() $L$
-functions that forms a key component of the proof of Proposition 5.8, and therefore of Theorem 6.1. However, we thought it worth isolating this special case. All the results presented in this section are more or less special cases of more general results of Abe and Caro proved in [Reference Abe and CaroAC14]. However, in the simple case that we are interested in one can give proofs using simpler machinery from the theory of arithmetic
$L$
-functions that forms a key component of the proof of Proposition 5.8, and therefore of Theorem 6.1. However, we thought it worth isolating this special case. All the results presented in this section are more or less special cases of more general results of Abe and Caro proved in [Reference Abe and CaroAC14]. However, in the simple case that we are interested in one can give proofs using simpler machinery from the theory of arithmetic
![]() ${\mathcal{D}}$
-modules on curves and formal curves, in particular that from [Reference CaroCar06] and [Reference CrewCre12].
${\mathcal{D}}$
-modules on curves and formal curves, in particular that from [Reference CaroCar06] and [Reference CrewCre12].
Since the situation we are interested in in this section is somewhat different from that in the rest of the article, we will change notation slightly (only for this section; we will return to the usual notation in the next section). So we will let
![]() $F$
be a global function field with field of constants
$F$
be a global function field with field of constants
![]() $k$
, and let
$k$
, and let
![]() $X$
be a smooth, projective model for
$X$
be a smooth, projective model for
![]() $F$
. We will let
$F$
. We will let
![]() $U$
be an open subset of
$U$
be an open subset of
![]() $X$
, and let
$X$
, and let
 $S=X\setminus U$
. For any place
$S=X\setminus U$
. For any place
![]() $v\in |X|$
(with some fixed local parameter
$v\in |X|$
(with some fixed local parameter
![]() $t_{v}$
) we will let
$t_{v}$
) we will let
![]() $k_{v}$
denote the residue field at
$k_{v}$
denote the residue field at
![]() $v$
,
$v$
,
![]() $K_{v}$
the unique unramified extension of
$K_{v}$
the unique unramified extension of
![]() $K$
with residue field
$K$
with residue field
![]() $k_{v}$
,
$k_{v}$
,
![]() $W_{v}$
its ring of integers and
$W_{v}$
its ring of integers and
![]() ${\mathcal{R}}_{v}$
a copy of the Robba ring at
${\mathcal{R}}_{v}$
a copy of the Robba ring at
![]() $v$
, considered as a subring of
$v$
, considered as a subring of
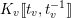 $K_{v}\unicode[STIX]{x27E6}t_{v},t_{v}^{-1}\unicode[STIX]{x27E7}$
. Let
$K_{v}\unicode[STIX]{x27E6}t_{v},t_{v}^{-1}\unicode[STIX]{x27E7}$
. Let
![]() $\unicode[STIX]{x1D711}_{v}$
denote a
$\unicode[STIX]{x1D711}_{v}$
denote a
![]() $\#k_{v}$
-power Frobenius on
$\#k_{v}$
-power Frobenius on
![]() ${\mathcal{R}}_{v}$
. We will also denote
${\mathcal{R}}_{v}$
. We will also denote
![]() $\#k_{v}$
-power Frobenius operators by
$\#k_{v}$
-power Frobenius operators by
![]() $F_{v}$
.
$F_{v}$
.
If we are given an overconvergent
![]() $F$
-isocrystal
$F$
-isocrystal
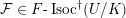 ${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
and a point
${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
and a point
![]() $v\in X$
we can consider Tsuzuki’s functor
$v\in X$
we can consider Tsuzuki’s functor
 $$\begin{eqnarray}\mathbf{i}_{v}^{\ast }:F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{v}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
$$\begin{eqnarray}\mathbf{i}_{v}^{\ast }:F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{v}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
to the category of
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{R}}_{v}$
, and therefore define the local
${\mathcal{R}}_{v}$
, and therefore define the local
![]() $L$
-factor of
$L$
-factor of
![]() ${\mathcal{F}}$
at
${\mathcal{F}}$
at
![]() $v$
by
$v$
by
 $$\begin{eqnarray}L_{v}({\mathcal{F}},t):=\det (1-t^{\deg v}\unicode[STIX]{x1D711}_{v}\mid (\mathbf{i}_{v}^{\ast }{\mathcal{F}})^{\unicode[STIX]{x1D6FB}=0}).\end{eqnarray}$$
$$\begin{eqnarray}L_{v}({\mathcal{F}},t):=\det (1-t^{\deg v}\unicode[STIX]{x1D711}_{v}\mid (\mathbf{i}_{v}^{\ast }{\mathcal{F}})^{\unicode[STIX]{x1D6FB}=0}).\end{eqnarray}$$
The global
![]() $L$
-function of
$L$
-function of
![]() ${\mathcal{F}}$
is then defined to be
${\mathcal{F}}$
is then defined to be
 $$\begin{eqnarray}\widehat{L}({\mathcal{F}},t):=\mathop{\prod }_{v\in |X|}L_{v}({\mathcal{F}},t);\end{eqnarray}$$
$$\begin{eqnarray}\widehat{L}({\mathcal{F}},t):=\mathop{\prod }_{v\in |X|}L_{v}({\mathcal{F}},t);\end{eqnarray}$$
the notation
![]() $\widehat{L}$
used here is in order to distinguish it from the Etesse–Le Stum
$\widehat{L}$
used here is in order to distinguish it from the Etesse–Le Stum
![]() $L$
-function
$L$
-function
![]() $L_{\text{EL}}$
from [Reference Etesse and Le StumEL93], which is defined by only considering local
$L_{\text{EL}}$
from [Reference Etesse and Le StumEL93], which is defined by only considering local
![]() $L$
-factors at points of
$L$
-factors at points of
![]() $U$
. Note that at places
$U$
. Note that at places
![]() $v\in U$
the local
$v\in U$
the local
![]() $L$
-factor can also be described (by Dwork’s trick) as the inverse characteristic polynomial
$L$
-factor can also be described (by Dwork’s trick) as the inverse characteristic polynomial
 $\det (1-t^{\deg v}F^{\deg v}\mid {\mathcal{F}}_{v})$
of the linearised Frobenius of
$\det (1-t^{\deg v}F^{\deg v}\mid {\mathcal{F}}_{v})$
of the linearised Frobenius of
![]() ${\mathcal{F}}$
acting on the stalk
${\mathcal{F}}$
acting on the stalk
![]() ${\mathcal{F}}_{v}$
of
${\mathcal{F}}_{v}$
of
![]() ${\mathcal{F}}$
at
${\mathcal{F}}$
at
![]() $v$
. Our first task will be to prove a function equation for
$v$
. Our first task will be to prove a function equation for
![]() $\widehat{L}({\mathcal{F}},t)$
, and to do so we will need to interpret the local factors
$\widehat{L}({\mathcal{F}},t)$
, and to do so we will need to interpret the local factors
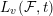 $L_{v}({\mathcal{F}},t)$
in terms of arithmetic
$L_{v}({\mathcal{F}},t)$
in terms of arithmetic
![]() ${\mathcal{D}}$
-modules on
${\mathcal{D}}$
-modules on
![]() $X$
.
$X$
.
So we will denote by
![]() ${\mathcal{X}}$
a lift of
${\mathcal{X}}$
a lift of
![]() $X$
to a smooth projective formal curve over
$X$
to a smooth projective formal curve over
![]() $W$
, by
$W$
, by
![]() ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
Berthelot’s ring of arithmetic differential operators on
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
Berthelot’s ring of arithmetic differential operators on
![]() ${\mathcal{X}}$
, and by
${\mathcal{X}}$
, and by
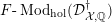 $F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
(respectively,
$F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
(respectively,
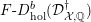 $F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
) the category of holonomic
$F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
) the category of holonomic
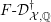 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules (respectively, the bounded derived category of
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules (respectively, the bounded derived category of
![]() ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules equipped with Frobenius structure, whose cohomology sheaves are holonomic). For any complex
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules equipped with Frobenius structure, whose cohomology sheaves are holonomic). For any complex
 ${\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
we will denote by
${\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
we will denote by
![]() $L({\mathcal{K}},t)$
the
$L({\mathcal{K}},t)$
the
![]() $L$
-function of
$L$
-function of
![]() ${\mathcal{K}}$
as defined in the introduction to [Reference CaroCar06]; let us quickly recall his definition. For
${\mathcal{K}}$
as defined in the introduction to [Reference CaroCar06]; let us quickly recall his definition. For
![]() $v\in |X|$
we may lift
$v\in |X|$
we may lift
![]() $v$
to a morphism
$v$
to a morphism
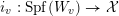 $i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
, and for any
$i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
, and for any
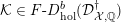 ${\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
we may consider the pullback
${\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
we may consider the pullback
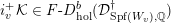 $i_{v}^{+}{\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{\operatorname{Spf}(W_{v}),\mathbb{Q}}^{\dagger })$
. Its cohomology sheaves are therefore
$i_{v}^{+}{\mathcal{K}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{\operatorname{Spf}(W_{v}),\mathbb{Q}}^{\dagger })$
. Its cohomology sheaves are therefore
![]() $F$
-isocrystals over
$F$
-isocrystals over
![]() $K_{v}$
; we may therefore consider
$K_{v}$
; we may therefore consider
![]() $F^{\deg v}$
as a
$F^{\deg v}$
as a
![]() $K$
-linear operator on these cohomology sheaves. Caro then defines
$K$
-linear operator on these cohomology sheaves. Caro then defines
 $$\begin{eqnarray}\displaystyle L_{v}({\mathcal{K}},t) & := & \displaystyle \mathop{\prod }_{i\in \mathbb{Z}}\textstyle \det _{K}(1-t^{\deg v}F^{\deg v}|H^{i}(i_{v}^{+}{\mathcal{K}}))^{(-1)^{r}/(\deg v)}\nonumber\\ \displaystyle L({\mathcal{K}},t) & := & \displaystyle \mathop{\prod }_{v\in |X|}L_{v}({\mathcal{K}},t).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle L_{v}({\mathcal{K}},t) & := & \displaystyle \mathop{\prod }_{i\in \mathbb{Z}}\textstyle \det _{K}(1-t^{\deg v}F^{\deg v}|H^{i}(i_{v}^{+}{\mathcal{K}}))^{(-1)^{r}/(\deg v)}\nonumber\\ \displaystyle L({\mathcal{K}},t) & := & \displaystyle \mathop{\prod }_{v\in |X|}L_{v}({\mathcal{K}},t).\nonumber\end{eqnarray}$$
The functional equation that we require will follow from the existence of the ‘intermediate extension’ functor
 $$\begin{eqnarray}j_{!+}:F\text{-}\operatorname{Isoc}(U/K)\rightarrow F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })\end{eqnarray}$$
$$\begin{eqnarray}j_{!+}:F\text{-}\operatorname{Isoc}(U/K)\rightarrow F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })\end{eqnarray}$$
which will be such that:
-
(i)
 $\widehat{L}({\mathcal{F}},t)=L(j_{!+}{\mathcal{F}},t)$
;
$\widehat{L}({\mathcal{F}},t)=L(j_{!+}{\mathcal{F}},t)$
; -
(ii)
 $\mathbf{D}(j_{!+}{\mathcal{F}})\cong j_{!+}({\mathcal{F}}^{\vee })$
, where
$\mathbf{D}(j_{!+}{\mathcal{F}})\cong j_{!+}({\mathcal{F}}^{\vee })$
, where
 $\mathbf{D}$
is the dual functor for
$\mathbf{D}$
is the dual functor for
 ${\mathcal{D}}$
-modules, and
${\mathcal{D}}$
-modules, and
 $(-)^{\vee }$
that for isocrystals.
$(-)^{\vee }$
that for isocrystals.
As noted at the beginning of this section, this is a special case of much more general results of [Reference Abe and CaroAC14], but we thought it worthwhile to go through the construction in detail in the simple case that we are interested in. To construct
![]() $j_{!+}$
, first let us consider Berthelot’s ring
$j_{!+}$
, first let us consider Berthelot’s ring
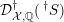 ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
of arithmetic differential operators on
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
of arithmetic differential operators on
![]() ${\mathcal{X}}$
with overconvergent singularities along
${\mathcal{X}}$
with overconvergent singularities along
![]() $S$
, and the corresponding categories
$S$
, and the corresponding categories
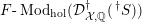 $F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
and
$F\text{-}\operatorname{Mod}_{\text{hol}}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
and
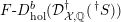 $F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
of (complexes of) holonomic
$F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
of (complexes of) holonomic
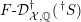 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-modules. Then, thanks to [Reference CaroCar06, Théorème 2.3.3], we may consider any
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-modules. Then, thanks to [Reference CaroCar06, Théorème 2.3.3], we may consider any
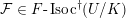 ${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
as a holonomic
${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
as a holonomic
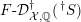 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module, which we shall do. We have canonical functors
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module, which we shall do. We have canonical functors
 $$\begin{eqnarray}\displaystyle j_{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }),\nonumber\\ \displaystyle j^{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle j_{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }),\nonumber\\ \displaystyle j^{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))\nonumber\end{eqnarray}$$
given by restriction and extension of scalars along
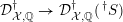 ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
respectively; these are both exact for the natural
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
respectively; these are both exact for the natural
![]() $t$
-structures on both sides. Define
$t$
-structures on both sides. Define
![]() $j_{!}$
to be
$j_{!}$
to be
![]() $\mathbf{D}j_{+}\mathbf{D}$
where
$\mathbf{D}j_{+}\mathbf{D}$
where
![]() $\mathbf{D}$
is the duality functor; note that this is exact for holonomic
$\mathbf{D}$
is the duality functor; note that this is exact for holonomic
![]() $F\text{-}{\mathcal{D}}$
-modules by (the proof of) [Reference VirrionVir00, Proposition III.4.4]. By [Reference VirrionVir00, Proposition I.4.4] we have
$F\text{-}{\mathcal{D}}$
-modules by (the proof of) [Reference VirrionVir00, Proposition III.4.4]. By [Reference VirrionVir00, Proposition I.4.4] we have
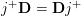 $j^{+}\mathbf{D}=\mathbf{D}j^{+}$
and hence we get adjoint pairs
$j^{+}\mathbf{D}=\mathbf{D}j^{+}$
and hence we get adjoint pairs
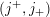 $(j^{+},j_{+})$
and
$(j^{+},j_{+})$
and
![]() $(j_{!},j^{+})$
. Also by [Reference CaroCar06, 1.1.8] we have
$(j_{!},j^{+})$
. Also by [Reference CaroCar06, 1.1.8] we have
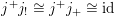 $j^{+}j_{!}\cong j^{+}j_{+}\cong \operatorname{id}$
.
$j^{+}j_{!}\cong j^{+}j_{+}\cong \operatorname{id}$
.
The closed immersion
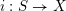 $i:S\rightarrow X$
lifts to a closed immersion
$i:S\rightarrow X$
lifts to a closed immersion
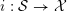 $i:{\mathcal{S}}\rightarrow {\mathcal{X}}$
and hence we obtain Berthelot’s pullback and pushforward functors
$i:{\mathcal{S}}\rightarrow {\mathcal{X}}$
and hence we obtain Berthelot’s pullback and pushforward functors
 $$\begin{eqnarray}\displaystyle i^{!}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{S}},\mathbb{Q}}^{\dagger }),\nonumber\\ \displaystyle i_{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{S}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }),\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle i^{!}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{S}},\mathbb{Q}}^{\dagger }),\nonumber\\ \displaystyle i_{+}:F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{S}},\mathbb{Q}}^{\dagger }) & \rightarrow & \displaystyle F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }),\nonumber\end{eqnarray}$$
and we define
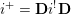 $i^{+}=\mathbf{D}i^{!}\mathbf{D}$
. It follows from [Reference BerthelotBer02, Théorème 4.3.13] that
$i^{+}=\mathbf{D}i^{!}\mathbf{D}$
. It follows from [Reference BerthelotBer02, Théorème 4.3.13] that
 $i_{+}\mathbf{D}=\mathbf{D}i_{+}$
and hence by [Reference CaroCar06, Théorème 1.2.21] we have two adjoint pairs
$i_{+}\mathbf{D}=\mathbf{D}i_{+}$
and hence by [Reference CaroCar06, Théorème 1.2.21] we have two adjoint pairs
![]() $(i^{+},i_{+})$
and
$(i^{+},i_{+})$
and
![]() $(i_{+},i^{!})$
. Also by [Reference BerthelotBer02, Théorème 5.3.3] we get
$(i_{+},i^{!})$
. Also by [Reference BerthelotBer02, Théorème 5.3.3] we get
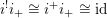 $i^{!}i_{+}\cong i^{+}i_{+}\cong \operatorname{id}$
. By [Reference CaroCar06, (1.1.6.5)] we have, for any
$i^{!}i_{+}\cong i^{+}i_{+}\cong \operatorname{id}$
. By [Reference CaroCar06, (1.1.6.5)] we have, for any
 ${\mathcal{E}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
, exact triangles
${\mathcal{E}}\in F\text{-}D_{\text{hol}}^{b}({\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
, exact triangles
 $$\begin{eqnarray}\displaystyle j_{!}j^{+}{\mathcal{E}} & \rightarrow & \displaystyle {\mathcal{E}}\rightarrow i_{+}i^{+}{\mathcal{E}}\overset{+1}{\rightarrow },\nonumber\\ \displaystyle i_{+}i^{!}{\mathcal{E}} & \rightarrow & \displaystyle {\mathcal{E}}\rightarrow j_{+}j^{+}{\mathcal{E}}\overset{+1}{\rightarrow }.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle j_{!}j^{+}{\mathcal{E}} & \rightarrow & \displaystyle {\mathcal{E}}\rightarrow i_{+}i^{+}{\mathcal{E}}\overset{+1}{\rightarrow },\nonumber\\ \displaystyle i_{+}i^{!}{\mathcal{E}} & \rightarrow & \displaystyle {\mathcal{E}}\rightarrow j_{+}j^{+}{\mathcal{E}}\overset{+1}{\rightarrow }.\nonumber\end{eqnarray}$$
In particular, taking
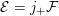 ${\mathcal{E}}=j_{+}{\mathcal{F}}$
, we get an exact triangle
${\mathcal{E}}=j_{+}{\mathcal{F}}$
, we get an exact triangle
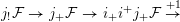 $j_{!}{\mathcal{F}}\rightarrow j_{+}{\mathcal{F}}\rightarrow i_{+}i^{+}j_{+}{\mathcal{F}}\overset{+1}{\rightarrow }$
, and we define
$j_{!}{\mathcal{F}}\rightarrow j_{+}{\mathcal{F}}\rightarrow i_{+}i^{+}j_{+}{\mathcal{F}}\overset{+1}{\rightarrow }$
, and we define
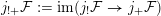 $j_{!+}{\mathcal{F}}:=\operatorname{im}(j_{!}{\mathcal{F}}\rightarrow j_{+}{\mathcal{F}})$
. Note that this makes sense as a
$j_{!+}{\mathcal{F}}:=\operatorname{im}(j_{!}{\mathcal{F}}\rightarrow j_{+}{\mathcal{F}})$
. Note that this makes sense as a
![]() ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-module because
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-module because
![]() $j_{+}$
and
$j_{+}$
and
![]() $j_{!}$
are exact. By exactness of
$j_{!}$
are exact. By exactness of
![]() $\mathbf{D}$
for holonomic
$\mathbf{D}$
for holonomic
![]() $F\text{-}{\mathcal{D}}^{\dagger }$
-modules we have
$F\text{-}{\mathcal{D}}^{\dagger }$
-modules we have
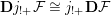 $\mathbf{D}j_{!+}{\mathcal{F}}\cong j_{!+}\mathbf{D}{\mathcal{F}}$
, and hence, using the main result of [Reference CaroCar05], we deduce that
$\mathbf{D}j_{!+}{\mathcal{F}}\cong j_{!+}\mathbf{D}{\mathcal{F}}$
, and hence, using the main result of [Reference CaroCar05], we deduce that
 $\mathbf{D}(j_{!+}{\mathcal{F}})\cong j_{!+}({\mathcal{F}}^{\vee })$
. To compare the
$\mathbf{D}(j_{!+}{\mathcal{F}})\cong j_{!+}({\mathcal{F}}^{\vee })$
. To compare the
![]() $L$
-functions of
$L$
-functions of
![]() ${\mathcal{F}}$
and
${\mathcal{F}}$
and
![]() $j_{!+}{\mathcal{F}}$
essentially boils down to the following local calculation from [Reference CrewCre12].
$j_{!+}{\mathcal{F}}$
essentially boils down to the following local calculation from [Reference CrewCre12].
Proposition 7.1. Let
![]() $v\in X$
, and let
$v\in X$
, and let
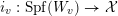 $i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
be a lifting of the inclusion
$i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
be a lifting of the inclusion
![]() $v\rightarrow X$
.
$v\rightarrow X$
.
-
(i) If
 $v\in U$
then
$v\in U$
then
 $(i_{v}^{+}j_{!+}{\mathcal{F}})[-1]\cong {\mathcal{F}}_{v}$
as
$(i_{v}^{+}j_{!+}{\mathcal{F}})[-1]\cong {\mathcal{F}}_{v}$
as
 $F_{v}$
-isocrystals over
$F_{v}$
-isocrystals over
 $K_{v}$
.
$K_{v}$
. -
(ii) If
 $v\in S$
then
$v\in S$
then
 $(i_{v}^{+}j_{!+}{\mathcal{F}})[-1]\cong (\mathbf{i}_{v}^{\ast }{\mathcal{F}})^{\unicode[STIX]{x1D6FB}=0}$
as
$(i_{v}^{+}j_{!+}{\mathcal{F}})[-1]\cong (\mathbf{i}_{v}^{\ast }{\mathcal{F}})^{\unicode[STIX]{x1D6FB}=0}$
as
 $F_{v}$
-isocrystals over
$F_{v}$
-isocrystals over
 $K_{v}$
.
$K_{v}$
.
Remark 7.2. (i) In both cases,
![]() $i_{v}^{+}j_{!+}{\mathcal{F}}$
is naturally an
$i_{v}^{+}j_{!+}{\mathcal{F}}$
is naturally an
![]() $F$
-isocrystal; taking a suitable power of the Frobenius allows us to regard it as a
$F$
-isocrystal; taking a suitable power of the Frobenius allows us to regard it as a
![]() $F_{v}$
-isocrystal. In the second case,
$F_{v}$
-isocrystal. In the second case,
![]() $\mathbf{i}_{v}^{\ast }{\mathcal{F}}$
is the
$\mathbf{i}_{v}^{\ast }{\mathcal{F}}$
is the
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module over
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module over
![]() ${\mathcal{R}}_{v}$
associated to
${\mathcal{R}}_{v}$
associated to
![]() ${\mathcal{F}}$
as above. Note also that implicit in both claims is that
${\mathcal{F}}$
as above. Note also that implicit in both claims is that
![]() $i_{v}^{+}j_{!+}{\mathcal{F}}$
is concentrated in degree
$i_{v}^{+}j_{!+}{\mathcal{F}}$
is concentrated in degree
![]() $-1$
.
$-1$
.
(iii) The proposition implies that the local
![]() $L$
-factors of
$L$
-factors of
![]() ${\mathcal{F}}$
and
${\mathcal{F}}$
and
![]() $j_{!+}{\mathcal{F}}$
agree at all places of
$j_{!+}{\mathcal{F}}$
agree at all places of
![]() $X$
, hence that
$X$
, hence that
 $\widehat{L}({\mathcal{F}},t)=L(j_{!+}{\mathcal{F}},t)$
.
$\widehat{L}({\mathcal{F}},t)=L(j_{!+}{\mathcal{F}},t)$
.
(iii) By Dwork’s trick, the formula in the second case is in fact also valid in the first.
Proof. The first case is noted in the proof of [Reference CaroCar06, Proposition 3.3.1] (in combination with the main result from [Reference CaroCar05]); we will consider the second. The key point is to show that we may calculate
![]() $j_{!}$
and
$j_{!}$
and
![]() $j_{+}$
entirely locally. So let
$j_{+}$
entirely locally. So let
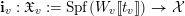 $\mathbf{i}_{v}:\mathfrak{X}_{v}:=\operatorname{Spf}(W_{v}\unicode[STIX]{x27E6}t_{v}\unicode[STIX]{x27E7})\rightarrow {\mathcal{X}}$
be a lifting of the map
$\mathbf{i}_{v}:\mathfrak{X}_{v}:=\operatorname{Spf}(W_{v}\unicode[STIX]{x27E6}t_{v}\unicode[STIX]{x27E7})\rightarrow {\mathcal{X}}$
be a lifting of the map
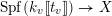 $\operatorname{Spf}(k_{v}\unicode[STIX]{x27E6}t_{v}\unicode[STIX]{x27E7})\rightarrow X$
of the completed local ring at
$\operatorname{Spf}(k_{v}\unicode[STIX]{x27E6}t_{v}\unicode[STIX]{x27E7})\rightarrow X$
of the completed local ring at
![]() $v$
into
$v$
into
![]() $X$
(previously,
$X$
(previously,
![]() $\mathbf{i}_{v}^{\ast }$
was simply notation, but shortly we shall see how to interpret it as a genuine pullback via
$\mathbf{i}_{v}^{\ast }$
was simply notation, but shortly we shall see how to interpret it as a genuine pullback via
![]() $\mathbf{i}_{v}$
). Let
$\mathbf{i}_{v}$
). Let
![]() ${\mathcal{D}}_{v}^{\dagger }$
denote the ring of arithmetic differential operators on
${\mathcal{D}}_{v}^{\dagger }$
denote the ring of arithmetic differential operators on
![]() $\mathfrak{X}_{v}$
as constructed by Crew in [Reference CrewCre12],
$\mathfrak{X}_{v}$
as constructed by Crew in [Reference CrewCre12],
![]() ${\mathcal{D}}_{v}^{\dagger }(v)$
the version with overconvergent singularities along the closed point of
${\mathcal{D}}_{v}^{\dagger }(v)$
the version with overconvergent singularities along the closed point of
![]() $\mathfrak{X}_{v}$
. Then (unlike the usual case in
$\mathfrak{X}_{v}$
. Then (unlike the usual case in
![]() ${\mathcal{D}}$
-module theory), the map
${\mathcal{D}}$
-module theory), the map
![]() $\mathbf{i}_{v}$
is actually a map of ringed spaces
$\mathbf{i}_{v}$
is actually a map of ringed spaces
 $(\mathfrak{X}_{v},{\mathcal{D}}_{v}^{\dagger })\rightarrow ({\mathcal{X}},{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
(respectively,
$(\mathfrak{X}_{v},{\mathcal{D}}_{v}^{\dagger })\rightarrow ({\mathcal{X}},{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger })$
(respectively,
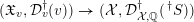 $(\mathfrak{X}_{v},{\mathcal{D}}_{v}^{\dagger }(v))\rightarrow ({\mathcal{X}},{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
) and hence we have natural pullback functors
$(\mathfrak{X}_{v},{\mathcal{D}}_{v}^{\dagger }(v))\rightarrow ({\mathcal{X}},{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S))$
) and hence we have natural pullback functors
 $$\begin{eqnarray}\displaystyle {\mathcal{E}} & \mapsto & \displaystyle {\mathcal{E}}\otimes _{{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }}{\mathcal{D}}_{v}^{\dagger }=:\mathbf{i}_{v}^{\ast }{\mathcal{E}},\nonumber\\ \displaystyle {\mathcal{E}} & \mapsto & \displaystyle {\mathcal{E}}\otimes _{{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)}{\mathcal{D}}_{v}^{\dagger }(v)=:\mathbf{i}_{v}^{\ast }{\mathcal{E}}\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{E}} & \mapsto & \displaystyle {\mathcal{E}}\otimes _{{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }}{\mathcal{D}}_{v}^{\dagger }=:\mathbf{i}_{v}^{\ast }{\mathcal{E}},\nonumber\\ \displaystyle {\mathcal{E}} & \mapsto & \displaystyle {\mathcal{E}}\otimes _{{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)}{\mathcal{D}}_{v}^{\dagger }(v)=:\mathbf{i}_{v}^{\ast }{\mathcal{E}}\nonumber\end{eqnarray}$$
from holonomic
 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules to holonomic
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules to holonomic
![]() $F\text{-}{\mathcal{D}}_{v}^{\dagger }$
-modules (respectively,
$F\text{-}{\mathcal{D}}_{v}^{\dagger }$
-modules (respectively,
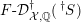 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-modules to
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-modules to
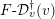 $F\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-modules). Note that we now have
$F\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-modules). Note that we now have
![]() $\mathbf{i}_{v}^{\ast }$
denoting three distinct functors; however, we shall shortly see that they are all compatible, so no essential confusion should arise.
$\mathbf{i}_{v}^{\ast }$
denoting three distinct functors; however, we shall shortly see that they are all compatible, so no essential confusion should arise.
As in the global case, we may define functors
![]() $j_{v+}$
and
$j_{v+}$
and
![]() $j_{v}^{+}$
to be restriction and extension of scalars respectively along the natural map
$j_{v}^{+}$
to be restriction and extension of scalars respectively along the natural map
 ${\mathcal{D}}_{v}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }(v)$
, as well as a duality functor
${\mathcal{D}}_{v}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }(v)$
, as well as a duality functor
![]() $\mathbf{D}$
using the machinery of [Reference VirrionVir00]. Defining
$\mathbf{D}$
using the machinery of [Reference VirrionVir00]. Defining
 $j_{v!}=\mathbf{D}j_{v+}\mathbf{D}$
, we then get adjoint pairs
$j_{v!}=\mathbf{D}j_{v+}\mathbf{D}$
, we then get adjoint pairs
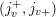 $(j_{v}^{+},j_{v+})$
and
$(j_{v}^{+},j_{v+})$
and
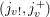 $(j_{v!},j_{v}^{+})$
as before, and hence we can define
$(j_{v!},j_{v}^{+})$
as before, and hence we can define
 $j_{v!+}{\mathcal{E}}=\operatorname{im}(j_{v!}{\mathcal{E}}\rightarrow j_{v+}{\mathcal{E}})$
for holonomic
$j_{v!+}{\mathcal{E}}=\operatorname{im}(j_{v!}{\mathcal{E}}\rightarrow j_{v+}{\mathcal{E}})$
for holonomic
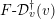 $F\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-module
$F\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-module
![]() ${\mathcal{E}}$
. Applying [Reference VirrionVir00, Proposition I.4.4] to the map of rings
${\mathcal{E}}$
. Applying [Reference VirrionVir00, Proposition I.4.4] to the map of rings
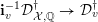 $\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }$
(respectively,
$\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }$
(respectively,
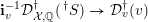 $\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)\rightarrow {\mathcal{D}}_{v}^{\dagger }(v)$
), we get
$\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)\rightarrow {\mathcal{D}}_{v}^{\dagger }(v)$
), we get
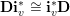 $\mathbf{D}\mathbf{i}_{v}^{\ast }\cong \mathbf{i}_{v}^{\ast }\mathbf{D}$
.
$\mathbf{D}\mathbf{i}_{v}^{\ast }\cong \mathbf{i}_{v}^{\ast }\mathbf{D}$
.
Claim. For any coherent
 ${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module
${\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module
![]() ${\mathcal{E}}$
we have
${\mathcal{E}}$
we have
 $$\begin{eqnarray}\mathbf{i}_{v}^{\ast }j_{+}{\mathcal{E}}\cong j_{v+}\mathbf{i}_{v}^{\ast }{\mathcal{E}}.\end{eqnarray}$$
$$\begin{eqnarray}\mathbf{i}_{v}^{\ast }j_{+}{\mathcal{E}}\cong j_{v+}\mathbf{i}_{v}^{\ast }{\mathcal{E}}.\end{eqnarray}$$
Proof of Claim.
In the usual manner we may reduce to the case of coherent
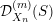 ${\mathcal{D}}_{X_{n}}^{(m)}(S)$
-modules
${\mathcal{D}}_{X_{n}}^{(m)}(S)$
-modules
![]() ${\mathcal{E}}$
, where
${\mathcal{E}}$
, where
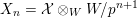 $X_{n}={\mathcal{X}}\otimes _{W}W/p^{n+1}$
and
$X_{n}={\mathcal{X}}\otimes _{W}W/p^{n+1}$
and
 $$\begin{eqnarray}{\mathcal{D}}_{X_{n}}^{(m)}(S):={\mathcal{B}}(t_{S},p^{m+1})\otimes {\mathcal{D}}_{X_{n}}^{(m)}\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{D}}_{X_{n}}^{(m)}(S):={\mathcal{B}}(t_{S},p^{m+1})\otimes {\mathcal{D}}_{X_{n}}^{(m)}\end{eqnarray}$$
is the ring of differential operators constructed in [Reference BerthelotBer96, § 4.2.5] (here
![]() $t_{S}$
is a section of
$t_{S}$
is a section of
![]() ${\mathcal{O}}_{{\mathcal{X}}}$
cutting out
${\mathcal{O}}_{{\mathcal{X}}}$
cutting out
![]() $S$
). Defining the local version
$S$
). Defining the local version
 $$\begin{eqnarray}{\mathcal{D}}_{v,n}^{(m)}(v):={\mathcal{B}}(t_{v},p^{m+1})\otimes {\mathcal{D}}_{v,n}^{(m)}\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{D}}_{v,n}^{(m)}(v):={\mathcal{B}}(t_{v},p^{m+1})\otimes {\mathcal{D}}_{v,n}^{(m)}\end{eqnarray}$$
entirely similarly, one can easily verify the isomorphism
 $$\begin{eqnarray}{\mathcal{D}}_{v,n}^{(m)}(v)\cong {\mathcal{D}}_{v,n}^{(m)}\otimes _{\boldsymbol{ i}_{v}^{-1}{\mathcal{D}}_{X_{n}}^{(m)}}\mathbf{i}_{v}^{-1}{\mathcal{D}}_{X_{n}}^{(m)}(S)\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{D}}_{v,n}^{(m)}(v)\cong {\mathcal{D}}_{v,n}^{(m)}\otimes _{\boldsymbol{ i}_{v}^{-1}{\mathcal{D}}_{X_{n}}^{(m)}}\mathbf{i}_{v}^{-1}{\mathcal{D}}_{X_{n}}^{(m)}(S)\end{eqnarray}$$
and the claim follows. ◻
Combining this with flatness of
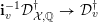 $\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }$
, we can see that we get an isomorphism
$\mathbf{i}_{v}^{-1}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }\rightarrow {\mathcal{D}}_{v}^{\dagger }$
, we can see that we get an isomorphism
 $$\begin{eqnarray}j_{v!+}(\mathbf{i}_{v}^{\ast }{\mathcal{E}})\cong \mathbf{i}_{v}^{\ast }(j_{!+}{\mathcal{E}})\end{eqnarray}$$
$$\begin{eqnarray}j_{v!+}(\mathbf{i}_{v}^{\ast }{\mathcal{E}})\cong \mathbf{i}_{v}^{\ast }(j_{!+}{\mathcal{E}})\end{eqnarray}$$
for any holonomic
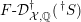 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }(\,^{\dagger }S)$
-module
![]() ${\mathcal{E}}$
. Let
${\mathcal{E}}$
. Let
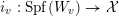 $i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
denote the composition of
$i_{v}:\operatorname{Spf}(W_{v})\rightarrow {\mathcal{X}}$
denote the composition of
![]() $\mathbf{i}_{v}$
with the inclusion of the zero section
$\mathbf{i}_{v}$
with the inclusion of the zero section
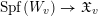 $\operatorname{Spf}(W_{v})\rightarrow \mathfrak{X}_{v}$
, so that
$\operatorname{Spf}(W_{v})\rightarrow \mathfrak{X}_{v}$
, so that
![]() $i_{v}^{+}$
factors through
$i_{v}^{+}$
factors through
![]() $\mathbf{i}_{v}^{\ast }$
. If we let
$\mathbf{i}_{v}^{\ast }$
. If we let
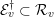 ${\mathcal{E}}_{v}^{\dagger }\subset {\mathcal{R}}_{v}$
denote a copy of the bounded Robba ring at
${\mathcal{E}}_{v}^{\dagger }\subset {\mathcal{R}}_{v}$
denote a copy of the bounded Robba ring at
![]() $v$
, then the pullback functor
$v$
, then the pullback functor
 $$\begin{eqnarray}\mathbf{i}_{v}^{\ast }:F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{v}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
$$\begin{eqnarray}\mathbf{i}_{v}^{\ast }:F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\rightarrow \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{R}}_{v}}^{\unicode[STIX]{x1D6FB}}\end{eqnarray}$$
factors through the category
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{E}}_{v}^{\dagger }}^{\unicode[STIX]{x1D6FB}}$
of
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{{\mathcal{E}}_{v}^{\dagger }}^{\unicode[STIX]{x1D6FB}}$
of
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{E}}_{v}^{\dagger }$
, and the diagram
${\mathcal{E}}_{v}^{\dagger }$
, and the diagram

commutes, where the right-hand vertical arrow is that viewing a
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module as a holonomic
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module as a holonomic
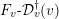 $F_{v}\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-module. The upshot of all these complicated trivialities is that to prove (2), we may reduce to the local case; in other words, we may replace
$F_{v}\text{-}{\mathcal{D}}_{v}^{\dagger }(v)$
-module. The upshot of all these complicated trivialities is that to prove (2), we may reduce to the local case; in other words, we may replace
![]() ${\mathcal{X}}$
by
${\mathcal{X}}$
by
![]() $\mathfrak{X}_{v}$
.
$\mathfrak{X}_{v}$
.
We may therefore suppose that we are given a
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-module
![]() $M$
over
$M$
over
![]() ${\mathcal{E}}_{v}^{\dagger }$
, and we must show that
${\mathcal{E}}_{v}^{\dagger }$
, and we must show that
 $$\begin{eqnarray}(i_{v}^{+}j_{v!+}M)[-1]\cong (M\otimes {\mathcal{R}}_{v})^{\unicode[STIX]{x1D6FB}=0}\end{eqnarray}$$
$$\begin{eqnarray}(i_{v}^{+}j_{v!+}M)[-1]\cong (M\otimes {\mathcal{R}}_{v})^{\unicode[STIX]{x1D6FB}=0}\end{eqnarray}$$
as
![]() $F_{v}$
-isocrystals over
$F_{v}$
-isocrystals over
![]() $K_{v}$
. Note that by [Reference CrewCre12, Proposition 5.1.3] the complex
$K_{v}$
. Note that by [Reference CrewCre12, Proposition 5.1.3] the complex
![]() $i_{v}^{+}j_{+}M$
is concentrated in degrees 0 and
$i_{v}^{+}j_{+}M$
is concentrated in degrees 0 and
![]() $-1$
. From the exact triangle
$-1$
. From the exact triangle
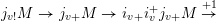 $j_{v!}M\rightarrow j_{v+}M\rightarrow i_{v+}i_{v}^{+}j_{v+}M\overset{+1}{\rightarrow }$
we therefore deduce the exact sequence
$j_{v!}M\rightarrow j_{v+}M\rightarrow i_{v+}i_{v}^{+}j_{v+}M\overset{+1}{\rightarrow }$
we therefore deduce the exact sequence
 $$\begin{eqnarray}0\rightarrow j_{v!+}M\rightarrow j_{v+}M\rightarrow i_{v+}H^{0}(i_{v}^{+}j_{v+}M)\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow j_{v!+}M\rightarrow j_{v+}M\rightarrow i_{v+}H^{0}(i_{v}^{+}j_{v+}M)\rightarrow 0\end{eqnarray}$$
of
![]() $F\text{-}{\mathcal{D}}_{v}^{\dagger }$
-modules. Now applying
$F\text{-}{\mathcal{D}}_{v}^{\dagger }$
-modules. Now applying
![]() $i_{v}^{+}$
and looking at the long exact sequence in cohomology, we can see that
$i_{v}^{+}$
and looking at the long exact sequence in cohomology, we can see that
 $i_{v}^{+}j_{v!+}M$
is concentrated in degree
$i_{v}^{+}j_{v!+}M$
is concentrated in degree
![]() $-1$
and that
$-1$
and that
 $i_{v}^{+}j_{!+}M[-1]\cong H^{-1}(i_{v}^{+}j_{v+}M)$
. Now the claim follows from again applying [Reference CrewCre12, Proposition 5.1.3].◻
$i_{v}^{+}j_{!+}M[-1]\cong H^{-1}(i_{v}^{+}j_{v+}M)$
. Now the claim follows from again applying [Reference CrewCre12, Proposition 5.1.3].◻
Hence applying Poincaré duality for
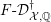 $F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules [Reference CaroCar06, Theorem 1.3.5] together with the cohomological formula for
$F\text{-}{\mathcal{D}}_{{\mathcal{X}},\mathbb{Q}}^{\dagger }$
-modules [Reference CaroCar06, Theorem 1.3.5] together with the cohomological formula for
![]() $L$
-functions [Reference CaroCar06, Théorème 3.3.4] gives the following corollary.
$L$
-functions [Reference CaroCar06, Théorème 3.3.4] gives the following corollary.
Corollary 7.3. For any
 ${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
we have
${\mathcal{F}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
we have
 $$\begin{eqnarray}\widehat{L}({\mathcal{F}},t)=\unicode[STIX]{x1D716}({\mathcal{F}},t)\widehat{L}({\mathcal{F}}^{\vee }(1),t^{-1})\end{eqnarray}$$
$$\begin{eqnarray}\widehat{L}({\mathcal{F}},t)=\unicode[STIX]{x1D716}({\mathcal{F}},t)\widehat{L}({\mathcal{F}}^{\vee }(1),t^{-1})\end{eqnarray}$$
where
![]() $\unicode[STIX]{x1D716}({\mathcal{F}},t)$
is a monomial in
$\unicode[STIX]{x1D716}({\mathcal{F}},t)$
is a monomial in
![]() $t$
.
$t$
.
The next key ingredient in the proof of Proposition 5.8 is the ability to twist overconvergent
![]() $F$
-isocrystals by characters of the idèle class group. So let
$F$
-isocrystals by characters of the idèle class group. So let
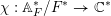 $\unicode[STIX]{x1D712}:\mathbb{A}_{F}^{\ast }/F^{\ast }\rightarrow \mathbb{C}^{\ast }$
be a finite-order character, unramified at all places of
$\unicode[STIX]{x1D712}:\mathbb{A}_{F}^{\ast }/F^{\ast }\rightarrow \mathbb{C}^{\ast }$
be a finite-order character, unramified at all places of
![]() $U$
. Via the isomorphism
$U$
. Via the isomorphism
 $\mathbb{A}_{F}^{\ast }/F^{\ast }\cong W_{F}^{\text{ab}}$
of global class field theory, we may view
$\mathbb{A}_{F}^{\ast }/F^{\ast }\cong W_{F}^{\text{ab}}$
of global class field theory, we may view
![]() $\unicode[STIX]{x1D712}$
as a character of the Weil group
$\unicode[STIX]{x1D712}$
as a character of the Weil group
![]() $W_{F}$
of
$W_{F}$
of
![]() $F$
, and, since the image of
$F$
, and, since the image of
![]() $\unicode[STIX]{x1D712}$
consists of roots of unity, we may choose a finite extension
$\unicode[STIX]{x1D712}$
consists of roots of unity, we may choose a finite extension
![]() $L/K$
and view
$L/K$
and view
![]() $\unicode[STIX]{x1D712}$
as a continuous character
$\unicode[STIX]{x1D712}$
as a continuous character
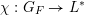 $\unicode[STIX]{x1D712}:G_{F}\rightarrow L^{\ast }$
, unramified at all places of
$\unicode[STIX]{x1D712}:G_{F}\rightarrow L^{\ast }$
, unramified at all places of
![]() $U$
. Hence we obtain a character
$U$
. Hence we obtain a character
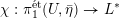 $\unicode[STIX]{x1D712}:\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})\rightarrow L^{\ast }$
where
$\unicode[STIX]{x1D712}:\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})\rightarrow L^{\ast }$
where
![]() $\bar{\unicode[STIX]{x1D702}}$
is some choice of geometric generic point.
$\bar{\unicode[STIX]{x1D702}}$
is some choice of geometric generic point.
Now, by [Reference CrewCre87, Theorem 2.1] there is an equivalence of categories between continuous
![]() $L$
-valued representations of
$L$
-valued representations of
 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
which have finite local monodromy at all places of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
which have finite local monodromy at all places of
![]() $S$
and the extension of scalars
$S$
and the extension of scalars
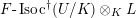 $F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
of the
$F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
of the
![]() $K$
-linear category
$K$
-linear category
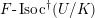 $F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
to
$F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
to
![]() $L$
. Hence we may associate to
$L$
. Hence we may associate to
![]() $\unicode[STIX]{x1D712}$
a rank-one isocrystal
$\unicode[STIX]{x1D712}$
a rank-one isocrystal
 ${\mathcal{E}}_{\unicode[STIX]{x1D712}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
. While we defined
${\mathcal{E}}_{\unicode[STIX]{x1D712}}\in F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
. While we defined
![]() $L$
-functions for objects in
$L$
-functions for objects in
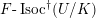 $F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
, essentially the same definition works for
$F\text{-}\operatorname{Isoc}^{\dagger }(U/K)$
, essentially the same definition works for
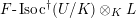 $F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
, and hence it makes sense to speak of the twisted
$F\text{-}\operatorname{Isoc}^{\dagger }(U/K)\otimes _{K}L$
, and hence it makes sense to speak of the twisted
![]() $L$
-function
$L$
-function
 $L({\mathcal{F}}\unicode[STIX]{x1D712},t):=L({\mathcal{F}}\otimes {\mathcal{E}}_{\unicode[STIX]{x1D712}},t)$
.
$L({\mathcal{F}}\unicode[STIX]{x1D712},t):=L({\mathcal{F}}\otimes {\mathcal{E}}_{\unicode[STIX]{x1D712}},t)$
.
To prove Proposition 5.8 we now proceed exactly as in the proof of [Reference DeligneDel73, Théorème 9.8], which shows that the semisimplifications
![]() $\{{\mathcal{F}}_{\ell ,c}^{\text{ss}}\}$
(as Weil–Deligne representations, i.e. not just their Frobenius semisimplifications) are weakly
$\{{\mathcal{F}}_{\ell ,c}^{\text{ss}}\}$
(as Weil–Deligne representations, i.e. not just their Frobenius semisimplifications) are weakly
![]() $\mathbb{Q}$
-compatible. Hence the traces of
$\mathbb{Q}$
-compatible. Hence the traces of
![]() $W_{F}$
of the graded pieces of the weight filtration have values in
$W_{F}$
of the graded pieces of the weight filtration have values in
![]() $\mathbb{Q}$
independence of
$\mathbb{Q}$
independence of
![]() $\ell$
, and we can then conclude using the weight monodromy theorem ([Reference DeligneDel80, Théorème 1.8.4] for
$\ell$
, and we can then conclude using the weight monodromy theorem ([Reference DeligneDel80, Théorème 1.8.4] for
![]() $\ell \neq p$
, [Reference CrewCre98, Theorem 10.8] for
$\ell \neq p$
, [Reference CrewCre98, Theorem 10.8] for
![]() $\ell =p$
), since this states that the weight filtration coincides with the monodromy filtration.
$\ell =p$
), since this states that the weight filtration coincides with the monodromy filtration.
Before moving on to unipotent fundamental groups over local fields, let us give an ‘application’ of Theorem 6.1 to give a new proof of a result of Abe and Caro [Reference Abe and CaroAC14, Corollary 4.3.13] concerning Hasse–Weil
![]() $L$
-functions of varieties over global function fields (at least in the semistable case). Of course, as remarked earlier, this ‘application’ is more of a special case of the proof of Proposition 5.8; however, we thought it was worth isolating as a separate result in its own right. So suppose that we have a smooth and proper variety
$L$
-functions of varieties over global function fields (at least in the semistable case). Of course, as remarked earlier, this ‘application’ is more of a special case of the proof of Proposition 5.8; however, we thought it was worth isolating as a separate result in its own right. So suppose that we have a smooth and proper variety
![]() $Y/F$
, then for any place
$Y/F$
, then for any place
![]() $v$
of
$v$
of
![]() $F$
, we can base change
$F$
, we can base change
![]() $Y$
to the completion
$Y$
to the completion
![]() $F_{v}$
, and consider the rigid cohomology groups
$F_{v}$
, and consider the rigid cohomology groups
 $H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})$
as
$H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})$
as
![]() $(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711}_{v},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{R}}_{v}$
.
${\mathcal{R}}_{v}$
.
Definition 7.4. Define
 $$\begin{eqnarray}\displaystyle L_{\text{HW},v}(F,H_{\text{rig}}^{i}(Y),t) & := & \displaystyle \det (1-\unicode[STIX]{x1D711}_{v}t^{\deg v}\mid H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})^{\unicode[STIX]{x1D6FB}=0}),\nonumber\\ \displaystyle L_{\text{HW}}(F,H_{\text{rig}}^{i}(Y),t) & = & \displaystyle \mathop{\prod }_{v}L_{\text{HW},v}(F,H_{\text{rig}}^{i}(Y),t).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle L_{\text{HW},v}(F,H_{\text{rig}}^{i}(Y),t) & := & \displaystyle \det (1-\unicode[STIX]{x1D711}_{v}t^{\deg v}\mid H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})^{\unicode[STIX]{x1D6FB}=0}),\nonumber\\ \displaystyle L_{\text{HW}}(F,H_{\text{rig}}^{i}(Y),t) & = & \displaystyle \mathop{\prod }_{v}L_{\text{HW},v}(F,H_{\text{rig}}^{i}(Y),t).\nonumber\end{eqnarray}$$
There is another
![]() $p$
-adic definition of the Hasse–Weil
$p$
-adic definition of the Hasse–Weil
![]() $L$
-function given by Abe and Caro in [Reference Abe and CaroAC14, § 4.3], which they denote by
$L$
-function given by Abe and Caro in [Reference Abe and CaroAC14, § 4.3], which they denote by
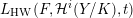 $L_{\text{HW}}(F,{\mathcal{H}}^{i}(Y/K),t)$
. To see that these two definitions coincide, given Proposition 7.1 above, it suffices to note that if we have a smooth projective model
$L_{\text{HW}}(F,{\mathcal{H}}^{i}(Y/K),t)$
. To see that these two definitions coincide, given Proposition 7.1 above, it suffices to note that if we have a smooth projective model
![]() ${\mathcal{Y}}\rightarrow U$
over some open subset of
${\mathcal{Y}}\rightarrow U$
over some open subset of
![]() $U$
, and let
$U$
, and let
![]() ${\mathcal{H}}^{i}$
denote Matsuda and Trihan’s overconvergent pushforward
${\mathcal{H}}^{i}$
denote Matsuda and Trihan’s overconvergent pushforward
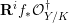 $\mathbf{R}^{i}f_{\ast }{\mathcal{O}}_{Y/K}^{\dagger }$
, then for any place
$\mathbf{R}^{i}f_{\ast }{\mathcal{O}}_{Y/K}^{\dagger }$
, then for any place
![]() $v$
of
$v$
of
![]() $F$
, we have
$F$
, we have
 $\mathbf{i}_{v}^{\ast }{\mathcal{H}}^{i}\cong H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})$
. This was noted in the proof of [Reference Lazda and PálLP16, Proposition 5.52]. We have the following ‘corollary’ of Theorem 6.1.
$\mathbf{i}_{v}^{\ast }{\mathcal{H}}^{i}\cong H_{\text{rig}}^{i}(Y_{F_{v}}/{\mathcal{R}}_{v})$
. This was noted in the proof of [Reference Lazda and PálLP16, Proposition 5.52]. We have the following ‘corollary’ of Theorem 6.1.
Corollary 7.5. Let
![]() $Y/F$
be a smooth and proper variety. Then
$Y/F$
be a smooth and proper variety. Then
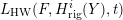 $L_{\text{HW}}(F,H_{\text{rig}}^{i}(Y),t)$
agrees with the Hasse–Weil
$L_{\text{HW}}(F,H_{\text{rig}}^{i}(Y),t)$
agrees with the Hasse–Weil
![]() $L$
-function
$L$
-function
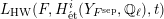 $L_{\text{HW}}(F,H_{\acute{\text{e}}\text{t}}^{i}(Y_{F^{\text{sep}}},\mathbb{Q}_{\ell }),t)$
attached to the
$L_{\text{HW}}(F,H_{\acute{\text{e}}\text{t}}^{i}(Y_{F^{\text{sep}}},\mathbb{Q}_{\ell }),t)$
attached to the
![]() $\ell$
-adic cohomology of
$\ell$
-adic cohomology of
![]() $Y$
.
$Y$
.
8 Unipotent fundamental groups over equicharacteristic local fields
We will now suppose that we have
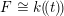 $F\cong k(\!(t)\!)$
equicharacteristic local. The main result of this section is the following theorem.
$F\cong k(\!(t)\!)$
equicharacteristic local. The main result of this section is the following theorem.
Theorem 8.1. Let
![]() $X/F$
be smooth and projective, with semistable reduction, and let
$X/F$
be smooth and projective, with semistable reduction, and let
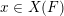 $x\in X(F)$
. Then
$x\in X(F)$
. Then
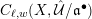 $C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
holds.
$C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
holds.
Of course, the basic strategy of proof will be identical to that in § 5. The first step will be in showing that an appropriate analogue of Propositions 5.3 and 5.4 holds for unipotent fundamental groups. So let
![]() ${\mathcal{X}}$
,
${\mathcal{X}}$
,
![]() ${\mathcal{X}}^{\times }$
,
${\mathcal{X}}^{\times }$
,
![]() $X_{0}^{\times }$
be as in the paragraph before Proposition 5.3, let
$X_{0}^{\times }$
be as in the paragraph before Proposition 5.3, let
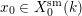 $x_{0}\in X_{0}^{\text{sm}}(k)$
denote the specialisation of our given point
$x_{0}\in X_{0}^{\text{sm}}(k)$
denote the specialisation of our given point
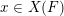 $x\in X(F)$
, and choose a geometric point
$x\in X(F)$
, and choose a geometric point
![]() $\bar{x}_{0}$
above it. For
$\bar{x}_{0}$
above it. For
![]() $\ell \neq p$
we will consider
$\ell \neq p$
we will consider
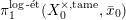 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})$
, the log-étale fundamental group of
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})$
, the log-étale fundamental group of
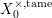 $X_{0}^{\times ,\text{tame}}$
as defined in [Reference LepageLep13, § 2]. This has a natural
$X_{0}^{\times ,\text{tame}}$
as defined in [Reference LepageLep13, § 2]. This has a natural
![]() $G_{F}$
-action (trivial on wild inertia
$G_{F}$
-action (trivial on wild inertia
![]() $P_{F}$
), and hence so does its
$P_{F}$
), and hence so does its
![]() $\mathbb{Q}_{\ell }$
-pro-unipotent completion
$\mathbb{Q}_{\ell }$
-pro-unipotent completion
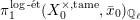 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})_{\mathbb{Q}_{\ell }}$
.
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})_{\mathbb{Q}_{\ell }}$
.
Proposition 8.2 [Reference LepageLep13, Theorems 2.5, 2.7].
There is an isomorphism
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})_{\mathbb{Q}_{\ell }}\cong \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},\bar{x}_{0})_{\mathbb{Q}_{\ell }}\cong \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},\bar{x})_{\mathbb{Q}_{\ell }}\end{eqnarray}$$
of pro-unipotent groups with
![]() $G_{F}$
-action.
$G_{F}$
-action.
For
![]() $\ell =p$
we will consider the category
$\ell =p$
we will consider the category
 $\operatorname{Uni}_{K}(X_{0}^{\times }/K^{\times })$
of unipotent log-isocrystals on
$\operatorname{Uni}_{K}(X_{0}^{\times }/K^{\times })$
of unipotent log-isocrystals on
![]() $X_{0}^{\times }$
, relative to
$X_{0}^{\times }$
, relative to
![]() $W$
equipped with the Teichmüller lift of the log structure on
$W$
equipped with the Teichmüller lift of the log structure on
![]() $k$
defined by
$k$
defined by
![]() $\mathbb{N}\rightarrow k$
,
$\mathbb{N}\rightarrow k$
,
![]() $1\mapsto 0$
, as defined in [Reference ShihoShi00, § 4]. This is a Tannakian category by [Reference ShihoShi00, Proposition 4.14], and
$1\mapsto 0$
, as defined in [Reference ShihoShi00, § 4]. This is a Tannakian category by [Reference ShihoShi00, Proposition 4.14], and
![]() $x_{0}$
defines a fibre functor
$x_{0}$
defines a fibre functor
 $$\begin{eqnarray}x_{0}^{\ast }:\operatorname{Uni}_{K}(X_{0}^{\times }/K^{\times })\rightarrow \operatorname{Vec}_{K}.\end{eqnarray}$$
$$\begin{eqnarray}x_{0}^{\ast }:\operatorname{Uni}_{K}(X_{0}^{\times }/K^{\times })\rightarrow \operatorname{Vec}_{K}.\end{eqnarray}$$
We let
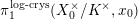 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
denote the corresponding pro-unipotent group. To put a Frobenius and monodromy operator on this pro-unipotent group, that is, to turn this into a non-abelian
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
denote the corresponding pro-unipotent group. To put a Frobenius and monodromy operator on this pro-unipotent group, that is, to turn this into a non-abelian
![]() $(\unicode[STIX]{x1D711},N)$
-module, we follow the approach of [Reference LazdaLaz16]. So let
$(\unicode[STIX]{x1D711},N)$
-module, we follow the approach of [Reference LazdaLaz16]. So let
![]() $k^{\times }$
denote
$k^{\times }$
denote
![]() $k$
considered with the log structure of the punctured point, and
$k$
considered with the log structure of the punctured point, and
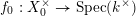 $f_{0}:X_{0}^{\times }\rightarrow \operatorname{Spec}(k^{\times })$
the structure morphism.
$f_{0}:X_{0}^{\times }\rightarrow \operatorname{Spec}(k^{\times })$
the structure morphism.
Consider the Tannakian category
 $$\begin{eqnarray}{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\end{eqnarray}$$
of relatively unipotent log-
![]() $F$
-isocrystals on
$F$
-isocrystals on
![]() $X_{0}$
, relative to
$X_{0}$
, relative to
![]() $W$
equipped with the trivial log structure. In other words,
$W$
equipped with the trivial log structure. In other words,
 $$\begin{eqnarray}{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\subset F\text{-Crys}(X_{0}^{\times }/W)_{\mathbb{ Q}}\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\subset F\text{-Crys}(X_{0}^{\times }/W)_{\mathbb{ Q}}\end{eqnarray}$$
is the full sub-category of objects which are iterated extensions of those pulled back from the category
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
of
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
of
![]() $(\unicode[STIX]{x1D711},N)$
-modules via
$(\unicode[STIX]{x1D711},N)$
-modules via
 $$\begin{eqnarray}f_{0}^{\ast }:\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}=F\text{-Isoc}(\operatorname{Spec}(k^{\times })/K)\rightarrow F\text{-Isoc}^{\dagger }(X_{0}^{\times }/K).\end{eqnarray}$$
$$\begin{eqnarray}f_{0}^{\ast }:\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}=F\text{-Isoc}(\operatorname{Spec}(k^{\times })/K)\rightarrow F\text{-Isoc}^{\dagger }(X_{0}^{\times }/K).\end{eqnarray}$$
We therefore have a pair of functors
 $$\begin{eqnarray}x_{0}^{\ast }:{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\leftrightarrows \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}:f_{0}^{\ast },\end{eqnarray}$$
$$\begin{eqnarray}x_{0}^{\ast }:{\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K)\leftrightarrows \text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}:f_{0}^{\ast },\end{eqnarray}$$
and using the formalism of [Reference LazdaLaz15, § 2.1], we may attach to this situation a ‘relative fundamental group’
 $$\begin{eqnarray}G_{X_{0}^{\times },x_{0}}^{\log \text{-}\text{crys}}:=G({\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K),x_{0}^{\ast })\end{eqnarray}$$
$$\begin{eqnarray}G_{X_{0}^{\times },x_{0}}^{\log \text{-}\text{crys}}:=G({\mathcal{N}}F\text{-Isoc}(X_{0}^{\times }/K),x_{0}^{\ast })\end{eqnarray}$$
in the terminology of [Reference LazdaLaz15]; this is a pro-unipotent group scheme over the category
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
. Concretely, then, such an object is something of the form
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
. Concretely, then, such an object is something of the form
 $\operatorname{Spec}(A)$
, where
$\operatorname{Spec}(A)$
, where
![]() $A$
is a Hopf
$A$
is a Hopf
![]() $K$
-algebra equipped with the structure of an ind-
$K$
-algebra equipped with the structure of an ind-
![]() $(\unicode[STIX]{x1D711},N)$
-module. Moreover, the Hopf-algebra and
$(\unicode[STIX]{x1D711},N)$
-module. Moreover, the Hopf-algebra and
![]() $(\unicode[STIX]{x1D711},N)$
-module structures are compatible in the sense that the maps
$(\unicode[STIX]{x1D711},N)$
-module structures are compatible in the sense that the maps
 $$\begin{eqnarray}\displaystyle A\otimes _{K}A\overset{m}{\rightarrow }A,\quad A\overset{c}{\rightarrow }A\otimes _{K}A, & & \displaystyle \nonumber\\ \displaystyle K\overset{u}{\rightarrow }A,\quad A\overset{\unicode[STIX]{x1D700}}{\rightarrow }K,\quad A\overset{\unicode[STIX]{x1D704}}{\rightarrow }A, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle A\otimes _{K}A\overset{m}{\rightarrow }A,\quad A\overset{c}{\rightarrow }A\otimes _{K}A, & & \displaystyle \nonumber\\ \displaystyle K\overset{u}{\rightarrow }A,\quad A\overset{\unicode[STIX]{x1D700}}{\rightarrow }K,\quad A\overset{\unicode[STIX]{x1D704}}{\rightarrow }A, & & \displaystyle \nonumber\end{eqnarray}$$
expressing the Hopf algebra structure of
![]() $A$
, are in fact morphisms of
$A$
, are in fact morphisms of
![]() $(\unicode[STIX]{x1D711},N)$
-modules. By [Reference LazdaLaz15, Proposition 2.3] this group scheme
$(\unicode[STIX]{x1D711},N)$
-modules. By [Reference LazdaLaz15, Proposition 2.3] this group scheme
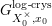 $G_{X_{0}^{\times },x_{0}}^{\log \text{-}\text{crys}}$
is uniquely characterised by the fact that its category of enriched representations, that is, representations in objects of the category
$G_{X_{0}^{\times },x_{0}}^{\log \text{-}\text{crys}}$
is uniquely characterised by the fact that its category of enriched representations, that is, representations in objects of the category
![]() $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
, is equivalent to
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{K}^{N}$
, is equivalent to
 ${\mathcal{N}}F\text{-}\operatorname{Isoc}(X_{0}^{\times }/K)$
in such a way that
${\mathcal{N}}F\text{-}\operatorname{Isoc}(X_{0}^{\times }/K)$
in such a way that
![]() $x_{0}^{\ast }$
gets identified with the forgetful functor.
$x_{0}^{\ast }$
gets identified with the forgetful functor.
By [Reference LazdaLaz16, Proposition 9.9] the affine group scheme over
![]() $K$
underlying
$K$
underlying
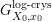 $G_{X_{0},x_{0}}^{\log \text{-}\text{crys}}$
, that is, the affine group scheme obtained by forgetting
$G_{X_{0},x_{0}}^{\log \text{-}\text{crys}}$
, that is, the affine group scheme obtained by forgetting
![]() $(\unicode[STIX]{x1D711},N)$
-module structures, is simply
$(\unicode[STIX]{x1D711},N)$
-module structures, is simply
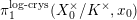 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
. Hence we may view this latter group scheme canonically as having the structure of a non-abelian
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
. Hence we may view this latter group scheme canonically as having the structure of a non-abelian
![]() $(\unicode[STIX]{x1D711},N)$
-module. Hence the base change
$(\unicode[STIX]{x1D711},N)$
-module. Hence the base change
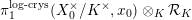 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\otimes _{K}{\mathcal{R}}_{K}$
can be viewed as a non-abelian
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\otimes _{K}{\mathcal{R}}_{K}$
can be viewed as a non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module over
![]() ${\mathcal{R}}_{K}$
. Alternatively, we may consider the non-abelian
${\mathcal{R}}_{K}$
. Alternatively, we may consider the non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
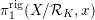 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)$
defined in § 2. The following result is then the non-abelian analogue of Proposition 5.4.
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)$
defined in § 2. The following result is then the non-abelian analogue of Proposition 5.4.
Proposition 8.3. There is a natural isomorphism
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\otimes _{K}{\mathcal{R}}_{K}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\otimes _{K}{\mathcal{R}}_{K}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x)\end{eqnarray}$$
of non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules.
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules.
Proof. By definition there exists a non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
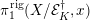 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
such that
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)$
such that
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\otimes _{{\mathcal{E}}_{K}^{\dagger }}{\mathcal{R}}_{K}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{R}}_{K},x).\end{eqnarray}$$
Let
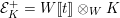 ${\mathcal{E}}_{K}^{+}=W\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7}\otimes _{W}K$
be the ring of bounded power series over
${\mathcal{E}}_{K}^{+}=W\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7}\otimes _{W}K$
be the ring of bounded power series over
![]() $K$
; thus we have
$K$
; thus we have
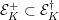 ${\mathcal{E}}_{K}^{+}\subset {\mathcal{E}}_{K}^{\dagger }$
as the sub-ring of series with no terms in negative powers of
${\mathcal{E}}_{K}^{+}\subset {\mathcal{E}}_{K}^{\dagger }$
as the sub-ring of series with no terms in negative powers of
![]() $t$
, and we also have a natural ‘evaluate at
$t$
, and we also have a natural ‘evaluate at
![]() $t=0$
map’
$t=0$
map’
 ${\mathcal{E}}_{K}^{+}\rightarrow K$
. Thanks to [Reference TsuzukiTsu98, Proposition 3.1.4], the category
${\mathcal{E}}_{K}^{+}\rightarrow K$
. Thanks to [Reference TsuzukiTsu98, Proposition 3.1.4], the category
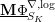 $\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{S_{K}}^{\unicode[STIX]{x1D6FB},\log }$
of log-
$\text{}\underline{\mathbf{M}\unicode[STIX]{x1D6F7}}_{S_{K}}^{\unicode[STIX]{x1D6FB},\log }$
of log-
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{E}}_{K}^{+}$
is Tannakian, and it was shown in [Reference LazdaLaz16, § 9] (see, in particular, Propositions 9.6 and 9.9) that there exists a non-abelian log-
${\mathcal{E}}_{K}^{+}$
is Tannakian, and it was shown in [Reference LazdaLaz16, § 9] (see, in particular, Propositions 9.6 and 9.9) that there exists a non-abelian log-
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-module
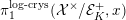 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)$
over
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)$
over
![]() ${\mathcal{E}}_{K}^{+}$
such that
${\mathcal{E}}_{K}^{+}$
such that
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x) & \cong & \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)\otimes _{{\mathcal{E}}_{K}^{+}}{\mathcal{E}}_{K}^{\dagger },\nonumber\\ \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0}) & \cong & \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)\otimes _{{\mathcal{E}}_{K}^{+}}K.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x) & \cong & \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)\otimes _{{\mathcal{E}}_{K}^{+}}{\mathcal{E}}_{K}^{\dagger },\nonumber\\ \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0}) & \cong & \displaystyle \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}({\mathcal{X}}^{\times }/{\mathcal{E}}_{K}^{+},x)\otimes _{{\mathcal{E}}_{K}^{+}}K.\nonumber\end{eqnarray}$$
We can thus conclude by applying [Reference Lazda and PálLP16, Proposition 5.34]. ◻
Now following the argument of the previous section, and given Theorem 4.1, to prove Theorem 8.1 it suffices to show the following. Suppose that we are given a smooth, geometrically connected curve
![]() $C/k$
,
$C/k$
,
 $c\in C(k)$
such that
$c\in C(k)$
such that
 $F\cong \widehat{k(C)}_{c}$
, and a semistable scheme
$F\cong \widehat{k(C)}_{c}$
, and a semistable scheme
 ${\mathcal{X}}\rightarrow C$
, smooth away from
${\mathcal{X}}\rightarrow C$
, smooth away from
![]() $c$
, such that
$c$
, such that
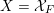 $X={\mathcal{X}}_{F}$
. Suppose further that
$X={\mathcal{X}}_{F}$
. Suppose further that
![]() $x$
comes from a section
$x$
comes from a section
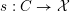 $s:C\rightarrow {\mathcal{X}}$
. Let
$s:C\rightarrow {\mathcal{X}}$
. Let
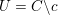 $U=C\setminus c$
. Then we want to show that there exist local systems
$U=C\setminus c$
. Then we want to show that there exist local systems
![]() ${\mathcal{F}}_{\ell ,k}$
on
${\mathcal{F}}_{\ell ,k}$
on
![]() $U$
for all
$U$
for all
![]() $\ell$
prime and
$\ell$
prime and
![]() $k\geqslant 1$
such that:
$k\geqslant 1$
such that:
-
(i) for any closed point
 $y\in U$
the fibre of
$y\in U$
the fibre of
 ${\mathcal{F}}_{\ell ,k}$
at
${\mathcal{F}}_{\ell ,k}$
at
 $y$
is isomorphic (as a
$y$
is isomorphic (as a
 $W_{k(y)}$
-representation) to the quotient of the universal enveloping algebra of
$W_{k(y)}$
-representation) to the quotient of the universal enveloping algebra of
 $\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }({\mathcal{X}}_{y},s(y))$
by the
$\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }({\mathcal{X}}_{y},s(y))$
by the
 $k$
th power of its augmentation ideal;
$k$
th power of its augmentation ideal; -
(ii) the fibre of
 ${\mathcal{F}}_{\ell ,k}$
at
${\mathcal{F}}_{\ell ,k}$
at
 $c$
is isomorphic (as a
$c$
is isomorphic (as a
 $W_{F}^{\prime }$
-representation) to the quotient of the universal enveloping algebra of
$W_{F}^{\prime }$
-representation) to the quotient of the universal enveloping algebra of
 $\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
by the
$\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\ell }(X,x)$
by the
 $k$
th power of its augmentation ideal.
$k$
th power of its augmentation ideal.
For
![]() $\ell =p$
these follow from the results of [Reference LazdaLaz15, § 3] and [Reference LazdaLaz16, § 6]. Specifically, in [Reference LazdaLaz15, § 3] it was shown how to define, in the above situation, a pro-unipotent affine group scheme
$\ell =p$
these follow from the results of [Reference LazdaLaz15, § 3] and [Reference LazdaLaz16, § 6]. Specifically, in [Reference LazdaLaz15, § 3] it was shown how to define, in the above situation, a pro-unipotent affine group scheme
 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)$
over the category
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)$
over the category
 $F\text{-}\operatorname{Isoc}(U/K)$
, such that for any closed point
$F\text{-}\operatorname{Isoc}(U/K)$
, such that for any closed point
![]() $y\in U$
the fibre
$y\in U$
the fibre
 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{y}$
can be identified with the unipotent rigid fundamental group
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{y}$
can be identified with the unipotent rigid fundamental group
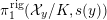 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{y}/K,s(y))$
of the fibre
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{y}/K,s(y))$
of the fibre
![]() ${\mathcal{X}}_{y}$
. It was then proved in [Reference LazdaLaz16, Theorem 6.1] that we have an isomorphism
${\mathcal{X}}_{y}$
. It was then proved in [Reference LazdaLaz16, Theorem 6.1] that we have an isomorphism
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{c}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{c}\cong \unicode[STIX]{x1D70B}_{1}^{\text{rig}}(X/{\mathcal{E}}_{K}^{\dagger },x)\end{eqnarray}$$
as non-abelian
![]() $(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
$(\unicode[STIX]{x1D711},\unicode[STIX]{x1D6FB})$
-modules over
![]() ${\mathcal{E}}_{K}^{\dagger }$
, where
${\mathcal{E}}_{K}^{\dagger }$
, where
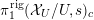 $\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{c}$
denotes the ‘fibre’ at
$\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)_{c}$
denotes the ‘fibre’ at
![]() $c$
. Taking the quotients of the universal enveloping algebra of
$c$
. Taking the quotients of the universal enveloping algebra of
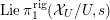 $\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)$
by the powers of the augmentation ideal then gives us the required
$\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\text{rig}}({\mathcal{X}}_{U}/U,s)$
by the powers of the augmentation ideal then gives us the required
![]() $F$
-isocrystals on
$F$
-isocrystals on
![]() $U/K$
.
$U/K$
.
For
![]() $\ell \neq p$
these two follow from Grothendieck’s homotopy exact sequence: we know that
$\ell \neq p$
these two follow from Grothendieck’s homotopy exact sequence: we know that
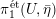 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
acts on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
acts on
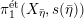 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))$
, hence on its pro-unipotent completion
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))$
, hence on its pro-unipotent completion
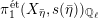 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }}$
and therefore on the universal enveloping algebra
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }}$
and therefore on the universal enveloping algebra
 $\hat{{\mathcal{U}}}(\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }})$
of the associated Lie algebra (again, here
$\hat{{\mathcal{U}}}(\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }})$
of the associated Lie algebra (again, here
![]() $\bar{\unicode[STIX]{x1D702}}$
is any geometric generic point). Hence there is a natural action of
$\bar{\unicode[STIX]{x1D702}}$
is any geometric generic point). Hence there is a natural action of
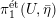 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U,\bar{\unicode[STIX]{x1D702}})$
on
 $\hat{{\mathcal{U}}}(\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }})/\mathfrak{a}^{k}$
for any
$\hat{{\mathcal{U}}}(\operatorname{Lie}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{\bar{\unicode[STIX]{x1D702}}},s(\bar{\unicode[STIX]{x1D702}}))_{\mathbb{Q}_{\ell }})/\mathfrak{a}^{k}$
for any
![]() $k$
(
$k$
(
![]() $\mathfrak{a}$
being the augmentation ideal), and this gives the required lisse
$\mathfrak{a}$
being the augmentation ideal), and this gives the required lisse
![]() $\mathbb{Q}_{\ell }$
-sheaf on
$\mathbb{Q}_{\ell }$
-sheaf on
![]() $U$
.
$U$
.
The astute reader will object that there is a slight gap in the argument outlined above, in that while we can certainly assume that our variety is globally defined, we have not explained why we can assume the existence of a global section. Examining the proof of Proposition 5.2, it suffices to observe that again the given formal section can be arbitrarily well approximated by a Henselian section, which must descend to some étale neighbourhood of
![]() $s$
. While this will not agree with our given formal section, it will do so on the special fibre, which suffices by Propositions 8.2 and 8.3.
$s$
. While this will not agree with our given formal section, it will do so on the special fibre, which suffices by Propositions 8.2 and 8.3.
9 Curves in mixed characteristic
Finally, we will use the results of the previous section to deduce an
![]() $\ell$
-independence result for the unipotent fundamental group of a semistable curve in mixed characteristic. So for this section
$\ell$
-independence result for the unipotent fundamental group of a semistable curve in mixed characteristic. So for this section
![]() $F$
will be assumed to be a mixed characteristic local field, with ring of integers
$F$
will be assumed to be a mixed characteristic local field, with ring of integers
![]() $R$
, and our main result is as follows.
$R$
, and our main result is as follows.
Theorem 9.1. Let
![]() ${\mathcal{X}}/R$
be a proper, semistable curve with generic fibre
${\mathcal{X}}/R$
be a proper, semistable curve with generic fibre
![]() $X$
, and
$X$
, and
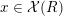 $x\in {\mathcal{X}}(R)$
. Then the conjecture
$x\in {\mathcal{X}}(R)$
. Then the conjecture
 $C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
holds.
$C_{\ell ,w}(X,\hat{{\mathcal{U}}}/\mathfrak{a}^{\bullet })$
holds.
Proof. The basic idea is that
![]() $(\hat{{\mathcal{U}}}/\mathfrak{a}^{k})_{\ell }$
(as a Weil–Deligne representation) only depends on the special fibre
$(\hat{{\mathcal{U}}}/\mathfrak{a}^{k})_{\ell }$
(as a Weil–Deligne representation) only depends on the special fibre
![]() $X_{0}^{\times }$
of
$X_{0}^{\times }$
of
![]() ${\mathcal{X}}$
as a log scheme, and since
${\mathcal{X}}$
as a log scheme, and since
![]() $X_{0}$
is a curve, this deforms to equicharacteristic. More concretely, when
$X_{0}$
is a curve, this deforms to equicharacteristic. More concretely, when
![]() $\ell \neq p$
we consider the log-unipotent fundamental group
$\ell \neq p$
we consider the log-unipotent fundamental group
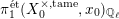 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}$
of the log scheme
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}$
of the log scheme
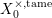 $X_{0}^{\times ,\text{tame}}$
as a
$X_{0}^{\times ,\text{tame}}$
as a
![]() $G_{F}$
-representation; then again applying [Reference LepageLep13, Theorems 2.3, 2.5], we have
$G_{F}$
-representation; then again applying [Reference LepageLep13, Theorems 2.3, 2.5], we have
 $$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}\cong \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},x)_{\mathbb{Q}_{\ell }}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}\cong \unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},x)_{\mathbb{Q}_{\ell }}\end{eqnarray}$$
as
![]() $G_{F}$
-representations. When
$G_{F}$
-representations. When
![]() $\ell =p$
we instead consider the log-crystalline fundamental group
$\ell =p$
we instead consider the log-crystalline fundamental group
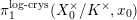 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
defined in § 8 above. Thus by [Reference Andreatta, Iovita and KimAIK15, Theorem 1.4] or [Reference LazdaLaz16, Theorem 10.2] we have an isomorphism
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
defined in § 8 above. Thus by [Reference Andreatta, Iovita and KimAIK15, Theorem 1.4] or [Reference LazdaLaz16, Theorem 10.2] we have an isomorphism
 $$\begin{eqnarray}\mathbf{D}_{\text{st}}(\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},x)_{\mathbb{Q}_{p}})\cong \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\end{eqnarray}$$
$$\begin{eqnarray}\mathbf{D}_{\text{st}}(\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{F^{\text{sep}}},x)_{\mathbb{Q}_{p}})\cong \unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})\end{eqnarray}$$
of
![]() $(\unicode[STIX]{x1D711},N)$
-modules over
$(\unicode[STIX]{x1D711},N)$
-modules over
![]() $K$
(after forgetting the Hodge filtration on the former).
$K$
(after forgetting the Hodge filtration on the former).
But now since the log-smooth deformation theory of curves is unobstructed by [Reference KatoKat96, Proposition 8.6], we may deform
![]() $X_{0}^{\times }$
to characteristic
$X_{0}^{\times }$
to characteristic
![]() $p$
; in other words, there exists a semistable family
$p$
; in other words, there exists a semistable family
 ${\mathcal{Y}}\rightarrow \operatorname{Spec}(k\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7})$
whose special fibre is isomorphic to
${\mathcal{Y}}\rightarrow \operatorname{Spec}(k\unicode[STIX]{x27E6}t\unicode[STIX]{x27E7})$
whose special fibre is isomorphic to
![]() $X_{0}^{\times }$
as a log scheme. Hence by applying Theorem 8.1 we know that the Weil–Deligne representations associated to the quotients of the universal enveloping algebras of
$X_{0}^{\times }$
as a log scheme. Hence by applying Theorem 8.1 we know that the Weil–Deligne representations associated to the quotients of the universal enveloping algebras of
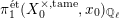 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}$
and
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X_{0}^{\times ,\text{tame}},x_{0})_{\mathbb{Q}_{\ell }}$
and
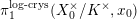 $\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
are weakly independent of
$\unicode[STIX]{x1D70B}_{1}^{\log \text{-}\text{crys}}(X_{0}^{\times }/K^{\times },x_{0})$
are weakly independent of
![]() $\ell$
, and we may conclude.◻
$\ell$
, and we may conclude.◻
Acknowledgements
Both authors would like to thank W. Nizioł for suggesting the question of
![]() $\ell$
-independence for unipotent fundamental groups over fields such as
$\ell$
-independence for unipotent fundamental groups over fields such as
![]() $k(\!(t)\!)$
, and C. Lazda would like to thank A. Pál for first interesting him in
$k(\!(t)\!)$
, and C. Lazda would like to thank A. Pál for first interesting him in
![]() $\ell$
-independence questions in general, as well as for useful discussions related to the contents of this article. We would also like to thank the anonymous referee for a careful reading of the article.
$\ell$
-independence questions in general, as well as for useful discussions related to the contents of this article. We would also like to thank the anonymous referee for a careful reading of the article.