Hostname: page-component-76fb5796d-vfjqv Total loading time: 0 Render date: 2024-04-27T00:36:45.923Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear rigidity of stationary stochastic processes

Published online by Cambridge University Press:  03 April 2017

ALEXANDER I. BUFETOV ,
YOANN DABROWSKI  and
YANQI QIU Open the ORCID record for YANQI QIU [Opens in a new window]
ALEXANDER I. BUFETOV
Affiliation:
Aix-Marseille Université, Centrale Marseille, CNRS, I2M, UMR7373, 39 rue F. Joliot Curie, 13453 Marseille Cedex 13, France email yqi.qiu@gmail.com Steklov Institute of Mathematics, 8 Gubkina Street, Moscow, 119991, Russia Institute for Information Transmission Problems, Bolshoy Karetny per. 19, 127994, Moscow, Russia National Research University Higher School of Economics, Myasnitskaya ul., 20, Moscow, 101000, Russia email bufetov@mi.ras.ru
YOANN DABROWSKI
Affiliation:
Université de Lyon, Université Lyon 1, Institut Camille Jordan, 43 bd. du 11 novembre 1918, 69622 Villeurbanne Cedex, France email dabrowski@math.univ-lyon1.fr
YANQI QIU
Affiliation:
Aix-Marseille Université, Centrale Marseille, CNRS, I2M, UMR7373, 39 rue F. Joliot Curie, 13453 Marseille Cedex 13, France email yqi.qiu@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider stationary stochastic processes 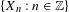$\{X_{n}:n\in \mathbb{Z}\}$ such that $X_{0}$ lies in the closed linear span of 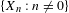$\{X_{n}:n\neq 0\}$; following Ghosh and Peres, we call such processes linearly rigid. Using a criterion of Kolmogorov, we show that it suffices, for a stationary stochastic process to be linearly rigid, that the spectral density vanishes at zero and belongs to the Zygmund class 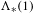$\unicode[STIX]{x1D6EC}_{\ast }(1)$. We next give a sufficient condition for stationary determinantal point processes on $\mathbb{Z}$ and on 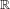$\mathbb{R}$ to be linearly rigid. Finally, we show that the determinantal point process on 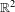$\mathbb{R}^{2}$ induced by a tensor square of Dyson sine kernels is not linearly rigid.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 38 , Issue 7 , October 2018 , pp. 2493 - 2507
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bufetov, A. I.. Rigidity of determinantal point processes with the Airy, the Bessel and the Gamma kernel. Bull. Math. Sci. 6(1) (2016), 163–172.Google Scholar
Costin, O. and Lebowitz, J. L.. Gaussian fluctuation in random matrices. Phys. Rev. Lett. 75(1) (1995), 69–72.Google Scholar
Daley, D. J. and Vere-Jones, D.. An Introduction to the Theory of Point Processes. Vol. I, Elementary Theory and Methods (Probability and its Applications) . Springer, New York, Berlin, Paris, 2003.Google Scholar
Fülöp, V. and Móricz, F.. Absolutely convergent multiple Fourier series and multiplicative Zygmund classes of functions. Analysis 28(3) (2008), 345–354.Google Scholar
Ghosh, S.. Determinantal processes and completeness of random exponentials: the critical case. Probab. Theory Related Fields 163(3–4) (2015), 643–665.Google Scholar
Ghosh, S. and Peres, Y.. Rigidity and tolerance in point processes: Gaussian zeros and Ginibre eigenvalues. Duke Math. J., to appear. Preprint, 2012, arXiv:1211.3506.Google Scholar
Holroyd, A. E. and Soo, T.. Insertion and deletion tolerance of point processes. Electron. J. Probab. 18(74) (2013), 24 pp.Google Scholar
Hough, J. B., Krishnapur, M., Peres, Y. and Virág, B.. Determinantal processes and independence. Probab. Surv. 3 (2006), 206–229.Google Scholar
Kolmogoroff, A. N.. Interpolation und Extrapolation von stationaeren zufaelligen Folgen. Izv. Math. (Izv. Akad. Nauk SSSR Ser. Mat.) 5(1) (1941), 3–14.Google Scholar
Kolmogorov, A. N.. Stationary sequences in Hilbert space. Vestnik MGU (Bull. Univ. Moscou) 2(6) (1941), 1–40.Google Scholar
Lyons, R.. Determinantal probability measures. Publ. Math. Inst. Hautes Études Sci. (98) (2003), 167–212.Google Scholar
Lyons, R. and Steif, J. E.. Stationary determinantal processes: phase multiplicity, Bernoullicity, entropy, and domination. Duke Math. J. 120(3) (2003), 515–575.Google Scholar
Macchi, O.. The coincidence approach to stochastic point processes. Adv. Appl. Probab. 7 (1975), 83–122.Google Scholar
Móricz, F.. Absolutely convergent Fourier series and function classes. J. Math. Anal. Appl. 324(2) (2006), 1168–1177.Google Scholar
Nikolski, N. K.. Operators, Functions, and Systems: An Easy Reading. Vol. 1: Hardy, Hankel, and Toeplitz (Mathematical Surveys and Monographs, 92) . American Mathematical Society, Providence, RI, 2002, translated from the French by Andreas Hartmann.Google Scholar
Soshnikov, A.. Determinantal random point fields. Uspekhi Mat. Nauk 55(5(335)) (2000), 107–160.Google Scholar