Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dörr, Aaron
and
Hardt, Steffen
2015.
Driven particles at fluid interfaces acting as capillary dipoles.
Journal of Fluid Mechanics,
Vol. 770,
Issue. ,
p.
5.
Tanaka, Shinpei
Sogabe, Yoshimi
and
Nakata, Satoshi
2015.
Spontaneous change in trajectory patterns of a self-propelled oil droplet at the air-surfactant solution interface.
Physical Review E,
Vol. 91,
Issue. 3,
Domínguez, Alvaro
Malgaretti, P.
Popescu, M. N.
and
Dietrich, S.
2016.
Effective Interaction between Active Colloids and Fluid Interfaces Induced by Marangoni Flows.
Physical Review Letters,
Vol. 116,
Issue. 7,
Domínguez, Alvaro
Malgaretti, P.
Popescu, M. N.
and
Dietrich, S.
2016.
Collective dynamics of chemically active particles trapped at a fluid interface.
Soft Matter,
Vol. 12,
Issue. 40,
p.
8398.
Girot, A.
Danné, N.
Würger, A.
Bickel, T.
Ren, F.
Loudet, J. C.
and
Pouligny, B.
2016.
Motion of Optically Heated Spheres at the Water–Air Interface.
Langmuir,
Vol. 32,
Issue. 11,
p.
2687.
Paven, Maxime
Mayama, Hiroyuki
Sekido, Takafumi
Butt, Hans‐Jürgen
Nakamura, Yoshinobu
and
Fujii, Syuji
2016.
Light‐Driven Delivery and Release of Materials Using Liquid Marbles.
Advanced Functional Materials,
Vol. 26,
Issue. 19,
p.
3199.
Malgaretti, P.
Popescu, M. N.
and
Dietrich, S.
2016.
Active colloids at fluid interfaces.
Soft Matter,
Vol. 12,
Issue. 17,
p.
4007.
Ng, Fei Chong
Abas, Aizat
Ishak, MHH
Abdullah, MZ
and
Aziz, Abdul
2016.
Effect of thermocapillary action in the underfill encapsulation of multi-stack ball grid array.
Microelectronics Reliability,
Vol. 66,
Issue. ,
p.
143.
Zöttl, Andreas
and
Stark, Holger
2016.
Emergent behavior in active colloids.
Journal of Physics: Condensed Matter,
Vol. 28,
Issue. 25,
p.
253001.
Popescu, Mihail N.
Uspal, William E.
and
Dietrich, Siegfried
2016.
Self-diffusiophoresis of chemically active colloids.
The European Physical Journal Special Topics,
Vol. 225,
Issue. 11-12,
p.
2189.
Kawashima, Hisato
Paven, Maxime
Mayama, Hiroyuki
Butt, Hans-Jürgen
Nakamura, Yoshinobu
and
Fujii, Syuji
2017.
Transfer of Materials from Water to Solid Surfaces Using Liquid Marbles.
ACS Applied Materials & Interfaces,
Vol. 9,
Issue. 38,
p.
33351.
Popescu, M N
Uspal, W E
and
Dietrich, S
2017.
Chemically active colloids near osmotic-responsive walls with surface-chemistry gradients.
Journal of Physics: Condensed Matter,
Vol. 29,
Issue. 13,
p.
134001.
Ryazantsev, Yuri S.
Velarde, Manuel G.
Rubio, Ramón G.
Guzmán, Eduardo
Ortega, Francisco
and
López, Pilar
2017.
Thermo- and soluto-capillarity: Passive and active drops.
Advances in Colloid and Interface Science,
Vol. 247,
Issue. ,
p.
52.
Irajizad, Peyman
Ray, Sahil
Farokhnia, Nazanin
Hasnain, Munib
Baldelli, Steven
and
Ghasemi, Hadi
2017.
Remote Droplet Manipulation on Self‐Healing Thermally Activated Magnetic Slippery Surfaces.
Advanced Materials Interfaces,
Vol. 4,
Issue. 12,
Janssens, Stoffel D.
Chaurasia, Vikash
and
Fried, Eliot
2017.
Effect of a surface tension imbalance on a partly submerged cylinder.
Journal of Fluid Mechanics,
Vol. 830,
Issue. ,
p.
369.
Fei, Wenjie
Gu, Yang
and
Bishop, Kyle J.M.
2017.
Active colloidal particles at fluid-fluid interfaces.
Current Opinion in Colloid & Interface Science,
Vol. 32,
Issue. ,
p.
57.
Oshanin, G
Popescu, M N
and
Dietrich, S
2017.
Active colloids in the context of chemical kinetics.
Journal of Physics A: Mathematical and Theoretical,
Vol. 50,
Issue. 13,
p.
134001.
Vandadi, Vahid
Kang, Saeed Jafari
and
Masoud, Hassan
2017.
Reverse Marangoni surfing.
Journal of Fluid Mechanics,
Vol. 811,
Issue. ,
p.
612.
Selmke, Markus
Khadka, Utsab
Bregulla, Andreas P.
Cichos, Frank
and
Yang, Haw
2018.
Theory for controlling individual self-propelled micro-swimmers by photon nudging I: directed transport.
Physical Chemistry Chemical Physics,
Vol. 20,
Issue. 15,
p.
10502.
Malgaretti, P.
Popescu, M. N.
and
Dietrich, S.
2018.
Self-diffusiophoresis induced by fluid interfaces.
Soft Matter,
Vol. 14,
Issue. 8,
p.
1375.
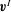 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}{\boldsymbol {v}}^{I}$ due to the stress discontinuity at the interface and the short-range velocity field
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}{\boldsymbol {v}}^{I}$ due to the stress discontinuity at the interface and the short-range velocity field 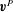 ${\boldsymbol {v}}^{P}$ imposed by the no-slip condition on the particle surface. Both contributions are evaluated for a spherical floater with temperature monopole and dipole moments. We find that the self-propulsion velocity is given by the amplitude of the ‘source doublet’ that belongs to the short-range contribution
${\boldsymbol {v}}^{P}$ imposed by the no-slip condition on the particle surface. Both contributions are evaluated for a spherical floater with temperature monopole and dipole moments. We find that the self-propulsion velocity is given by the amplitude of the ‘source doublet’ that belongs to the short-range contribution 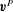 ${\boldsymbol {v}}^{P}$. Hydrodynamic interactions, on the other hand, are determined by the long-range Marangoni flow
${\boldsymbol {v}}^{P}$. Hydrodynamic interactions, on the other hand, are determined by the long-range Marangoni flow 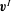 ${\boldsymbol {v}}^{I}$. Its dipolar part results in an asymmetric advection pattern of neighbouring particles, which in turn may perturb the known hexatic lattice or even favour disordered states.
${\boldsymbol {v}}^{I}$. Its dipolar part results in an asymmetric advection pattern of neighbouring particles, which in turn may perturb the known hexatic lattice or even favour disordered states.

