Article contents
Comparison of variational balance models for the rotating shallow water equations
Published online by Cambridge University Press: 07 June 2017
Abstract
We present an extensive numerical comparison of a family of balance models appropriate to the semi-geostrophic limit of the rotating shallow water equations, and derived by variational asymptotics in Oliver (J. Fluid Mech., vol. 551, 2006, pp. 197–234) for small Rossby numbers 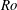 $Ro$ . This family of generalized large-scale semi-geostrophic (GLSG) models contains the
$Ro$ . This family of generalized large-scale semi-geostrophic (GLSG) models contains the 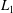 $L_{1}$ -model introduced by Salmon (J. Fluid Mech., vol. 132, 1983, pp. 431–444) as a special case. We use these models to produce balanced initial states for the full shallow water equations. We then numerically investigate how well these models capture the dynamics of an initially balanced shallow water flow. It is shown that, whereas the
$L_{1}$ -model introduced by Salmon (J. Fluid Mech., vol. 132, 1983, pp. 431–444) as a special case. We use these models to produce balanced initial states for the full shallow water equations. We then numerically investigate how well these models capture the dynamics of an initially balanced shallow water flow. It is shown that, whereas the 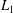 $L_{1}$ -member of the GLSG family is able to reproduce the balanced dynamics of the full shallow water equations on time scales of
$L_{1}$ -member of the GLSG family is able to reproduce the balanced dynamics of the full shallow water equations on time scales of 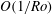 $O(1/Ro)$ very well, all other members develop significant unphysical high wavenumber contributions in the ageostrophic vorticity which spoil the dynamics.
$O(1/Ro)$ very well, all other members develop significant unphysical high wavenumber contributions in the ageostrophic vorticity which spoil the dynamics.
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 6
- Cited by


