Article contents
Friction properties of superhydrophobic ridges
Published online by Cambridge University Press: 13 March 2020
Abstract
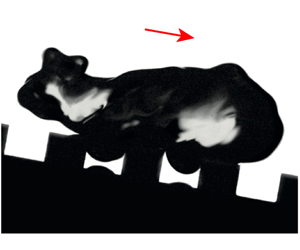
The extreme mobility of droplets on non-wetting materials implies the necessity of controlling their motion, direction or speed. In this paper, we show how ridges allow us to tune drop friction. Depending on the liquid speed and viscosity, two regimes emerge: fast drops with low viscosity dynamically deform and undergo inertial friction, so that their velocity is eventually fixed by the deformations induced by the ridges; in contrast, viscous drops hardly interact with the texture, so that their velocity is classically limited by viscous dissipation, as on a flat substrate. The transition between these two regimes reveals spectacular morphological changes: drops with intermediate viscosity elongate and adopt worm-like shapes, which we qualitatively describe.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
de Maleprade et al. supplementary movie 1
Water droplet with volume 100µL deposited on a superhydrophobic track tilted by 10.4°, with texture of wavelength 3 mm and depth 1 mm. The movie is slowed down 100x, and the camera is tilted by the same angle as the track. The vertical orange lines highlight the length over which bulges hit merlons.
de Maleprade et al. supplementary movie 2
100µL-droplet of viscosity 500 mPa.s, on a superhydrophobic track tilted by 11.5°, with texture of wavelength 3 mm and depth 1 mm. The movie is slowed down 4x, and the camera is tilted by the same angle as the track.
de Maleprade et al. supplementary movie 3
100µL-droplet of viscosity 2 mPa.s, on a superhydrophobic track tilted by 12.4°, with texture of wavelength 3 mm and depth 1 mm. The movie is slowed down 60x, and the camera is tilted by the same angle as the track.
de Maleprade et al. supplementary movie 4
100µL-droplet of viscosity 3 mPa.s, on a superhydrophobic track tilted by 12.4°, with texture of wavelength 3 mm and depth 1 mm. The movie is slowed down 60x, and the camera is tilted by the same angle as the track.
de Maleprade et al. supplementary movie 5
100µL-droplet of viscosity 5 mPa.s, on a superhydrophobic track tilted by 12.4°, with texture of wavelength 3 mm and depth 1 mm. The movie is slowed down 60x, and the camera is tilted by the same angle as the track.
- 9
- Cited by

