Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Espinosa-Gayosso, Alexis
Ghisalberti, Marco
Ivey, Gregory N.
and
Jones, Nicole L.
2013.
Particle capture by a circular cylinder in the vortex-shedding regime.
Journal of Fluid Mechanics,
Vol. 733,
Issue. ,
p.
171.
Espinosa-Gayosso, Alexis
Ghisalberti, Marco
Ivey, Gregory N.
and
Jones, Nicole L.
2015.
Density-ratio effects on the capture of suspended particles in aquatic systems.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
p.
191.
Duru, Paul
and
Hallez, Yannick
2015.
A Three-Step Scenario Involved in Particle Capture on a Pore Edge.
Langmuir,
Vol. 31,
Issue. 30,
p.
8310.
Lowe, Ryan J.
and
Falter, James L.
2015.
Oceanic Forcing of Coral Reefs.
Annual Review of Marine Science,
Vol. 7,
Issue. 1,
p.
43.
Dersoir, B.
Schofield, A. B.
and
Tabuteau, H.
2017.
Clogging transition induced by self filtration in a slit pore.
Soft Matter,
Vol. 13,
Issue. 10,
p.
2054.
Kuruneru, Sahan T.W.
Sauret, Emilie
Saha, Suvash C.
and
Gu, Yuan Tong
2017.
A coupled finite volume & discrete element method to examine particulate foulant transport in metal foam heat exchangers.
International Journal of Heat and Mass Transfer,
Vol. 115,
Issue. ,
p.
43.
Fu, Pengbo
Jiang, Xia
Ma, Liang
Yang, Qiang
Bai, Zhishan
Yang, Xuejing
Chen, Jianqi
Yuan, Wei
Wang, Hualin
and
Lv, Wenjie
2018.
Enhancement of PM2.5 Cyclone Separation by Droplet Capture and Particle Sorting.
Environmental Science & Technology,
McCombe, Dori
and
Ackerman, Josef D.
2018.
Collector Motion Affects Particle Capture in Physical Models and in Wind Pollination.
The American Naturalist,
Vol. 192,
Issue. 1,
p.
81.
Divi, Raj V.
Strother, James A.
and
Paig-Tran, E. W. Misty
2018.
Manta rays feed using ricochet separation, a novel nonclogging filtration mechanism.
Science Advances,
Vol. 4,
Issue. 9,
Irvine, Joshua Lelemia
and
Kim, Albert S.
2019.
Hydraulic design perspectives of bioswale vegetation layers: a meta-research theory.
Desalination and Water Treatment,
Vol. 143,
Issue. ,
p.
137.
Liu, Q.
Zhao, B.
and
Santamarina, J. C.
2019.
Particle Migration and Clogging in Porous Media: A Convergent Flow Microfluidics Study.
Journal of Geophysical Research: Solid Earth,
Vol. 124,
Issue. 9,
p.
9495.
Chang, You-Im
Liao, Keng-Yi
and
Jang, Larry
2019.
New correlations for the initial collection efficiency of Brownian aerosol particles with the bounce-off effect considered in granular filtration.
Separation and Purification Technology,
Vol. 229,
Issue. ,
p.
115586.
Chen, Hongsheng
Liu, Wenwei
and
Li, Shuiqing
2019.
Deposition of wet microparticles on a fiber: Effects of impact velocity and initial spin.
Powder Technology,
Vol. 357,
Issue. ,
p.
83.
Yao, Xin
and
Marcos
2020.
Creeping flow of a sphere nearby a cylinder.
Applied Mathematical Modelling,
Vol. 79,
Issue. ,
p.
18.
Spencer, Thomas L.
Mohebbi, Nina
Jin, Guangyuan
Forister, Matthew L.
Alexeev, Alexander
and
Hu, David L.
2020.
Moth-inspired methods for particle capture on a cylinder.
Journal of Fluid Mechanics,
Vol. 884,
Issue. ,
Boudina, Mouad
Gosselin, Frédérick P.
and
Étienne, Stéphane
2020.
Direct interception or inertial impaction? A theoretical derivation of the efficiency power law for a simple and practical definition of capture modes.
Physics of Fluids,
Vol. 32,
Issue. 12,
Secchi, Eleonora
Vitale, Alessandra
Miño, Gastón L.
Kantsler, Vasily
Eberl, Leo
Rusconi, Roberto
and
Stocker, Roman
2020.
The effect of flow on swimming bacteria controls the initial colonization of curved surfaces.
Nature Communications,
Vol. 11,
Issue. 1,
Boudina, Mouad
Gosselin, Frédérick P.
and
Étienne, Stéphane
2021.
Vortex-induced vibrations: a soft coral feeding strategy?.
Journal of Fluid Mechanics,
Vol. 916,
Issue. ,
Ji, Junhong
Li, Deqiang
Du, Hongxian
Jiang, Yu
Zhang, Sitong
and
Huang, Youkang
2021.
Behavior of Inertial Capture for Micron Particles with a Droplet in a Wide Diameter Range.
SSRN Electronic Journal ,
Espinosa-Gayosso, Alexis
Ghisalberti, Marco
Shimeta, Jeff
Ivey, Gregory N.
and
Tian, Fang-Bao
2021.
On predicting particle capture rates in aquatic ecosystems.
PLOS ONE,
Vol. 16,
Issue. 12,
p.
e0261400.
 ), the rate of particle capture determines the efficiencies of a range of processes such as seagrass pollination, suspension feeding by corals and larval settlement. In this paper, we use direct numerical simulation (DNS) of a two-dimensional laminar flow to accurately quantify the rate of capture of low-inertia particles by a cylindrical collector for
), the rate of particle capture determines the efficiencies of a range of processes such as seagrass pollination, suspension feeding by corals and larval settlement. In this paper, we use direct numerical simulation (DNS) of a two-dimensional laminar flow to accurately quantify the rate of capture of low-inertia particles by a cylindrical collector for 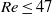 (i.e. a range where there is no vortex shedding). We investigate the dependence of both the capture rate and maximum capture angle on both the collector Reynolds number and the ratio of particle size to collector size. The inner asymptotic expansion of Skinner (Q. J. Mech. Appl. Maths, vol. 28, 1975, pp. 333–340) for flow around a cylinder is extended and shown to provide an excellent framework for the prediction of particle capture and flow close to the leading face of a cylinder up to
(i.e. a range where there is no vortex shedding). We investigate the dependence of both the capture rate and maximum capture angle on both the collector Reynolds number and the ratio of particle size to collector size. The inner asymptotic expansion of Skinner (Q. J. Mech. Appl. Maths, vol. 28, 1975, pp. 333–340) for flow around a cylinder is extended and shown to provide an excellent framework for the prediction of particle capture and flow close to the leading face of a cylinder up to 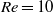 . Our results fill a gap between theory and experiment by providing, for the first time, predictive capability for particle capture by aquatic collectors in a wide (and relevant) Reynolds number and particle size range.
. Our results fill a gap between theory and experiment by providing, for the first time, predictive capability for particle capture by aquatic collectors in a wide (and relevant) Reynolds number and particle size range.
