Hostname: page-component-848d4c4894-x5gtn Total loading time: 0 Render date: 2024-05-14T17:54:51.062Z Has data issue: false hasContentIssue false
  • English
  • Français

The moduli space of polynomial maps and their fixed-point multipliers: II. Improvement to the algorithm and monic centered polynomials

Part of: Complex dynamical systems Families, fibrations Combinatorics

Published online by Cambridge University Press:  03 February 2023

TOSHI SUGIYAMA
TOSHI SUGIYAMA*
Affiliation:
Mathematics Studies, Gifu Pharmaceutical University, Mitahora-higashi 5-6-1, Gifu-city, Gifu 502-8585, Japan
*
e-mail: sugiyama-to@gifu-pu.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider the family 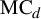$\mathrm {MC}_d$ of monic centered polynomials of one complex variable with degree 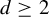$d \geq 2$, and study the map 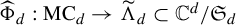$\widehat {\Phi }_d:\mathrm {MC}_d\to \widetilde {\Lambda }_d \subset \mathbb {C}^d / \mathfrak {S}_d$ which maps each 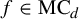$f \in \mathrm {MC}_d$ to its unordered collection of fixed-point multipliers. We give an explicit formula for counting the number of elements of each fiber 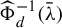$\widehat {\Phi }_d^{-1}(\bar {\unicode{x3bb} })$ for every 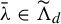$\bar {\unicode{x3bb} } \in \widetilde {\Lambda }_d$ except when the fiber 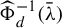$\widehat {\Phi }_d^{-1}(\bar {\unicode{x3bb} })$ contains polynomials having multiple fixed points. This formula is not a recursive one, and is a drastic improvement of our previous result [T. Sugiyama. The moduli space of polynomial maps and their fixed-point multipliers. Adv. Math. 322 (2017), 132–185] which gave a rather long algorithm with some induction processes.

Keywords

complex dynamicsfixed-point multipliersmoduli space of polynomial mapspartition of integersinclusion-exclusion formula

MSC classification

Primary: 37F10: Polynomials; rational maps; entire and meromorphic functions
Secondary: 05A19: Combinatorial identities, bijective combinatorics 14D20: Algebraic moduli problems, moduli of vector bundles
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 43 , Issue 11 , November 2023 , pp. 3777 - 3795
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

DeMarco, L. and McMullen, C.. Trees and the dynamics of polynomials. Ann. Sci. Éc. Norm. Supér. (4) 41 (2008), 337–383.10.24033/asens.2070CrossRefGoogle Scholar
Fujimura, M.. Projective moduli space for the polynomials. Dyn. Contin. Discrete Impuls. Syst. Ser. A Math. Anal. 13 (2006), 787–801.Google Scholar
Fujimura, M.. The moduli space of rational maps and surjectivity of multiplier representation. Comput. Methods Funct. Theory 7(2) (2007), 345–360.10.1007/BF03321649CrossRefGoogle Scholar
Fujimura, M. and Taniguchi, M.. A compactification of the moduli space of polynomials. Proc. Amer. Math. Soc. 136(10) (2008), 3601–3609.10.1090/S0002-9939-08-09344-1CrossRefGoogle Scholar
Gorbovickis, I.. Algebraic independence of multipliers of periodic orbits in the space of rational maps of the Riemann sphere. Mosc. Math. J. 15(1) (2015), 73–87.10.17323/1609-4514-2015-15-1-73-87CrossRefGoogle Scholar
Gorbovickis, I.. Algebraic independence of multipliers of periodic orbits in the space of polynomial maps of one variable. Ergod. Th. & Dynam. Sys. 36(4) (2016), 1156–1166.10.1017/etds.2014.103CrossRefGoogle Scholar
Hutz, B. and Tepper, M.. Multiplier spectra and the moduli space of degree 3 morphisms on ${\mathbb{P}}^1$ . JP J. Algebra, Number Theory Appl. 29(2) (2013), 189–206.Google Scholar
McMullen, C.. Families of rational maps and iterative root-finding algorithms. Ann. of Math. (2) 125(3) (1987), 467–493.10.2307/1971408CrossRefGoogle Scholar
Milnor, J.. Remarks on iterated cubic maps. Exp. Math. 1(1) (1992), 5–24.Google Scholar
Milnor, J.. Geometry and dynamics of quadratic rational maps. Exp. Math. 2(1) (1993), 37–83.10.1080/10586458.1993.10504267CrossRefGoogle Scholar
Milnor, J.. Dynamics in One Complex Variable (Annals of Mathematics Studies, 160), 3rd edn. Princeton University Press, Princeton, NJ, 2006.Google Scholar
Nishizawa, K. and Fujimura, M.. Moduli space of polynomial maps with degree four. Josai Inform. Sci. Res. 9 (1997), 1–10.Google Scholar
Silverman, J. H.. The space of rational maps on ${\mathbf{P}}^1$ . Duke Math. J. 94(1) (1998), 41–77.10.1215/S0012-7094-98-09404-2CrossRefGoogle Scholar
Sugiyama, T.. The moduli space of polynomial maps and their fixed-point multipliers. Adv. Math. 322 (2017), 132–185.10.1016/j.aim.2017.10.013CrossRefGoogle Scholar