Article contents
The strain-induced martensitic phase transformation of Fe–C alloys considering C addition: A molecular dynamics study
Published online by Cambridge University Press: 30 June 2020
Abstract
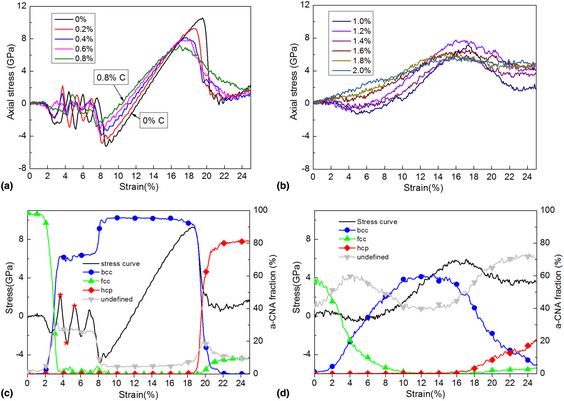
This study investigates the effect of C on the deformation mechanisms in Fe–C alloys by molecular dynamics simulations. In uniaxial tensile simulations, the face-centered-cubic (fcc) structures of Fe–C alloys undergo the following deformation processes: (i) fcc→body-centered-cubic (bcc) martensitic transformation, (ii) deformation of bcc phase, and (iii) bcc→hcp martensitic transformation, which are significantly influenced by the C concentration. For the low C concentrations (0–0.8 wt%) fcc phase, the fcc→bcc phase transformation accords a two-stage shear transformation mechanism based on the Bain model, the deformation mechanism of the bcc phase is the first migration of twinning structures and then elastic deformation, and the bcc→hcp phase transformation follows Burgers relations resulting from the shear of the bcc close-packed layers. However, for the fcc phase with high C concentrations (1.0–2.0 wt%), the fcc→bcc phase transformation follows a localized Bain transformation mechanism impeded by the C atoms, the bcc phase only experiences elastic deformation, and the bcc→hcp phase transformation also conforms to Burgers relations but become localized due to the addition of more C atoms. Because of the different phase transformation mechanisms between the high C and low C supercells, the dislocation generation mechanism is also different.
Keywords
- Type
- Article
- Information
- Copyright
- Copyright © Materials Research Society 2020
References
- 5
- Cited by



