Article contents
Taylor–Couette turbulence at radius ratio  ${\it\eta}=0.5$: scaling, flow structures and plumes
${\it\eta}=0.5$: scaling, flow structures and plumes
Published online by Cambridge University Press: 23 June 2016
Abstract
Using high-resolution particle image velocimetry, we measure velocity profiles, the wind Reynolds number and characteristics of turbulent plumes in Taylor–Couette flow for a radius ratio of 0.5 and Taylor number of up to 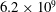 $6.2\times 10^{9}$. The extracted angular velocity profiles follow a log law more closely than the azimuthal velocity profiles due to the strong curvature of this
$6.2\times 10^{9}$. The extracted angular velocity profiles follow a log law more closely than the azimuthal velocity profiles due to the strong curvature of this 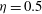 ${\it\eta}=0.5$ set-up. The scaling of the wind Reynolds number with the Taylor number agrees with the theoretically predicted
${\it\eta}=0.5$ set-up. The scaling of the wind Reynolds number with the Taylor number agrees with the theoretically predicted 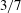 $3/7$ scaling for the classical turbulent regime, which is much more pronounced than for the well-explored
$3/7$ scaling for the classical turbulent regime, which is much more pronounced than for the well-explored 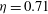 ${\it\eta}=0.71$ case, for which the ultimate regime sets in at much lower Taylor number. By measuring at varying axial positions, roll structures are found for counter-rotation while no clear coherent structures are seen for pure inner cylinder rotation. In addition, turbulent plumes coming from the inner and outer cylinders are investigated. For pure inner cylinder rotation, the plumes in the radial velocity move away from the inner cylinder, while the plumes in the azimuthal velocity mainly move away from the outer cylinder. For counter-rotation, the mean radial flow in the roll structures strongly affects the direction and intensity of the turbulent plumes. Furthermore, it is experimentally confirmed that, in regions where plumes are emitted, boundary layer profiles with a logarithmic signature are created.
${\it\eta}=0.71$ case, for which the ultimate regime sets in at much lower Taylor number. By measuring at varying axial positions, roll structures are found for counter-rotation while no clear coherent structures are seen for pure inner cylinder rotation. In addition, turbulent plumes coming from the inner and outer cylinders are investigated. For pure inner cylinder rotation, the plumes in the radial velocity move away from the inner cylinder, while the plumes in the azimuthal velocity mainly move away from the outer cylinder. For counter-rotation, the mean radial flow in the roll structures strongly affects the direction and intensity of the turbulent plumes. Furthermore, it is experimentally confirmed that, in regions where plumes are emitted, boundary layer profiles with a logarithmic signature are created.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
- 16
- Cited by



