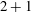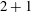Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cohen, David
and
Verdier, Olivier
2016.
MultiSymplectic Discretization of Wave Map Equations.
SIAM Journal on Scientific Computing,
Vol. 38,
Issue. 2,
p.
A953.
Lou, Qiong
2020.
Global existence of smooth solutions for wave maps in de Sitter spacetime.
Journal of Mathematical Analysis and Applications,
Vol. 487,
Issue. 1,
p.
123966.