If a thin elastic circular plate B: ∣z∣ < 1 is clamped (simply supported, respectively) along its edge ∣z∣ = 1, its deflection at z ∈ B under a point load at ζ ∈ B, measured positively in the direction of the gravitational pull, is the biharmonic Green's function β(z, ζ) of the clamped plate (γ(z, ζ) of the simply supported plate, respectively). We ask: how do β(z, ζ) and γ(z, ζ) compare with the corresponding deflections β0(z, ζ) and γ0(z, ζ) of the punctured circular plate B0: 0 < ∣ z ∣ < 1 that is “clamped” or “simply supported”, respectively, also at the origin? We shall show that γ(z, ζ) is not affected by the puncturing, that is, γ(·, ζ) = γ0(·, ζ), whereas β(·, ζ) is:

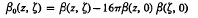
on B0 × B0. Moreover, while β((·, ζ) is of constant sign, β0(·, ζ) is not. This gives a simple counterexmple to the conjecture of Hadamard [6] that the deflection of a clampled thin elastic plate be always of constant sign:

The biharmonic Gree's function of a clampled concentric circular annulus is not of constant sign if the radius of the inner boundary circle is sufficiently small.

Earlier counterexamples to Hadamard's conjecture were given by Duffin [2], Garabedian [4], Loewner [7], and Szegö [9]. Interest in the problem was recently revived by the invited address of Duffin [3] before the Annual Meeting of the American Mathematical Society in 1974. The drawback of the counterexample we will present is that, whereas the classical examples are all simply connected, ours is not. In the simplicity of the proof, however, there is no comparison.