We characterise bounded and compact generalised weighted composition operators acting from the weighted Bergman space $A^p_\omega $ , where $0<p<\infty $ and 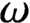$\omega $ belongs to the class $\mathcal {D}$ of radial weights satisfying a two-sided doubling condition, to a Lebesgue space 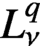$L^q_\nu $ . On the way, we establish a new embedding theorem on weighted Bergman spaces $A^p_\omega $ which generalises the well-known characterisation of the boundedness of the differentiation operator 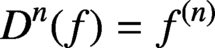$D^n(f)=f^{(n)}$ from the classical weighted Bergman space 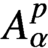$A^p_\alpha $ to the Lebesgue space $L^q_\mu $ , induced by a positive Borel measure 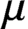$\mu $ , to the setting of doubling weights.