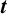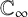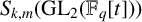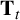Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bandini, Andrea
and
Valentino, Maria
2023.
Fourier coefficients and slopes of Drinfeld modular forms.
International Journal of Number Theory,
Vol. 19,
Issue. 10,
p.
2523.
DALAL, TARUN
and
KUMAR, NARASIMHA
2023.
NOTES ON ATKIN–LEHNER THEORY FOR DRINFELD MODULAR FORMS.
Bulletin of the Australian Mathematical Society,
Vol. 108,
Issue. 1,
p.
50.