Let f be analytic in the unit disk $\mathbb {D}=\{z\in \mathbb {C}:|z|<1 \}$ and let ${\mathcal S}$ be the subclass of normalised univalent functions with 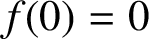$f(0)=0$ and 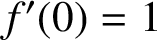$f'(0)=1$, given by 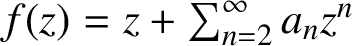$f(z)=z+\sum _{n=2}^{\infty }a_n z^n$. Let F be the inverse function of f, given by $F(\omega )=\omega +\sum _{n=2}^{\infty }A_n \omega ^n$ for 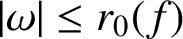$|\omega |\le r_0(f)$. Denote by 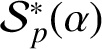$ \mathcal {S}_p^{* }(\alpha )$ the subset of 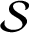$ \mathcal {S}$ consisting of the spirallike functions of order $\alpha $ in 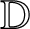$\mathbb {D}$, that is, functions satisfying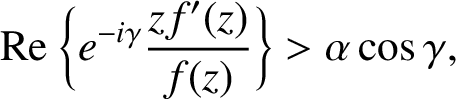

$$\begin{align*}{\mathrm{Re}} \ \bigg\{e^{-i\gamma}\dfrac{zf'(z)}{f(z)}\bigg\}>\alpha\cos \gamma, \end{align*}$$

for 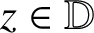$z\in \mathbb {D}$, 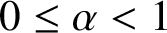$0\le \alpha <1$ and 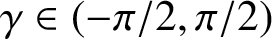$\gamma \in (-\pi /2,\pi /2)$. We give sharp upper and lower bounds for both 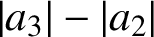$ |a_3|-|a_2| $ and 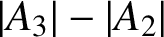$ |A_3|-|A_2| $ when 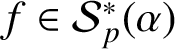$f\in \mathcal {S}_p^{* }(\alpha )$, thus solving an open problem and presenting some new inequalities for coefficient differences.