Hostname: page-component-8448b6f56d-gtxcr Total loading time: 0 Render date: 2024-04-24T12:25:47.431Z Has data issue: false hasContentIssue false
  • English
  • Français

Characterisation Results for Steiner Triple Systems and Their Application to Edge-Colourings of Cubic Graphs

Published online by Cambridge University Press:  20 November 2018

Daniel Král’ ,
Edita Máčajová ,
Attila Pór  and
Jean-Sébastien Sereni
Daniel Král’
Affiliation:
(Král) Institute for Theoretical Computer Science, Faculty of Mathematics and Physics, Charles University, Prague, Czech Republic, e-mail: kral@kam.mff.cuni.cz
Edita Máčajová
Affiliation:
(Máčajová) Department of Computer Science, Faculty of Mathematics, Physics and Informatics, Comenius University, Bratislava, Slovakia, e-mail: macajova@dcs.fmph.uniba.sk
Attila Pór
Affiliation:
(Sereni) CNRS (LIAFA, Université Denis Diderot), Paris, France and Department of Applied Mathematics and Theoretical Computer Science, Faculty of Mathematics and Physics, Charles University, Prague, Czech Republic, e-mail: por@kam.mff.cuni.cz, sereni@kam.mff.cuni.cz
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

It is known that a Steiner triple system is projective if and only if it does not contain the four-triple configuration 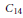${{C}_{14}}$. We find three configurations such that a Steiner triple system is affine if and only if it does not contain one of these configurations. Similarly, we characterise Hall triple systems using two forbidden configurations.

Our characterisations have several interesting corollaries in the area of edge-colourings of graphs. A cubic graph $G$ is $S$-edge-colourable for a Steiner triple system $S$ if its edges can be coloured with points of $S$ in such a way that the points assigned to three edges sharing a vertex form a triple in $S$. Among others, we show that all cubic graphs are $S$-edge-colourable for every non-projective non-affine point-transitive Steiner triple system $S$.

Keywords

05B0705C15
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 62 , Issue 2 , 01 April 2010 , pp. 355 - 381
Copyright
Copyright © Canadian Mathematical Society 2010

References

[1] Archdeacon, D., Generalizations of Tait coloring cubic graphs. In: Problems in topological graph theory. http://www.emba.uvm.edu/\-﹛﹜archdeac/problems/problems.html.Google Scholar
[2] Colbourn, C. J. and Rosa, A.. Triple Systems. Oxford University Press, New York, 1999.Google Scholar
[3] Fu, H.-L., A generalization of Tait coloring cubic graphs. Bull. Inst. Combin. Appl. 31(2001), 45–49.Google Scholar
[4] Grannell, M. J., Griggs, T. S., Knor, M., and Škoviera, M., A Steiner triple system which colours all cubic graphs. J. Graph Theory 46(2004), no. 1, 15–24. doi:10.1002/jgt.10166Google Scholar
[5] Grannell, M. J., Griggs, T. S., and Mendelsohn, E., A small basis for four-line configurations in Steiner triple systems. J. Combin. Des. 3(1995), no. 1, 51–59. doi:10.1002/jcd.3180030107Google Scholar
[6] Hall, M., Jr., Automorphisms of Steiner triple systems. IB M J. Res. Develop. 4(1960), 460–472.Google Scholar
[7] Holroyd, F. C. and Škoviera, M., Colouring of cubic graphs by Steiner triple systems. J. Combin. Theory Ser. B. 91(2004), no. 1, 57–66. doi:10.1016/j.jctb.2003.10.003Google Scholar
[8] Král’, D., Máčajová, E., Pangrác, O., Raspaud, A., Sereni, J.-S., and Škoviera, M., Projective, affine and abelian colorings of cubic graphs. European J. Combin. 30(2009), no. 1, 53–69. doi:10.1016/j.ejc.2007.11.029Google Scholar
[9] Kaiser, T., Král’, D., and Norine, S., Unions of perfect matchings in cubic graphs. In: Topics in Discrete Mathematics. Algorithms Combin. 26. Springer, Berlin, 2006, pp. 225–230.Google Scholar
[10] Pál, D. and Škoviera, M., Colouring cubic graphs by small Steiner triple systems. Graphs Combin. 23(2007), no. 2, 217–228. doi:10.1007/s00373-007-0696-1Google Scholar
[11] Stinson, D. R. and Y. J.Wei, Some results on quadrilaterals in Steiner triple systems. Discrete Math. 105(1992), no. 1-3, 207–219. doi:10.1016/0012-365X(92)90143-4Google Scholar
[12] Teirlinck, L.. On linear spaces in which every plane is either projective or affine. Geom. Dedicata 4(1975), no. 1, 39–44.Google Scholar
[13] Vizing, V. G., Colouring the vertices of a graph with prescribed colours. (Russian) Metody Diskretnogo Analiza Teorii Kodov i Skhem 29(1976), 3–10, 101.Google Scholar