Article contents
The Frequency of Elliptic Curve Groups over Prime Finite Fields
Published online by Cambridge University Press: 20 November 2018
Abstract
Letting 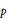 $p$ vary over all primes and
$p$ vary over all primes and 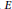 $E$ vary over all elliptic curves over the finite field
$E$ vary over all elliptic curves over the finite field  ${{\mathbb{F}}_{p}}$ , we study the frequency to which a given group
${{\mathbb{F}}_{p}}$ , we study the frequency to which a given group 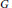 $G$ arises as a group of points
$G$ arises as a group of points 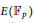 $E\left( {{\mathbb{F}}_{p}} \right)$ . It is well known that the only permissible groups are of the form
$E\left( {{\mathbb{F}}_{p}} \right)$ . It is well known that the only permissible groups are of the form 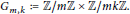 ${{G}_{m,\,k}}\,:=\,\mathbb{Z}\,/m\mathbb{Z}\,\times \,\mathbb{Z}/mk\mathbb{Z}$ . Given such a candidate group, we let
${{G}_{m,\,k}}\,:=\,\mathbb{Z}\,/m\mathbb{Z}\,\times \,\mathbb{Z}/mk\mathbb{Z}$ . Given such a candidate group, we let 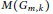 $M\left( {{G}_{m,\,k}} \right)$ be the frequency to which the group
$M\left( {{G}_{m,\,k}} \right)$ be the frequency to which the group 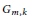 ${{G}_{m,\,k}}$ arises in this way. Previously, C.David and E. Smith determined an asymptotic formula for
${{G}_{m,\,k}}$ arises in this way. Previously, C.David and E. Smith determined an asymptotic formula for 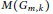 $M\left( {{G}_{m,\,k}} \right)$ assuming a conjecture about primes in short arithmetic progressions. In this paper, we prove several unconditional bounds for
$M\left( {{G}_{m,\,k}} \right)$ assuming a conjecture about primes in short arithmetic progressions. In this paper, we prove several unconditional bounds for 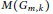 $M\left( {{G}_{m,\,k}} \right)$ , pointwise and on average. In particular, we show that
$M\left( {{G}_{m,\,k}} \right)$ , pointwise and on average. In particular, we show that 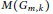 $M\left( {{G}_{m,\,k}} \right)$ is bounded above by a constant multiple of the expected quantity when
$M\left( {{G}_{m,\,k}} \right)$ is bounded above by a constant multiple of the expected quantity when 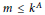 $m\,\le \,{{k}^{A}}$ and that the conjectured asymptotic for
$m\,\le \,{{k}^{A}}$ and that the conjectured asymptotic for 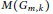 $M\left( {{G}_{m,\,k}} \right)$ holds for almost all groups
$M\left( {{G}_{m,\,k}} \right)$ holds for almost all groups 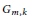 ${{G}_{m,\,k}}$ when
${{G}_{m,\,k}}$ when 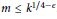 $m\,\le \,{{k}^{1/4-\in }}$ . We also apply our methods to study the frequency to which a given integer
$m\,\le \,{{k}^{1/4-\in }}$ . We also apply our methods to study the frequency to which a given integer  $N$ arises as a group order
$N$ arises as a group order  $\#E\left( {{\mathbb{F}}_{p}} \right)$ .
$\#E\left( {{\mathbb{F}}_{p}} \right)$ .
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2016
References
- 6
- Cited by

