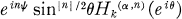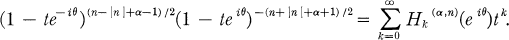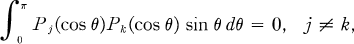Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gasper, George
1981.
Summation Formulas for Basic Hypergeometric Series.
SIAM Journal on Mathematical Analysis,
Vol. 12,
Issue. 2,
p.
196.
Dunkl, Charles F.
1984.
The Poisson kernel for Heisenberg polynomials on the disk.
Mathematische Zeitschrift,
Vol. 187,
Issue. 4,
p.
527.
Temme, N. M.
1986.
Uniform asymptotic expansion for a class of polynomials biorthogonal on the unit circle.
Constructive Approximation,
Vol. 2,
Issue. 1,
p.
369.
Hendriksen, E.
and
van Rossum, H.
1986.
Orthogonal Laurent polynomials.
Indagationes Mathematicae (Proceedings),
Vol. 89,
Issue. 1,
p.
17.
Ranga, A.
2010.
Szegő polynomials from hypergeometric functions.
Proceedings of the American Mathematical Society,
Vol. 138,
Issue. 12,
p.
4259.
Wei, Chuanan
2016.
Minton-Karlsson identities and summation formulae involving generalized harmonic numbers.
Integral Transforms and Special Functions,
Vol. 27,
Issue. 7,
p.
592.
Castillo, Kenier
2022.
Markov’s Theorem for Weight Functions on the Unit Circle.
Constructive Approximation,
Vol. 55,
Issue. 2,
p.
605.
Chen, Dandan
and
Liu, Zhiguo
2025.
New proofs of theorems on q-orthogonal functions.
International Journal of Number Theory,
Vol. 21,
Issue. 02,
p.
473.