Article contents
Rigidity and Height Bounds for Certain Post-critically Finite Endomorphisms of ℙN
Published online by Cambridge University Press: 20 November 2018
Abstract
The morphism 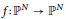 $f\,:\,{{\mathbb{P}}^{N}}\,\to \,{{\mathbb{P}}^{N}}$ is called post-critically finite
$f\,:\,{{\mathbb{P}}^{N}}\,\to \,{{\mathbb{P}}^{N}}$ is called post-critically finite  $\left( \text{PCF} \right)$ if the forward image of the critical locus, under iteration of
$\left( \text{PCF} \right)$ if the forward image of the critical locus, under iteration of 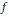 $f$ , has algebraic support. In the case
$f$ , has algebraic support. In the case  $N\,=\,1$ , a result of Thurston implies that there are no algebraic families of PCF morphisms, other than a well-understood exceptional class known as the flexible Lattés maps. A related arithmetic result states that the set of PCF morphisms corresponds to a set of bounded height in the moduli space of univariate rational functions. We prove corresponding results for a certain subclass of the regular polynomial endomorphisms of
$N\,=\,1$ , a result of Thurston implies that there are no algebraic families of PCF morphisms, other than a well-understood exceptional class known as the flexible Lattés maps. A related arithmetic result states that the set of PCF morphisms corresponds to a set of bounded height in the moduli space of univariate rational functions. We prove corresponding results for a certain subclass of the regular polynomial endomorphisms of 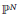 ${{\mathbb{P}}^{N}}$ for any
${{\mathbb{P}}^{N}}$ for any 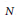 $N$ .
$N$ .
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2016
References
- 4
- Cited by

