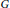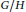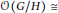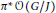Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Al-Abdallah, A. R.
and
Gilligan, B.
2021.
Compact homogeneous Leviflat CR-manifolds.
Complex Analysis and its Synergies,
Vol. 7,
Issue. 3-4,